Swaminathan N., Bray K.N.C. (eds.) Turbulent Premixed Flames

Подождите немного. Документ загружается.

2.4 Transported Probability Density Function Methods 113

Table 2.2. Mixing model and turbulent transport closure

effects on computed u

(m/s) using the ‘standard’

constant-time-scale ratio shown in Eq. (2.152)

PDF transport PDF transport

Mixing model via Eq. (2.147)viaEq.(2.149)

Binomial Langevin [250] 1.24 1.62

Binomial sampling [253] 0.75 1.39

Modiﬁed Curl’s [254 ] 1.32 1.65

LMSE [252] 0.54 0.84

Note: Experimental data suggest that u

≈ 2m/s(seeFig.2.21)

for the conditions considered (u

rms

= 1m/s, = 20 mm, C

= 2,

and u

= 0.385 m/s).

asymptotically approaches that of the standard time-scale expression at high u

rms



ratios as u



→ 0. Comparisons as Da → 0 are clearly not meaningful as ﬁnite-

rate chemistry effects become increasingly important, and an accurate evaluation of

turbulent burning velocities would require a more comprehensive representation of

the chemistry. Furthermore, it can be expected that the coupling between chemical

and diffusion processes will become weaker. The impact of different SDR models

on the corresponding predictions of turbulent burning velocities in the context of

moment-based methods was further assessed in Section 2.3.

2.4.7 The Impact of Molecular-Mixing Terms

A common feature of transported PDF methods within a single-point single-time

context is that modelling approximations are required to account for mixing pro-

cesses, as previously discussed in the context of Eq. (2.156). Mixing essentially

amounts to transport of the PDF in composition space. Lindstedt and V

aos [251]

considered the classical LMSE model (see [252]) and nonlinear integral mod-

els [253, 254]. More sophisticated approaches covered include the binomial Langevin

model [250], originally proposed to simulate Brownian motion, which can readily be

recovered from the Fokker–Planck equation for the special case in which the drift

coefﬁcient is a linear function of the state variable and the diffusion coefﬁcient is

constant.

Results have been obtained through the use of gradient-diffusion (Eq. 2.147)

and second-moment-based (Eq. 2.149) closures for the turbulent transport of the

PDF in physical space. The former is based on the classical eddy-diffusivity–two-

equation-type turbulence model and the latter features the GLM coupled with a

LMSE-type formulation for the conditional turbulence intensity terms in the PDF

equation, as shown in Eq. (2.149). The applied thermochemistry was identical to that

previously presented with the instantaneous source term extracted from a calculation

of an unstrained CH

–air ﬂame. Results suggest that integral properties, such as the

turbulent burning velocity, are affected by the choice of mixing model and the closure

for the transport of the PDF in physical space as shown in Table 2.2.

The absolute values of the predicted turbulent burning velocities are obviously

affected by the chosen conditions. However, the relative inﬂuence of the mixing and

turbulent transport closures can be expected to be reasonably general. Under all

conditions a gradient-diffusion closure results in reduced burning velocities. This

114 Modelling Methods

0.0 0.2 0.4 0.6 0.8 1.0

0.0

0.5

1.0

Normalised Reaction Rate

Figure 2.22. Predictions of normalised

mean-reaction-rate proﬁles in scalar space

by use of a second-moment closure [251].

() Measurements of Cheng and Shep-

herd [35]; (—) binomial Langevin model;

(– ·–) modiﬁed Curl’s model; (−−) bino-

mial sampling model.

observation, combined with the unambiguous manner in which PDF methods allow

the incorporation of scalar reaction rates, leads to further concerns regarding the ef-

fectiveness of gradient-diffusion closures for premixed turbulent ﬂames. An example

of the predictive capability of the more accurate models is shown in Fig. 2.22.The

same approach was also applied to the computation of a 2D slightly lean CH

–air

(φ = 0.9) ﬂame stabilised in an opposed jet geometry, as investigated experimentally

by Mastorakos [289]. The conﬁguration is identical to that studied by Lindstedt and

aos [17], who used a moment method. The calculation procedure featured a ﬁnite-

volume part for the integration of the momentum and the second-moment equations

and a Monte Carlo part for the integration of the scalar PDF. An average number

of 400 weighted Lagrangian particles per cell was used.

The application of the standard expression for the scalar time scale [see

Eq. (2.152)] results in a failure to stabilise the ﬂame. The behaviour belies a problem

consistent with the preceding discussion. Accordingly, variations in the turbulent

transport and mixing model closures were found to be ineffective and yield either

the same result (modiﬁed Curl’s model) or more rapid extinction (all other mixing

models and gradient-diffusion closures). Jones and Prasetyo [291] also performed a

transported PDF study of the ﬂame considered here – using a gradient-diffusion clo-

sure and a global four-step scheme – and they found that, although the LMSE model

resulted in ﬂame extinction, the modiﬁed Curl’s model yielded accurate predictions

with respect to the ﬂame location. However, the time-scale ratio had to be signif-

icantly increased to promote ﬂame stabilization. Inspection of Figs. 2.23 and 2.24

reveals that application of the extended time-scale expression shown in Eq. (2.155)

results in reasonable predictions. Higher values for C

, C

∗

, or both, would cause the

ﬂame to stabilise farther upstream.

2.4.8 Closure of Pressure Terms

The balance equation for the Favre-averaged kinetic energy of turbulence in a high-

ﬂow can, for a 1D steady-state ﬂame, be written as





u



=−









−





−







2

du

− u



+ p



∂u



∂x

−





ε. (2.157)

2.4 Transported Probability Density Function Methods 115

0246810

Distance alon

centreline

(

)

0.0

0.5

1.0

u / u

Figure 2.23. Predicted normalised axial velocity (u/u

) proﬁles along the centreline [291].

Symbols: (◦) experimental data by Mastorakos [289]. Lines: (—) presumed PDF approach

using the fractal reaction-rate model implied by Eq. 2.155;(−−) transported PDF approach

using the binomial Langevin model and the scalar time-scale expression of Eq. (2.155). In

both cases a full second-moment closure is adopted for the turbulent transport of scalar and

momentum. Velocity normalised by burner exit velocity on centreline (u

The ﬁfth term on the right-hand side of Eq. (2.157) describes pressure–dilatation,

which is zero in constant-density turbulence and is usually neglected in models of

turbulent combustion. However, Zhang and Rutland [292] performed a DNS of

planar premixed turbulent ﬂames propagating upstream in a decaying turbulence

ﬁeld at relatively low (0.67 and 1.5) expansion ratios τ (= ρ

/ρ

− 1) and found that

pressure–dilatation is dominant under such conditions. The value of the term cannot

be extracted from a PDF calculation at the current closure level, though a modelled

term may readily be formulated to estimate the magnitude.

In the ﬂamelet regime of combustion, the preceding term is non-zero only within

a ﬂamelet. If it is assumed that the latter has a structure close to that of a laminar

ﬂame, then the expected value of the term conditioned upon the ﬂuid being within

the ﬂamelet can be estimated by integrating an expression based on the momentum

0246810

Distance along centreline (mm)

0.0

0.2

0.4

0.6

0.8

1.0

Figure 2.24. Predicted progress variable (



c) proﬁle along the centreline [291]. Lines, symbols,

and closures are as in Figure 2.23.

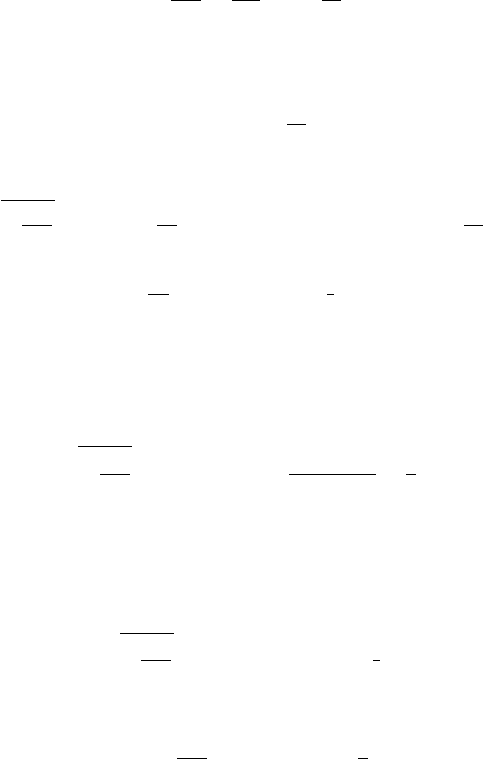
116 Modelling Methods

equation. From mass conservation it follows that

∂u

∂x

∂u

∂ζ

= u

∂c

∂ζ

, (2.158)

where ζ is the coordinate normal to the laminar ﬂame. If viscous forces are neglected

in the momentum equation, the pressure can be obtained as [283]

c=c

∗

≈ p |

c→0



ζ|

c=c

∗

ζ|

c→0



−ρ

τu

∂c

∂ζ



dζ = p|

c→0

− ρ

τu

∗

, (2.159)

and it follows that





∂u

∂x



ﬂamelet





ζ|

c→1

ζ|

c→0





c→0

− ρ

τu

c(ζ)



∂c

∂ζ

dζ



$



|c → 0



−

, (2.160)

where δ

= ζ|

c→1

− ζ|

c→0

is the thickness of the ﬂamelet. If the volume fraction

of ﬂamelets within the turbulent ﬂame brush is estimated by use of the expected

surface-to-volume ratio of the ﬂame sheet as δ

, the following expression for the

pressure–dilatation term is obtained:



∂u



∂x

= ρ

(u

τ)







|c → 0



−



. (2.161)

To form a model, an approximation has to be found for the conditional expecta-

tion





|c → 0



. Neglecting viscous dissipation and introducing the simple assumption





|c → 0







|c = 0



, which ignores any pressure rise ahead of the ﬂamelet, the

following expression is obtained [246]:



∂u



∂x

= ρ

(u

τ)





−

. (2.162)

Zhang and Rutland [292] proposed an alternative expression:





∂u



∂x



= ρ

(u

τ)



. (2.163)

A PDF solution may be used to analyse the budget of Eq. (2.157) and to compare

the magnitude of the known terms with the preceding models for pressure–dilatation.

For the purpose oforder-of-magnitude estimates, the surface-to-volume ratio can be

obtained as  =







S/(ρ

)[147], with the mean reaction rate



S obtained from the

PDF solution.

The enhancement of turbulence in the turbulent ﬂame structure is exempliﬁed

in Figs. 2.25 and 2.26. Generally, the higher the value u

rms

, the stronger the turbu-

lence generation in the ﬂame. This applies to the cases of decreasing u

rms

(Fig. 2.25)

and increasing integral length scale ( Fig. 2.26). As shown later, the dominant turbu-

lent production term is the pressure–work term [the fourth term on the right-hand

side of Eq. (2.157)]. The mean turbulent scalar ﬂux and its scaling with the turbulence

intensity and the integral length scale is shown in Figs. 2.27 and 2.28. The fact that

counter-gradient transport prevails in most of the ﬂame brush for the current planar

ﬂames, except at the ﬂame t ip [7, 66], suggests that gradient-based models for the

2.4 Transported Probability Density Function Methods 117

0.0 0.2 0.4

0.6

0.8 1.0

0.0

1.0

2.0

3.0

4.0

k / k

Figure 2.25. Proﬁles of the kinetic energy of turbulence, normalised with its upstream value,

as functions of the reaction progress variable [246]. The integral length scale of turbulence is

 = 40 mm, and the proﬁles are plotted for u

rms

values of 1, 2.5, and 10 (top) m/s. Comparisons

for CH

–air ﬂames obtained with (no symbols) the Haworth and Pope [248, 264] and (lines

with symbols) the Lagrangian [265] variant of the Speziale et al. [249] closure for velocity

statistics.

correlation





are inadequate. Results shown in Figs. 2.27 and 2.28 suggest that

the width of the region of gradient transport at the cold front (which is responsible

for the propagation of the ﬂame front and thus determines the burning velocity)

increases for larger u

rms

and smaller . A budget of the terms in the



k balance

equation (2.157) for a steady ﬂame is presented in Fig. 2.29. Production is mainly

by means of the pressure–work term. The main sink term is the third term on the

right-hand side of Eq. (2.157) – traditionally called the ‘turbulence production term’

in constant-density ﬂows.

0.0 0.2 0.4

0.6

0.8 1.0

0.0

1.0

2.0

3.0

4.0

k / k

Figure 2.26. Proﬁles of the kinetic energy of turbulence, normalised with its upstream value,

as functions of the reaction progress variable [246]. The upstream turbulence intensity is

rms

= 1ms

−1

, and the proﬁles are plotted for  = 10, 20 and 40 (top) mm. Lines and symbols

are as in Fig. 2.25.

118 Modelling Methods

0.0 0.2 0.4

0.6

0.8 1.0

0.00

0.10

0.20

0.30

0.40

0.50

c"u" / u

Figure 2.27. Proﬁles of the velocity–scalar correlation





, normalised with the turbulent

burning velocity u

, as functions of the reaction progress variable [246]. The dependency of

the values on u

rms

is shown for a ﬁxed  = 40 mm. Lines and symbols are as in Fig. 2.25.

The convection, destruction, and generation terms in Fig. 2.29 show a behaviour

similar to that obtained by Bradley et al. [228], who used a presumed β-PDF ap-

proach closed at the second-moment level. However, other features show qualitative

differences [246]. This is not surprising as a turbulent scalar ﬂux (





) consistent

with both scalar and velocity ﬁelds is obtained naturally in a PDF solution, whereas

in moment methods very little information is known about important terms in the

scalar ﬂux equation (e.g., correlations between ﬂuctuating velocity components and

chemical source terms) such as the





S term. The models for the pressure–dilatation

term [Eqs. (2.162) and (2.163)] are also shown, and with the current high expan-

sion ratio the term does not dominate the budget. However, models of the type

of Eqs. (2.162) and (2.163) are fairly easy to implement in a



k equation. Further

discussion on the subject in the context of moment-based methods can be found in

0.0 0.2 0.4

0.6

0.8 1.0

0.00

0.10

0.20

0.30

0.40

0.50

c"u"/ u

Figure 2.28. Proﬁles of the velocity–scalar correlation





, normalised with the turbulent

burning velocity, as functions of the reaction progress variable [246]. The dependency of the

values on  is shown for a ﬁxed u

rms

= 1ms

−1

. Lines and symbols are as in Fig. 2.26.

2.4 Transported Probability Density Function Methods 119

0.0 0.2 0.4

0.6

0.8 1.0

-60

-40

-20

(kg m

–1

–3

)

Figure 2.29. Budget of the terms in



k equation (2.157)foru

rms

= 1ms

−1

and  = 20 mm

and a stoichiometric methane–air ﬂame [246]. The extracted values are (—) convection,

−





u



k/dx

;(···) diffusion, 1 − 0.5d







3

/dx

; (- - -) diffusion, 2 −d









2

/dx

;

(– –) destruction, −







2

du

/dx

;(· –) pressure work, −











/dx

. Pressure–dilatation

models: Eq. (2.162) (square and solid line) and Eq. (2.163) (square and dotted line).

the studies by Lindstedt and V

aos [17], Domingo and Bray [18], and Lipatnikov and

Chomiak [293].

The effect of adding an approximation of the combustion-induced pressure

gradient to the pressure-acceleration term driving stochastic particles in a PDF

simulation was investigated by H

ulek and Lindstedt [266], and Eq. (2.150) becomes

(p )



−

∂p

∂x



(p )

+ G

k

(p )



−u



)



dt +(C

ε)

1/2

, (2.164)

where

∂p

∂x



(p )

∂





∂x



ρ u

(p )

− u







. (2.165)

In Eq. (2.165) n

= n is the normal vector of the ﬂame sheet. The expression for

the pressure gradient [(2.165)] was formulated so as to preserve the mean pressure

gradient value and thus the overall momentum. The last term on the right-hand side

of (2.165) may be interpreted as a ‘zero-order approximation’ of the dispersion of

the ﬂamelet-induced pressure gradients in the non-reacting ﬂuid.

The Lagrangian time derivative Dc

(p )

/Dt in Eq. (2.165) is obtained naturally

as part of the scalar statistics solution. The statistics of n was obtained with the

suggestion by Cant et al. [206],





=−







, (2.166)

where  is the surface-to-volume ratio of the ﬂame sheet. The PDF of the n compo-

nent normal to the turbulent ﬂame brush was approximated with a linear combina-

tion of a δ peak and a uniform PDF. The distribution of the components parallel to

the turbulent ﬂame was assumed to be isotropic, and their PDF then follows from the

120 Modelling Methods

0.0 0.2 0.4

0.6

0.8 1.0

-0.1

0.0

0.1

0.2

0.3

u" c" u

–1

Figure 2.30. Comparison of PDF predictions of the scalar–velocity correlation [266] obtained

with (—) and without (- - -) the ﬂamelet-acceleration model of Eq. (2.165) and the DNS data

(···) of Rutland and Cant [43].

condition |n|=1. Equation (2.166) involves division with surface-to-volume density

 {again estimated as  ≈







S/(ρ

)[147]}. The mean source term



S approaches

zero at both the hot and cold ends of the ﬂame, and the value estimated from a PDF

simulation contains statistical errors. The singularity at the limits



c → 0 and



c → 1

was overcome by bounding the obtained values for





between 0 and 1.

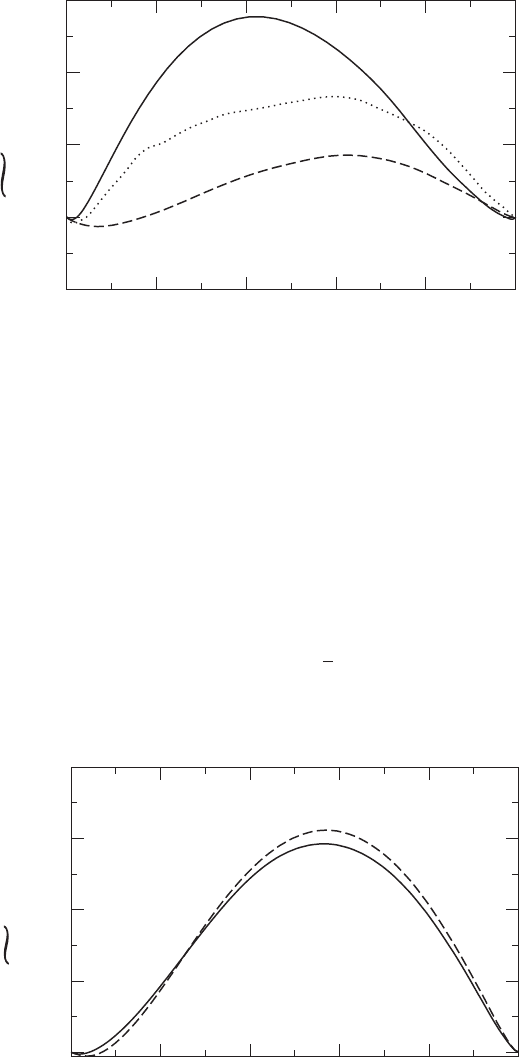
Two cases of planar premixed turbulent ﬂame propagation are shown in Figs. 2.30

and 2.31. The ﬁrst is a ﬂame in a decaying turbulence ﬁeld as solved with a DNS

by Rutland and Cant [43]. The ﬂame has an expansion ratio of τ = 2.3, an in-

let turbulence intensity of u

rms

√

, and a turbulence Reynolds number of

= 56.7. Because of the unstable nature of the ﬂame, transient effects exert

0.0 0.2 0.4

0.6

0.8 1.0

0.0

0.5

1.0

1.5

2.0

u"c" u

–1

Figure 2.31. Comparison of PDF predictions of the scalar–velocity correlation in a planar

premixed steady-state turbulent ﬂame [266]. Stoichiometric methane–air mixture with an

upstream turbulence intensity u

rms

= 2.5ms

−1

and an integral length scale  = 40 mm. Lines

as in Fig. 2.30.

2.4 Transported Probability Density Function Methods 121

some inﬂuence. Nevertheless, the DNS data are expected to provide some guid-

ance. The results obtained show that the joint velocity–scalar statistics (in terms of

moments like







ρ u











ρ u





) certainly are affected by the model. For

example, Fig. 2.30 shows comparisons of the quantity





against



c. The sec-

ond case is a stoichiometric methane–air ﬂame (τ ≈ 7 and laminar burning velocity



= 0.386 m s

−1

). The case shown here features u

rms

= 2.5ms

−1

and  = 40 mm.

The upstream Reynolds number of turbulence is Re

≈ 6496 (based on the integral

length scale ). Results shown in Fig. 2.31 suggest that, at higher expansion ratios

and with u

rms

signiﬁcantly higher than u



, the inﬂuence of the model is very small.

This indicates that for more strongly turbulent ﬂames ‘ﬂamelet acceleration’ is not

necessarily an important mechanism. This ﬁnding is not surprising in view of the scal-

ing characteristics of the pressure gradient terms in Eq. (2.165). The mean pressure

gradient can for a steady-state planar ﬂame be obtained from the mean momentum

equation [66]:

=−







2

− ρ



, (2.167)

where the second term on the right-hand side is dominant. Therefore the mean

pressure gradient in Eq. (2.165) scales in a steady-state turbulent ﬂame with u

where u

is roughly proportional to u

rms

. At the same time, the current model term

in Eq. (2.165) scales effectively with u

dζ

≈−ρ u

=−ρ

dζ

. (2.168)

Overall, the relative importance of ﬂamelet acceleration can vary by orders of mag-

nitude, depending on the ratio u

(or u

rms

). The effect may thus be important

for only a s ubrange of turbulent conditions. However, some caution is required with

respect to the current analysis, as Vervisch et al. [294] showed by means of DNS

that the ﬂamelet approximation breaks down close to the scalar bounds (c → 0 and

c → 1).

2.4.9 Premixed Flames at High Reynolds Numbers

The strongly piloted high-Reynolds-number premixed turbulent ﬂames investigated

experimentally by Chen et al. [256] present challenges related to the conﬁguration,

as outlined by Pitsch and de Lageneste [76] and Lindstedt and V

aos [211]. Heat

losses to the burner surface were estimated to reach up to 20%, and the close

proximity of the ﬂame to the burner plate was expected to inﬂuence the scalar time-

scale r atio. The role of entrainment was also highlighted. However, the data sets

include a case featuring Da ≈ 1 and permit the further evaluation of the inﬂuence

of modelling parameters. The inﬂuence of molecular transport in physical space can

be expected to be reduced at lower Damk

ohler numbers [see Eq. (2.156)] as the

coupling between molecular transport and chemical reaction inherent in the laminar

ﬂamelet assumption will weaken.

The applied calculation procedure featured enthaply as a solved scalar that per-

mits arbitrary enthalpies to be set for the different reactant streams. Computations

featuring a systematic variation of the time-scale ratio (2 ≤ C

≤ 8) were performed

122 Modelling Methods

0.2

0.4

0.6

0.8

0.2

0.4

0.6

0.8

0.2

0.4

0.6

0.8

x75(%)

0.2

0.4

0.6

0.8

0.50

r/D

0.2

0.4

0.6

0.8

0.5

r/D

x/D=10.5

x/D=8.5

x/D=6.5

x/D=4.5

x/D=2.5

Figure 2.32. Mean temperature and OH mass fraction for a stoichiometric CH

/–air ﬂame

at Re = 52 500 [211]. Experiments: (◦) Chen et al. [256], transported PDF calculations with

modiﬁed Curl’s model [ 254]andEq.(2.155) with C

∗

= 0andT

= 2005 K. C

= 2(···), 4

(– · –), 6 (– ·· –), and 8 (—).

to illustrate sensitivities. The inﬂuence of the time-scale ratio was shown to be modest

for stably burning mixtures and signiﬁcantly close to the extinction of non-premixed

piloted diffusion ﬂames [295]. The sensitivity is exempliﬁed here in the context of a

premixed turbulent ﬂame close to global extinction. The corresponding joint com-

position PDF in low-Mach-number ﬂows is considered with the thermochemistry

described by up to 12 independent scalars and enthalpy. The applied augmented

systematically reduced chemistry is identical to that of previous studies [234](with

the nitrogen-containing species omitted) and features 15 solved species (H, O, OH,

O, H

,CH

,CO,CO

, and N

). Steady-

state approximations were applied to 14 species (C, CH,

,CHO,CH

OH, CH

O, C

H, C

HO, and CH

CO). The chemical source

term was computed by means of a direct integration technique featuring a Newton

method with the Jacobian evaluated analytically. The mean mass and momentum

conservation equations were solved in a similarity transformed coordinate system in

a manner similar to that of Lindstedt et al. [233, 295]. The Reynolds stresses were

obtained through the second-moment closure of Speziale et al. [249]. The applied

closure level does not account for non-gradient transport. However, it has the ben-

eﬁt of relative simplicity and constitutes the natural starting point for the further

investigation of premixed turbulent ﬂames featuring comprehensive closures for the

chemical source term.

Results were obtained with a pilot temperature of T

= 2005 K, the standard

closure model given in Eq. (2.152), and with the time-scale ratio varied in the range

2 ≤ C

≤ 8. The effects of the time-scale ratio on the temperature-based reaction

progress variable [

c = (T − T

)/(T

− T

) and T

= 2248] [256] and OH concen-

tration are shown in Fig. 2.32. A value of C

= 2 leads to extinction shortly after

x/D = 2.5 and C

= 4 shows strong indications of blowoff farther downstream. The