Swaminathan N., Bray K.N.C. (eds.) Turbulent Premixed Flames
Подождите немного. Документ загружается.

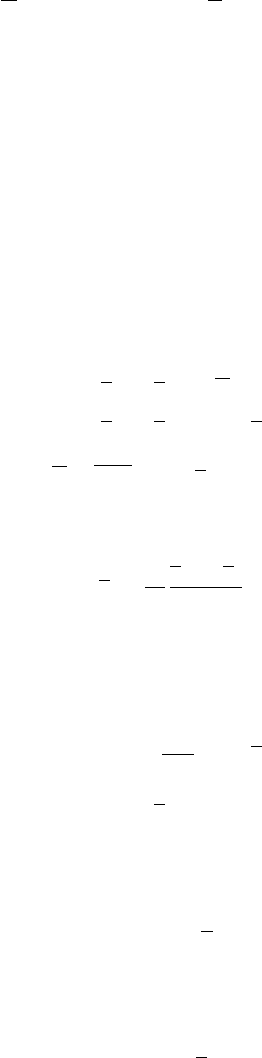
2.2 Flame Surface Density and the G Equation 73
The FSD may be obtained by the solution of Eq. (2.60), thus ensuring a full
coupling between FSD and detailed chemistry tabulation, the species mass fraction
being approximated as
Y
i
(x, t) =
1
0
Y
MFM
i
(ϕ
∗
)P(ϕ
∗
; x, t)dϕ
∗
, (2.80)
where Y
MFM
i
(ϕ
∗
) denotes the low-dimensional manifold trajectory relating the
progress variable to the ith species and obtained with Eqs. (2.69) and (2.70). The
progress-variable source term is, however, preferably obtained from relation (2.52)
to ensure correct flame propagation [133].
With this simple procedure, the FSD modeling is enriched with the possibility
of addressing pollutants emissions, and the presumed PDF approach is improved
because it accounts for the existence of a thin-flame and propagating-flame interface.
RELATION BETWEEN SGS VARIANCE AND SCALAR GRADIENT. The specific premixed-
type -PDF is useful to explore SGS scalar variance behaviour. Relation (2.75)
suggests that
ϕ
v
= ϕ(1 − ϕ) − δ
ϕ
=
ϕ(1 −ϕ) − δ
ϕ
|∇ϕ|, (2.81)
where the generalized FSD
= |∇ϕ|=|∇ϕ| is expressed with the magnitude of
the resolved gradient, thanks to the wrinkling factor .
Experimental results have shown that the following relation holds [134]:
|∇
ϕ|=
1
C
s
ϕ(1 −ϕ)
, (2.82)
where C
s
is a model constant and is the characteristic length of the LES filter. From
the previous relations, the link between the SGS scalar variance and the resolved
gradient reads
ϕ
v
∝
C
s
−
δ
ϕ
|∇
ϕ|. (2.83)
The SGS variance then scales as |∇
ϕ|, a relation that is in line with results
from experimental measurements [134] and the method of manufactured solution
(MMS) [135], which reported that the time average of the SGS variance was indeed
scaling as
ϕ
v
∝
|∇
ϕ|
, (2.84)
where is the LES filter size and the angle brackets
·
denote time averaging.
Notice that this scaling differs from the usual one obtained from a chemically frozen
flow production–dissipation equilibrium hypothesis of the SGS variance [132, 136],
which leads to the quadratic law
ϕ
v
(|∇
ϕ|)
2
, but was not observed after space
filtering of both experiments [134] and MMS [135].
74 Modelling Methods
2.2.5 Conclusion
Turbulent premixed flames are easily identified as surfaces separating fresh and burnt
gases. The FSD and field equation (or G equation) were developed to collect infor-
mation on the behaviour of a given isoscalar surface, or a collection of isosurfaces in
the case of the generalized FSD. These quantities provide a generic framework for
developing flame modelling that reproduces basic premixed flame properties as the
response of their propagation speed and characteristic thickness to various chemical
and turbulence parameters.
In future highly resolved simulations, aside from the mandatory accurate nu-
merics and high performance of the computing tools, it is mostly detailed chemistry
tabulation that will actually be important because of it ability to include all effects
related to the complex interaction between reactants and their local environment
before they enter the reaction zone (viz., dilution by hot burnt gases, or mixing with
vitiated air). Within this context, multidimensional flamelets appear as a promising
approach to incorporate more physics into the chemical look-up table, which will
then be more able to reproduce complex flame features observed in the forthcoming
lean premixed flame burners developed to reduce pollutant emissions.
In simulations using coarser meshes, the modelling of unresolved fluctuations
of flame parameters is still the main concern. There, the modelling of thin reaction
zones from FSD or the G equation is of great help to complete closures reproducing
experimentally observed turbulent flame properties.
2.3 Scalar-Dissipation-Rate Approach
By N. Chakraborty, M. Champion, A. Mura, and N. Swaminathan
The objective of turbulent combustion modelling is to seek a way to express the
averaged reaction rate and reactive scalar fluxes in terms of known quantities such
as turbulence length and time scales, usually of the turbulence integral scales, as has
been noted in Chapter 1. The exact form of the relationship depends on the model-
ling approach. In this section, we discuss the mean-reaction-rate modelling using the
SDR and its relationship to other methods presented in this chapter.
The SDR is an important quantity in turbulent combustion. This quantity de-
notes the mixing rate of fuel and oxidiser in turbulent non-premixed flames, whereas
it denotes the rate of mixing of hot and cold fluids on the flame surface required to
sustain combustion in turbulent premixed flames. The instantaneous SDR is defined
as N
c
= ˆα
(
∂c/∂x
k
)(∂c/∂x
k
)
, where c is a reaction progress variable with diffusivity
ˆα. It is clear that the gradient of the reaction progress variable and thus the physical
processes affecting the gradient ∂c/∂x
k
will influence the SDR. This will be eluci-
dated based on strong theoretical foundations later in this section using data from
physical and numerical experiments on t urbulent premixed flames. The SDR is di-
rectly related to the heat release rate in premixed [21, 62] as well as in non-premixed
[124, 137] flames. A wealth of information on the SDR of mixture fraction, which
is a chemically conserved scalar (see Section 1.3.2), is available in the literature
[70, 138, 139]. However, the information on N
c
is limited, but it is growing. One of
the aims of this section is to bring the available information together to show and
discuss the role of the SDR approach and its success in recent studies [140–145].

2.3 Scalar-Dissipation-Rate Approach 75
The progress variable c may be defined using any reactive scalars; a tempera-
ture or fuel mass fraction is commonly used, but alternative choices [146] are also
available. The instantaneous c, irrespective of how it is defined, is governed by
ρ
Dc
Dt
= ˙ω
c
+ D, (2.85)
where ρ is the fluid density and D/Dt is the total or substantial derivative defined by
Eqs. (1.1) in Chapter 1. The first term on the right-hand side, ˙ω
c
, is the chemical source
term, and the second term represents the diffusive flux of c, D ≡ ∂[ρ ˆα∂c/∂x
k
]/∂x
k
.
Here, as in the earlier sections, the repeated indices imply summation over them. In
RANS simulations (the current workhorse for engineering calculations), a balance
equation [see Eq. (1.29)] for the Favre-averaged progress variable
c is solved along
with models for the mean reaction rate
˙ω
c
and the turbulent flux
u
k
c
.IntheLES
approach, the SGS reaction rate and scalar flux are to be modelled (see Chapter 1).
In the following discussion, the primary focus is on the RANS modelling of the SDR
and its relation to mean-reaction-rate closure. The LES modelling of the SDR is
discussed briefly in a later part of this section.
The challenging problem of mean-reaction-rate closure can be simplified to
a tractable form if one assumes the flame scales to be smaller than typical fluid
dynamic (Kolmogorov) scales of relevance [147]. This assumption implies high-
Damk
¨
ohler-number or thin-flamelets combustion (see Section 1.6) and allows the
PDF of c to be approximated as a double-delta function, representing the unburnt
and burnt mixtures [148]. This approximation leads to many attractive simplifications,
as noted in Section 2.1. A couple of relevant points for our discussion here are (1)
the progress-variable variance is given by
c
2
= g
c
(
1 −
c
)
and g is equal to one when
the combustion occurs in thin flamelets and (2) the mean reaction rate in Eq. (1.36)
is rewritten here for convenience as
˙ω
c
=
2
2C
m
− 1
ρ
c
. (2.86)
The Favre-averaged SDR
c
is defined as
c
≡ ρ ˆα
(
∂c
/∂x
k
)(∂c
/∂x
k
)
/
ρ, and it is
one half of the dissipation rate of the progress-variable variance. Modelling of
this dissipation rate with the turbulent time scale alone is insufficient for premixed
flames [142, 149, 150] because of strong coupling among turbulence, chemical, and
molecular-diffusion processes in premixed flames, and an SDR-based approach can
capture this coupling naturally as one will see later in this section.
The mean-reaction-rate model in Eq. (2.86) is for situations in which the turbu-
lent eddies do not enter the reaction zone and they create only large-scale wrinkling
[124, 151]. When the small length and time scales of turbulence are comparable to
flame scales, then the small-scale turbulent eddies can enter the reaction zone and
disturb it, as noted in Section 1.6. For these situations, the burning mode part of the
BM PDF will be non-negligible, and it must be included in the analysis. Bray et al.
[41] showed how to account for these effects by including an additional variable that
quantifies the deviation from the situation of thin flamelets. This additional variable
is related to the Favre variance of the progress variable. Bradley [152] s uggested

76 Modelling Methods
modelling the mean reaction rate by
˙ω
c
=
˙ω
c
(
ζ, ψ
)
P
(
ζ, ψ
)
dζ dψ, (2.87)
where ζ and ψ are the sample space variables for c and the stretch rate κ induced by
the turbulent eddies, respectively. The JPDF
P
(
ζ, ψ
)
is obtained by use of presumed
forms for the marginal PDFs
P
c
(ζ) and P
κ
(ψ) and treating them as statistically
independent. Details of these two approaches are discussed in Section 2.1.The
relevant point for our discussion here is that either of these approaches requires a
solution to the Favre variance equation, given by Eq. (1.34), which requires a model
for the mean SDR,
c
. The reaction-rate contribution, the first term on the right-hand
side of Eq. (1.34), can be closed exactly by use of
c
˙ω
c
= (C
m
−
c) ˙ω
c
, (2.88)
and the last two terms are negligible in large-Reynolds-number premixed flames.
The models for the turbulent fluxes are discussed in Sections 1.7 and 2.1.
If one chooses to avoid the flamelets description to model lean premixed com-
bustion and proposes to employ alternative methods such as transported PDFs [95]
or CMC [96], then again a model for the SDR is r equired, but the SDR must be con-
ditional on the reaction progress variable. Despite some attempts in this direction
[153], it is to be noted that these two approaches have not evolved to the same level
as for the non-premixed flames primarily because of issues surrounding the (condi-
tional) SDR modelling. Recent developments in the JPDFs approach are discussed
in Section 2.4.
Few experimental studies [154–157] have been carried out to explain the be-
haviour of N
c
. These studies, except [157], show that the values of N
c
in turbulent
premixed flames are low compared with unstrained laminar flame values. Analysis
[158] of results from DNS shows an increase in N
c
compared with the laminar flame
values. A reconciliation of these differences is not attempted here, but it is hoped
that the concepts and ideas provided here can help such an investigation.
2.3.1 Interlinks among SDR, FSD, and Mean Reaction Rate
Equation (2.86) clearly shows the direct relationship between the mean reaction rate
and the averaged SDR when the combustion occurs in thin flamelets. Also, for this
situation the average reaction rate may be modelled [147] as the product of mean
reaction rate per unit flame surface area and the FSD, . Thus
˙ω
c
= (ρ
u
S
0
L
I
0
), where
ρ
u
is the unburnt mixture density, S
0
L
is the unstretched laminar burning velocity, and
I
0
represents the effect of flame stretch on the laminar burning velocity. This effect
is captured by means of a correlation involving Karlovitz and Markstein numbers
[159]. It is apparent from the preceding expression that the amplification of reaction
rate by turbulence is predominantly represented by .
Various approaches have been suggested [70] to model . The BML approach
in Section 2.1 yields an algebraic expression for . In an another approach discussed
in detail in Section 2.2, a balance equation f or is solved. This balance equation,
postulated by Marble and Broadwell [89], was rigorously derived by Pope [94] and
Candel and Poinsot [103]. The FSD is related to the SDR by
c
= K
S
L
, where
K
is a constant [160]. Pope [95] deduced that the SDF of an isosurface c = ζ is

2.3 Scalar-Dissipation-Rate Approach 77
p
(ζ; x, t) =
!
N/ ˆα|c = ζ P
c
(ζ) without invoking the assumption of thin flame.
There should not, however, be any local extinction on the surface c = ζ as this would
invalidate the continuity of c on the particular surface. The quantity within the angle
brackets denotes conditional average. The FSD can be obtained from the SDF
p
either by choosing a particular value of ζ or by averaging =
p
dζ [70]. Under
the assumption of thin flamelets, one gets
because the FSD evaluation is not
sensitive to the choice of the c isosurface. In the preceding discussion, one sees that
the SDR is closely related to the FSD and to the reaction rate. The dissipation-
rate-based and FSD-based approaches are closely related to each other, and these
methods have their own merits.
The relevance of SDR for turbulent combustion modelling being established
from the preceding discussion, we now introduce the background works on this
topic. In the forthcoming sections, the transport equation for the SDR is derived
first. The analysis of this equation is taken up next in the order of increasing level of
complexity: non–reactive scalar, reactive with negligible thermal-expansion effects,
and finally the case of premixed flames in which the scalar and velocity fields are
coupled by thermal-expansion effects. Lumley and his co-workers [161–163] derived
a balance equation for the dissipation rate of non-reactive scalar in homogeneous
isotropic turbulence. Borghi and his co-workers [160, 164–166] adapted the approach
for turbulent flows with chemical reactions, which was the first attempt to capture
the effects of chemical reactions on SDR but with a constant-density approximation.
Jones and Musonge [167] included the effects of inhomogeneities in the non-reactive
flow. Swaminathan and Bray [150] included thermal-expansion effects of combus-
tion. In the following subsections, emphasis is placed on the significance of various
processes that would influence the SDR in various situations, and order-of-magnitude
analysis (OMA) is presented in detail for each situation. The strengths and weak-
nesses of OMA are also briefly stressed, thus emphasising the important role of DNS
and laser diagnostic data to investigate such crucial small-scale quantities.
2.3.2 Transport Equation for the SDR
The transport equation for the instantaneous SDR N
c
can be written as
ρ
DN
c
Dt
−
∂
∂x
k
ρ ˆα
∂N
c
∂x
k
=−2ρ ˆα
2
∂
∂x
k
∂c
∂x
2
− 2ρ ˆα
∂c
∂x
k
∂u
k
∂x
∂c
∂x
−2ˆα
˙ω
c
+ D
ρ
∂c
∂x
k
∂ρ
∂x
k
+ 2ˆα
∂ ˙ω
c
∂x
k
∂c
∂x
k
, (2.89)
after Eq. (2.85) is differentiated with respect to x
k
and then multiplied by ˆα∂c/∂x
k
.
The diffusivity ˆα is taken to be weakly dependent on temperature. Following this
procedure and using a transport equation for the Favre-averaged reaction progress
variable
c, a balance equation for ! = ˆα
(
∂
c/∂x
k
)(∂
c/∂x
k
)
can be obtained. Subtract-
ing this balance equation from the Favre-averaged form of Eq. (2.89), one obtains a
transport equation for
c
=
N
c
− !. This equation is written as [150, 168]
∂
ρ
c
∂t
(I)
+
∂u
k
ρ
c
∂x
k
(II)
−
∂
∂x
ρ ˆα
∂
c
∂x
(III)
= (IV) + (IV-b) + (V)
+(VI) + (VII) − (VIII) + (IX) + (X), (2.90)
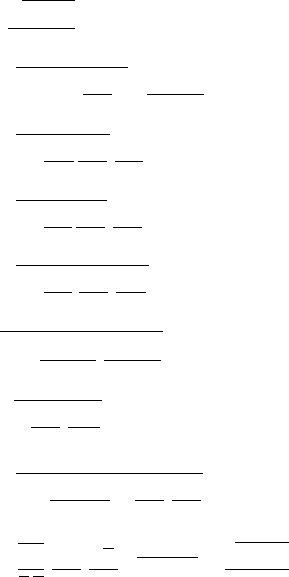
78 Modelling Methods
where
(IV) =−
∂
ρ u
k
c
∂x
k
,
(IV-b) =−2
ρ ˆα u
∂c
∂x
k
∂
2
c
∂x
∂x
k
,
(V) =−2
ρ ˆα
∂u
∂x
k
∂c
∂x
k
∂
c
∂x
,
(VI) =−2
ρ ˆα
∂c
∂x
∂c
∂x
k
∂u
∂x
k
,
(VII) =−2
ρ ˆα
∂c
∂x
∂c
∂x
k
∂u
∂x
k
,
(VIII) = 2
ρ ˆα
2
∂
2
c
∂x
∂x
k
∂
2
c
∂x
∂x
k
,
(IX) = 2
ˆα
∂c
∂x
k
∂ ˙ω
∂x
k
,
(X) =−2
ˆα
˙ω
c
+ D
ρ
∂c
∂x
k
∂ρ
∂x
k
+2
ρ ˆα
ρ ρ
∂
c
∂x
k
∂ρ
∂x
k
˙ω
c
+ D −
∂
ρ u
c
∂x
. (2.91)
The first term on left-hand side of Eq. (2.90) denotes the unsteady evolution,
the second term represents the mean advection, and the third term represents the
molecular diffusion. The various terms on the right-hand side are defined in Eq (2.91)
and the physical meanings of these terms are as follows. The turbulent transport of the
dissipation rate is denoted by (IV) and the influence of curvature of the mean scalar
field is denoted by (IV-b). The turbulence–scalar interaction effects are denoted by
three terms, viz., (V), (VI), and (VII). The influence of the molecular-dissipation
process is denoted by (VIII), which is a positive-definite term. The last two terms
appear specifically for turbulent premixed flames because they involve a chemical
reaction rate. Specifically, the production of scalar gradients by the chemical reactions
is denoted by (IX), and the thermal-expansion (dilatation) effects are denoted by
(X). Equation (2.90) is valid for general premixed combustion in turbulent flows
and it can be simplified for specific cases such as premixed combustion with unity
Lewis number, negligible thermal-expansion effects, etc. Many of the terms in the
preceding transport equation need to be modelled, which is quite challenging, and,
as already noted, the role of DNS on this modelling front is inevitable. Before
studying the combustion situations, one would like to establish a reference situation
of non-reactive scalars.
2.3.3 A Situation of Reference – Non-Reactive Scalars
If one takes the scalar c to be non-reactive then ˙ω
c
= 0inEq.(2.85). Using ρ
u
,a
reference value for the density and the scalar Taylor microscale λ
c
=
c
Re
−1/2
T
with

2.3 Scalar-Dissipation-Rate Approach 79
c
as the scalar integral length scale, one gets a scaling [169] for the dissipation rate
as
ρ
c
≡ ρ ˆα
∂c
∂x
k
∂c
∂x
k
O
ρ
u
u
rms
c
2
;Re
0
T
, (2.92)
where the Schmidt number Sc = ν/ ˆα is taken to be unity and the scalar integral length
scale is equal to the turbulence integral length scale . This result confirms that
ρ
c
is of the order of Re
0
T
– that is, Re
T
raised to the power zero – and consequently it
remains finite as the turbulence Reynolds number becomes very large (Re
T
→∞).
The transport equation for this situation can be obtained from Eq. (2.90)by
approximating the molecular-diffusion coefficients to be constant and the Lewis
numbers for all species to be close to unity. The density is taken to be constant but,
for convenience, the equation is presented within the Favre-averaging formalism.
This will ease subsequent comparisons with situations involving a reactive scalar (1)
under isothermal conditions corresponding to a ‘cold flame’ and (2) in combustion
including thermal-expansion effects. It is understood that the fluctuations involved
will not be Favre fluctuations, and the transport equation will then be exactly the
same as Eq. ( 2.90) with (IX) = (X) = 0, because there are no chemical reactions and
dilatation.
ORDER-OF-MAGNITUDE ANALYSIS. The classical rules of OMA proposed by Ten-
nekees and Lumley [169] are used. The spatial derivatives of mean quantities and
fluctuating quantities are scaled by the turbulence integral length scale and Taylor
microscale λ, respectively. The time derivative of the mean quantity is scaled using
the turbulence time scale, τ
T
≡ /u
rms
. This gives
(I) O
ρ
u
u
rms
2
c
2
;Re
0
T
,
(II) O
ρ
u
u
rms
2
c
2
;Re
0
T
,
(III) O
ρ
u
u
rms
2
c
2
;Re
−1
T
,
(IV) O
ρ
u
u
rms
2
c
2
;Re
0
T
,
(IV-b) O
ρ
u
u
rms
2
c
2
;Re
−1/2
T
,
(V) O
ρ
u
u
rms
2
c
2
;Re
0
T
,
(VI) O
ρ
u
u
rms
2
c
2
;Re
0
T
,
(VII) O
ρ
u
u
rms
2
c
2
;Re
1/2
T
, (2.93)
in standard notations. Because there is no a priori scaling rule for the second-order
derivative of scalar fluctuations, it is not possible to derive an order of magnitude
from the expression of term (VIII) in Eqs. (2.91). However, because for a passive
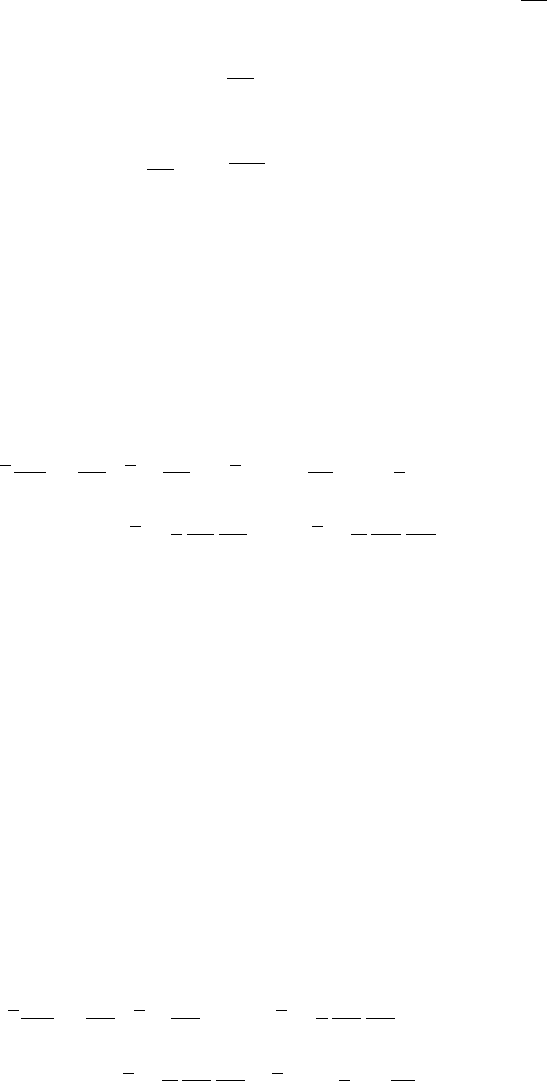
80 Modelling Methods
scalar (VII) is Re
1/2
T
, one can expect (VIII) to be of the same order of magnitude
in such a manner that, at leading order, (VII) and (VIII) balance together; further
details of these arguments are provided in [166].
Let us now envisage the simplest linear closures for each of the terms just dis-
cussed. The turbulence–scalar interaction term is represented by (VII) = A
e
ρ
c
/τ
T
,
where A
e
is a modelling constant and τ
T
is the integral time scale of turbulence; the
closure for the dissipation term (VIII) is similar except that the time scale is the one
associated with the scalar field (VIII) = β
ρ
c
/τ
s
,withβ being a modelling constant
and τ
s
=
c
2
/
c
. Invoking the balance between (VII) and (VIII), a classical algebraic
model, also known as the linear relaxation closure, for the mean SDR is recovered:
ρ
c
= C
D
ρc
2
/τ
T
, (2.94)
where the constant C
D
is found to be equal to the ratio A
e
/β. This model can also
be obtained by simply balancing the dissipation and the turbulence production of
the scalar variance; see Eq. (1.34). Unfortunately, the turbulence-to-scalar integral
time-scale ratio C
D
is unlikely to have a single value for all types of flow, even for
the passive scalar situation [170–174].
MODELLED SDR TRANSPORT EQUATION. The modelled transport equation proposed
by Lumley and his co-workers [161, 162] and studied by Newman et al. [163] and
Jones and Musonge [167]iswrittenas
ρ
D
c
Dt
=
∂
∂x
ρ ˆα
T
∂
c
∂x
−
ρ
c
C
D
1
c
c
2
+ C
D
2
ε
k
+C
D
3
ρ ˆα
T
ε
k
∂
c
∂x
∂
c
∂x
+ C
D
4
ρν
T
c
k
∂u
k
∂x
∂u
k
∂x
, (2.95)
where gradient models are used for the scalar fluxes and Reynolds stresses.
In this equation it must be recalled that the last three terms are supposed to
represent the sum of the two largest terms, namely (VII) and (VIII). Thus, these
terms, of the order of Re
1/2
T
, compensate themselves and the r emainder is of the
order of Re
0
T
. Moreover, terms proportional to mean gradients of concentration
and of velocity appearing in the unclosed equation are supposed to be negligible
because of the assumption of small-scale isotropy and of small-scale independence
(see [166]). Now, it must be also recalled that the modelled equation proposed in
[165, 166] differs from Eq. (2.95) as (1) the terms related to mean gradients of c and
of velocity, i.e., (V) and (VI), are retained but not only as a contribution coming from
(VII) and (VIII) because the assumption of small-scale isotropy is not supported by
experiments [170]; and (2) terms (VII) and (VIII) are modelled separately, and the
result is one production term proportional to
c
in addition to a destruction term
proportional to (
c
)
2
.
Accordingly, following the OMA proposed in [165, 166], the modelled transport
equation can be written as
ρ
D
c
Dt
=
∂
∂x
k
ρ ˆα
T
∂
c
∂x
k
+ C
P
c
ρ ˆα
T
ε
k
∂
c
∂x
k
∂
c
∂x
k
+ C
P
U
ρν
T
c
k
∂u
∂x
k
∂u
∂x
k
+ ρ
c
A
e
ε
k
− β
c
c
2
, (2.96)
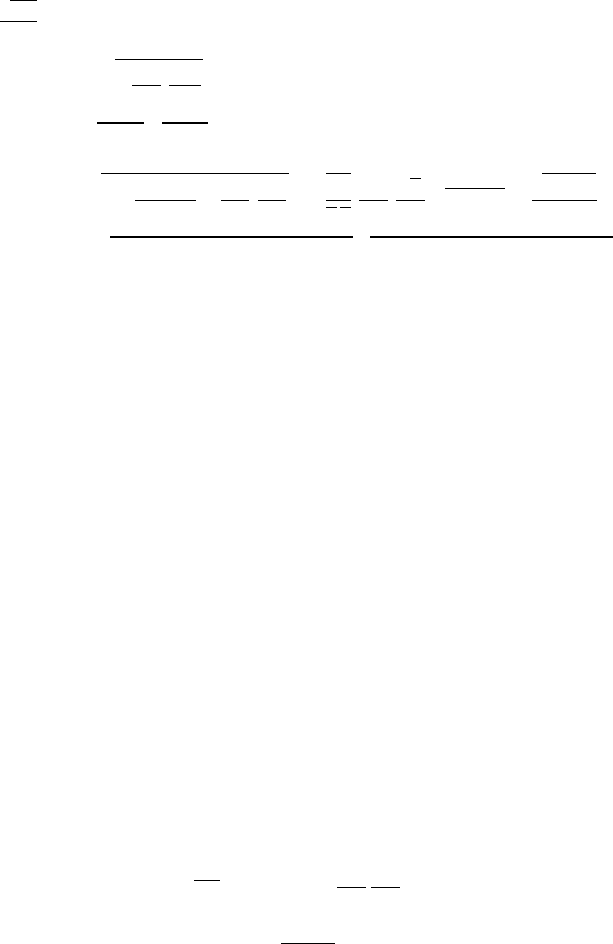
2.3 Scalar-Dissipation-Rate Approach 81
where gradient models have been used for the scalar fluxes and Reynolds stresses. It
is to be noted that the last contribution of Eq. (2.96) differs from previous proposals
of Zeman and Lumley [162] and Jones and Musonge [167]: the sign of the third term
on the right-hand side of Eq. (2.95) is negative.
2.3.4 SDR in Premixed Flames and Its Modelling
EFFECTS OF CHEMICAL REACTION. Let us now consider the mean dissipation rate of
a reactive scalar c(x, t):
∂
ρ
c
∂t
=−(II) + (III) + (IV) + (V) + (VI) + (VI-b) +(VII) − (VIII)
+ 2
ˆα
∂c
∂x
k
∂ ˙ω
∂x
k
(IX)
− 2
ˆα
˙ω
c
+ D
ρ
∂c
∂x
k
∂ρ
∂x
k
+ 2
ρ ˆα
ρ ρ
∂
c
∂x
k
∂ρ
∂x
k
˙ω
c
+ D −
∂
ρ u
c
∂x
.
(X)
(2.97)
There are two additional contributions, (IX) and (X). Term (IX) corresponds to the
influence of passive chemical reaction only whereas the term ( X) includes the effects
of variable density that are due to heat release.
OMA FOR REACTIVE SCALAR IN THE FAST CHEMISTRY LIMIT. In the special case of
turbulent premixed combustion within the flamelet regime, the OMA is modified as
follows: the instantaneous quantities involving the species gradient must be scaled
by the flamelet thickness, δ
0
L
. This is because such quantities are non-zero only inside
flamelets. Moreover, for a quantity that is zero for c = 1 (fully burned products)
and c = 0 (fresh reactants), the averaging procedure is nothing else but a product
by the reactive scalar PDF
P(c), which is of the order of Da
−1
for intermediate
values c ∈ [0 : 1]; see [21, 62]. The Damk
¨
ohler number is defined as Da ≡ τ
T
/τ
c
=
S
L
/u
rms
δ
0
L
. The previous point is of particular importance and should be kept in
mind. If this point is not taken into account, then the scaling of the mean reactive
scalar dissipation term is found to be Da,
1
an unrealistic feature because Eq. (2.86)
clearly shows that, in the flamelet regime of turbulent premixed combustion, the
mean reaction rate is proportional to this quantity and it must remain finite when
Da →∞, as emphasised in the early analyses of Bray, Champion, and Libby [175].
Using the definition of SDR and recalling that both molecular viscosity and
diffusivity scale as S
0
L
δ
0
L
, one obtains the order of magnitude of the mean reactive
SDR as follows:
ρ
c
=
c=1
−
c=0
+
ρ D
∂c
∂x
k
∂c
∂x
k
P(c)dc
O
ρ
u
u
rms
c
2
;Da
0
Re
0
T
. (2.98)
82 Modelling Methods
This expression is similar to Eq. (2.92) obtained for a passive scalar; as expected,
ρ
c
is O
Re
0
T
Da
0
and remains finite whatever, the values of the Damk
¨
ohler and
Reynolds numbers, i.e., as Da or Re
T
→∞. This behaviour confirms the relevance
of the proposed scalings.
The OMA of the different terms (II)–(XI) appearing on the right-hand side of
Eq. (2.97) can be performed with the new scalings. Several studies have been already
performed in this direction, and interested readers are referred to [150, 165, 176, 177]
for further details. In fact, even if attention is focused on the flamelet regime, the
results can differ from one reference to another. This is because, there is not only
a priori one single possible scaling for the fluctuating velocity gradient. The choice
should depend on the level of exothermicity [178]. This question was not raised in
the early work of Mantel and Borghi because thermal expansion across a flamelet
was not considered by taking the fluid density to be constant. The corresponding
issue is rather general and largely exceeds the restricted scope of the SDR transport-
equation modelling. It concerns the description of reactive scalar transport (see for
instance [179]), and it is also related to the closure of certain terms in the scalar
flux transport equation [28, 180]. We now see how the analysis is modified by the
chemical reaction and associated thermal expansion.
For the thin-flamelet combustion regime, one may write the progress-variable
transport equation as ρ
u
S
L
n
k
∂c/∂x
k
= ˙ω
c
+ D, where n
k
is kth component of the
flame normal pointing towards the product side. The dissipation term (VIII) in
Eqs. (2.91) can be rewritten as
(VIII) =
ρ ˆα
2
(c
,n
)
,n
(c
,n
)
,n
+ 4ρ ˆα
2
(c
,n
)
,
ˆ
t
(c
,n
)
,
ˆ
t
+ 4ρ ˆα
2
(c
,
ˆ
t
)
,
ˆ
t
(c
,
ˆ
t
)
,
ˆ
t
, (2.99)
where local isotropy has been assumed in the flamelet tangential directions that
are indiscriminately denoted by the subscript
ˆ
t. The symbol c
,k
denotes the deriva-
tive of c in direction k. Combining the instantaneous progress-variable budget with
Eq. (2.99), it can be shown that the reactive contribution (IX) simplifies with a part
of the dissipation contribution (VIII), as first established in [165]. As a result, the
reactive contribution (IX) no longer deserves to be considered, and the remaining
part of the dissipation term, denoted as (VIII)
∗
, is written as
(VIII)
∗
= 8ρ ˆα
2
(c
,n
)
,
ˆ
t
(c
,n
)
,
ˆ
t
+ 8ρ ˆα
2
(c
,
ˆ
t
)
,
ˆ
t
(c
,
ˆ
t
)
,
ˆ
t
. (2.100)
In this manner, the consequence of the persistence of flamelet structures that are
sustained in the high-Da limit is that the complex correlation that involves the
chemical rate does not require further consideration. This peculiarity was evidenced
in [165], proposing the following modelled SDR transport equation:
∂
ρ
c
∂t
+
∂
∂x
k
ρ(u
k
−
U
L
k
)
c
=
∂
∂x
ρ ˆα
T
∂
c
∂x
+C
P
c
ρ ˆα
T
ε
k
∂
c
∂x
k
∂
c
∂x
k
+ C
P
U
ρν
T
c
k
∂u
∂x
k
∂u
∂x
k
+ρA
e
Re
1/2
T
ε
k
c
−
2
3
ρβ Re
1/2
T
2
c
c
2
3
2
− C
c
S
L
k
1/2
, (2.101)
