Salby M.L. Fundamentals of Atmospheric Physics

Подождите немного. Документ загружается.

350

11 Atmospheric Equations

of Motion

cos~j

2~ sin~k

Figure

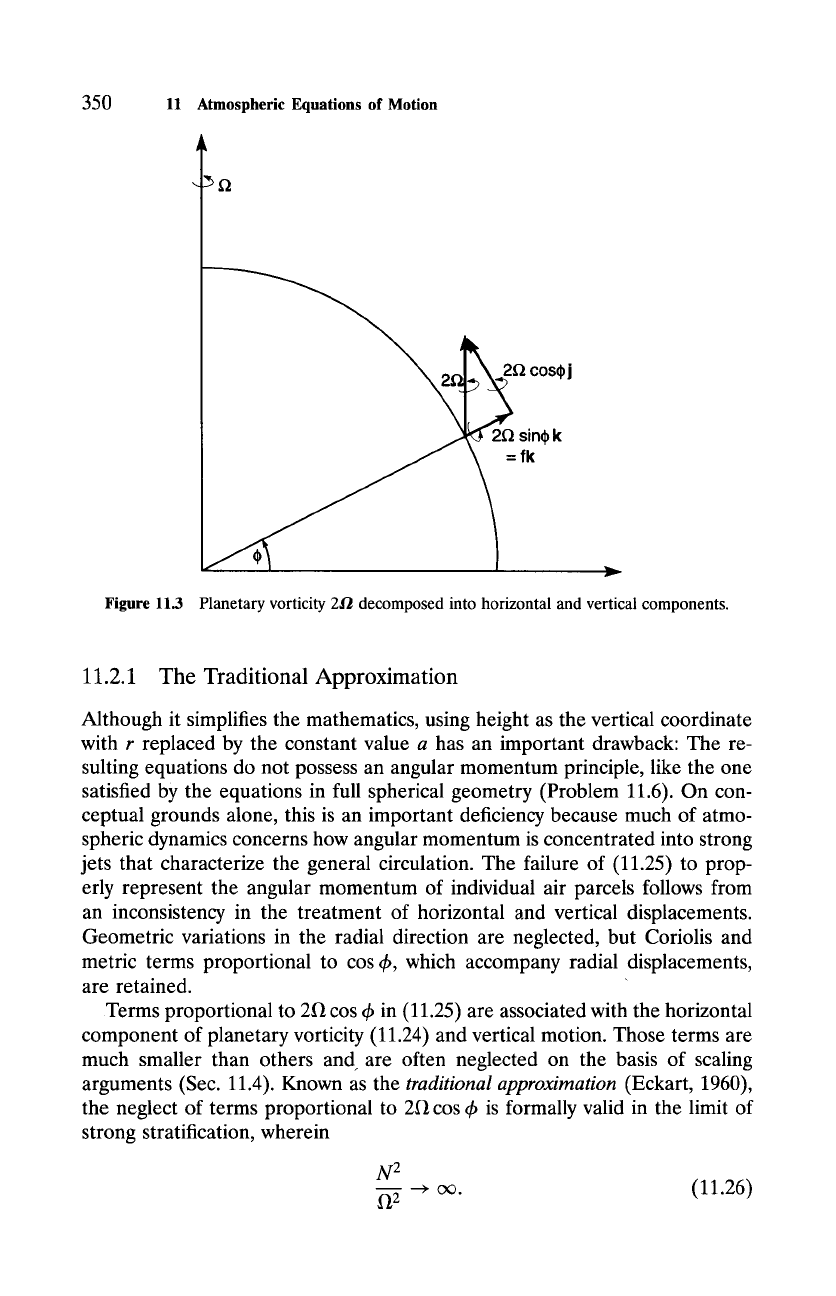
11.3

Planetary vorticity 2g~ decomposed into horizontal and vertical components.

11.2.1 The Traditional Approximation

Although it simplifies the mathematics, using height as the vertical coordinate

with r replaced by the constant value a has an important drawback: The re-

suiting equations do not possess an angular momentum principle, like the one

satisfied by the equations in full spherical geometry (Problem 11.6). On con-

ceptual grounds alone, this is an important deficiency because much of atmo-

spheric dynamics concerns how angular momentum is concentrated into strong

jets that characterize the general circulation. The failure of (11.25) to prop-

erly represent the angular momentum of individual air parcels follows from

an inconsistency in the treatment of horizontal and vertical displacements.

Geometric variations in the radial direction are neglected, but Coriolis and

metric terms proportional to cos ~b, which accompany radial displacements,

are retained.

Terms proportional to 213 cos ~b in (11.25) are associated with the horizontal

component of planetary vorticity (11.24) and vertical motion. Those terms are

much smaller than others and are often neglected on the basis of scaling

arguments (Sec. 11.4). Known as the

traditional approximation

(Eckart, 1960),

the neglect of terms proportional to 213 cos ~b is formally valid in the limit of

strong stratification, wherein

N 2

c~. (11.26)

122

11.2

Spherical Coordinates

351

Air is then constrained to move quasi-horizontally, which makes the vertical

component of planetary vorticity 2[1 sin ~b dominant in the Coriolis accelera-

tion 2.0 • v. For a lapse rate of F - 6.5 Kkm -1, (7.10) gives N 2 of order

l0 -4

S -2

and

~~2 ,~ 5 • 10 -9 S -2, SO

N2/~ 2

x 10 4

is in good agreement with

(11.26). Weak or unstable stratification is controlled by convection, which op-

erates on timescales short enough to render both components of the Coriolis

acceleration unimportant.

An alternate derivation of the equations of motion (Phillips, 1966) provides

a rationale for neglecting the aforementioned terms. The component equations

assume a simpler form if derived from the momentum equations in vector-

invariant form. In an inertial reference frame, the vector equations of motion

can be expressed

(3Lii) ( Lii'Lii)2 Lii 1

-~ V \ + (V x Lie) x -- ---re --

(11.27.1)

\ dt

i P

where the subscript refers to the velocity and local time rate of change ap-

parent in the inertial flame (Problem 11.7). Evaluating metric scale factors

at r - a before derivatives are taken recovers the vector operations (11.23).

Then expressing the velocity and time derivative in terms of those apparent in

the rotating flame of the earth

L1 t -- 13 -1-

[la cos ~bi, (11.27.2)

o) _o 110

~ i at O~a (11.27.3)

and incorporating (11.23) produces the simplified momentum equations

du(

utan 6) 1

01)

dt f + v - -~ Da,

a pa

cos ~b 3A

(11.28.1)

dr(

utan 6) lap

dt+ f+ u- D6,

a pa 34)

(11.28.2)

dto

where the

Coriolis parameter

lol)

p Oz

g-Dz,

(11.28.3)

f - 2f~ sin ~b (11.28.4)

represents the vertical component of planetary vorticity (Fig. 11.3).

Equations (11.28) are free of metric and Coriolis terms proportional to

w in the horizontal momentum equations and all such terms in the vertical

momentum equation. The above system possesses the conservation principle

d (1

~p+DA )

(11.29)

dt[(u + aa cos 4')a cos 4'] - -a cos 4'

cos 4' O~

352

11 Atmospheric Equations of

Motion

wherein the angular momentum of an air parcel changes according to the

torque about the axis of rotation exerted on it by longitudinal forces (Prob-

lem 11.8). Consistent with the shallow atmosphere approximation, angular

momentum is treated as though the air parcel remains at r = a.

11.3 Special Forms of Motion

Certain conditions simplify the governing equations. For

incompressible motion,

the specific volume of an individual material element is conserved, so

dP = o.

Then the continuity equation (11.25.4) reduces to

V.v-O. (11.30)

Because p is conserved, a material element that coincides initially with a partic-

ular isochoric surface, p = const, remains on that surface~despite movement

of that surface. Thus isochoric surfaces are material surfaces (namely, they are

comprised of a fixed collection of matter). If the motion is steady, p surfaces

are also stream surfaces, to which the motion is tangential. These conditions

hold automatically for an incompressible fluid like water. They also hold for

a compressible fluid like air if the motion is steady and orthogonal to the gra-

dient of density. Because large-scale motions are quasi-horizontal, the latter

condition is approximately satisfied by atmospheric circulations.

For

adiabatic motion,

individual material elements experience no heat trans-

fer with their surroundings (Sec. 3.6.1). The thermodynamic equation (10.38)

then reduces to

-- -0,

(11.31)

which asserts that the potential temperature of a material element is con-

served. Thus, an air parcel that coincides initially with an isentropic surface,

0 = const, remains on that surface (refer to Fig. 2.9) and isentropic surfaces

are material surfaces.

The cases just discussed are particular examples of a conserved property,

which behaves as a material tracer. More generally, a property r that is con-

served for individual material elements obeys the continuity equation

-- = 0.

(11.32)

Mixing ratios of long-lived chemical species approximately satisfy (11.32) be-

cause the rates of production and destruction for such species, which formally

belong on the right-hand side, are much slower than advective changes in the

Lagrangian derivative, which appear on the left-hand side. Ozone, which has a

11.4 Prevailing Balances

353

photochemical lifetime in the lower stratosphere of several weeks, and water

vapor, which is conserved away from clouds and the earth's surface, behave

approximately in this manner (see Fig. 1.15). Hence, to a first approximation,

particular values of mixing ratio track the movement of individual bodies of

air and the surfaces r - const are material surfaces.

11.4 Prevailing Balances

The preceding equations govern motion in a compressible, stratified, and ro-

tating atmosphere, so they apply to a wide range of phenomena. While de-

scribing planetary-scale circulations that involve timescales of days and longer,

the equations of motion also describe small-scale acoustic waves that have

timescales of only fractions of a second. This generality needlessly complicates

the description of phenomena that figure in the general circulation. To eluci-

date essential balances controlling large-scale atmospheric motions, it is useful

to examine the relative sizes of various terms.

11.4.1 Motion-Related Stratification

The stratification, which is represented in the distributions of pressure and

density, is comprised of two components: (1) a basic component associated

with static conditions and (2) a small departure from it that is related directly

to motion:

Ptot "--

PO(Z) -+- p(x, t),

Ptot :"

PO(Z) -~- p(X, t),

(11.33)

where x = (x, y, z) follows from (11.17). The static components P0 and P0,

which are functions of height alone, are symbolized by the global-mean pres-

sure and density. Those components overshadow vertical variations associated

with motion, so it is instructive to eliminate them from the governing equa-

tions.

Incorporating (11.33) into the horizontal momentum equation transforms

the pressure gradient force into

(po+p____~Vh(p ~ +p),~ _lpo

(1--P~O)

Vhp

where

V h

denotes the horizontal gradient and

P/Po

<< 1 and

P/Po

<< 1

have been used in combination with the binomial expansion to ignore higher

order terms. Because

po(z)

depends on height alone, it drops out of (11.34)

to leave the horizontal momentum equations in their original form (11.28).

~-- Vhp,

(11.34)

354

II Atmospheric Equations of Motion

In the vertical momentum equation, the basic components of stratification

introduce vertical gradients that cannot be ignored. The right-hand side of

(11.28.3) contains the net buoyancy force acting on an air parcel (7.2). Incor-

porating (11.33) transforms the buoyancy force into

1 0 1 (1 -

p)(OPo Op)

f b = -- ( P o + P) 3 z ( P O + p) -- g ~ -- P--o0 -~0 \ O Z + -~Z -- g

)

- oo ~+pg ,

wherein the basic stratification automatically satisfies hydrostatic equilibrium.

Then the vertical momentum equation becomes

dw 1 Op p

= g-O z.

(11.36)

dt Po Oz Po

11.4.2 Scale Analysis

With dependent variables related directly to motion, terms in the momentum

equations can now be evaluated for relative importance. Away from the earth's

surface and regions of organized convection, the frictional drag D is small

enough to be ignored. Large-scale atmospheric motions are then characterized

by the scales

U = 10

m s -1

= 10 3

km P- 10 mb-

10 3

= 10 -2 ms -1

H- 10 km fo

= 10-4

s-l,

where U and W refer to horizontal and vertical motion, respectively, L and H

are horizontal and vertical length scales that characterize the motion field, and

f0 = 212sin 4'0 is the planetary vorticity at a representative latitude q'0. The

pressure P characterizes the departure from pure static conditions, namely, the

pressure variation associated directly with motion. If the Lagrangian derivative

is dominated by advective changes,

L/U

represents a timescale for advection

that can be used to characterize

d/dt.

Scaling velocities, lengths, and the Lagrangian derivative by the scales given

above (e.g.,

u --+ Uu, x --+ Lx, d/dt --+ (U/L)d/dt,

with u, x, and

d/dt

then

nondimensional) transforms the horizontal momentum equations (11.28.1) and

(11.28.2) into the dimensionless forms

Ro-~ -

sin

4~v - Ro

tan

4)uv = -~

P lop

9

(11.37.1)

foPoo UL Po Ox'

Ro-~ +

sin

dpu + Ro a

tan ~u 2 -- -~

P 10p

foPoo UL Po Oy

(11.37.2)

11.4

Prevailing Balances

355

where Poo = po(0) and all variables are nondimensional, of order unity, and

are related to their dimensional counterparts through multiplication by the

above scale factors. The

Rossby number

= (11.38)

foL

appearing in the scaled momentum equations is a dimensionless parameter

that represents the ratio of the rotational timescale fo 1 to the advective

timescale L/U.

With the preceding scales, dimensionless factors in (11.37) are characterized

by the following orders of magnitude:

Ro-10

-1

Ro(L)=IO -2 P =

a foPooUL 1

Hence, the horizontal momentum equations are dominated by a balance be-

tween the Coriolis acceleration associated with the vertical component of plan-

etary vorticity and the horizontal pressure gradient force, both of which are of

order unity. Relative to these, the material acceleration, which is of order Ro,

is small because the timescale for advection is long compared to the rotational

timescale. Therefore, horizontal motion of an individual air parcel is domi-

nated by rotation. Accelerations associated with metric terms and with vertical

advection of momentum in

d/dt

are even smaller, whereas those proportional

to w that were eliminated in the derivation of (11.28) are much smaller.

Similar treatment transforms the vertical momentum equation (11.36) into

the dimensionless form

(W) dw

(L) P lop .....

(~oo) g p (11.39),

Ro dt =- foPoo UL Po ~z fo ~J Po

where P00 = p0(0). The preceding scales imply the orders of magnitude

P _10 2 ( P g

This scale analysis demonstrates that the vertical momentum equation for

large-scale motions is dominated by a balance between the vertical pressure

gradient and gravitational forces. Hence, the motion-related component of

stratification also satisfies hydrostatic equilibrium, other vertical forces being

much smaller. Vertical forces involved in hydrostatic equilibrium are also two

orders of magnitude greater than accelerations in the horizontal momentum

equations, which illustrates the comparative influences of gravity and rotation

(11.26).

356

11 Atmospheric Equations of Motion

The foregoing analysis allows the equations of motion to be simplified.

Inclusive of the basic stratification, the dimensional equations governing large-

scale atmospheric motion in spherical coordinates then become

dUdt (f +

u tan 4~)a

v= -~pa

cosl r 3A~ D~, (11.40.1)

dv (

utan~b) 1 Op

dt + f + u - D4,, (11.40.2)

a pa ~49

lop

pOz

= -g, (11.40.3)

at,

dt + pV. v = O, (11.40.4)

pcv--d- [

+ pV. v = qnet, (11.40.5)

which are termed the

primitive equations

because they constitute the starting

point for investigations of large-scale atmospheric dynamics. Metric terms

proportional to tan 4, and horizontal drag have been retained in (11.40), but,

while often included in numerical integrations, they are small enough to be

omitted for many analyses. In chemical applications, the primitive equations

are augmented by continuity equations of the form

dri

=/~i -/)i

(11.40.6)

for the mixing ratio of the ith species, where/5 i and/)i denote the local rates

of production and destruction of that species.

The governing equations must be closed with boundary conditions. Spher-

ical geometry is periodic, so continuity suffices for horizontal boundary con-

ditions. Vertical boundary conditions constrain the upward motion. At the

ground, the motion must be tangential to that lower boundary. If the surface

has elevation

Zs(A, 49, 0, 2

this is equivalent to requiring an air parcel initially

in contact with it to track along the same elevation:

dzs

w- dt'

(11.41)

where

d/dt

is given by (11.25.6). 3 In the presence of viscosity, the motion must

also satisfy the

no-slip condition,

which requires the velocity at the ground to

vanish. Upper boundary conditions are more difficult to apply because the

2The lower boundary condition can be applied on a surface internal to the fluid, in which case

the elevation is time dependent.

3Even though a fluid element at the ground must remain in contact with that boundary,

neighboring elements can achieve large vertical displacements (e.g., in convection; refer to Fig. 5.4)

through deformation of finite bodies of fluid.

11.5 Thermodynamic Coordinates 357

domain is unbounded, but similar constraints are usually imposed at a finite

height.

11.5 Thermodynamic Coordinates

11.5.1 Isobaric Coordinates

Because they involve hydrostatic equilibrium, the primitive equations simplify

when formulated with pressure as the vertical coordinate. Hence, we consider

a transformation from the standard spherical coordinates x = (x, y, z) given

by (11.17) to the modified coordinates

Xp = (x, y, p).

Then isobaric surfaces

p = const replace constant height surfaces z --- const as coordinate surfaces,

along which horizontal derivatives must be evaluated. Using p as a vertical

coordinate is possible because hydrostatic equilibrium (11.40.3) ensures that

pressure varies with height monotonically and therefore is a single-valued

function of z.

In casting the equations into isobaric coordinates, the variables p and z are

interchanged. Pressure then becomes an independent variable and z becomes

a dependent variable. For a specified pressure,

z(p)

represents the height of

an isobaric surface, which is contoured in Fig. 1.9. Isobaric surfaces are not

perpendicular to the other coordinate surfaces, so this representation does not

constitute an orthogonal coordinate system.

Consider a scalar quantity

g/(x, y, z, t) = (O[x, y, p(x, y, z, t), t].

(11.42)

Hereafter, the caret will be omitted with the pressure dependence understood.

By the chain rule, the vertical derivative can be expressed

( )xy - ( )xy

1143

where subscripts denote variables that are held fixed. Likewise, differentiation

with respect to x becomes

oq, oqJ

)

yzt ypt -~X / yzt

and similarly for differentiation with respect to y. Thus, the horizontal gradient

evaluated on surfaces of constant height V~ translates into

(O~O) VzP,

(11.44.1)

Vzq, - Vpq, + Upp xy,

where

() 11442

i -~- -~Y xpt

Vp- -~x ypt

358

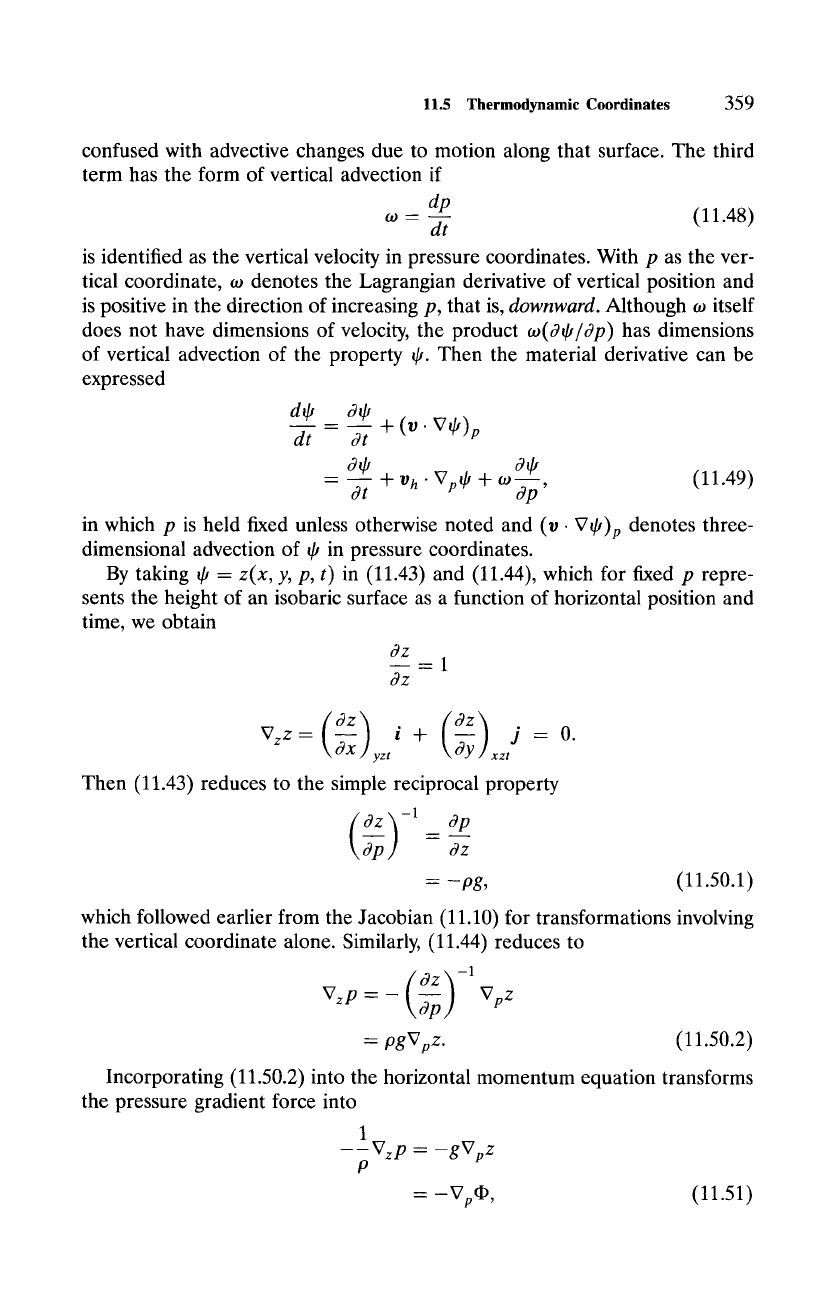
11 Atmospheric Equations of Motion

z T

I /

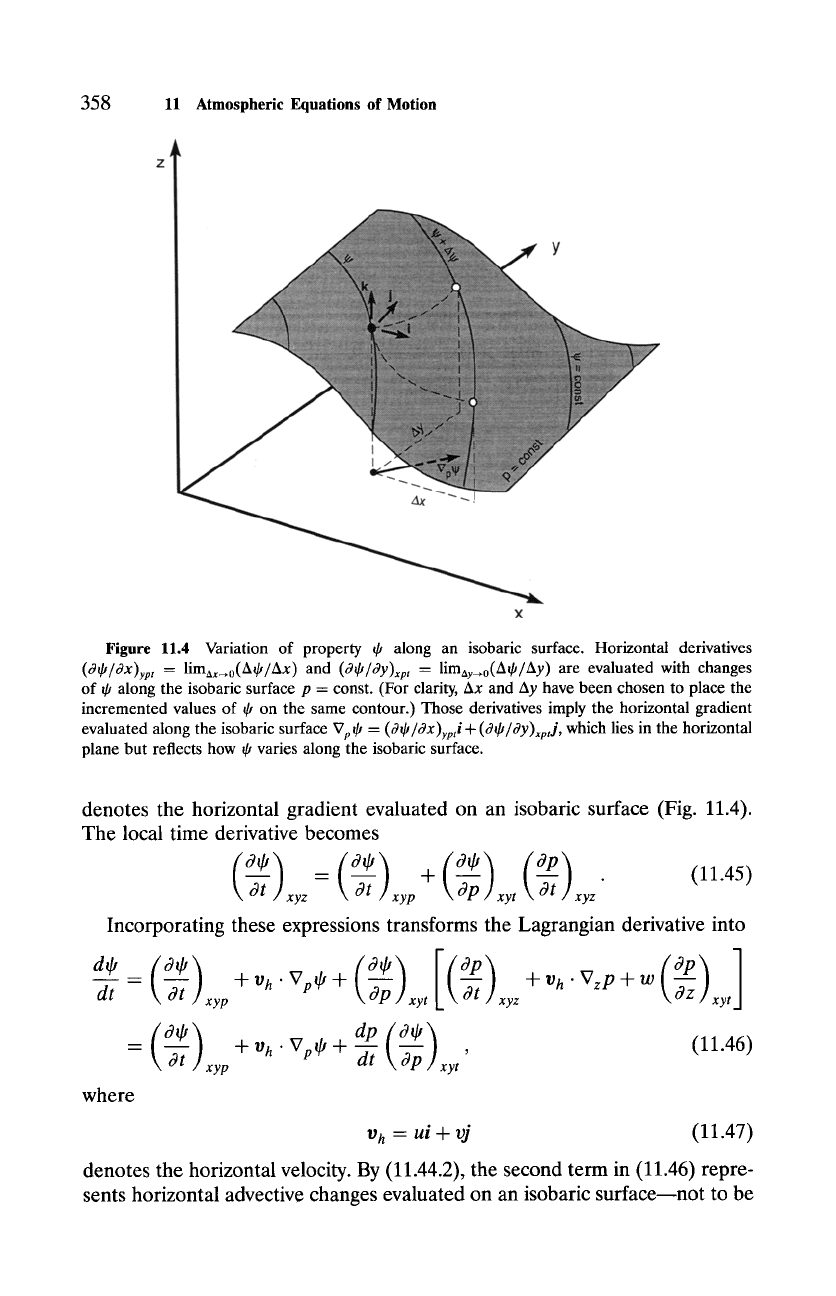
Figure 11.4 Variation of property q/ along an isobaric surface. Horizontal derivatives

(t~ll/t~X)ypt --"

limax__,o(Ad//Ax )

and

(d~/dY)xpt =

limay_,o(Ad//Ay)

are evaluated with changes

of ~, along the isobaric surface p - const. (For clarity, Ax and Ay have been chosen to place the

incremented values of 6 on the same contour.) Those derivatives imply the horizontal gradient

evaluated along the isobaric surface Vp~, =

(dcp/dX)rpti + (dd//OY)xptJ,

which lies in the horizontal

plane but reflects how ~, varies along the isobaric surface.

denotes the horizontal gradient evaluated on an isobaric surface (Fig. 11.4).

The local time derivative becomes

(~t )xyz-- (--~)xypW (-~P)xyt (~t)xyz.

(11.45,

Incorporating these expressions transforms the Lagrangian derivative into

dt = --~ + oh " Vp~ + ~ xyt ~ + l~h " Vzp + 113 ~Z xyt

xyp xyz

(dd/) dp (dq~)

(11.46)

-- --~ xyp -~- llh " VPr -~- --d-f ~ xyt'

where

Vh = ui + vj

(11.47)

denotes the horizontal velocity. By (11.44.2), the second term in (11.46) repre-

sents horizontal advective changes evaluated on an isobaric surface~not to be

11.5 Thermodynamic Coordinates

359

confused with advective changes due to motion along that surface. The third

term has the form of vertical advection if

w = dt

(11.48)

is identified as the vertical velocity in pressure coordinates. With p as the ver-

tical coordinate, to denotes the Lagrangian derivative of vertical position and

is positive in the direction of increasing p, that is,

downward.

Although to itself

does not have dimensions of velocity, the product

oo(OqJ/Op)

has dimensions

of vertical advection of the property qJ. Then the material derivative can be

expressed

~- (t~ 9 V~)p

+ Vh" VpO _ + w~

oqJ

dp' (11.49)

in which p is held fixed unless otherwise noted and (v.

V~)p

denotes three-

dimensional advection of qJ in pressure coordinates.

By taking qJ =

z(x, y, p, t)

in (11.43) and (11.44), which for fixed p repre-

sents the height of an isobaric surface as a function of horizontal position and

time, we obtain

--=1

()

Oz i+

j=O.

VzZ -- -~X yzt xzt

Then (11.43) reduces to the simple reciprocal property

(Oz)-loP

= -pg,

(11.50.1)

which followed earlier from the Jacobian (11.10) for transformations involving

the vertical coordinate alone. Similarly, (11.44) reduces to

Vzp = -- VpZ

= pgVpz.

(11.50.2)

Incorporating (11.50.2) into the horizontal momentum equation transforms

the pressure gradient force into

--Vzp = -gVpz

= -Vp., (11.51)