Salby M.L. Fundamentals of Atmospheric Physics
Подождите немного. Документ загружается.


320 9
Aerosol and Clouds
9.46.
9.47.
either side of the interface to derive Snell's law:
sin 02 nl
sin 01 n2
where 01 and 02 denote the zenith angles of incident and refracted ra-
diation and n 1
--
Co/C 1
and n2 =
Co/C2
are the corresponding indices of
refraction, which are defined in terms of the speeds Cl and c2 at which
light propagates in media 1 and 2.
Scattering of SW radiation is enhanced in polar regions, where solar
zenith angles are large. As a simple model, consider conservative scatter-
ing of SW radiation from a wind-blown ocean surface, which randomly
occupies all angles a from the horizontal between +90 ~ (a) Use Snell's
law and conservation of energy crossing the surface to determine that
fraction of incident radiation arriving at zenith angle
Os
> 0 which is
scattered into the zenith angle 0. (b) For 0~ = 30 ~ compare the inten-
sity of light which is forward scattered
(Os
< 0) against that which is
backward scattered
(0 s > 0).
Use the directionality of scattering to assess the change in overall SW
transmission accompanYing the reduction of direct transmission following
the eruption of E1 Chichon in Fig. 9.5.

Chapter 10
Atmospheric Motion
Under radiative equilibrium, the first law reduces to a balance between
radiative transfers of energy, which determines the radiative-equilibrium ther-
mal structure. This simple balance is valid for a resting atmosphere, but breaks
down for an atmosphere in motion because heat is then also transferred me-
chanically by air movement. Discrepancies of the radiative-equilibrium ther-
mal structure (Fig. 8.21) from the observed global-mean temperature (Fig. 1.2)
point to the importance of mechanical heat transfer. Radiative-convective
equilibrium, which accounts for vertical heat transfer by convection, recon-
ciles the equilibrium thermal structure with that observed, but only in the
global-mean.
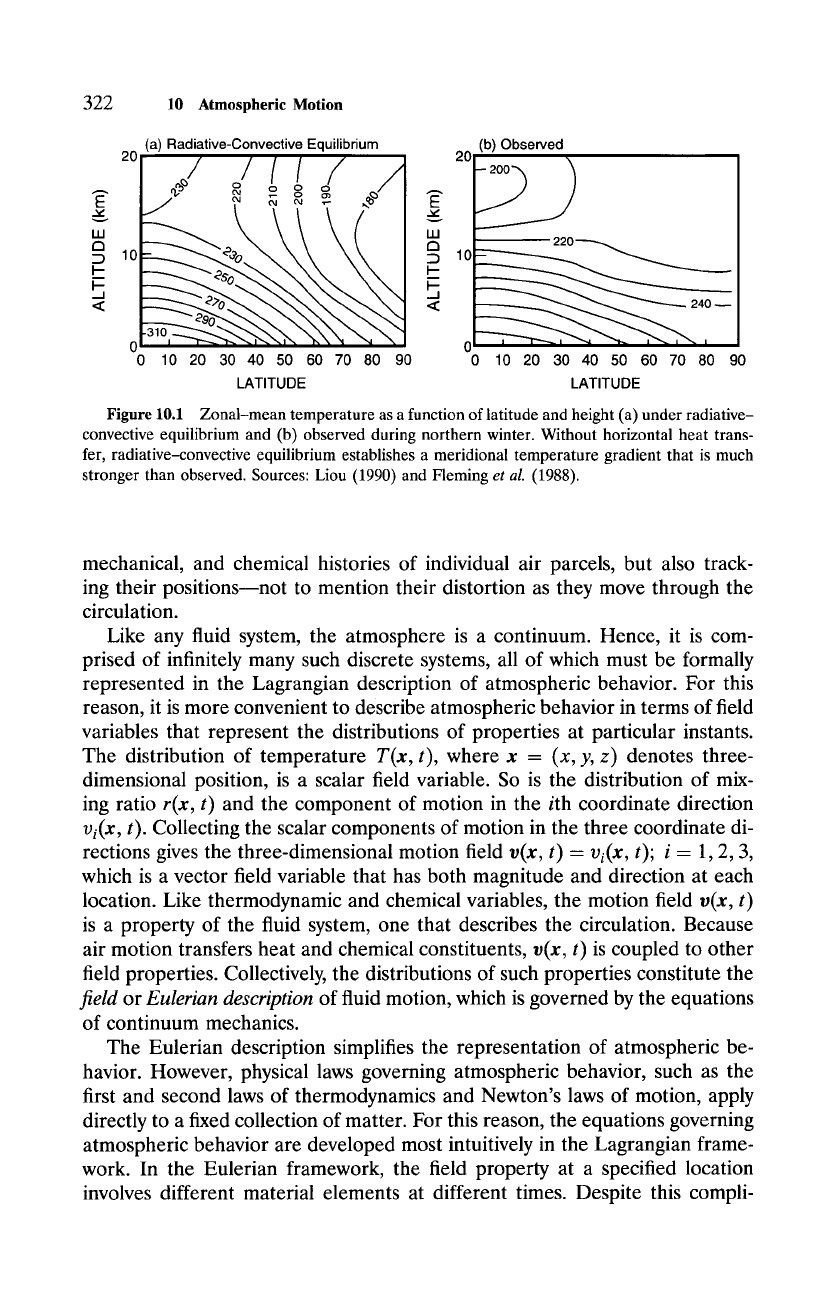
Radiative and radiative-convective equilibrium both produce a meridional
temperature gradient that is too steep compared to observed thermal structure
(Fig. 10.1). Each accounts only for vertical transfers of energy. According to
the distribution of net radiation (Fig. 1.29), low latitudes experience radiative
heating, whereas middle and high latitudes experience radiative cooling. Ther-
mal equilibrium then requires those radiative imbalances to be compensated
by a poleward transfer of heat. That horizontal heat transfer is accomplished in
large part by the atmospheric circulation. Thus, understanding how observed
thermal structure is maintained requires an understanding of atmospheric mo-
tion and the horizontal transfers of energy that it supports.
10.1 Descriptions of Atmospheric Motion
Until now, the development has focused on an infinitesimal fluid element
that defines an individual air parcel. Thermodynamic and hydrostatic influ-
ences acting on that discrete system must now be complemented by dynamical
considerations that control the movement of individual bodies of air. To-
gether, these properties constitute the individual or
Lagrangian description
of
fluid motion, which represents atmospheric behavior in terms of the collec-
tive properties of material elements comprising the atmosphere. Because the
basic laws of physics apply to a discrete bounded system, they are developed
most simply within the Lagrangian framework. However, the Lagrangian de-
scription of atmospheric behavior requires representing not only the thermal,
321
322
I0 Atmospheric Motion
20
E
uJ
a
10
F--
..3
<
01
' , ~...~ ~ -~, ~ ~, ~ ,~ ~ 9 ~, ~ 9
0 10 20 30 40 50 60 70 80 90
(a) Radiative-Convective Equilibrium (b) Observed
o/ o/f o// A
5
0 10 20 30 40 50 60 70 80 90
LATITUDE LATITUDE
Figure
10.1 Zonal-mean temperature as a function of latitude and height (a) under radiative-
convective equilibrium and (b) observed during northern winter. Without horizontal heat trans-
fer, radiative-convective equilibrium establishes a meridional temperature gradient that is much
stronger than observed. Sources: Liou (1990) and Fleming
et al.
(1988).
mechanical, and chemical histories of individual air parcels, but also track-
ing their positionsmnot to mention their distortion as they move through the
circulation.
Like any fluid system, the atmosphere is a continuum. Hence, it is com-
prised of infinitely many such discrete systems, all of which must be formally
represented in the Lagrangian description of atmospheric behavior. For this
reason, it is more convenient to describe atmospheric behavior in terms of field
variables that represent the distributions of properties at particular instants.
The distribution of temperature
T(x, t),
where x - (x, y, z) denotes three-
dimensional position, is a scalar field variable. So is the distribution of mix-
ing ratio
r(x, t)
and the component of motion in the ith coordinate direction
vi(x, t).
Collecting the scalar components of motion in the three coordinate di-
rections gives the three-dimensional motion field v(x, t) -
vi(x,
t); i - 1, 2, 3,
which is a vector field variable that has both magnitude and direction at each
location. Like thermodynamic and chemical variables, the motion field
v(x, t)
is a property of the fluid system, one that describes the circulation. Because
air motion transfers heat and chemical constituents, v(x, t) is coupled to other
field properties. Collectively, the distributions of such properties constitute the
field
or
Eulerian description
of fluid motion, which is governed by the equations
of continuum mechanics.
The Eulerian description simplifies the representation of atmospheric be-
havior. However, physical laws governing atmospheric behavior, such as the
first and second laws of thermodynamics and Newton's laws of motion, apply
directly to a fixed collection of matter. For this reason, the equations governing
atmospheric behavior are developed most intuitively in the Lagrangian flame-
work. In the Eulerian framework, the field property at a specified location
involves different material elements at different times. Despite this compli-
10.2 Kinematics of Fluid Motion 323
cation, the Eulerian description is related to the Lagrangian description by a
fundamental kinematic constraint: The field property at a given location and
time must equal the property possessed by the material element occupying
that position at that instant.
10.2 Kinematics of Fluid Motion
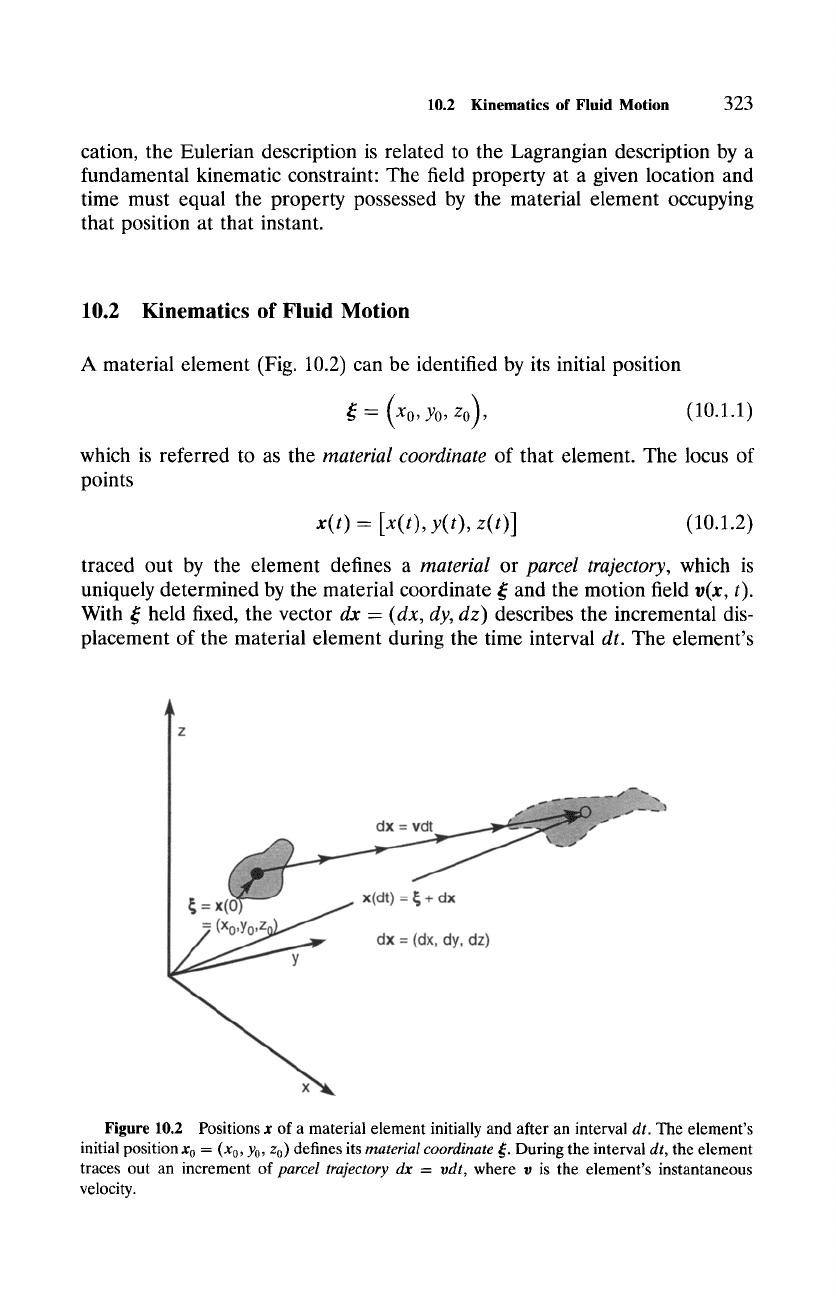
A material element (Fig. 10.2) can be identified by its initial position
~--(x0, Y0, z0), (10.1.1)
which is referred to as the
material coordinate
of that element. The locus of
points
x(t)
= [x(t),
y(t),
z(t)] (10.1.2)
traced out by the element defines a
material
or
parcel trajectory,
which is
uniquely determined by the material coordinate ~: and the motion field
v(x, t).
With ~ held fixed, the vector dx =
(dx, dy, dz)
describes the incremental dis-
placement of the material element during the time interval
dt.
The element's
= x(o ~ x(et) = e, + dx
=
(x~176 dx = (dx, dy, dz)
Figure
10.2 Positions x of a material element initially and after an interval
dt. The
element's
initial position x0 = (x0, Y0, z0) defines its
material coordinate ~.
During the interval
dt,
the element
traces out an increment of
parcel trajectory dx = vdt,
where v is the element's instantaneous
velocity.

324
I0 Atmospheric Motion
velocity is then
dx
dt'
(10.2.1)
with components
dx
u~
dt'
"o--
dt'
dz
W ~ ~,
dt
(10.2.2)
Because it is evaluated for fixed ~:, the time derivative in (10.2) corresponds
to an individual material element and therefore to the Lagrangian time rate
of change on which the development has focused until now. By the kinematic
constraint relating Eulerian and Lagrangian descriptions, the velocity in (10.2)
must then equal the field value
v(x, t)
at the material element's position
x(t)
at time t.
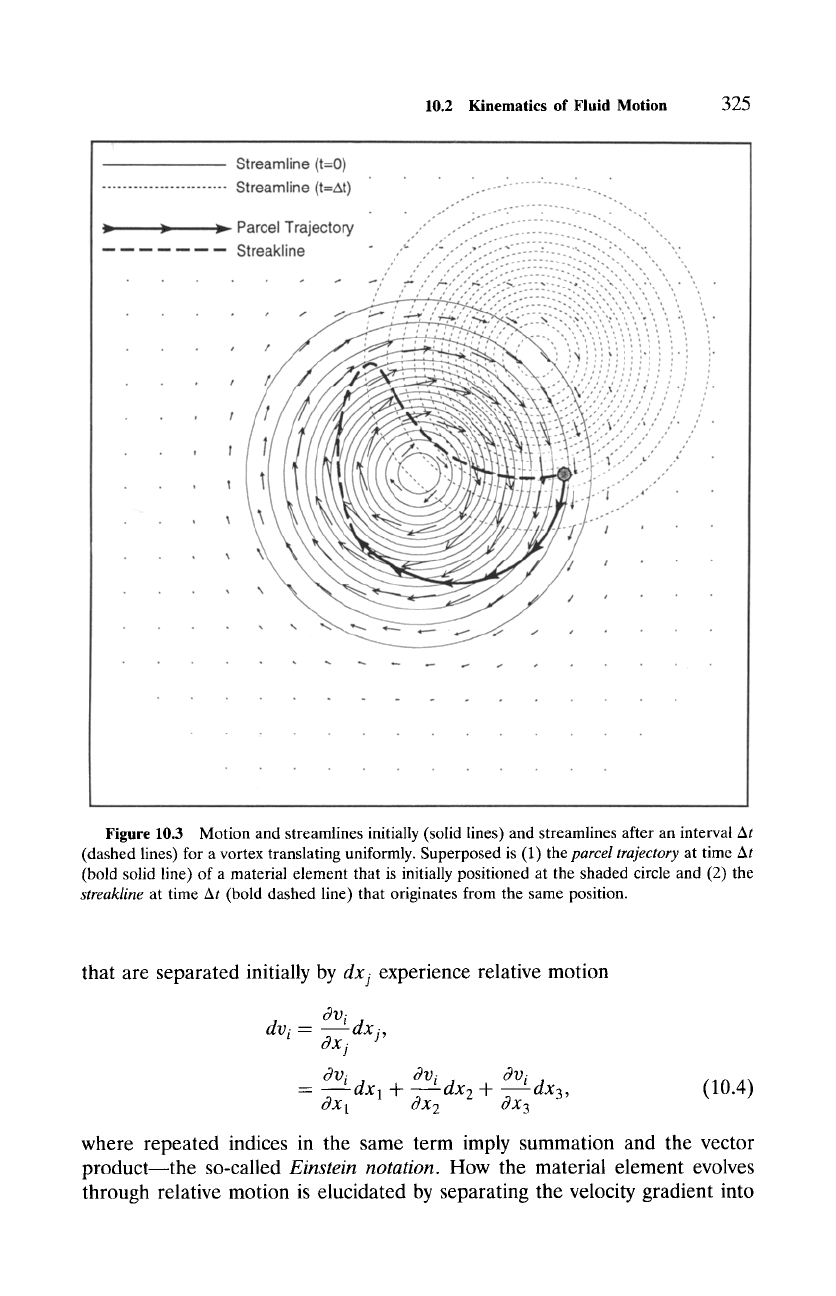
At any instant, the velocity field defines a family of
streamlines
(Fig. 10.3)
that are everywhere tangential to
v(x, t).
If the motion field is
steady,
namely,
if local velocities do not change with time, parcel trajectories coincide with
streamlines. This follows from (10.2) because a material element is then al-
ways displaced along the same streamline, which remains fixed. Under un-
steady conditions, parcel trajectories do not coincide with streamlines, which
then evolve to new positions. Initially tangential to one streamline, the veloc-
ity of a material element will displace it to a different streamline, with which
its velocity is again temporarily tangential. A
streakline,
which also character-
izes the motion, is the locus of points traced out by a dye that is released
into the flow at a particular location. As illustrated in Fig. 10.3, a streakline
represents the positions at a given time of contiguous material elements that
have passed through the location where the tracer is released. Like parcel
trajectories, streaklines coincide with streamlines only if the motion field is
steady.
In addition to translation, a material element can undergo rotation and
deformation as it moves through the circulation. These effects are embodied
in the velocity gradient
o91) i
Vv = ~, i, j = 1, 2, 3, (10.3)
Oxj
which is a two-dimensional tensor with the subscripts i and j referring to three
Cartesian coordinate directions. The local velocity gradient Vv(x) determines
the relative motion between material coordinates and hence the distortion
experienced by the material element located at x. Two material coordinates
10.2 Kinematics of Fluid Motion
325
Streamline (t=O)
........................
Streamline (t=At)
~ ~ Parcel Trajectory
.......
Streakline
-..:.22 2--_--:::..:..-......,.',.',,
', ',
'
t Z "'','",';," '" ," " ," ;' ," '; ':
t
.:. :: ~ .: ., . .,
,,
t "'- I"'.'"" ?" ,
Figure 10.3 Motion and streamlines initially (solid lines) and streamlines after an interval At
(dashed lines) for a vortex translating uniformly. Superposed is (1) the
parcel trajectory
at time At
(bold solid line) of a material element that is initially positioned at the shaded circle and (2) the
streakline
at time At (bold dashed line) that originates from the same position.
that are separated initially by
dxj
experience relative motion
dv i - c~vi dxj,
r3xj
_ ~ 3V i
~ dx 1 + dx 2 + dx 3,
- 3x x ~
(10.4)
where repeated indices in the same term imply summation and the vector
product~the so-called
Einstein notation.
How the material element evolves
through relative motion is elucidated by separating the velocity gradient into

326
10
Atmospheric Motion
symmetric and antisymmetric components"
o~v i
dxj = eij + oJij,
(10.5.1)
where
1( O~vi
O~UJ t (10.5.2)
e ij -- 2 --~x j + O X i / ,
l(dv i dry)
wiJ = -2 dxj dxi . (10.5.3)
The symmetric component
e(x, t)
of the velocity gradient defines the
rate
of strain
or
deformation tensor
acting on the material element at x. Diagonal
components of e, which describe variations of velocity "in the direction of
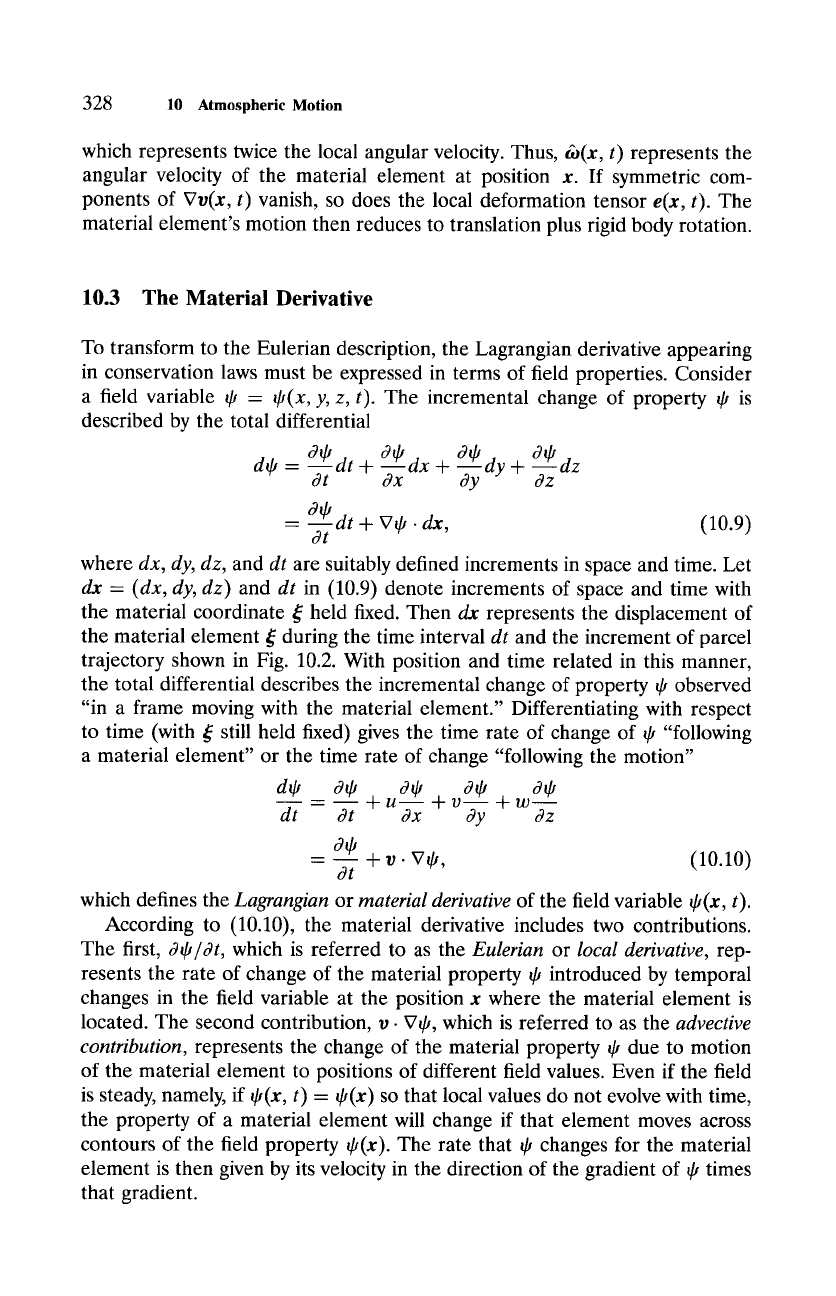
motion," characterize the rate of longitudinal deformation, or "stretching,"
in the three coordinate directions. For example, ell describes the rate of
elongation of a material segment aligned in the direction of coordinate 1
(Fig. 10.4a). Collecting all three components of stretching gives the
trace
of e
and the divergence of the velocity field
o~Ui
= V.v, (10.6)
dx i
which reflects the rate of increase in volume or
dilatation
of the material
element (Problem 10.4). In the case of pure shear, for which the field
v(x, t)
varies only in directions transverse to the motion, diagonal elements of e
vanish. The material element may then undergo distortion, but no change of
volume.
Off-diagonal components of
e(x,
t), which describe variations of velocity
"orthogonal to the motion," characterize the rate of transverse deformation,
or "shear," experienced by the material element at x. As pictured in Fig. 10.4b,
2e12
represents the rate of decrease of the angle between two material seg-
ments aligned initially in the directions of coordinates 1 and 2 (Problem 10.5).
In the case of rectilinear motion that varies only longitudinally, off-diagonal
components of
e(x, t)
vanish. The material element at x may then experience
stretching, but no shear.
The antisymmetric component of
Vv(x, t)
also describes relative motion,
but not deformation. Because it contains only three independent components,
the tensor co defines a vector
= -oJa3 . (10.7)
0.112
The vector ~(x, t) is related to the curl of the motion field or
vorticity
2~b = V x v, (10.8)

X
Xl
Figure
10.4
(a) Stretching
of
a material element
in
a longitudinally varying flow. The element's rate
of
elongation is reflected
in
the
normal strain
rate
el,.
(b)
Shearing
of
a material element in a transversely varying flow. The rate at which the material angle
0
decreases is reflected in the
shear strain
rate e,2.
328 10
Atmospheric Motion
which represents twice the local angular velocity. Thus, go(x, t) represents the
angular velocity of the material element at position x. If symmetric com-
ponents of
Vv(x, t)
vanish, so does the local deformation tensor
e(x, t).
The
material element's motion then reduces to translation plus rigid body rotation.
10.3 The Material Derivative
To transform to the Eulerian description, the Lagrangian derivative appearing
in conservation laws must be expressed in terms of field properties. Consider
a field variable ~O = qJ(x, y, z, t). The incremental change of property ~0 is
described by the total differential
dqJ - dqJ dt + dx + dy + --dz
= ~dt
+ V0. dx, (10.9)
0t
where
dx, dy, dz,
and
dt
are suitably defined increments in space and time. Let
dr. = (dx, dy, dz)
and
dt
in (10.9) denote increments of space and time with
the material coordinate ~: held fixed. Then dx represents the displacement of
the material element ~ during the time interval
dt
and the increment of parcel
trajectory shown in Fig. 10.2. With position and time related in this manner,
the total differential describes the incremental change of property 6 observed
"in a frame moving with the material element." Differentiating with respect
to time (with ~ still held fixed) gives the time rate of change of ~ "following
a material element" or the time rate of change "following the motion"
d t = O t + u --~x + v d--~y + W ~d z
oqJ
= at + v.
V~, (10.10)
which defines the
Lagrangian
or
material derivative
of the field variable ~(x, t).
According to (10.10), the material derivative includes two contributions.
The first,
d~/dt,
which is referred to as the
Eulerian
or
local derivative,
rep-
resents the rate of change of the material property ~, introduced by temporal
changes in the field variable at the position x where the material element is
located. The second contribution, v. V~, which is referred to as the
advective
contribution,
represents the change of the material property ~ due to motion
of the material element to positions of different field values. Even if the field
is steady, namely, if ~,(x, t) = ~(x) so that local values do not evolve with time,
the property of a material element will change if that element moves across
contours of the field property ~,(x). The rate that ~, changes for the material
element is then given by its velocity in the direction of the gradient of ~ times
that gradient.

10.4 Reynolds'
Transport Theorem
329
10.4 Reynolds' Transport Theorem
Owing to the kinematic relationship between Lagrangian and Eulerian de-
scriptions, the material derivative emerges naturally in the laws governing
field properties. Changes of g, described by (10.10) apply to an infinitesimal
material element. Consider now changes for a finite material volume
V(t),
i.e., one containing a fixed collection of matter. Because
V(t)
has finite di-
mension, we must account for variations of velocity v and property q, across
the material volume. Consider the integral property
fv g,(x, y, z, t)dV'
(t)
over the finite system. The time rate of change of this property follows by
differentiating the volume integral. However, the time derivative
d/dt
cannot
be commuted inside the integral because the limits of integration (namely, the
position and form of the material volume) are themselves variable.
The time rate of change of the integral material property has two con-
tributions (Fig. 10.5), analogous to those treated earlier for an infinitesimal
material element:
1. Values of g,(x, t) within the instantaneous material volume change
temporally due to unsteadiness of the field.
2. The material volume moves to regions of different field values. Rel-
ative to a frame moving with
V(t),
such motion introduces a flux of
property q, across the material volume's surface
S(t).
The first contribution is just the collective time rate of change of q,(x, t) within
the material volume:
(t) ~t
The second is the net transfer of ~ across the boundary of
V(t).
If
S(t)
has
the local outward normal n and local velocity v, the local flux of ~p relative to
that section of material surface is -~v. Then the flux of ~ into the material
volume is given by -~pv.-n = ~pv. n. Integrating over
S(t)
gives the net
rate that ~ is transferred into the material volume
V(t)
due to motion across
contours of ~:
f
(t) ~v . ndS'.
Collecting the two contributions and applying Gauss' theorem obtains
dt
(t) (t) ~ + V. (vq~)
dV'
(t) --d7 + q~V. v dV'
(10.11)
