Salby M.L. Fundamentals of Atmospheric Physics

Подождите немного. Документ загружается.

330

10 Atmospheric Motion

~ " ~ ~S(t+dt)

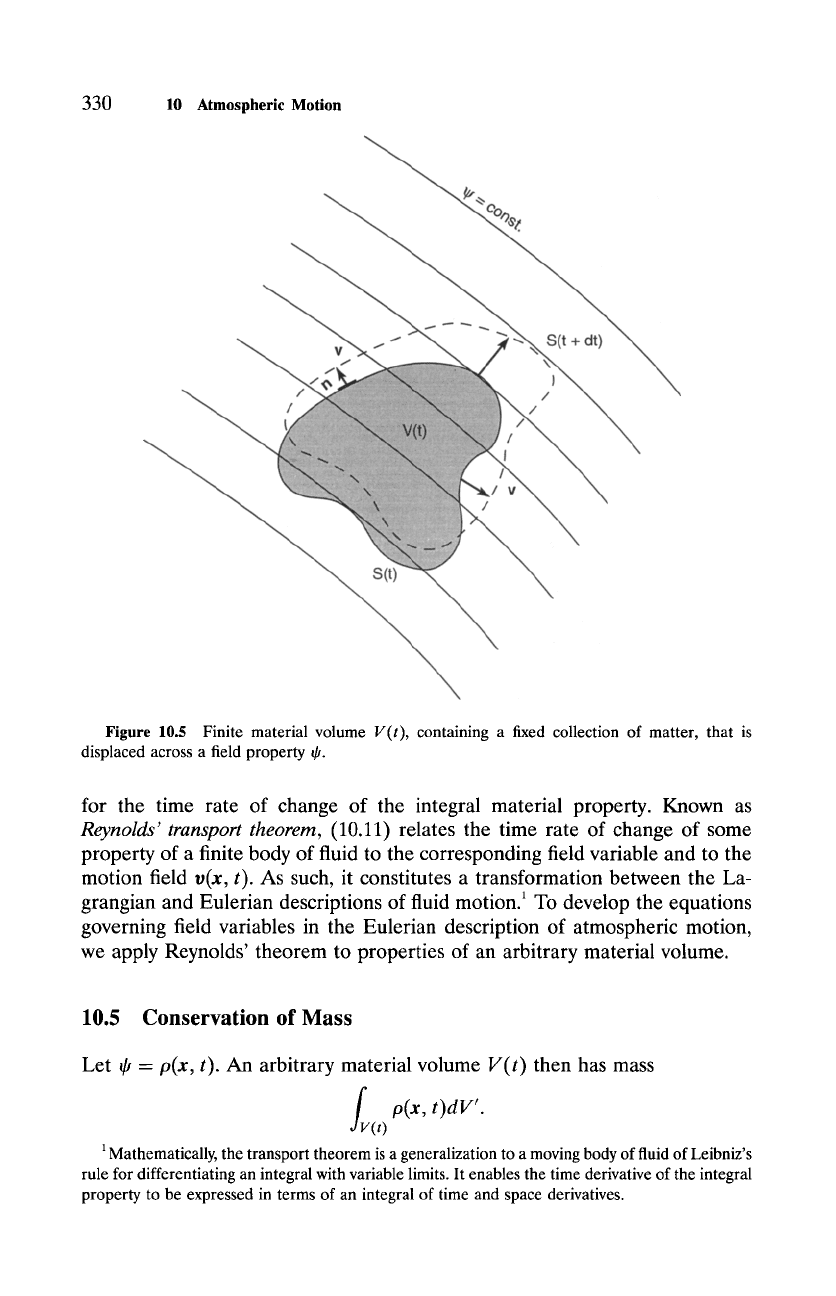
Figure 10.5

Finite material volume V(t), containing a fixed collection of matter, that is

displaced across a field property q,.

for the time rate of change of the integral material property. Known as

Reynolds' transport theorem,

(10.11) relates the time rate of change of some

property of a finite body of fluid to the corresponding field variable and to the

motion field

v(x, t).

As such, it constitutes a transformation between the La-

grangian and Eulerian descriptions of fluid motion. ~ To develop the equations

governing field variables in the Eulerian description of atmospheric motion,

we apply Reynolds' theorem to properties of an arbitrary material volume.

10.5 Conservation of Mass

Let @ =

p(x, t). An

arbitrary material volume

V(t)

then has mass

v(t) p(x, t)dV'.

1 Mathematically, the transport theorem is a generalization to a moving body of fluid of Leibniz's

rule for differentiating an integral with variable limits. It enables the time derivative of the integral

property tobe expressed in terms of an integral of time and space derivatives.

10.6 The Momentum Budget 331

Because the system is comprised of a fixed collection of matter, the time rate

of change of its mass must vanish

p(x, t)dV'

= 0. (10.12)

dt (r

Applying Reynolds' transport theorem transforms (10.12) into

(t) -~ + pV. v dV' = O.

(10.13)

This relation must hold for "arbitrary" material volume

V(t).

It follows that

the quantity in braces must vanish identically. Thus, conservation of mass for

individual bodies of air requires

d---t -t- pV-v- 0 (10.14.1)

-- + V . (pv) = O,

(10.14.2)

which provides a constraint on the field variables p(x, t) and

v(x, t).

Known

as the

continuity equation,

(10.14) must hold pointwise throughout the domain

and continuously in time.

Consider a specific field property f (namely, one referenced to a unit mass).

Then

tp = pf

represents the absolute concentration of that property. Reynolds'

transport theorem implies

dtdfgpfdg'-fg{d(t)

(t) }

--~(pf) + pf(V. v) dV'

: + v]}

(10.15)

By the continuity equation, the term in square brackets vanishes, so (10.15)

reduces to the identity

dt fv(t) pfdV' = fv(t) P~tdV'.

(10.16)

Hence, for an absolute concentration, the time rate of change of an integral

material property assumes this simpler form.

10.6 The Momentum Budget

10.6.1 Cauchy's Equations of Motion

In an inertial reference frame, Newton's second law of motion applied to the

material volume

V(t)

can be expressed

d fv

pvdV' = fv

pf dV' + fs r ndS',

(10.17)

dt (t) (t) (t)

332

Atmospheric Motion

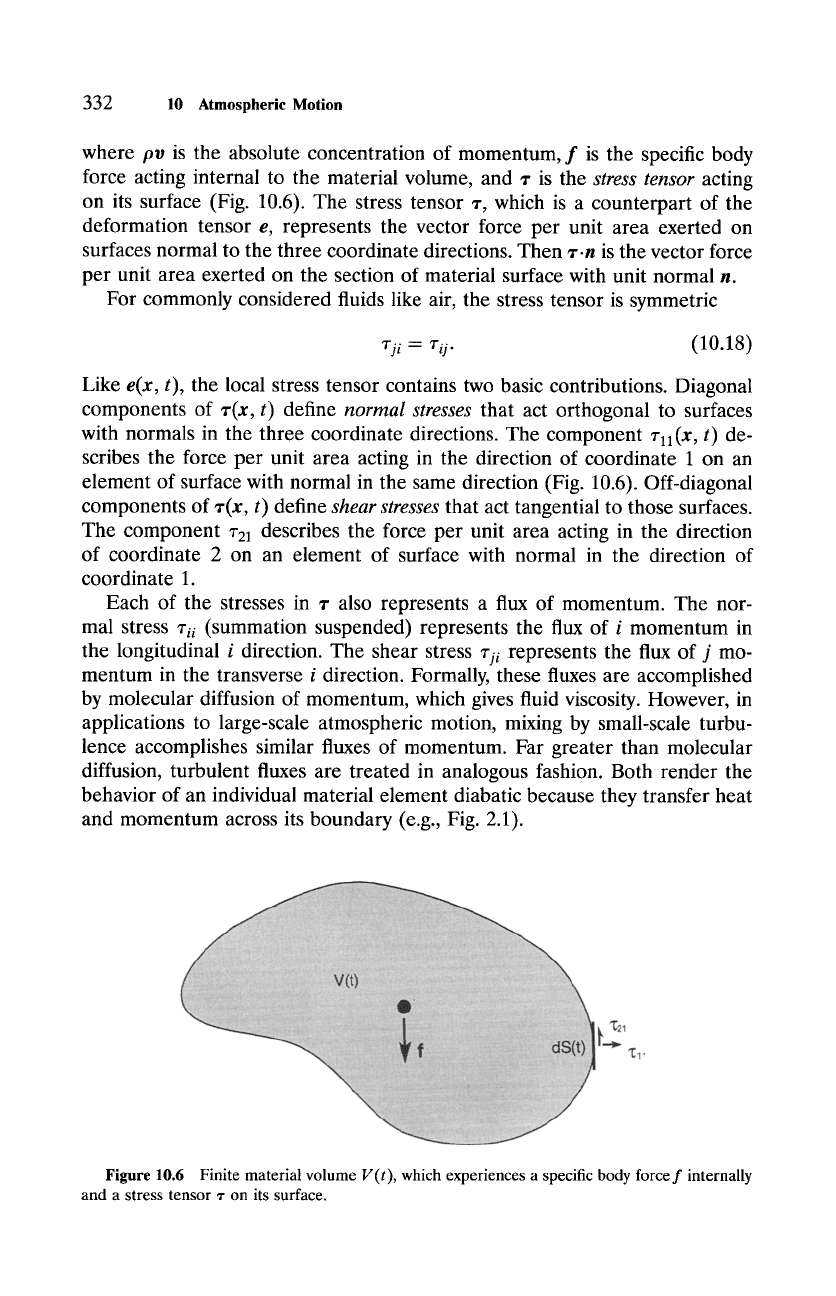
where

is the absolute concentration of momentum, f is the specific body

force acting internal to the material volume, and ~- is the

stress tensor

acting

on its surface (Fig. 10.6). The stress tensor 7, which is a counterpart of the

deformation tensor e, represents the vector force per unit area exerted on

surfaces normal to the three coordinate directions. Then ~'.n is the vector force

per unit area exerted on the section of material surface with unit normal n.

For commonly considered fluids like air, the stress tensor is symmetric

Tji--

T 0.

(10.18)

e(x, t),

the local stress tensor contains two basic contributions. Diagonal

components of ~-(x, t) define

normal stresses

that act orthogonal to surfaces

with normals in the three coordinate directions. The component ~'11(x, t) de-

scribes the force per unit area acting in the direction of coordinate 1 on an

element of surface with normal in the same direction (Fig. 10.6). Off-diagonal

components of ~-(x, t) define

shear stresses

that act tangential to those surfaces.

The component ~'2a describes the force per unit area acting in the direction

of coordinate 2 on an element of surface with normal in the direction of

coordinate 1.

Each of the stresses in ~- also represents a flux of momentum. The nor-

mal stress

"rii

(summation suspended) represents the flux of i momentum in

the longitudinal i direction. The shear stress

Tji

represents the flux of j mo-

mentum in the transverse i direction. Formally, these fluxes are accomplished

by molecular diffusion of momentum, which gives fluid viscosity. However, in

applications to large-scale atmospheric motion, mixing by small-scale turbu-

lence accomplishes similar fluxes of momentum. Far greater than molecular

diffusion, turbulent fluxes are treated in analogous fashion. Both render the

behavior of an individual material element diabatic because they transfer heat

and momentum across its boundary (e.g., Fig. 2.1).

~11

Figure 10.6 Finite material volume V(t), which experiences a specific body force f internally

and a stress tensor ~" on its surface.

10.6

The Momentum Budget

333

In the atmosphere, the body force and stress tensor are either prescribed or

determined by the motion field. Incorporating Reynolds' transport theorem for

an absolute concentration (10.16) and Gauss' theorem transforms (10.17) into

fv dv

p-~dV' = {pf + V. ~} dV'

(t) (t)

(t) P-d-[ - pf - V. ~- dV' = O.

(10.19)

As before, (10.19) must hold for arbitrary material volume, so the quantity

in braces must vanish identically. Thus, Newton's second law for individual

bodies of air requires

P -d-[ - of

+ v.~-, (10.20)

which provides constraints on the field properties

p(x, t)

and

v(x, t).

Known

Cauchy's equations,

(10.20) hold pointwise throughout the domain and con-

tinuously in time. 2

The body force relevant to the atmosphere is gravity

f =g, (10.21)

which is prescribed. On the other hand, internal stresses are determined au-

tonomously by the motion. A

Newtonian fluid

like air has a stress tensor that

is linearly proportional to the local rate of strain

e(x, t)

and that, in the ab-

sence of motion, reduces to the normal stresses exerted by pressure. For our

purposes, it suffices to define the stress tensor as

2txe ij

"r m

-p

2 tze ji

-p_

(10.22)

where/, is the

coefficient of viscosity. 3

Equation (10.22) is the form assumed

by the stress tensor for an incompressible fluid (Problem 10.16). Shear stresses

internal to the fluid then arise from shear in the motion field

dvi/dxj.

When

expressed in terms of specific momentum, the governing equations involve

the

kinematic viscosity v = Iz/p,

which is also termed the molecular

diffusivity

and has dimensions length/time 2. Turbulent momentum transfer is frequently

modeled as diffusion, but with an eddy diffusivity in place of v (Chapter 13).

2 In the absence of friction, these are called

Euler's equations.

3Strictly, normal stresses also include a contribution from viscosity, but that effect is small

enough to be neglected for most applications (see, e.g., Aris, 1962).

334

10 Atmospheric Motion

where

With r defined by (10.22), Cauchy's equations reduce to

dv 1

dt = g - -Vp - D, (10.23.1)

D- --V 9 ~-

1 1 o ~

691) i

= --V. (/zVv) .... /z~ (10.23.2)

p p tgXj o~Xj

denotes the specific

drag force

exerted on the material element at location x.

Known as the

momentum equations, (10.23)

are a simplified form of the

Navier

Stokes equations,

which embody the full representation of ~- in terms of e and

constitute the formal description of fluid motion. Conceptually, (10.23) asserts

that the momentum of a material element changes according to the resultant

force exerted on it by gravity, pressure gradient, and frictional drag.

10.6.2 Momentum Equations in a Rotating Reference Frame

Because they follow from Newton's laws of motion, the momentum equations

apply in an inertial reference frame. The reference frame of the earth (in which

we observe atmospheric motion), however, is rotating and therefore noniner-

tial. Consequently, the momentum equations must be modified to apply in

that reference frame. Scalar quantities like

p(x, t)

appear the same in inertial

and noninertial reference frames, as do their Lagrangian derivatives. However,

vector quantities differ between those reference frames. Vector variables de-

scribing a material element's motion (e.g., x, v =

dx/dt,

and a =

dv/dt)

must

therefore be corrected to account for acceleration of the earth's reference

frame.

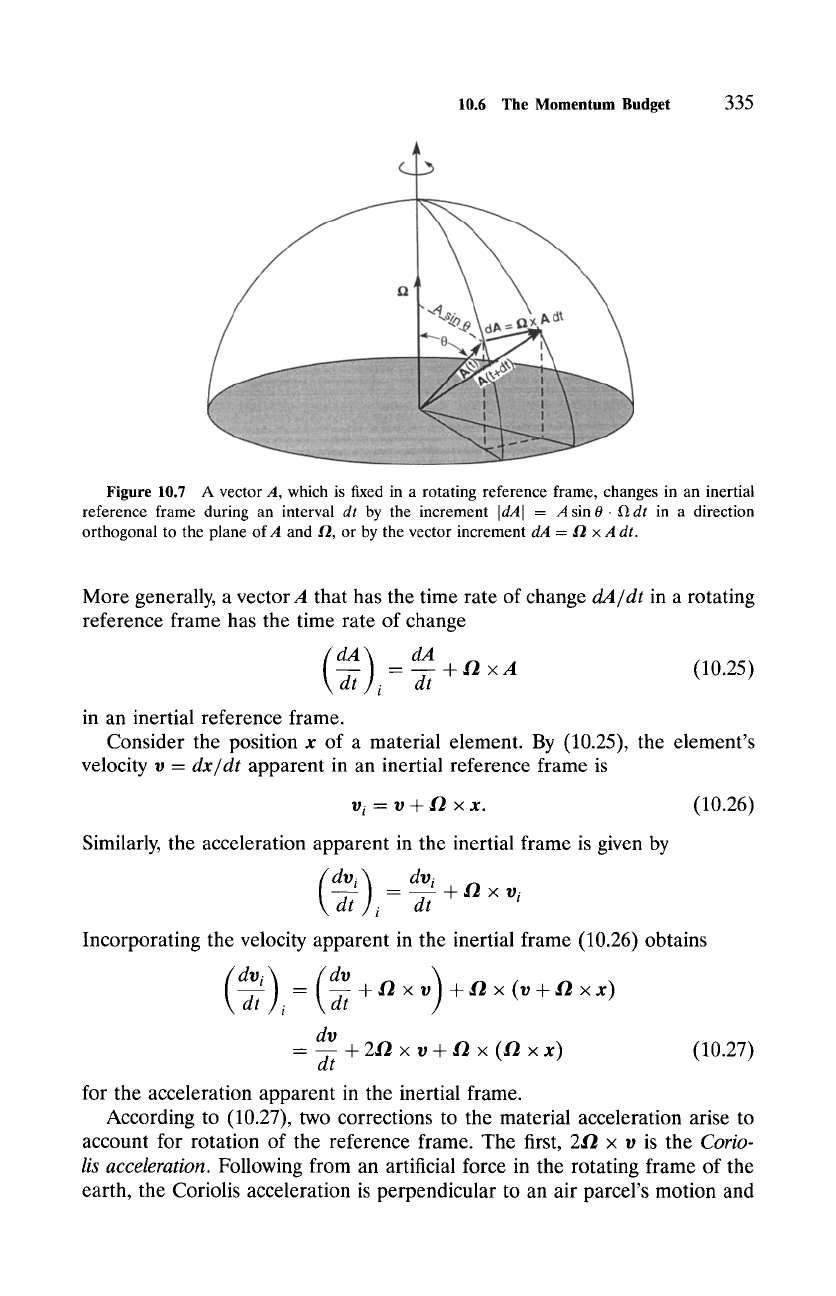
Consider a reference frame rotating with angular velocity g~ (Fig. 10.7). A

vector A that is constant in that frame must rotate when viewed in an inertial

reference frame. During an interval

dt, A

will change by a vector increment

dA,

which is perpendicular to the plane of A and g~ and has magnitude

Ida[

- A sin 0.1~

dt,

where 0 is the angle between A and J2. Hence, in an inertial reference frame,

the vector A changes at the rate

= AOsin 0

and in a direction perpendicular to the plane of A and J2. It follows that the

time rate of change of A apparent in an inertial reference frame is described by

\ ~/--d-t" i

10.6

The Momentum Budget

335

Figure 10.7 A vector A, which is fixed in a rotating reference frame, changes in an inertial

reference flame during an interval

by the increment

IdA] = A

sin 0-gldt in a direction

orthogonal to the plane of A and g2, or by the vector increment

dA = g~ x A dt.

More generally, a vector A that has the time rate of change

dA/dt

in a rotating

reference frame has the time rate of change

-37 i -37 + • a (10.25)

in an inertial reference frame.

Consider the position x of a material element. By (10.25), the element's

velocity v =

dx/dt

apparent in an inertial reference frame is

1) i ~-- 11 -Jr- ~ X X.

(10.26)

Similarly, the acceleration apparent in the inertial frame is given by

(dvi)- dvi

--~ i -'~-{-~ X V i

Incorporating the velocity apparent in the inertial frame (10.26) obtains

dt i ~" +oxv

+~x(v+~xx)

= dt +2oxv+ox(oxx)

(10.27)

for the acceleration apparent in the inertial frame.

According to (10.27), two corrections to the material acceleration arise to

account for rotation of the reference frame. The first, 2g~ x v is the

Corio-

lis acceleration.

Following from an artificial force in the rotating frame of the

earth, the Coriolis acceleration is perpendicular to an air parcel's motion and

336

Atmospheric Motion

to the

planetary vorticity

2g~. It is important for motions with timescales com-

parable to that of the earth's rotation (Chapter 12). The second correction:

g~ • (.Q • x) is the

centrifugal acceleration

of an air parcel due to the earth's

rotation, which was treated in Chapter 6. When geopotential coordinates are

used, the artificial force corresponding to this correction is automatically ab-

sorbed into effective gravity.

Incorporating the material acceleration apparent in an inertial reference

frame (10.27) transforms the momentum equations into a form valid in the

rotating frame of the earth:

dv 1

+ 20 x v - --Vp- gk- D,

(10.28)

dt p

where g is understood to denote effective gravity and k the upward normal

in the direction of increasing geopotential (i.e.,

= V~). The correction

2~ • v enters as the "Coriolis acceleration" when it appears on the left-hand

side of the momentum balance. Moved to the right-hand side, it enters as the

"Coriolis force" -2g~ • v, a fictitious force that appears to act on a material

element in the rotating frame of the earth. Because it acts orthogonal to the

displacement, the Coriolis force performs no work on the material element.

10.7 The First Law of Thermodynamics

Applied to the material volume V(t), the first law can be expressed

is do

d pcvTdV' - - q. ndS' - pp-d-[dV' + pgldV',

-dt

(t) (t) (t) (t)

(10.29)

where

coT

represents the specific internal energy. Forcing it on the right-hand

side, q is the local heat flux so

-q.n

represents the heat flux "into" the material

volume, a =

1/p

is the specific volume so

p(da/dt)

represents the specific

work rate, and q denotes the specific rate of internal heating (e.g., associated

with the latent heat release and frictional dissipation of motion).

The local rate of expansion work is related to the dilatation of the material

element occupying that position. Because

l dp d (~)

p dt dt

1 dc~

adt'

(10.30)

the continuity equation (10.14) can be expressed

1 da

a dt

=V.v.

(10.31)

10.7

The First Law of Thermodynamics

337

Incorporating (10.31) along with Reynolds' transport theorem (10.16) and

Gauss' theorem transforms (10.29) into

pc,, + V + pV Pit dV'

(t) --~ " q 9 v - = O.

Again, since

V(t)

is arbitrary, the quantity in braces must vanish identically.

Therefore, the first law applied to individual bodies of air requires

= -V.q- pV. v + PO,

(10.32)

pCv dt

which provides a constraint on the field variables involved. Of the three ther-

modynamic properties represented, only two are independent because they

must also satisfy the gas law. Similarly, the heat flux q and the internal heat-

ing rate c) are determined autonomously by properties already represented, so

they introduce no additional unknowns.

It is convenient to separate the heat flux into radiative and diffusive com-

ponents:

q -- qR + qT

=F-kVT,

(10.33)

where F is the net radiative flux (Sec. 8.2) and k denotes the thermal conduc-

tivity in Fourier's law of heat conduction. The first law then becomes

pc v

~-~ + pV. v - -V. F + V. (kVT) +

Oil.

(10.34)

Known as the

thermodynamic equation,

(10.34) expresses the rate that a ma-

terial element's internal energy changes in terms of the rate that work is per-

formed on it and the net rate it absorbs heat through convergence of radiative

and diffusive energy fluxes.

The thermodynamic equation can be expressed more compactly in terms of

potential temperature. For an individual air parcel, the fundamental relation

for internal energy

du - Tds - pda

(10.35.1)

relates the change of entropy to other material properties. Incorporating the

identity between entropy and potential temperature (3.25) transforms this into

cpTdln 0 - du + pda.

(10.35.2)

Differentiating with respect to time, with the material coordinate ~ held fixed,

obtains

d In 0

du dee

cpT d-----~ - dt + p dt '

(10.36)

338 10 Atmospheric Motion

where d/dt represents the Lagrangian derivative (10.10). Multiplying by p and

introducing the continuity equation (10.4) transforms this into

pcpT dO dT

0 dt = PCv--~ + pV. v. (10.37)

Then incorporating (10.37) into (10.34) absorbs the compression work into the

time rate of change of 0 to yield the thermodynamic equation

cpT dO

P--if- d-~ = -V. F + V. (kVT) + pq. (10.38)

Collectively, the continuity, momentum, and thermodynamic equations

represent five partial differential equations in five dependent field variables:

three components of motion and two independent thermodynamic proper-

ties. Advective contributions to the material derivative, like advection of mo-

mentum v. Vv and of temperature v. VT, make the governing equations

quadratically nonlinear. Their solution requires initial conditions that specify

the preliminary state of the atmosphere and boundary conditions that specify

its properties along physical borders. Referred to as the equations of motion,

these equations govern the behavior of a compressible, stratified atmosphere

in a rotating reference flame. As such, they constitute, the starting point for

dynamical investigations, as well for investigations of chemistry and radiation

in the presence of motion.