Salby M.L. Fundamentals of Atmospheric Physics
Подождите немного. Документ загружается.


340
I0 Atmospheric Motion
10.12.
10.13.
10.14.
10.15.
10.16.
10.17.
Demonstrate that the Coriolis force does not enter the budget of spe-
cific kinetic energy Ivl2/2.
Show that the vorticity ~" = k. 7 • v is a conserved property for two-
dimensional nondivergent motion in an inertial reference frame.
Simplify the equations of motion for the special case of (a) incompress-
ible motion, wherein the volume of a material element is conserved,
and (b) adiabatic motion.
Describe the circumstances under which a property qJ is conserved, yet
varies spatially in a steady flow.
The stress tensor in a Newtonian fluid is described by
Tij _P6ij_q_(tZ,
2)
= - -~/x 6ijV. v + 2/~eij,
where/x' is the so-called
bulk viscosity.
(a) Determine the average of
the normal stresses in the three coordinate directions and discuss its
relationship to the thermodynamic pressure p. (b) As in part (a), but
for/z' = 0, which describes a
Stokesian fluid.
(c) Show that the stress
tensor reduces to (10.22) and the drag to (10.23.2) if the motion is
incompressible.
A free surface is one that moves to alleviate any stress and maintain
z = 0. If the rate of strain tensor is dominated by the vertical shears
Ou/Oz
and
Ov/dz,
describe how the horizontal velocity varies adjacent
to a free surface.
10.18.
A geostationary satellite is positioned over 30 ~ latitude and 0 ~ longi-
tude. From it, a projectile is fired northward at a speed v0. By assuming
the deflection of the projectile's trajectory to be small and ignoring
sphericity and the satellite's altitude, estimate the longitude where the
projectile crosses 45 ~ latitude if (a) v0 = 1000 m s -], (b) v0 = 100
m s -1,
and (c) v0 = 10 ms -1. (d) For each of the preceding results, evaluate
the dimensionless timescale for the traversal scaled by that for rotation
of the earth.
10.19. Consider a parcel with local speed v, the natural coordinate s measured
along the parcel's trajectory, and a unit vector s that is everywhere
tangential to the trajectory. (a) Express the material derivative in terms
of v, s, and s. (b) Show that the material acceleration is described by
dv dv ds
dt dt dt
(c) Interpret the two accelerations appearing on the right.
10.20. In terms of Newton's third law, explain how turbulent mixing can exert
drag on a moving air parcel.

Chapter II
Atmospheric Equations of Motion
In vector form, the equations of motion are valid in any coordinate system.
However, those equations do not lend themselves to application and standard
methods of solution. To be useful, the governing equations must be expressed
in scalar form, which then depend on the coordinate system. We develop
the scalar equations of motion within the general framework of curvilinear
coordinates. In addition to accounting for geometric distortions inherent to
spherical coordinates, that framework allows a straightforward development
of the equations in thermodynamic coordinates, which afford a number of
simplifications.
II.I Curvilinear Coordinates
Consider the Cartesian coordinates
X---(XI, X2, X3) ,
(11.1.1)
which are measured from planar coordinate surfaces: x i
--
const. If those
planes are perpendicular, (Xl, x2, x3) are referred to as rectangular Cartesian
coordinates. More generally, the curvilinear coordinates
.1~ = ('~1, "~2, X3)
(11.1.2)
are measured from coordinate surfaces that need not be planar nor mutually
orthogonal (Fig. 11.1). Insofar as x and ,~ are both viable coordinate systems,
there exists a transformation
.~ = .~(x) (11.2)
from one representation to the other, which constitutes a mapping between
all coordinates x in the original system and coordinates ,~ in the curvilinear
coordinate system. The mapping (11.2) is unique and therefore invertible,
provided that the Jacobian of the transformation
J(x, .r) =
~Xi
(11.3)
341
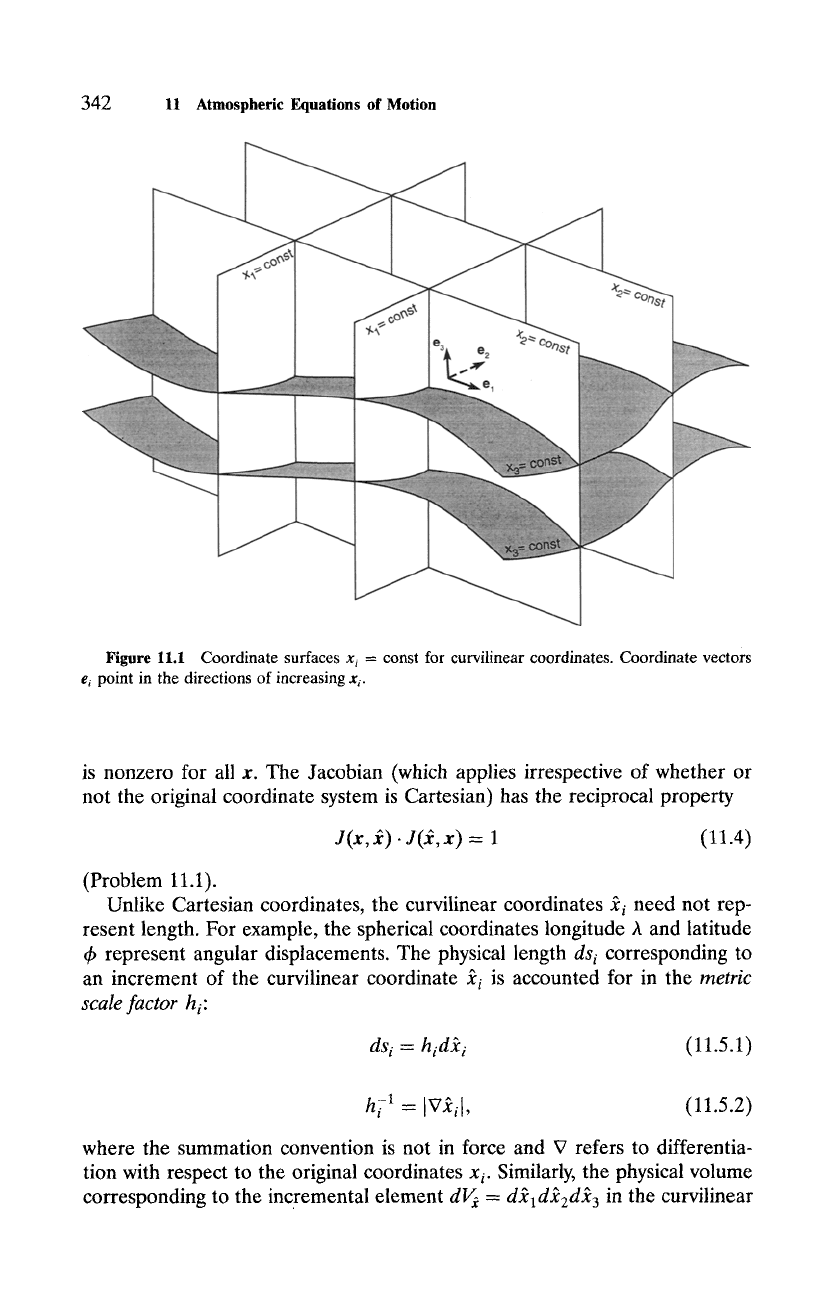
342
11
Atmospheric Equations of Motion
J
Figure ll.! Coordinate surfaces x i = const for curvilinear coordinates. Coordinate vectors
ei point in the directions of increasing xi.
is nonzero for all x. The Jacobian (which applies irrespective of whether or
not the original coordinate system is Cartesian) has the reciprocal property
J(x,.~)-
J(.f,x)
= 1 (11.4)
(Problem 11.1).
Unlike Cartesian coordinates, the curvilinear coordinates 2i need not rep-
resent length. For example, the spherical coordinates longitude A and latitude
~b represent angular displacements. The physical length
dsi
corresponding to
an increment of the curvilinear coordinate
-~i is
accounted for in the
metric
scale factor hi:
ds i = hid2 i
(11.5.1)
hi -1--- hV~i[, (11.5.2)
where the summation convention is not in force and V refers to differentia-
tion with respect to the original coordinates
x i.
Similarly, the physical volume
corresponding to the incremental element dV~ =
dYcldic2dYc 3
in the curvilinear
11.1 Curvilinear Coordinates
343
coordinate system is given by
dE -
1
= ~d~. (11.6)
The curvilinear coordinate system is said to be
orthogonal
if coordinate
surfaces are mutually perpendicular. Under those circumstances, coordinate
vectors ei, which point in the directions of increasing 2 i (Fig. 11.1), are likewise
mutually perpendicular
Then the Jacobian reduces to
ei "ej - ~/j.
J(~,x)-
hlh2h3
(11.7)
(Problem 11.2) and the physical volume corresponding to the element
d21d22d23
in the curvilinear system is just the product of the corresponding
physical lengths (11.5).
The Jacobian accounts for distortions of physical length in all three curvi-
linear coordinates. In the special case when only the third coordinate is trans-
formed
,I~(X) -- (X1, X2, "~3) (11.8)
(e.g., when surfaces of constant height are replaced by isobaric surfaces), the
Jacobian is given by
J(x,,) -
1 0 0
0 1 0
a2_._13 a2_.._!3 02..__!3
o~X 1 o~X 2 o~X 3
023
Ox3 "
(11.9)
For this special class of transformations, (11.4) reduces to the simple reciprocal
property
1
= , (11.10)
ax3 \ a23 /
which is not true in general.
344
11 Atmospheric Equations of Motion
Vector operations in arbitrary curvilinear coordinates can now be expressed
in terms of the corresponding metric scale factors:
1 00 1 0r ^ 1
00
gilt = h--71 0 x---71 ~1 -~ g-~x2
e 2 -- h--3 0 x---3 ~" 3 '
(11.11.1)
V.A=
e e ]
1
3 (hgh3A1) +
(h
h3A2) +
(hlhaA3) (11.11.2)
hl h2h3 ~ 1 ~X 3 ,
VxA=
hie 1 h2~'2 h3e 3
a d d
021 ax 2 ax 3
hlZl h2A2 h3A3
(11.11.3)
V21// --
1
hlh2h3 ~Xl hi 021
0 (hlh30~)
d (hlh20@)]
q- ~2 h2 d3:2 q-~3 h3 0J:3 "
(11.11.4)
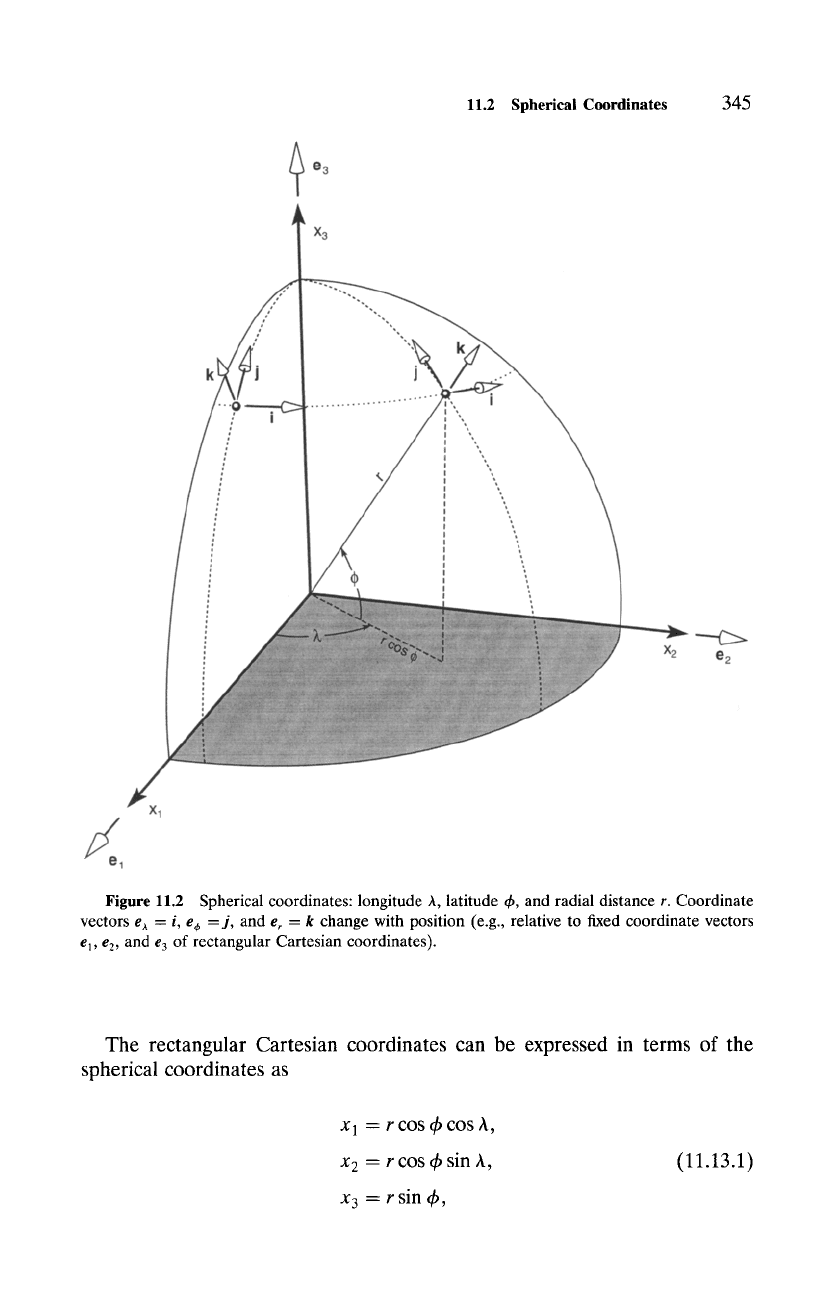
11.2 Spherical Coordinates
Consider the rectangular Cartesian coordinates x
= (Xl, x2, x3)
having origin
at the center of the earth and the spherical coordinates
a~ = (A, ~b, r),
both fixed with respect to the earth (Fig. 11.2). Spherical coordinate surfaces
A = const, 4~ = const, and r = const are then (1) vertical planes intersecting
the Cartesian x3 axis, (2) conical shells with apex at the origin x = 0, and
(3) concentric spherical shells, respectively. The corresponding unit vectors
ex -- i,
e, =j,
e r -- k,
(11.12)
are everywhere perpendicular to those coordinate surfaces. Because coordi-
nate surfaces are mutually perpendicular, so are the unit vectors, and the
spherical coordinates (A, ~b, r) constitute an orthogonal coordinate system.
11.2 Spherical Coordinates
345
/ :
,
,
~
ea
I"
"'%
---C>
e2
x1
Figure 11.2 Spherical coordinates: longitude A, latitude 4', and radial distance r. Coordinate
vectors
e, = i, e~ =j,
and er = k change with position (e.g., relative to fixed coordinate vectors
el, e2, and e3 of rectangular Cartesian coordinates).
The rectangular Cartesian coordinates can be expressed in terms of the
spherical coordinates as
x l - r cos ~b cos A,
X 2 --
r cos th sin A,
X 3 --
rsin ~b,
(11.13.1)
346 11 Atmospheric Equations of Motion
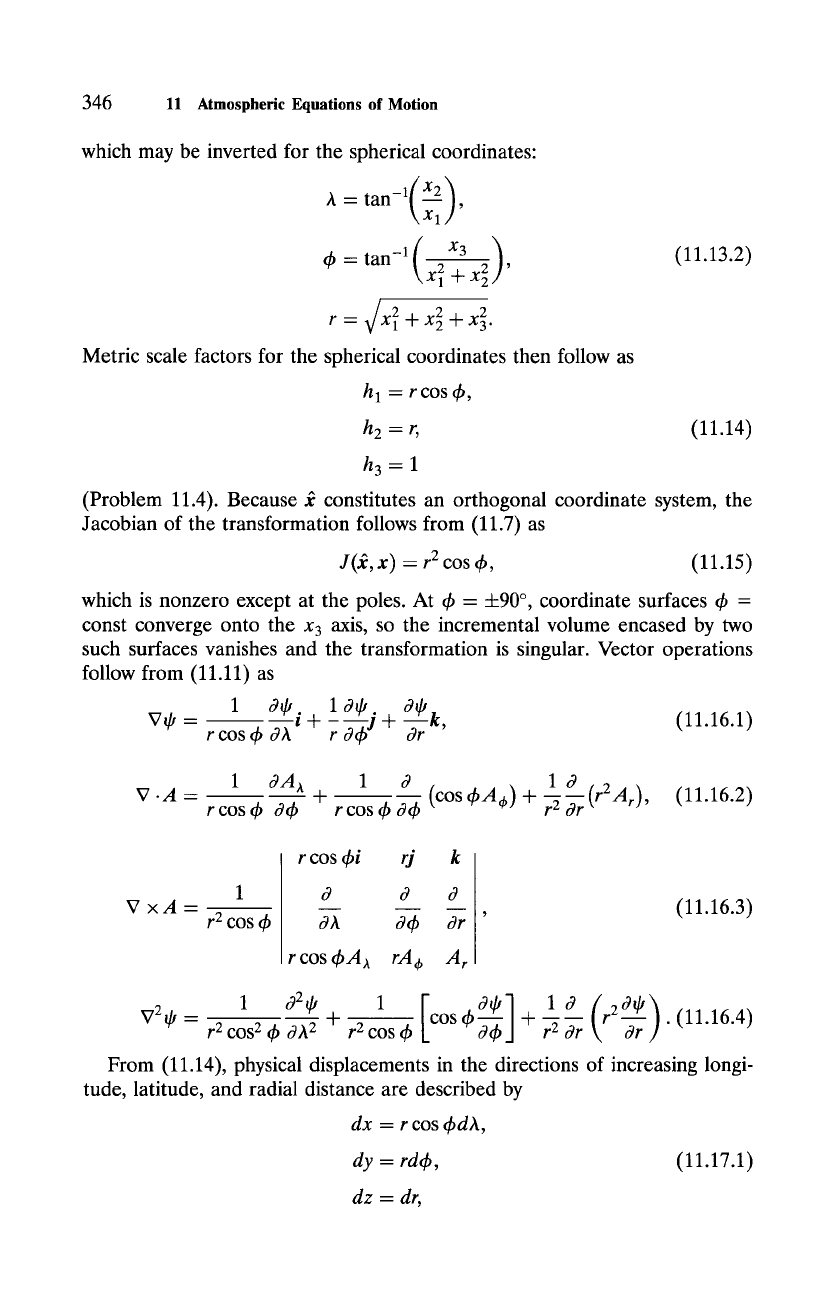
which may be inverted for the spherical coordinates:
h - tan-l(X2),\Xl
4~ = tan -1
+ '
r- V/x + +
Metric scale factors for the spherical coordinates then follow as
h 1 = rcos ~b,
(11.13.2)
h 2 -- r, (11.14)
h3=l
(Problem 11.4). Because .s constitutes an orthogonal coordinate system, the
Jacobian of the transformation follows from (11.7) as
J(.~,x)
= r 2 cos q~, (11.15)
which is nonzero except at the poles. At ~b = +90 ~ coordinate surfaces ~b =
const converge onto the x3 axis, so the incremental volume encased by two
such surfaces vanishes and the transformation is singular. Vector operations
follow from (11.11) as
100.
1 OqJi + - + 3qJk
(11.16.1)
V~ -- r cos
qb 3A r -~J dr '
1 3A x 1 d 1 3
V-A = t (cos
dpA4, ) + (rZAr)
(11.16.2)
r cos q~ 3~b r cos ~b 3~b ~ ~rr '
VxA=
r 2 COS ~b
r cos
dpi rj k
0 0 0
OA 3dp Or
r cos
dpAa rAq,
A r
(11.16.3)
1
t~ O~2~O~A 2
r2 cos q ~1 [ d~] r-2~rrl 3 (0q/)
V2~ -- rZcos2 ~ cos q~--~ + r 2~ . (11.16.4)
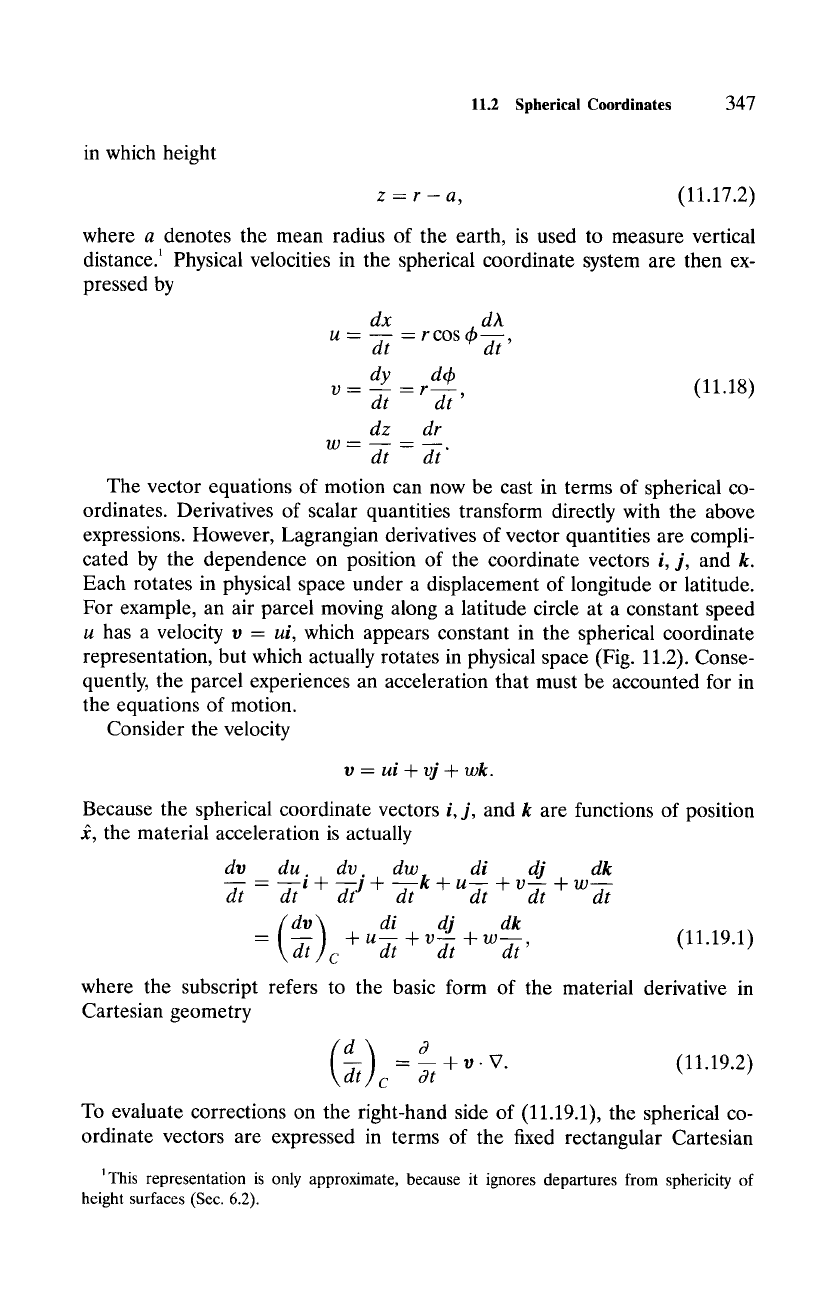
From (11.14), physical displacements in the directions of increasing longi-
tude, latitude, and radial distance are described by
dx = r
cos ~bdA,
dy
= rd~b, (11.17.1)
dz = dr,
11.2 Spherical Coordinates 347
in which height
z -- r - a, (11.17.2)
where a denotes the mean radius of the earth, is used to measure vertical
distance. 1 Physical velocities in the spherical coordinate system are then ex-
pressed by
dx dA
u - dt
= rcos~b
dt'
dy dcb
(11 18)
v= dt-rd--7'
dz dr
dt dt
The vector equations of motion can now be cast in terms of spherical co-
ordinates. Derivatives of scalar quantities transform directly with the above
expressions. However, Lagrangian derivatives of vector quantities are compli-
cated by the dependence on position of the coordinate vectors i, j, and k.
Each rotates in physical space under a displacement of longitude or latitude.
For example, an air parcel moving along a latitude circle at a constant speed
u has a velocity v =
ui,
which appears constant in the spherical coordinate
representation, but which actually rotates in physical space (Fig. 11.2). Conse-
quently, the parcel experiences an acceleration that must be accounted for in
the equations of motion.
Consider the velocity
v = ui + vj +
wk.
Because the spherical coordinate vectors i, j, and k are functions of position
.~, the material acceleration is actually
dv du . dr. dW k di dj dk
dt - --dT' + --d7 J + --dt + u-~ + v-~ + w d--t
(dr) didjdk
- -s + + + W-dT,
(11.19.1)
where the subscript refers to the basic form of the material derivative in
Cartesian geometry
d-t
c ~ + v.V. (11.19.2)
To evaluate corrections on the right-hand side of (11.19.1), the spherical co-
ordinate vectors are expressed in terms of the fixed rectangular Cartesian
~This representation is only approximate, because it ignores departures from sphericity of
height surfaces (Sec. 6.2).
348
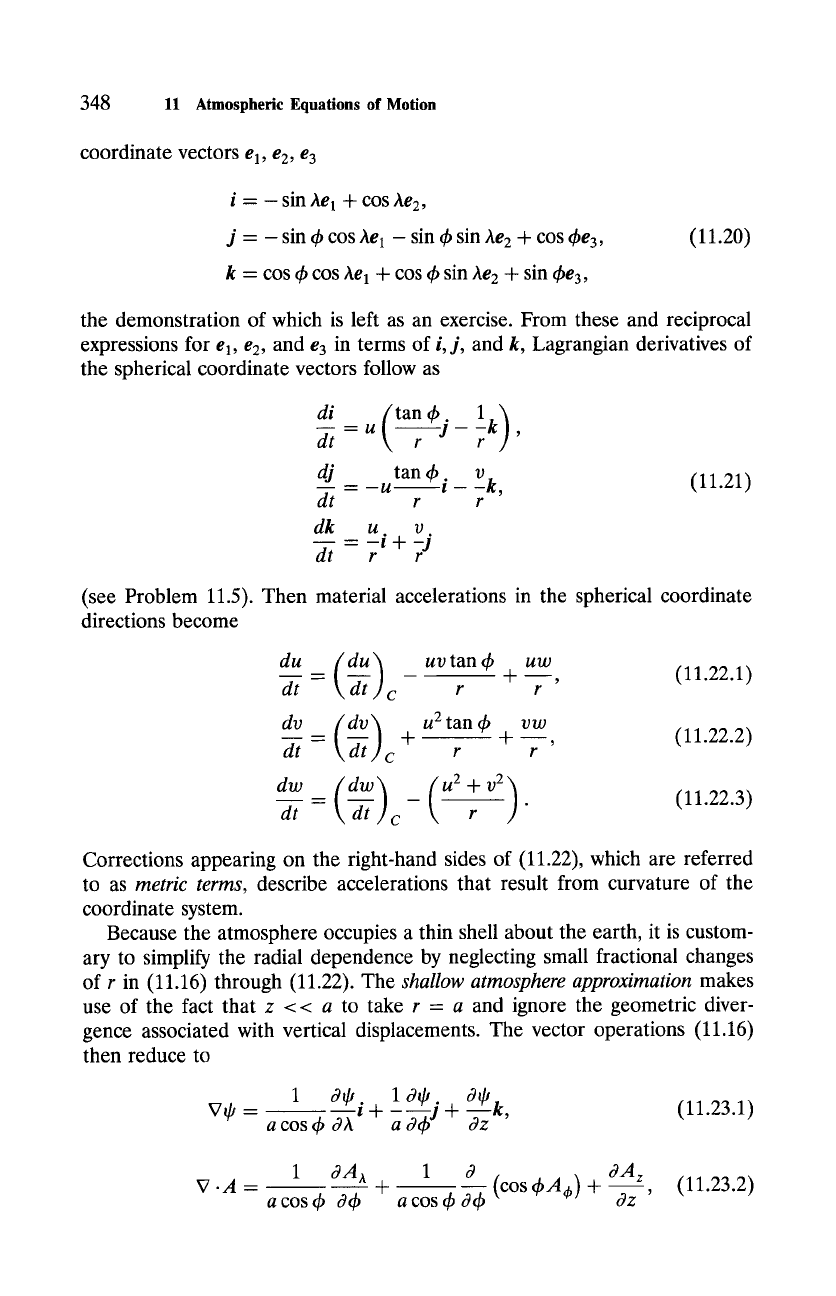
11 Atmospheric Equations of Motion
coordinate
vectors el, e2, e 3
i = - sin
he 1
+
COS Ae2,
j - - sin 4'
cos he 1
-
sin ~b
sin
he 2 + cos
t~e3,
k = cos ~b
cos he I + cos q~ sin he 2 + sin q~e3,
(11.20)
the demonstration of which is left as an exercise. From these and reciprocal
expressions for el, e2, and e 3 in terms of i, j, and k, Lagrangian derivatives of
the spherical coordinate vectors follow as
di
(ta;4~ !)
dt=U j- k
__ _ tan 4, v
dj _ -u i - -k,
dt r r
dk u v
= -i + -j
dt r r
(11.21)
(see Problem 11.5). Then material accelerations in the spherical coordinate
directions become
du (du)
uvtan ~b
uw
dt = -~ c--- r~- t ---~-,
(11.22.1)
dv (dv)
u2 tan 4~
vw
+ t r ' (11.22.2)
-~ d-t c r
dw (dw) _ (u2nt-v 2)
dt
= ~ c r " (11.22.3)
Corrections appearing on the right-hand sides of (11.22), which are referred
to as
metric terms,
describe accelerations that result from curvature of the
coordinate system.
Because the atmosphere occupies a thin shell about the earth, it is custom-
ary to simplify the radial dependence by neglecting small fractional changes
of r in (11.16) through (11.22). The
shallow atmosphere approximation
makes
use of the fact that z < < a to take r = a and ignore the geometric diver-
gence associated with vertical displacements. The vector operations (11.16)
then reduce to
1 OqJ 1 Oq~.
O0
VO= i+- + -~-' k, (11.23.1)
a cos
~ OA a -O-~J Oz
1 3A A 1 0 OA z
V .A = t (cos 4~A,/,) + -- (11.23.2)
a cos 4~ 04~ a cos 4~ ,34,
O z '
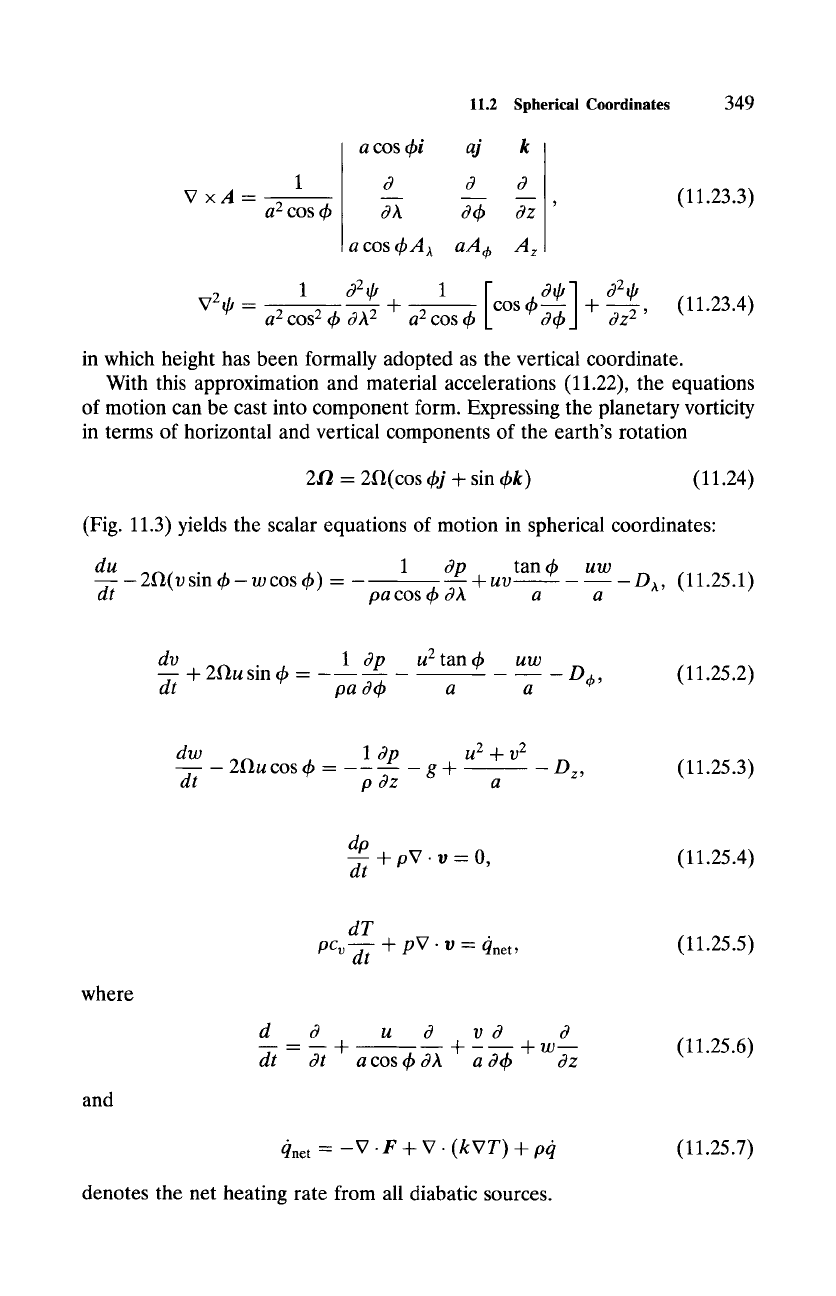
11.2
Spherical Coordinates
349
VxA=
a 2 cos 4)
a cos
chi aj k
3 3 3
3A o~4, ~z
acoschA
A aAr A~
(11.23.3)
1
~2 q,
~ cos 4' -Jr- --, (11 23.4)
V21/t -- a 2 cos 2 4) o~/~. 2 a 2 cos ~ ~ ~z 2 "
in which height has been formally adopted as the vertical coordinate.
With this approximation and material accelerations (11.22), the equations
of motion can be cast into component form. Expressing the planetary vorticity
in terms of horizontal and vertical components of the earth's rotation
292 = 2O(cos ~bj + sin ~bk)
(11.24)
(Fig. 11.3) yields the scalar equations of motion in spherical coordinates:
du 1 3p
tan 4,
uw
dt
211(vsin~b-wcos4~)- pacos~bdh
+-uv a a
D,, (11.25.1)
dv 1 Op
u2 tan ~b
uw
..... D4, ,
dt F- 2Ilu
sin 4, =
pa d4~ a a
(11.25.2)
dw 1 69p u 2 -~- 1) 2
211u cos
cb = g + - D z,
dt p 3z a
(11.25.3)
dp
dt
-- + pV. v = O,
(11.25.4)
dT
pc~
~ + p V. v
= qnet,
where
and
d 3 u d
dt=Ot I acosdpOA
vd 3
~--
+w--
a -ff-~
3z
qnet -- --V. F + V.
(kVT)
+ pq
denotes the net heating rate from all diabatic sources.
(11.25.5)
(11.25.6)
(11.25.7)
