Kelter P., Mosher M., Scott A. Chemistry. The Practical Science

Подождите немного. Документ загружается.

628 Chapter 15 Chemical Kinetics

0 50 100 150 200 250 300

Days

100

Percentage of original concentration

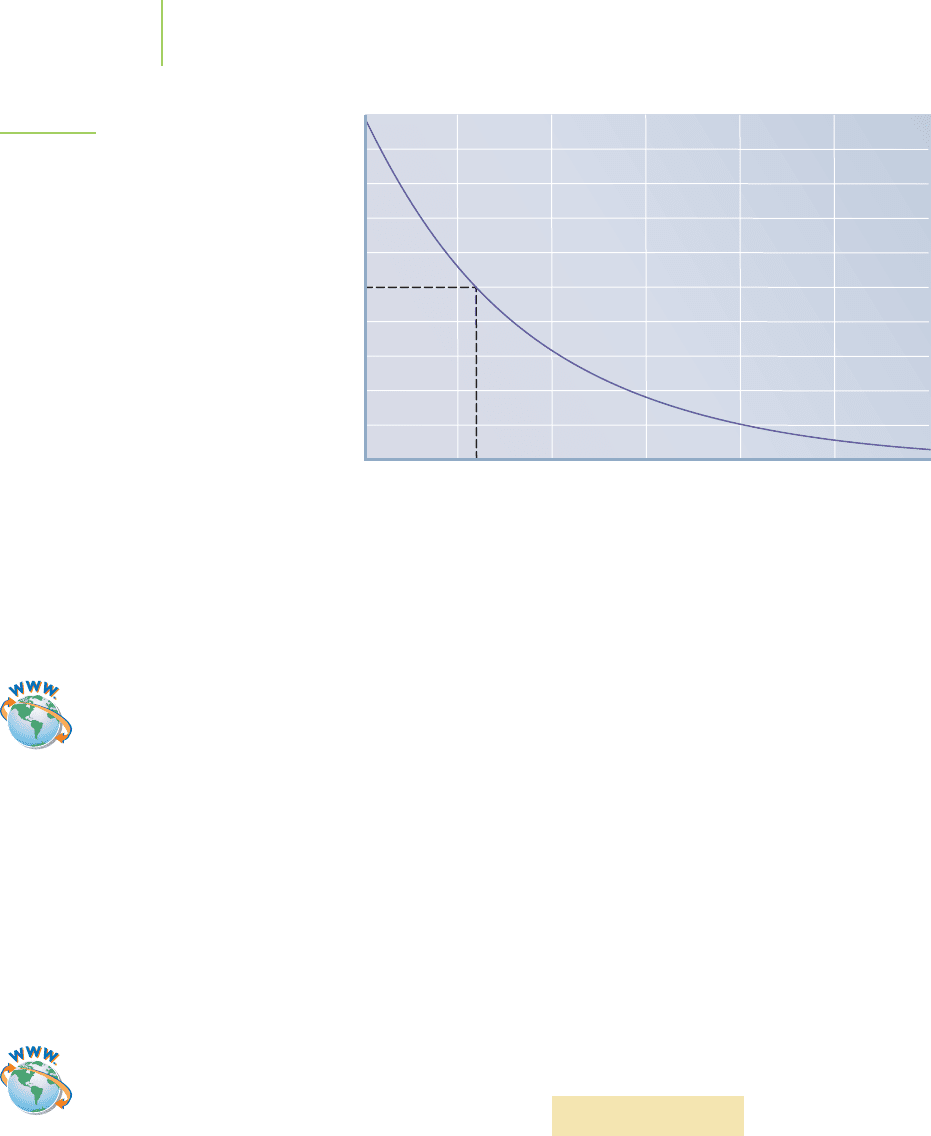
FIGURE 15.6

The environmental decomposition of

atrazine in groundwater. The half-life is

the time it takes for a given concentra-

tion to be halved.

because of its ability to oxidize microbes. We observe by experiment that the rate

of the reaction depends on the concentration of hydrogen peroxide. As the con-

centration decreases, the rate of the reaction decreases.

Rate ∝ [H

]

When we examine the relationship more closely, the following mathematical

equation, called a

rate law, emerges. This rate law states that the rate of the reac-

tion of hydrogen peroxide is equal to the product of a constant (which we call the

rate constant) times the concentration of H

(aq) n 2H

O(l) + O

(g)

Rate = k[H

]

From the rate law, we determine that the reaction is ﬁrst order in hydrogen

peroxide. That is, the rate depends on the concentration of H

raised to the ﬁrst

power. The reaction is ﬁrst order overall because the rate equation for the reaction

is dependent only on the concentration of H

to the ﬁrst power—that is, it is

linearly related. It is important to remember that the value of the rate constant,

the compounds included in the rate law, and the orders of the compounds in the

equation can be found only experimentally.

The rate law, as we will see, can be used to determine the rate of a reaction at

any reactant concentration. It will also tell you which species are the most impor-

tant contributors to the rate of a reaction. The typical rate law has the following

form:

Rate = k[A]

[B]

where k is the rate constant, [A] and [B] are the concentrations of substances

involved in the reaction, and n and m are the orders of the corresponding com-

pounds. The values of n and m are measures of how dependent the rate is on the

concentration of a particular reactant, and they must be experimentally deter-

mined. The reaction also can be described in terms of the overall order, which is

calculated by adding n and m (and the exponents of any other reactants in the

rate law). The order of a compound or the overall order of a reaction can cer-

tainly be negative or even a noninteger number. Again, it is important to remem-

ber that the order of a compound in a reaction cannot be determined just by looking

at the reaction.

The reaction of nitrogen monoxide gas with hydrogen gas is

2NO(g) + 2H

(g) n 2H

O(l) + N

(g)

Video Lesson: Rate Laws: How

the Reaction Rate Depends on

Concentration

Video Lesson: Determining the

Form of a Rate Law

The experimentally determined rate law is

Rate = k[NO]

]

The reaction is said to be second order in nitrogen monoxide, because the rate

depends on [NO] to the second power. The rate is ﬁrst order with respect to hy-

drogen gas, because the exponent is 1, which means there is a linear relationship

between the rate and [H

]. The reaction is third order (the sum of the individual

orders, 2

+ 1) overall. A reaction can have fractional orders, though we will not

deal with that here.

EXERCISE 15.3 The Rate Law

Environmental chemists are concerned with the damaging effects of compounds on

the ozone layer. One such class of compounds is the nitrogen oxides, such as NO(g)

and NO

(g). Under certain conditions, the reaction of NO(g), an air pollutant re-

leased in automobile exhaust, with oxygen can produce N

(g). The rate law for

this reaction was determined experimentally and is shown below. What is the reac-

tion order of each of the compounds in the rate law? What is the overall order of the

reaction?

Solution

The experimentally determined rate law says that the reaction is ﬁrst order in NO(g)

and ﬁrst order in O

(g). Overall, then, the reaction is second order.

PRACTICE 15.3

The following reaction proceeds at 300°C. What is the reaction order of the com-

pound in the following reaction with the given rate law? What is the order of the

reaction?

See Problems 19 and 20.

Collision Theory

In Exercise 15.3 and Practice 15.3, the rate law is expressed as the concentration

of the reactants raised to a power. This is not uncommon, but as we’ve mentioned

before, it isn’t true for reactions in general. Why do the orders of the rate law and

the stoichiometric factors of a reaction often differ? Reactions are typically more

complex than they appear when written on paper. To understand how this can

cause the rate law to differ from what we may expect, we need to consult

collision

theory

. This theory describes how the rate of a reaction is related to the number of

properly oriented collisions of the molecules involved. Collision theory is heavily

based on the kinetic molecular theory we discussed in Chapter 11.

Kinetic molecular theory says that the thermal motion of particles (the ki-

netic energy) can be used to explain how a gas behaves. For instance, the pressure

15.2 An Introduction to Rate Laws 629

(g)

Rate = k[NO][O

]

+2NO(g)N

(g)

Rate = k[HI]

+2HI(g)I

(g)

Visualization: Gas Phase

Reaction of NO and Cl

Video Lesson: The Collision

Model

630 Chapter 15 Chemical Kinetics

of a gaseous system is related to the number of collisions of the molecules with

the sides of their container in a given time. If we increase the number of mole-

cules per unit volume, the number of collisions per second also increases, assum-

ing the temperature is constant. The pressure of the system can be increased if we

raise the temperature of the gas and leave the volume constant. This is because

the molecules are moving faster (more kinetic energy) and engage in more colli-

sions per second. In short, higher kinetic energy equals more collisions.

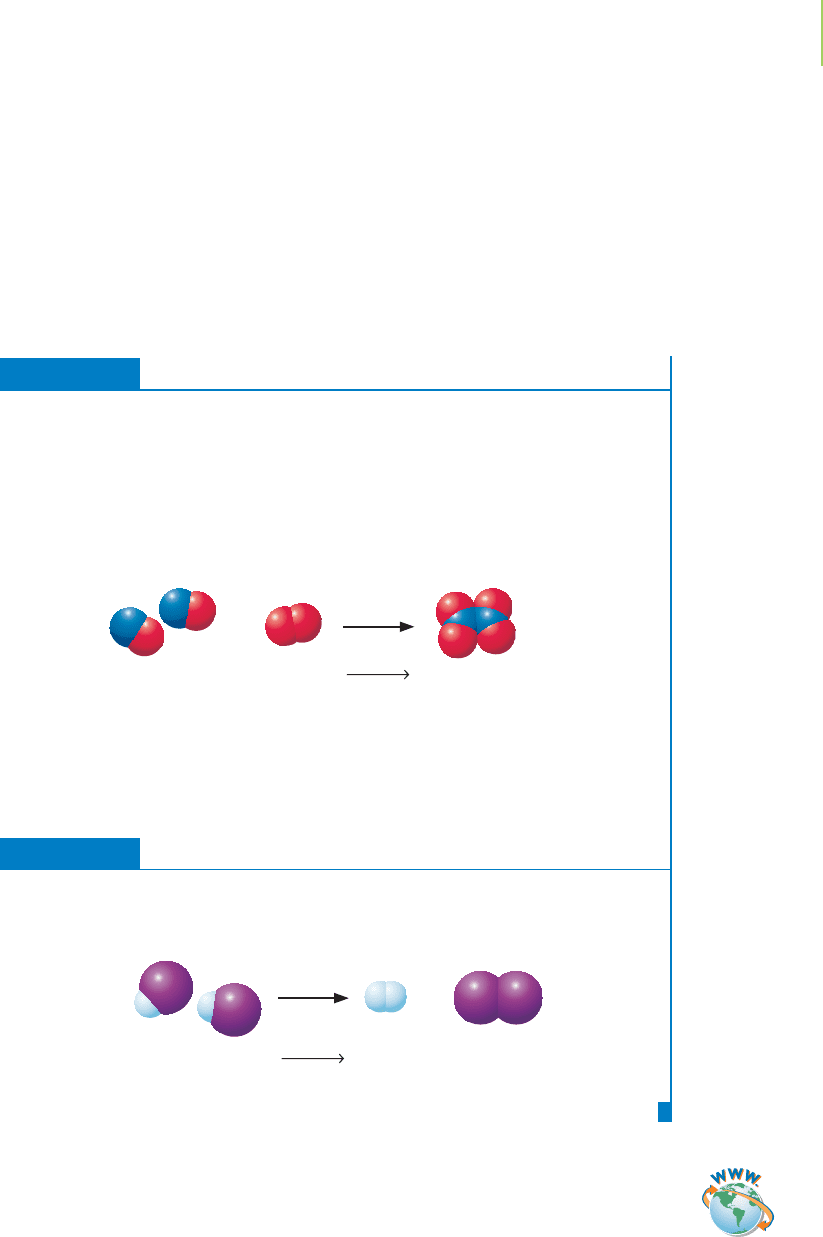
Collision theory, which is summarized in Table 15.1, requires that molecules

collide in order to react. One of the more important statements in collision the-

ory, as shown in Figure 15.7(a), says that the collisions

must be energetic enough to make a product. The

minimum energy required, the

activation energy (E

is speciﬁc to a particular reaction. However, an ener-

getic collision alone is not enough to cause a reaction

to occur. The collision must also occur between prop-

erly oriented molecules; see Figure 15.7(b). Because

the equation we write to describe a reaction doesn’t

address all of these issues, rate laws are difﬁcult to

derive by simply examining the overall equation.

We’ll revisit this statement in Section 15.6.

Cold = low T

Low kinetic energy

Hot = high T

High kinetic energy

Increasing the temperature of a reaction

increases the kinetic energy of the com-

ponents of the reaction. This means that

the particles move faster and, as a re-

sult, have many more collisions than

they do in the colder reaction. More col-

lisions mean a faster reaction.

(a)

(

)

Low KE

No reaction

Reaction

No reactionNot proper orientation

ReactionProper orientation

High KE

FIGURE 15.7

Collision theory. (a) Collisions must be

energetic enough to be considered suc-

cessful. (b) Successful collisions only

occur between properly oriented

molecules.

Collision Theory

A reaction occurs when the following conditions have been met:

• Molecules collide.

• Molecules have enough kinetic energy.

• Molecules are oriented properly.

Implications:

• Larger concentrations have faster reaction rates.

• Reactions with higher temperatures have faster rates.

• Rates depend on the number of properly oriented collisions.

• Predicting the rate of a reaction is difﬁcult.

TABLE 15.1

(a)

(b)

HERE’S WHAT WE KNOW SO FAR

■

The rate of a reaction is the change in concentration (M) per unit time. Rates

are always positive numbers, often reported in M/s.

■

Average rates and instantaneous rates differ only in the time measurement.

Instantaneous rates have ∆t ≈ 0.

■

The rate law (Rate = k[A]

[B]

) indicates the relationship between the rate

and the concentrations of reactants.

■

The order of a reactant can be used to indicate quickly the relationship be-

tween the reactant concentration and the rate.

■

Collision theory explains why the rate of a reaction typically decreases as time

passes.

15.3 Changes in Time—The Integrated Rate Law

After the discovery in 1939 that DDT (1,1,1-trichloro-2,2-bis-(4-chlorophenyl)

ethane, or dichlorodiphenyltrichloroethane) can be used to control mosquito-

borne malaria, its use soared. Especially important was its use to protect soldiers

who were ﬁghting in the Paciﬁc Rim countries in World War II. Since its discov-

ery, DDT has been sprayed to eliminate insects from cotton crops, spiders from

residences, and mosquitoes from towns all across the globe (Figure 15.8). Initial

testing showed that the compound wasn’t very toxic to mammals. However, be-

cause the metabolism of DDT is very slow, small amounts of DDT in the envi-

ronment tended to accumulate in animals (including humans) until toxic levels

were present. Evidence of this caused Sweden in 1970 and the United States in

1972 to ban the use of DDT as a pesticide, although it is still used in some other

countries, such as Ethiopia and South Africa. Despite the 30-year ban on DDT

use in the United States, the insecticide can still be found in the environment,

mostly in waterways like that shown in Figure 15.9. The hazard of DDT accumu-

lation in the food chain versus the beneﬁt of saving human lives by preventing

malaria in the populations of, for example, East African countries is still a topic of

intense debate.

In organisms that are resistant to DDT, an enzyme known as dehydrochlori-

nase converts DDT into dichlorodiphenyldiethene (DDE). Unfortunately, DDE

can accumulate within birds and weaken their eggshells by interfering with the

15.3 Changes in Time—The Integrated Rate Law 631

Application

HEMICAL

ENCOUNTERS:

Decomposition

of DDT

FIGURE 15.8

DDT and the mosquito. DDT is still one of the most cost-

effective methods of controlling mosquito-borne malaria.

Although many countries have banned its use because of

DDT’s persistence in the environment, many homes in

central Africa are still sprayed inside and out.

DDT

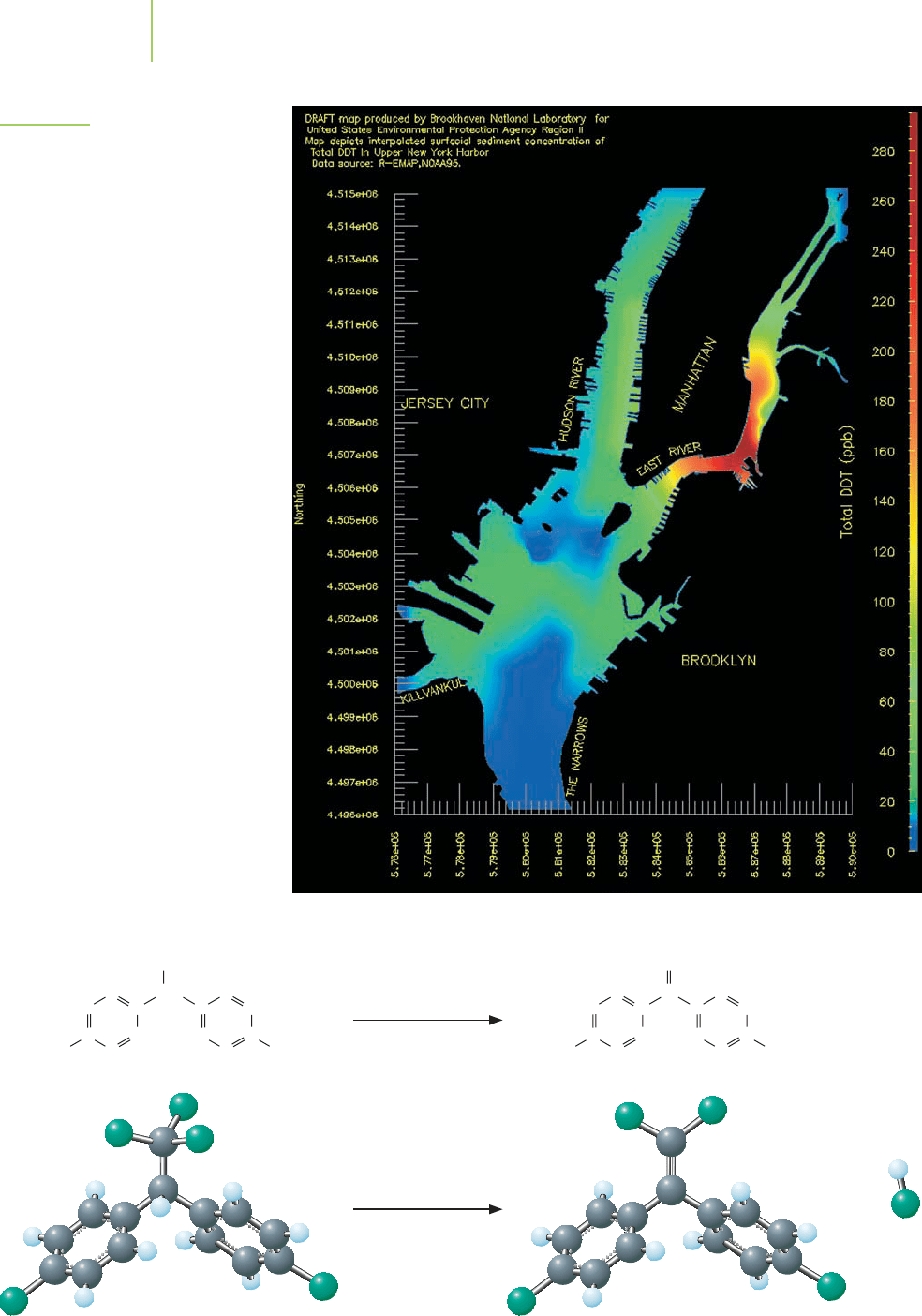
DDT DDE

CCH

CCl

CCl Cl

HC C

CHC

CCl

CCl Cl

HC C

CHC

HCl

DDT

Dehydrochlorinase

632 Chapter 15 Chemical Kinetics

FIGURE 15.9

Even though the use of DDT has been

banned in the United States since the

1970s, DDT is still present in the environ-

ment. This plot of New York harbor shows

that the sediments in the East River harbor

still contain large quantities of DDT.

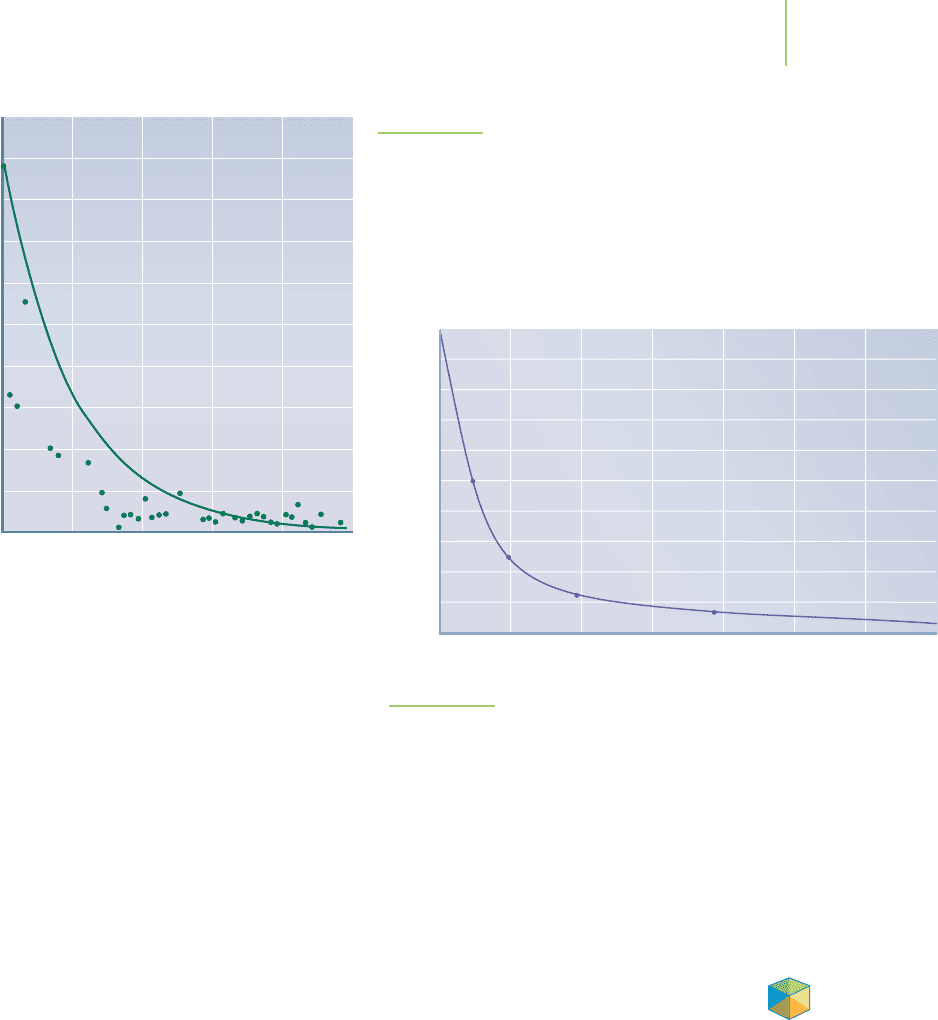
FIGURE 15.11

Plot of the radioactive decay of plutonium-239.

complex process of constructing the shells. This leads to shells that break under

the normal pressures naturally associated with nesting. For this reason, several

species of birds, such as the peregrine falcon, were nearly wiped out in the United

States.

The rate of decomposition of DDT by soil microbes is plotted against time in

Figure 15.10. Initially the rate is relatively high; then, as time passes, the rate be-

gins to decline.

Does this make sense according to what we know about collision

theory?

Yes. Reducing the number of reactant molecules reduces the number of

collisions that would result in the formation of the product.

What if we’re interested in determining what concentration of a chemical will

exist after a certain amount of time has passed? For example, the Hanford

Nuclear Reservation on the banks of the Columbia River just north of Richland,

Washington, has produced 54 million gallons of radioactive plutonium waste

over the past half-century. The waste is currently being stored in 177 under-

ground tanks as a watery sludge. How long will it take for the radioactive waste

stored at the Hanford Nuclear Reservation to decay to 1% of its current concen-

tration? We could examine the plot shown in Figure 15.11 to ﬁgure this out, but

we would need either to have a plot of the radioactive decay reaction or to know

the rate law, the rate, and rate constant of the reaction. Can we determine the

concentration at a speciﬁc time without knowing the rate or even having a plot of

the reaction? Calculus comes to our rescue. With some manipulation of the rate

law, we can make a more useful description of the rate of a reaction that will en-

able us to perform these calculations. These equations are referred to as the

inte-

grated rate laws

Integrated First-Order Rate Law

We discussed the decomposition of hydrogen peroxide to make oxygen and water

near the start of Section 15.2. Experimentally, it has been determined that the rate

15.3 Changes in Time—The Integrated Rate Law 633

0 102030 4050

Time (weeks)

Concentration (parts per million)

100

FIGURE 15.10

An experiment to illustrate the bioremediation (biological recycling) of DDT-

contaminated ground by soil microbes and nutrients. The decomposition of DDT

occurs rapidly at ﬁrst and then slows. Note that the concentration of DDT is

reported in parts per million (1 ppm of DDT = 1 µg of DDT per gram of soil).

Although the curve appears to be placed incorrectly, it is not. Computer analysis

of this set of data, and additional data not shown, produced this curve.

0 50,000 100,000 150,000 200,000 250,000 300,000 350,000

Ye a rs

100

Percentage of original concentration

Application

law for this process describes a ﬁrst-order reaction. Unfortunately, this doesn’t tell

us the concentration of H

at any point during the reaction, unless we know

the rate of the reaction at that time and the rate constant (k). In order to calculate

the concentration of H

at any point during the reaction, we can mathemati-

cally convert the rate law into an

integrated ﬁrst-order rate law.

(aq) n 2H

O(l) + O

(g)

Rate = k[H

]

The integrated rate law gets its name from the mathematical process, known as

integration, that we follow to generate the relationship between concentration and

time. When we integrate the rate law from t

= 0 to some future time, the inte-

grated rate law for the decomposition of hydrogen peroxide becomes



]



=−kt

where [H

]

is the concentration of hydrogen peroxide initially, [H

]

is the

concentration of hydrogen peroxide at a particular time t, and k is the rate con-

stant. In practice, we need know only three of the four values in the equation

(time, rate constant, initial concentration, and concentration at time t) in order

to ﬁnd the fourth.

This equation is general for all ﬁrst-order reactions, which we will designate

using the general form

A n Products

where A is any single reactant. Note that the stoichiometric coefﬁcient for A is 1.

The natural logarithm (ln) of the quotient of the ﬁnal reactant concentration,

[A]

, divided by the initial concentration [A]

is equal to the negative of the rate

constant, k, times the time, t, shown in the left-hand side of the box below. We can

use this equation to calculate the time required to reach a given concentration.

Alternatively, we can rearrange the equation to that shown on the right-hand side

of the box if we wish to calculate the concentration at a particular time:



[A]



=−kt

[A]

= [A]

−kt

We can transpose this into an equation in the form

y = mx + b

by recognizing

that



[A]



= ln[A]

− ln[A]

Substituting for



[A]



yields

ln[A]

− ln[A]

=−kt

which enables us to come up with the ﬁnal y = mx + b form:

ln[A]

= −kt + ln[A]

y = mx + b

where the y axis = ln[A]

the x axis = t

the slope

m =−k

the intercept b = ln[A]

How do we use the ﬁrst-order equation to ﬁnd the concentration of hydrogen

peroxide after 5.00 min if we have a solution in which the initial concentration,

634 Chapter 15 Chemical Kinetics

Video Lesson: First-Order

Reactions

]

, is equal to 0.100 M? The rate constant for this reaction was determined

by experiment to be 3.10

–3

–1

. Using this equation, we can calculate the

amount of peroxide still remaining after 5.00 min. We ﬁrst convert our time to

match the units for the rate constant (5.00 min

= 3.00

s) and then insert our

known values into the integrated ﬁrst-order rate law.

ln[H

]

− ln[H

]

=−kt

ln[H

]

− ln(0.100 M) =−(3.10 × 10

−3

−1

)(3.00 ×10

ln[H

]

− ln(0.100 M) =−0.930

ln[H

]

+ 2.3026 =−0.930

ln[H

]

=−3.2326

]

= e

−3.2326

= 0.0395 M

The concentration of hydrogen peroxide remaining after 5.00 min is 0.0395 M.

EXERCISE 15.4 Working with the Integrated First-Order Rate Law

Archaeologists near the Dead Sea in 1998 reported the discovery of a substance that

they believe ancient peoples used as glue. A sample of newly prepared collagen

exhibits 15.2 disintegrations per minute per gram (15.2 dis/min/g) of carbon. (A

disintegration is the decomposition of a radioactive nucleus such as

C; see Chap-

ter 21.) The

C decay rate for a sample of the ancient glue (made from collagen) was

found to be 5.60 disintegrations per minute per gram (5.60 dis/min/g) of carbon.

What is the age of the glue? The decomposition of

C is a ﬁrst-order process with a

rate constant of 1.209

–4

year

–1

First Thoughts

This problem shows that the integrated ﬁrst-order rate law can be used with ra-

dioactive compounds to determine decay rates, concentrations, or times. In these

cases, we consider the concentration of a reactant to be directly proportional to the

number of disintegrations per minute per gram. In the laboratory, this method can

be used to accurately determine the date of objects that are between 200 and 50,000

years old. Also note that we do not need to convert dis/min/g to dis/yr/g because the

units cancel in the equation.

Solution

If we assume that fresh collagen has the same activity of

C that the ancient glue did

when it was ﬁrst made, we can calculate the length of time it would take a fresh sam-

ple of collagen to have the same activity of

C as the glue.



5.60 dis/min/g

15.2 dis/min/g



=−(1.209 ×10

−4

year

−1

ln(0.3684) =−(1.209 × 10

−4

year

−1

−0.9985 =−(1.209 × 10

−4

year

−1

t = 8259 year = 8260 year

The calculation reveals that it would take 8260 years for the activity in the fresh

sample to decay to the level observed in the ancient piece of wood. This implies that

the glue is 8260 years old.

Further Insight

Radiocarbon dating has been used extensively in determining the age of archaeo-

logical artifacts. The process relies on the assumption that the ratio of carbon-14 to

carbon-12 in nature has always been constant. However, when an organism dies, the

ratio begins to change as the radioactive carbon-14 decays. Controversy over the

validity of this dating method has been addressed by compensating for small

15.3 Changes in Time—The Integrated Rate Law 635

Video Lesson: Radiochemical

Dating

Application

HEMICAL

ENCOUNTERS:

Persistent

Pesticides

ﬂuctuations in the original ratio. These ﬂuctuations have been determined by dat-

ing objects with an age known by other methods. More information about radio-

carbon dating can be found in Chapter 21.

PRACTICE 15.4

Hanford’s nuclear waste contains large quantities of plutonium (mostly

239

Pu).

Researchers have determined that approximately 875 kg of solid waste plutonium

are buried there. How long will it take for the mass of plutonium-239 to drop to

10% of its original value? Assume the rate constant that describes the decay of

239

is 2.874

−5

year

−1

. How long will it take for the mass of plutonium-239 to

reach half of its original concentration?

See Problems 33 and 34.

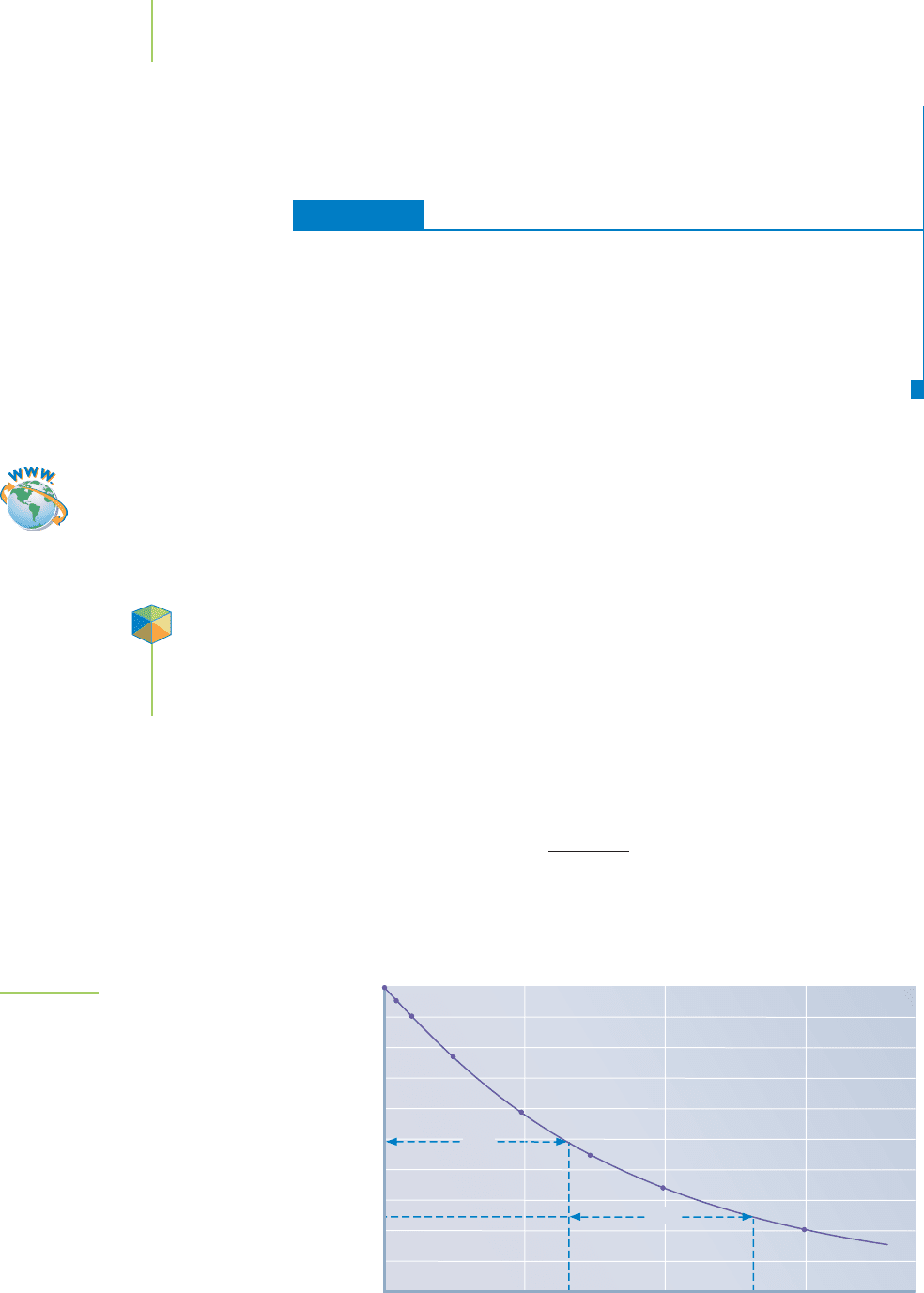
Half-life

The persistence of pesticides and herbicides in the environment is often reported

as the amount of time that it takes for half of the original concentration to de-

compose. In general, any reaction can be reported in this manner by calculating

the amount of time it takes for the reaction to proceed to 50% completion. This

value is known as the

half-life (t

1/2

) of the reaction (Figure 15.12). Perhaps you’ve

heard this term used to express the rate of decay for a radioactive element, such

as the radioactive waste stored at the Hanford Nuclear Reservation, or in ac-

counts of the dating of ancient artifacts. The half-lives of pesticides and herbi-

cides indicated in Table 15.2 are used to judge the safety of the compounds and to

establish guidelines for the frequency of their application. How does the half-life

ﬁt in with our description of the integrated ﬁrst-order rate law?

Consider the ﬁrst-order hydrolysis of atrazine in groundwater. The rate con-

stant for this reaction in water has been found to be 0.001733 day

−1

. Note that

we’re using a rate constant with units of day

−1

instead of s

−1

. This will be impor-

tant in the answer we generate from the integrated rate law equation.



[atrazine]



=−kt

636 Chapter 15 Chemical Kinetics

0 102030

Time (seconds)

0.025

0.050

0.100

Concentration (M)

1/2

FIGURE 15.12

The half-life of a reaction is the amount of time

required for the reaction to reach 50% of the

original concentrations. In terms of radioactive

decay or the decomposition of pesticides, the

passage of one half-life reduces the concen-

tration in half. Two half-lives reduce the

concentration to one-quarter of the original.

Visualization: Half-Life of

Reactions

Video Lesson: A Kinetics

Problem

Tutorial: Half-Life of Reactions

When the hydrolysis has consumed half of the original concentration of atrazine,

[atrazine]

⁄

2[atrazine]

= 0.5[atrazine]

. Substituting this into the equation

above, we ﬁnd that



0.5[atrazine]

[atrazine]



=−kt

1/2

The concentration of atrazine ([atrazine]

) cancels. Simplifying the equation fur-

ther yields

ln 0.5 =−kt

1/2

−0.693 =−kt

1/2

0.693

Our derivation reveals that the half-life (t

1/2

) of a ﬁrst-order reaction is,

throughout the reaction, a constant that depends only on the rate constant and not

on the concentration of the reactant. This means that if we know the half-life for a

particular ﬁrst-order reaction, we can calculate the rate constant, and vice versa.

Substituting the rate constant for atrazine into this equation, we ﬁnd that the

half-life for the hydrolysis in water agrees with the experimental observation we

noted at the start of this chapter. Half of the atrazine added to water in the envi-

ronment disappears after 400 days.

1/2

0.693

0.001733 day

−1

= 4.00 × 10

days

15.3 Changes in Time—The Integrated Rate Law 637

Solubility and Half-life in Soil for Selected Pesticides

The sorption index is the ratio of pesticide concentration bound to soil particles

divided by the concentration in the aqueous phase. Pesticides with low sorption

indices are more likely to be leached into groundwater supplies, because a low

proportion of each binds to the soil. The half-life is reported for the pesticide in

sterile soil. 2,4-D, for example, is of greater environmental concern because of its

sorption index value than is DDT.

Sorption Index

Trade Name/ Water Solubility (higher = greater Soil Half-life

Brand Name (ppm) binding to the soil) (days)

2,4-D 890 20 10

Alachlor/Lasso 240 170 15

Atrazine/Aatrex 33 100 60

Dicamba/Banvel 400,000 2 14

Carbaryl/Sevin 110 300 10

Chlorsulfuron/Glean 7000 40 160

DDT 0.0055 24,000 3000

Diazinon 60 1000 40

Malathion/Cythion 145 1800 1

Metolachlor/Dual 530 200 90

Methoxychlor/Marlate 0.10 80,000 120

Pendimethalin/Prowl <1 5000 90

Pronamide/Kerb 15 200 60

Terbacil/Sinbar 710 55 120

Terbufos/Counter 4.5 500 5

Triﬂuralin/Treﬂan <1 8000 60

Source: Institute of Agriculture and Natural Resources, Univ. Nebraska Lincoln, Factors That Affect Soil-

Applied Herbicides, 2005.

TABLE 15.2