Kelter P., Mosher M., Scott A. Chemistry. The Practical Science

Подождите немного. Документ загружается.

classify as “insoluble” in water, our universal solvent, can dissolve at least a little.

One such chemical is oxygen (O

), and that is fortunate for ﬁsh and other sea life

that depend on it. Another such chemical is carbon tetrachloride, a nonpolar in-

dustrial solvent; that is not so fortunate, because even in low concentrations in

water, it is considered an environmental hazard.

11.7 Measures of Solution Concentration

We have said that seawater is largely an aqueous solution of dissolved sodium

chloride. However, there are many other components of this solution, including

a good deal of Mg

,SO

2–

, and Ca

; smaller quantities of iron, phosphorus,

and copper; and really small amounts of dissolved oxygen, cadmium, and even

gold. What do we mean by “a good deal,” “smaller quantities,” and “really small

amounts”? It depends on whom you ask. And “it depends” is too vague in a sci-

entiﬁc community that requires clarity when communicating the results of mea-

surements. We need descriptions of concentration that have consistent meaning

to everyone reading the data.

Measures Based on Moles

Molarity (M)

We ﬁrst examined molarity in Chapter 4 as a measure of moles of solute per liter

of solution.

M =

mol solute

L solution

Molarity is a standard concentration unit in the chemical laboratory. We can

speak of the initial molarity of a solute added to a solution, as in “What is the ini-

tial molarity of the sodium chloride?” We can also discuss the actual molarity of

each ion after the solute has dissolved, as in “What is the molarity of the sodium

ion in the solution?”

Molality (m)

Molality is a measure of moles of solute per kilogram of solvent.

m =

mol solute

kg solvent

The molality of a solute in solution is independent of temperature because it is

based on measuring the mass of the solvent, rather than the volume of the solution.

It is useful in exploring properties at a variety of temperatures, as we shall discuss

in Section 11.8. And because we can measure mass accurately and precisely,

molality can be determined to many signiﬁcant ﬁgures, if necessary.

Mole Fraction (χ

)

The mole fraction of a substance is the ratio of the number of moles of a substance

present per total moles of all substances in the solution. If there are three solutes,

i, j, and k, in aqueous solution, then the mole fraction of solute i is

mol i

mol i +mol j + mol k +mol water

It is important, when we calculate the mole fraction, to include the contribu-

tion of all components in the system. Note that the denominator in the equation

indicates that we add the number of moles of i, j, k, and water to obtain the total

number of moles in the system.

468 Chapter 11 The Chemistry of Water and the Nature of Liquids

Video Lesson: Molality

Video Lesson: Molarity and the

Mole Function

EXERCISE 11.8 Practice with Mole-Based Units of Concentration

Fructose is one of the three important “simple sugars,” the other two being galactose

and glucose. What are the values for molarity, molality, and mole fraction of 36.0 g

of fructose (C

) in 250.0 mL of a fruit-ﬂavored drink? The density of the

water-based drink is

1.05 g/mL, and you may neglect the presence of ﬂavorings.

Solution

The addition of fructose changes the density of the solution compared to pure

water, so we would expect the molarity (which is based on the solution volume) and

molality (based on the solvent mass) to be different. When we calculate the mole

fraction, we must take into account both the moles of fructose and the moles of the

solvent, water. All three measures of concentration require us to know the number

of moles of fructose (C

mol fructose = 36.0 g fructose

1 mol fructose

180.0gfructose

= 0.200 mol fructose

Molarity of fructose =

mol fructose

L solution

0.200 mol fructose

0.2500 L solution

= 0.800 M

The molality calculation requires that we know the mass of water. We can ﬁnd this

via the mass of the solution and its density.

Mass of solution = 250.0 mL solution

1.05 g solution

mL solution

= 262.5 g solution

(Note that we keep the extra ﬁgure for this calculation and round only the number

that will be our ﬁnal answer.)

Mass of water = mass of solution − mass of fructose = 262.5 g − 36.0 g

= 226.5 g water

Molality

mol fructose

kg solvent

0.200 mol fructose

0.2265 kg water

= 0.883 M

We can covert the mass of water to moles for our mole fraction calculation.

mol water = 226.5 g water

1 mol water

18.0 g water

= 12.58 mol water

Mole fraction of fructose =

fructose

mol fructose

mol fructose + mol water

0.200

0.200 +12.58

= 0.0156

Fructose

11.7 Measures of Solution Concentration 469

PRACTICE 11.8

What mass of potassium hydroxide (KOH) is required to prepare 600.0 mL of a

1.40 M KOH solution? What is the mole fraction of water in this solution? (Assume

that the density of the solution is 1.07 g/mL.)

See Problems 67, 70, 71, and 73.

Measures Based on Mass

We discussed the two useful sets of mass-based concentration measures, weight

percent and

parts per million, parts per billion and parts per trillion, in Section 4.2.

We present them again here for purposes of review and for application in the

context of our discussion of seawater.

Weight Percent (wt %)

Weight percent is a measure of the mass fraction of a substance in a solution,

expressed as a percentage.

wt % =

g substance

g solution

× 100%

If you were to prepare a reference solution that has the same weight percent

of sodium chloride as seawater, it would contain 29.5 g of sodium chloride per

1.00 × 10

g of solution, a weight percent of 2.95% NaCl.

29.5gNaCl

1.00 ×10

g solution

100% = 2.95% NaCl

Other related units are weight-to-volume and volume-to-volume.

Parts per Million, Billion, and Trillion (ppm, ppb, ppt)

In discussing the concentration of possible health hazards in water, the Environ-

mental Protection Agency (EPA) cites its

maximum contaminant level (MCL) the

highest acceptable level in a solution, as 10 parts per million for nitrate and

5 parts per billion for cadmium. Very low solute concentrations

(sometimes called trace concentrations) are often expressed this

way. The density of very dilute aqueous solutions is close enough to

that of pure water, 1.0 g/mL, that we may use these volume-based

conversions that we derived in Section 4.2.

ppm =

1 g solute

g solution

≈

1 mg solute

L solution

ppb =

1 g solute

g solution

≈

1 g solute

L solution

ppt =

1 g solute

g solution

≈

1 ng solute

L solution

The average concentration, in parts per million, of the major ions

that are present in seawater are listed in Table 11.4.

EXERCISE 11.9 Conversion Between Mole and Mass Concentration Units

The maximum concentration of O

in seawater is 2.2 ×10

−4

M at 25

◦

C. What is this

concentration in parts per million of oxygen?

470 Chapter 11 The Chemistry of Water and the Nature of Liquids

Application

HEMICAL ENCOUNTERS:

Composition of Seawater

Application

Average Composition

of Major Ions in Seawater

Element Parts per Million

(main form in seawater) (mg/L)

Chlorine (Cl

−

) 19,000

Sodium (Na

) 10,500

Magnesium (Mg

) 1,250

Sulfur (SO

2−

) 900

Calcium (Ca

) 400

Potassium (K

) 380

Bromine (Br

−

)65

Bicarbonate (HCO

−

)30

Strontium (Sr

)12

Source: HPS Certiﬁed Reference Materials.

TABLE 11.4

11.8 The Effect of Temperature and Pressure on Solubility 471

Application

HEMICAL ENCOUNTERS:

Impact of the

Solubility of Oxygen

in Fresh Water

Solubility of O

in Fresh Water at

Several Temperatures (in air at 1 atm)

Temperature (°C) Parts per Million (mg/L)

0 14.6

5 13.1

10 11.3

15 10.1

20 9.1

25 8.3

30 7.6

35 6.9

Solution

We can solve this via dimensional analysis, as follows.

2.2 ×10

−4

mol O

L seawater

32 g O

mol O

1000 mg O

1gO

= 7.0 ppm O

Does the answer make sense? We expect the solubility of (nonpolar) oxygen to be

quite low in water, and our answer conﬁrms that.

PRACTICE 11.9

The MCL for arsenic in drinking water is 10 ppb, according to Environmental

Protection Agency guidelines. Convert this value to molarity.

See Problems 74–76, 79, 80, and 111.

11.8 The Effect of Temperature

and Pressure on Solubility

Temperature Effects

We saw in Exercise 11.9 that oxygen is nearly insoluble in seawater, yet 7.0 ppm

at 25

◦

C is still enough to allow the seas to teem with life. The concentration of

oxygen in the seas and in freshwater lakes, ponds, and rivers varies as natural

processes such as photosynthesis and respiration cycle oxygen into and out of the

water. The other important factor that determines oxygen’s solubility in water

(see Table 11.5) is temperature. Note the trend, followed by all gases, that solubil-

ity of a gas decreases with temperature. Figure 11.28 shows this behavior for several

common gases.

Small changes in temperature do not dramatically affect the

solubility of oxygen, but a large increase in temperature can sig-

niﬁcantly lower the oxygen concentration in a waterway, and

the harm done can be felt throughout the aquatic food chain.

The artiﬁcial raising of the ambient water temperature is called

thermal pollution and is of concern in the design of nuclear

power plants, in which river water is used to cool the nuclear

core of the reactor (see Chapter 21). The heated river water is

passed through a

cooling tower (Figure 11.29, on page 472)

before it ﬂows back to the river.

Temperature (°C)

Solubility (mg gas/100 g H

4.00

3.50

3.00

2.50

2.00

1.50

1.00

0.50

010203040506070

Total air

80 90 10

FIGURE 11.28

The solubility of gases decreases with temperature.

This is especially important with O

, where “ther-

mal pollution” can have important consequences

for the aquatic food chain.

TABLE 11.5

Video Lesson: Temperature

Change and Solubility

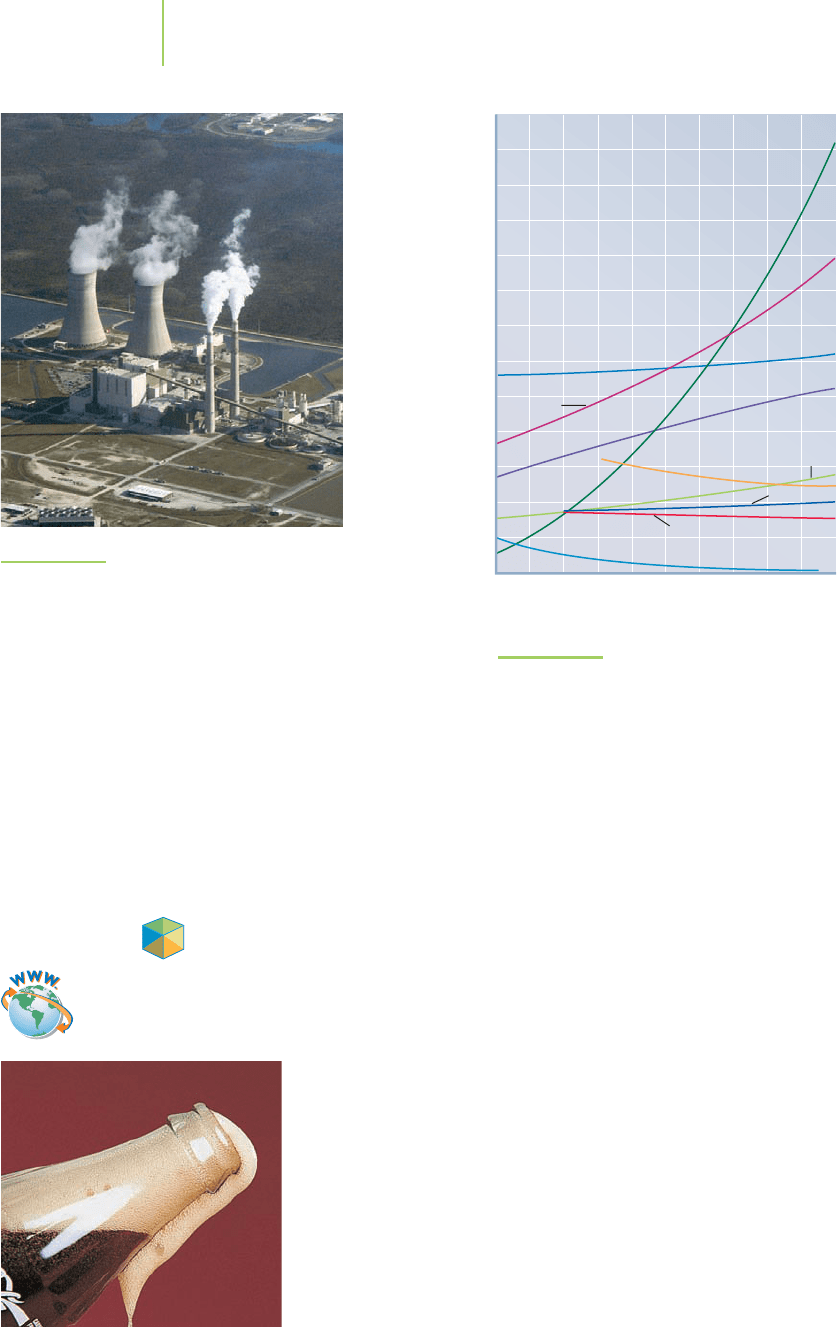
FIGURE 11.30

The solubility of some salts in water as a function of

temperature. Note that the solubility of the majority of

these salts increases as the temperature increases. Li

decreases solubility as the temperature increases.

In contrast to gases, the solubility of ionic solids in water generally increases

with temperature, as shown in Figure 11.30. For example, the solubility of sodium

chloride in water at 0

◦

C is 35.7 g/100 mL, and at 100

◦

C it is 39.1 g/100 mL. Some

solids show a much more marked increase in solubility. Sodium carbonate

decahydrate (Na

· 10H

O) has an aqueous solubility of 22 g/mL at 0

◦

Cto

420 g/mL at 100

◦

C, a 22-fold increase. A few salts, such as manganese(II) sulfate

hexahydrate (MnSO

· 6H

O) and sodium sulfate (Na

), have lower solubility

with increasing temperature.

Pressure Effects

The solubilities of solid solutes in water are not especially responsive to modest

pressure changes. The solubility of gases in water, on the other hand, is quite sen-

sitive to the external pressure. This is important in the preparation of soft drinks,

in which CO

is combined with water, sweetener, and other ﬂavorings at pres-

sures between about 6 and 15 atm. The “ﬁzz” in the soda is due to the dissolved

. When the can is opened, the CO

above the soda escapes from the container,

and the pressure of the can suddenly drops to atmospheric pressure. The lower

pressure decreases the solubility of the CO

in the soda, and bubbles of CO

form.

William Henry (1775–1836), an English chemist, noted this behavior.

Henry’s

law

says that at constant temperature, the solubility of a gas is directly propor-

tional to the pressure that the gas exerts above the solution.

gas

= k

gas

where P

gas

= the pressure of the gas above the solution

gas

= the concentration of the gas in the solution

gas

= a constant relating the gas pressure above the solution and its

concentration

472 Chapter 11 The Chemistry of Water and the Nature of Liquids

FIGURE 11.29

A cooling tower lowers the temperature of water

that has been heated as part of the operation of

power plants. This water is close to the tempera-

ture of the waterway from which it was taken

and to which it will be returned, so the impact

of thermal pollution is minimized.

Temperature (°C)

Solubility (g solute/100 g H

140

160

180

200

220

240

260

120

100

201003040506070

80 90

100

KNO

NaNO

NaBr

KBr

KCl

NaCl

(SO

)

Application

Carbon dioxide is released from a

glass of freshly opened soda.

Video Lesson: Pressure Change

and Solubility

In pure water at 1 atm and 0

◦

C, the solubility of CO

is 3.48 g/L. According

to Henry’s law, if we triple the CO

pressure, the solubility will roughly triple.

Overall, this law holds best for gases such as N

and O

that do not signiﬁcantly

interact with the solvent. It does not hold well for HCl, which ionizes in water to

form the hydrated ions H

(aq) and Cl

−

(aq). However, as we have noted, even

molecules such as O

interact with water to some degree.

EXERCISE 11.10 Henry’s Law and CO

in Soda

In air in which the pressure of CO

is 3.4 × 10

−

atm at 0

◦

C, the solubility of CO

in water is

1.18 × 10

−3

g/L water. If the pressure of the CO

above the water

is increased to 6.00 atm, what will be the solubility of the CO

in the water? Do

these data lead to a conclusion consistent with our prior assertion that sodas go

“whoosh” when they are opened?

Solution

The CO

pressure is being increased by a huge factor, so we would expect the solu-

bility to increase about proportionately. Assuming proportionality, we can elimi-

nate the need to calculate the Henry’s law constant and just solve the problem with

ratios.

1.18 ×10

−3

g/L

3.4 ×10

−4

atm

x g/L

6.00 atm

x = 21 g/L

The solubility of the gas increased sharply at high external CO

pressure, in accor-

dance with Henry’s law. As is consistent with much of our discussion on solutions,

the formulas we cite work best for very dilute solutions. When the can of soda is

opened, the pressure of CO

above the liquid sharply decreases, lowering the solu-

bility of the gas and therefore contributing to the escape of gas that we hear when

we open the soda.

PRACTICE 11.10

A researcher adds 22.7 g of NaCl to 55.0 mL of water. Examine Figure 11.30. Will all

of the salt dissolve in the given amount of water at 25

◦

C? If not, how much water

will need to be added to just dissolve the salt completely?

See Problems 83 and 84.

11.9 Colligative Properties

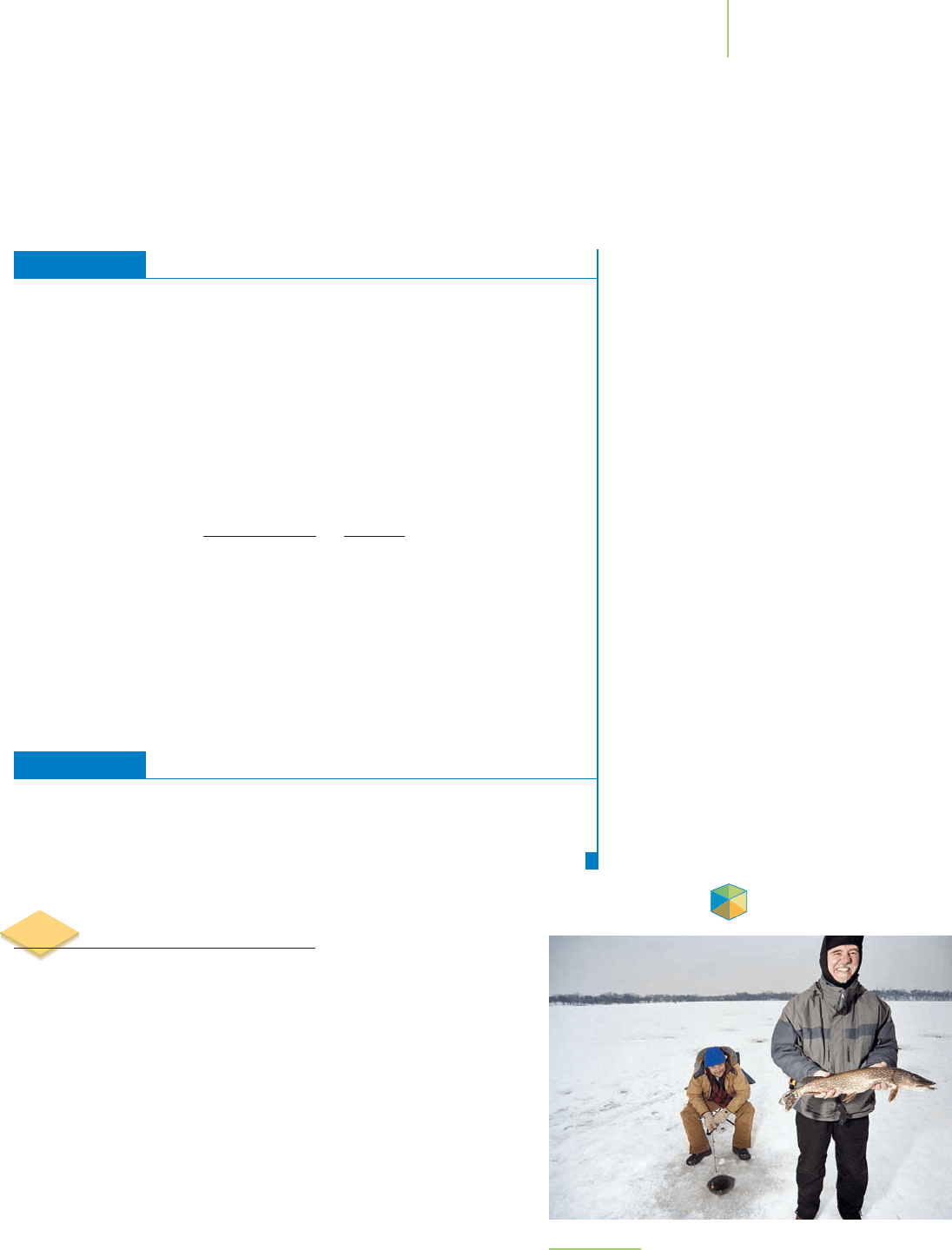
In the dead of winter at Lake Riley in Minnesota, it is common to see

people brave the harsh winter weather (Figure 11.31) to sit around

meter-deep ﬁshing holes in the ice. The ice ﬁshing season here typically

runs from late January through mid-March. We take it for granted, but

ice ﬁshing is possible only because the water in the freshwater lake has

a relatively low concentration of dissolved solutes and has a freezing

point just below 0

◦

C. If Lake Riley were as salty as the Red Sea or the

Persian Gulf, with sodium and chloride ion concentrations equal to 40

parts per thousand, its freezing point would be about −2.5

◦

C, and the

ice ﬁshing season would be shorter (if not nonexistent), as anglers

waited for sufﬁcient ice to form to make the lake safe for the ﬁshing

shacks and vehicles that transport them. Why does the presence of the

salt lower the freezing point of water? Does the amount of salt affect

the freezing point, and does the nature of the salt matter? Are there any

other solution properties that are affected in this way?

11.9 Colligative Properties 473

Application

FIGURE 11.31

Ice ﬁshing on Lake Riley in Minnesota can be rewarding.

Let’s answer the last question ﬁrst. Properties of a solution that approximately

depend only on the number of nonvolatile solute particles, irrespective of their

nature, are called

colligative properties (from the Latin colligatus, which means

“collected together”). There are four useful colligative properties: vapor pressure

lowering, freezing-point depression, boiling-point elevation, and osmotic pres-

sure. Understanding vapor pressure lowering will help us answer our questions

about dissolving salts in water, as well as give us insight into the other three

colligative properties.

Vapor Pressure Lowering

1,2,3-Propanetriol (C

) is the systematic name for the nonvolatile substance

we commonly call glycerol or glycerin. The colorless, viscous liquid is used as a

lubricant and moistener, especially in cosmetics, and to reduce swelling in med-

ical procedures, such as eye examinations. The presence of three OH groups on

the molecule leads to signiﬁcant hydrogen bonding, making glycerol completely

soluble in water. We noted in Section 11.3 that water has a vapor pressure equal

to 23.8 torr at 25

◦

C. (Judging on the basis of our discussion in that section about

intermolecular forces, structure, and vapor pressure,

does it make sense that

water should be volatile, whereas glycerol is nonvolatile?

) Glycerol has essentially

no vapor pressure at room temperature. When glycerol and water are mixed, the

total vapor pressure of the resulting solution is dependent only on the vapor pres-

sure of pure water,

multiplied by its mole fraction,

, in the solution.

Vapor pressure of the solution =

solution

= χ

For example, if we add enough glycerol to water so that the mole fraction of the

water is reduced to 0.900, the resulting vapor pressure of the solution will be re-

duced. At 25

◦

C, the vapor pressure of the solution would be

solution

= χ

solution

= 0.900 ×23.8torr= 21.4torr

The relationship of the vapor pressure of the solution, P

solution

, to the mole

fraction, χ

solvent

, and vapor pressure, P°

solvent

, of the volatile solvent holds true for

any ideal solution containing a nonvolatile solute. It is known as

Raoult’s law,

named after the French chemist Francois-Marie Raoult (1830–1901).

solution

= χ

solvent

An ideal solution exists when the properties of the solute and solvent are not

changed by dilution. This means that other than being diluted, combining solute

and solvent in an ideal solution does not release or absorb heat, and the total vol-

ume in the solution is the sum of the volumes of the solute

and solvent. Only very dilute solutions approach ideal

behavior, so although Raoult’s law is a good ﬁrst approxi-

mation, actual measurements are required to properly

describe vapor pressure changes in mixtures of solutions.

Figure 11.32 shows the general trend: The vapor pressure is

depressed with the addition of a nonvolatile solute.

474 Chapter 11 The Chemistry of Water and the Nature of Liquids

FIGURE 11.32

The vapor pressure of water (red line) is lowered by the

addition of a nonvolatile solute. This is described for an

ideal solute by Raoult’s law.

1 atm

Pressure (atm)

∆T

Freezing

point of

solution

Freezing point

of solvent

Boiling point

of solvent

Boiling point

of solution

Temperature (°C)

Vapor pressure

of pure solvent

Vapor pressure

of solution

Glycerol

Visualization: Vapor Pressure

Lowering: Liquid/Vapor

Equilibrium

Visualization: Vapor Pressure

Lowering: Addition of a Solute

Visualization: Vapor Pressure

Lowering: Solution/Vapor

Equilibrium

Video Lesson: Vapor Pressure

Lowering

EXERCISE 11.11 Vapor Pressure Lowering

You stir 3 teaspoons (45.0 g) of sucrose, table sugar (C

, molar mass =

342 g/mol), into a cup of tea containing 250.0 mL of water at 90.0

◦

C (density =

0.965 g/mL). What is the new vapor pressure of the solution?

526 torr at

90.0

◦

First Thoughts

The key problem-solving hurdle is calculating the mole fraction of water in the

solution. To do this, we must calculate the number of moles of each component. We

will retain an extra, nonsigniﬁcant ﬁgure until the end of the calculations.

Solution

mol sucrose = 45.0 g

1 mol

342 g

= 0.1316 mol sucrose

g water = 250.0 mL

0.965 g water

1 mL water

1 mol water

18.02 g water

= 13.39 mol water

water

mol water

mol glucose + mol water

13.39

0.1316 +13.39

= 0.9903

solution

= χ

water

solution

= 0.9903 ×526 torr = 520.9torr≈ 5.2 × 10

torr

Further Insights

As expected, the vapor pressure of the solvent decreases as a consequence of the ad-

dition of the sucrose. Note the use of the symbol ≈, which means “is approximately

equal to.” Raoult’s law is strictly followed only for ideal solutions. This solution has

enough sucrose in it that it does not approach ideal behavior.

PRACTICE 11.11

The vapor pressure of ethanol (C

OH) at 40

◦

C is 135.3 torr. Calculate the vapor

pressure of a solution containing 26.8 g of glycerin (C

) a nonvolatile solute,

in 127.9 g of ethanol.

See Problems 87, 88, 93, 95, and 96.

Boiling-Point Elevation

At 1 atm, the temperature of any solution must be elevated until its vapor pres-

sure equals 760 torr in order to reach the boiling point. Concentrated solutions

possess lowered vapor pressures (which we discussed earlier), because the solu-

tion must have a vapor pressure equal to the atmospheric pressure in order to

boil. The more solute is dissolved in a solution, the more the vapor pressure of the

solution is lowered, and the higher the boiling point. The temperature of the so-

lution must be elevated for the vapor pressure to reach that of the surroundings.

For fairly dilute solutions of nonelectrolytes, the following formula approximately

describes the

boiling-point elevation due to the addition of a nonvolatile solute.

T

= K

where T

= the change in boiling point in

◦

= the boiling-point elevation constant, which depends on the solvent,

in units of °C/m

m = the molality of the solute in moles of solute per kilogram of solvent

11.9 Colligative Properties 475

Visualization: Boiling-Point

Elevation: Liquid/Vapor

Equilibrium

Visualization: Boiling-Point

Elevation: Addition of a Solute

Visualization: Boiling-Point

Elevation: Solution/Vapor

Equilibrium

Video Lesson: Boiling-Point

Elevation and Freezing-Point

Depression

The value of K

for water is 0.512

◦

C/m. This means that a

2.00 molal aqueous sugar solution would have a boiling-point

elevation of approximately 1.02

◦

C (2.00 m × 0.512

◦

C/m) and a

boiling point of about 101

◦

C. We say “about” because the

boiling-point elevation constant begins to deviate signiﬁcantly

from the ideal solution value at higher concentrations.

Boiling-point elevation constants for several liquids are listed in

Table 11.6.

Colligative properties depend on the number, not the nature,

of the particles in the solution. We would expect solutions con-

taining compounds at the same concentration to have the same

elevated boiling point. However,we must consider the total num-

ber of particles that result from making the solution. For example, what would

happen to the boiling point of our aqueous sucrose solution if our solute were 2.00

molal cobalt(II) chloride (CoCl

) instead of sucrose? Because cobalt(II) chloride

is a strong electrolyte that dissociates to form cobalt ion and chloride ion,

CoCl

(s)

−−−−−→

(aq) + 2Cl

−

(aq)

we would expect three moles of particles (ions, in this case) for every mole of

CoCl

added to the solution. That is, the concentration of ions in the solution

should be 6.00 molal, and the boiling point of the solution should be raised by

(6.00 m × 0.512

◦

C/m) = 3.07

◦

C. This suggests that when dealing with strong

electrolytes, we can modify our boiling-point elevation formula to take into

account the dissociation of strong electrolytes into i particles.

T

= iK

For CoCl

, i =3 if the solution behaves ideally. The actual boiling-point elevation

for this solution is 4.6

◦

C, not 3.1

◦

C, which tells us that at this relatively high con-

centration (2.00 m), the solution does not behave even close to ideally. The value

i is known as the

van’t Hoff factor, after J. R. van’t Hoff (1852–1911), a chemist

from the Netherlands who suggested its use in the 1880s. Table 11.7 shows the

van’t Hoff factors for several electrolytes. Note that there is signiﬁcant deviation

from the expected values as the solute concentration increases, so results are only

approximate even at relatively low concentrations.

EXERCISE 11.12 Boiling-Point Elevation

Recipes for cooking spaghetti often call for putting a little table salt in the water

before boiling it and adding the spaghetti. Does this help the spaghetti cook faster?

Assume that we add 10.0 g of NaCl to 6.00 L of water and that the density of the

solution is 1.00 g/mL. Also assume that this very dilute solution behaves ideally, so

i = 2. The value of K

for water is 0.512

◦

C/m.

Solution

mol NaCl = 10.0 g NaCl

1 mol NaCl

58.5gNaCl

= 0.171 mol NaCl

kg water = 6.00 L water

1.00 kg water

L water

= 6.00 kg water

Molality of NaCl =

mol NaCl

kg water

0.171 mol NaCl

6.00 kg water

= 0.0285 m NaCl

T

= iK

= 2.0 ×



0.512

◦



× 0.0285 m

T

= 0.03

◦

476 Chapter 11 The Chemistry of Water and the Nature of Liquids

Boiling-Point Elevation Constants

for Several Liquids

Solvent K

(

◦

C/m) T

(

◦

Acetone 1.7 56.5

Benzene 2.6 80.1

Carbon tetrachloride 5.0 76.7

Ethanol (ethyl alcohol) 1.2 78.5

Methanol (methyl alcohol) 0.80 64.7

Water 0.51 100.0

TABLE 11.6

Jacobus Henricus van’t Hoff

(1852–1911) was a Dutch chemist who

initially shook the basic ideas of chem-

istry with his description of the three-

dimensional nature of molecules. In

1901 he won the ﬁrst Nobel Prize in

chemistry for his work on solutions.

Video Lesson: Boiling-Point

Elevation Problem

This shows that there is nearly no elevation in the boiling point of water with the

addition of a little table salt. The salt is added for taste.

PRACTICE 11.12

What is the predicted boiling point of each of these solutions? (Assume that each

follows ideal behavior.)

a. 1.00 m NaCl in water

b. 0.35 m FeCl

in water

c. 1.50 m KCl in methanol

See Problems 89, 90, and 97.

In contrast to the minimal impact of adding a dash of table salt to water when

cooking spaghetti, making an aqueous solution that is 40% by volume ethylene

glycol (C

) elevates the boiling point to 105°C (221

◦

F). This solution, called

“antifreeze,” helps protect your automobile engine in hot weather.

11.9 Colligative Properties 477

van’t Hoff Factors for Several Electrolytes

Expected

Compound Value of im = 0.005 m = 0.01 m = 0.05 m = 0.10 m = 0.20 m = 1.00 m = 2.00

HCl 2 1.95 1.94 1.90 1.89 1.90 2.12 2.38

Cl 2 1.95 1.92 1.88 1.85 1.82 1.79 1.80

CuSO

2 1.54 1.45 1.22 1.12 1.03 0.93 —

CoCl

3 2.80 2.75 2.64 2.62 2.66 3.40 4.58

3 2.77 2.70 2.45 2.32 2.17 — —

TABLE 11.7

Ethylene glycol is a major

component of many

antifreeze solutions.

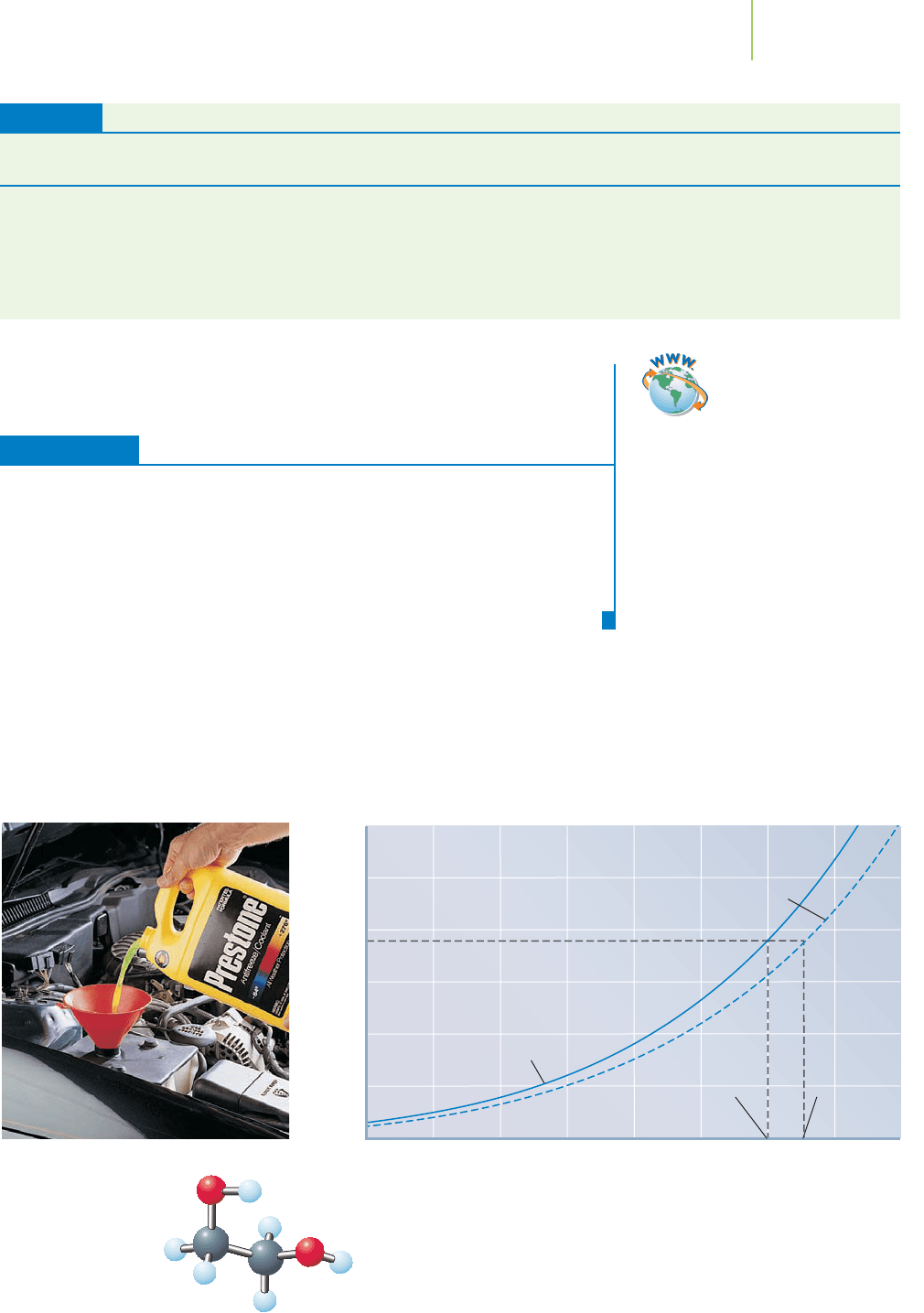
40 50 60 70 80 90 100 110 120

200

400

600

800

1000

1200

Temperature (˚C)

760 torr

Pure water

Vapor pressure (torr)

Boiling point

of water

Boiling point

of 10 m

ethylene glycol

10 m ethylene glycol

The boiling point of a 40% by volume solution of ethylene glycol is 105°C.

Video Lesson: Colligative

Properties of Ionic Solutions