Bemelmans J. et. al. (editor) Elliptic and Parabolic Problems: Rolduc and Gaeta 2001
Подождите немного. Документ загружается.


250
and (3.1) follows
in
this case too. By Lemma 3.1 we deduce that condition (Co) holds.
(ii) Without loss of generality we can assume that
x
0
=
0, hence 5
0
(x)
=
\x\. Put
w =
\PM
and
wi =
w(l
- *) on
B
r
(0).
Assume first that
p ^ N.
Since
w
G W
o
1,p
(iJ
r
(0)) we get
from Hardy inequality (see [7]),
/ \Vw\" > |1
-
N/p\"
f
(\w\/\x\)",
(3.4)
JB
T
(0) JB
T
(0)
so that
w/\x\ e
U(B
r
{Q)). Since clearly w
x
/\x\
€
U(B
r
{Q))
it
follows that
u/\x\ £
LP(B
r
{Q)).
But we
have also
Vu €
V{B
r
{Q)). We deduce thus
as
above that (3.1)
holds,
hence condition (Co)
is
satisfied.
In
case
p
=
N
we argue as above, but with (3.4)
replaced
by
the following improved Hardy inequality due
to
Adimurthi, Chaudhuri and
Ramaswamy [1, Theorem 1.1],
/ \Vw\'>C
[
(\w\/\x\r(\ogR/\x\)-",
(3.5)
JB
r
(0) JB
r
(0)
V(0)
JB
r
(0)
with
R
>
re
2/p
.
It
follows then that,
u
e L"(B
r
(0)).
(3.6)
\x\\og(R/\x\)
In order
to
show that condition (C
0
) is satisfied we take
a = 1/2
and set,
rf
=
(l/2)"
+
'~\
j =
l,...,n,
for
n >
— j
5
^.
Since we always assume that
p > 1
we have
p = N > 2. By
Young
inequality,
(^)
2
|V
U
r<(2/
P
)^
+
(
l-2/p)|V<.
Therefore,
i E
/
(M/M)'+
i
E
/
iv«r
2
(i«i/ix|)
2
<
^/ (H/lxD'
+ c/
|Vur:=/{"
)
+
^"
)
.
W
V{2-
2
"<|:c|<2-»} ^{2-
2
"<|x|<2-»}
Since Vu
e
I^(B
r
(0))
it
is clear that liirin-Kx,/|
n)
=
0. Further,
/j
n)
<
— (lo
gj
R
+
2nlog2)
p
f (. ,,
*"[,.,
,.V -> 0 (by (3.6)).
It follows that condition (Co)
is
satisfied.
•
Remark 3.1.
A
comparison lemma related
to (ii) of
Proposition
3.1
was proved
by
Sreenadh [10].

251
Next
we
prove
a
uniqueness result
for
global solutions.
Theorem
3.1. Let a(x) €
L]
0C
(R
N
\
{0})
and
suppose that
u
G
C(R
N
\
{0})
n
WJ£(R
N
\
{0})
is a
positive solution
of
the equation
(1.3) inQ.
—
R
N
\
{0} which satisfies condition
(3.2),
and
for
some
a €
(0,1),
lim inf
r
-
>0
'{°r<\x\<r)
,
3?
.
f (\u\/\x\)"
< oo, if p€
{1,2),
f (M/My
+
lVur^M/liD^oo, tf
P
e(2,oo),
J
{<TT<\x\<r}
lim inf
r->0
or more generally, assume
u
satisfies conditions
(Cx,) and (Co)
with
T
0
= {0}. Let
v
e C(R
N
\
{0})
n
WJ£(R
N
\
{0}) be another positive solution
to
(1.3). Then,
v = cu for
some constant
c > 0.
(3.8)
Proof.
Consider
any x
r
G R
N
\ {0} and set a :=
vixj/uixi).
Put Qi = R
N
\
{0,X!}
and apply Theorem
1.3 for n = Qi and Ti = {x^} to
deduce that i)(a;)/M(a;)
> «i =
D(:TI)/U(:EI)
for
every
x ^ 0.
Since
x
t
and x are
arbitrary,
we
deduce that
u/v = c. •
Remark
3.2.
Theorem
3.1
generalizes Theorem
1.3 of
[8]
which treated
the
special case
a(x)
= |1
—
N/p\
p
\x\~
p
in
which
a
positive solution
to (1.3) is
given explicitly
by u(x) =
x
l-N/p
We close with
a
uniqueness result
in
bounded domains.
Theorem
3.2. Let O be a
bounded domain with boundary
T
0
. Let a(x) e
L}
0C
(Q)
and
suppose that
u e C(O) n W^(Q) is a
positive solution
of (1.3)
which satisfies (3.1),
or
more generally, condition
(Co). Let v € C(Q)
PI
W^(Q) be
another positive solution
to
(1.3).
Then,
v = cu for
some constant
c > 0.
Proof.
Simply repeat
the
argument
of
Theorem
3.1,
this time applying Theorem
1.3 to
the domain
£2
\
{ii}, whose boundary
is the
disjoint union
of
To
= dQ and r
x
= {^i},
for
any X\ €
Q.
•
Remark
3.3. Let Q be a
bounded domain
of
class
C
2
and let
r\
G
C(Q)
satisfy r\
> 0 in
CI
and
rj
= 0 on dQ. In
[6, Theroem 1.2]
it
was shown that
for a
certain critical value
A*,
there exists
a
positive solution
u
t
to the
equation,
-A
p
u
t
=
(y
V
+
(l-l/pr)
l
-^p±,
(3.9)
which satisfies
the
growth condition,
C-
1
6
1
-
1
'"<u
t
<C8
1
-
1/
'',inn,
for
some
C > 0,
(3.10)
where
we
denoted
5(x) =
dist(x,dil).
It is
easy
to
show that (3.10) implies
(3.1)
(with
8
0
= 8).
Indeed,
in
case
p £ (1,2]
this
is
immediate, while
for p > 2 we
need only
to remark that
by [9,
Lemma
A.3],
(3.10) implies that
|Vw,| <
C8~
l
^
v
in
Q,,
and the
conclusion follows
as
well. Therefore
we
infer from Theorem
3.2
that
u
t
is
actually
the
unique positive solution
to
(3.9),
up to a
multiplicative factor.

252
References
[1] Adimurthi, N. Chaudhuri and M. Ramaswamy, An improved Hardy-Sobolev inequality
and its application, Proc. Amer. Math. Soc. 130 (2002), 489-505.
[2] S. Agmon, Bounds on exponential decay of eigenfunctions, in "Schrodinger Opera-
tors",
ed. S. Gram, Lecture Notes in Math., Vol. 1159, Springer-Verlag, Berlin, 1985,
1-38.
[3] W. Allegretto and Y. X. Huang, A Picone's identity for the p—Laplacian and appli-
cations, Nonlinear Analysis TMA 32 (1998), 819-830.
[4] H. Brezis and M. Marcus, Hardy's inequality revisited, Ann. Sc. Norm. Pisa. 25
(1997),
217-237.
[5] M. Marcus, V. J. Mizel and Y. Pinchover, On the best constant for Hardy's inequality
in
R™,
Trans. A.M.S. 350 (1998), 3237-3255.
[6] M. Marcus and I. Shafrir, An eigenvalue problem related to Hardy's IP inequality,
Ann. Sc. Norm. Pisa. 29 (2000), 581-604.
[7] B. Opic, A. Kufner, "Hardy-type Inequalities", Pitman Research Notes in Math.,
Vol. 219, Longman 1990.
[8] A. Poliakovsky and I. Shafrir, Uniqueness of positive solution for singular equations
involving the p-Laplacian, preprint.
[9] I. Shafrir, Asymptotic behavior of minimizing sequences for Hardy's inequality, Com-
mun. Contemp. Math., 2 (2000), 151-189.
[10] K. Sreenadh, On the Fucik spectrum of
Hardy-Sobolev
operator, to appear in Nonlin-
ear Analysis TMA..

Supercritical variational problems with unique critical
points
Wolfgang Reichel
Mathematisches Institut der Universitat Basel, Rheinsprung 21
CH-4051 Basel, Switzerland
Email : reichel@math.unibas.ch
Abstract
We study how a simple class of one-parameter transformation-groups inter-
acts with functionals
jC[u]
=
j
n
L(x,u,
Vu) dx. We show how one can understand
Pohozaev's identity as a formula for the rate-of-change of £ under the action of the
transformation group. Uniqueness of the critical point of L will follow provided the
transformation group reduces the values of
C.
As an application we find a class of
conformally contractible domains, which includes the class of star-shaped domains,
and on which Pohozaev's uniqueness result remains valid.
1 Transformation groups
We begin our study with one-parameter transformation groups. Let Q C R
n
be a bounded
smooth domain with exterior unit normal v{x) for x 6 dil. We consider a one-parameter
family of maps G = {g
e
: dom<?
£
C fl x 1 -1 (1x1} enjoying the group-property
5ei °
&2
=
&1+E2!
ffo
= W. A particularly nice example of such a group arises when g
e
is
defined as the flow-map at time e of the dynamical system
x = £(x,u), u =
(f>(x,u),
(1)
with smooth functions £,
(/>.
To be precise, let (xe(x
0
,u
0
),ip
e
(xo,uo)) denote the solution
of (1) at time e with initial conditions (x
0
,uo). Then a one-parameter group G arises by
setting g
e
(x
0
,u
0
) = (x
£
(a:o,«o)^e(^o, Wj), for every (x
0
,u
0
) eSlxR, In a forthcoming
publication [5], we investigate transformation groups arising from the dynamical system
(1),
together with their interactions with variational problems.
In this paper, we study a simple type of transformation group arising from the following
dynamical system:
x = £(x), u = a(x)u. (2)
The system (2) is a special case of (1) and can be integrated semi-explicitly. Since
the two equations in (2) can be decoupled, we denote by Xc{
x
) the solution of the first
equation at time e, starting with the initial condition x at time e = 0. Then ip
e
(x,u) =
253

254
exp(/
0
£
a(x
T
(x))d,T)u solves the second equation, with the initial condition u at time e = 0.
Let us now take an initial function u(x). We can insert its graph (x,u(x)) into the
flow and obtain after time e the transformed graph (x
e
{x),i>
l
{x,u{x)). For sufficiently
small e, this will represent the graph of a new function (x,u(x)) with new independent
variable x =
Xe(
x
)-
Expressing x =
X-e{
x
)
we find the following explicit formula for the
transformed function
u(x)
expf / a(xr-
e
{x))dTJ u(x-
€
(x))
exp f / a(xr(x))dTJ u(x-
€
(x)),
(3)
where x belongs to Q
e
= Xe(0). We will write g
e
u for the new function u(x). In the
following, we will use summation convention, i.e., double indices will automatically be
summed from 1 to n.
Proposition 1.1 Let G = {<?
e
}e>o be a transformation group generated by (2). Then for
every i = 1,..., n and every x € O
e
, the following holds
am
. «,(/ *,,,»*)gfe^gw
f° da _ dx
j
( f° \ -
+
J dx"
(
'
XT
^~dx
Z
^
dTeXP
[j
a
(X
T
W>
dT
)
u
(X-e{i))-
Proof.
(4) follows directly from (3). D
In order to state the following Proposition, let us introduce the space CQ{D) of con-
tinuously differentiable functions on D with zero boundary values and the space C
0
' (D)
of uniformly Lipschitz-continuous functions on D with zero boundary values.
Proposition 1.2 Suppose £(x)
•
v(x) < 0 for all x € 80.. Then for
e
> 0 we have fi
e
C fi.
Extending u by zero outside fi, the transformed function g
e
u is zero in Q \ Q
e
. Moreover,
if the initial function u belongs to the space Cg(Q) or C
0
' (fi) then for small e > 0 we
have g
e
u €
CQ(Q
€
),C
0
'
(f2
e
), respectively. In both cases, we have g
e
u e C
0
' (fl) for small
e>0.
A transformation half-group {g
€
}
e
>o arising from a flow of type (2) and with the
property £(x)
•
v(x) < 0 on 80. will be called a contracting transformation half-group.
2 Rate of change of a functional under a transforma-
tion group
We consider a functional
C[u]
= J
Q
L(x, u, Vujdx, where L
:
Q x K"
+1
—»
R is a continu-
ously differentiable Lagrangian. Let us suppose w.l.o.g that L(x,0,0) = 0, for all x 6 fl-
it is simple to see that £ is Frechet-differentiable on the space
CQ'
1
(Q).
255
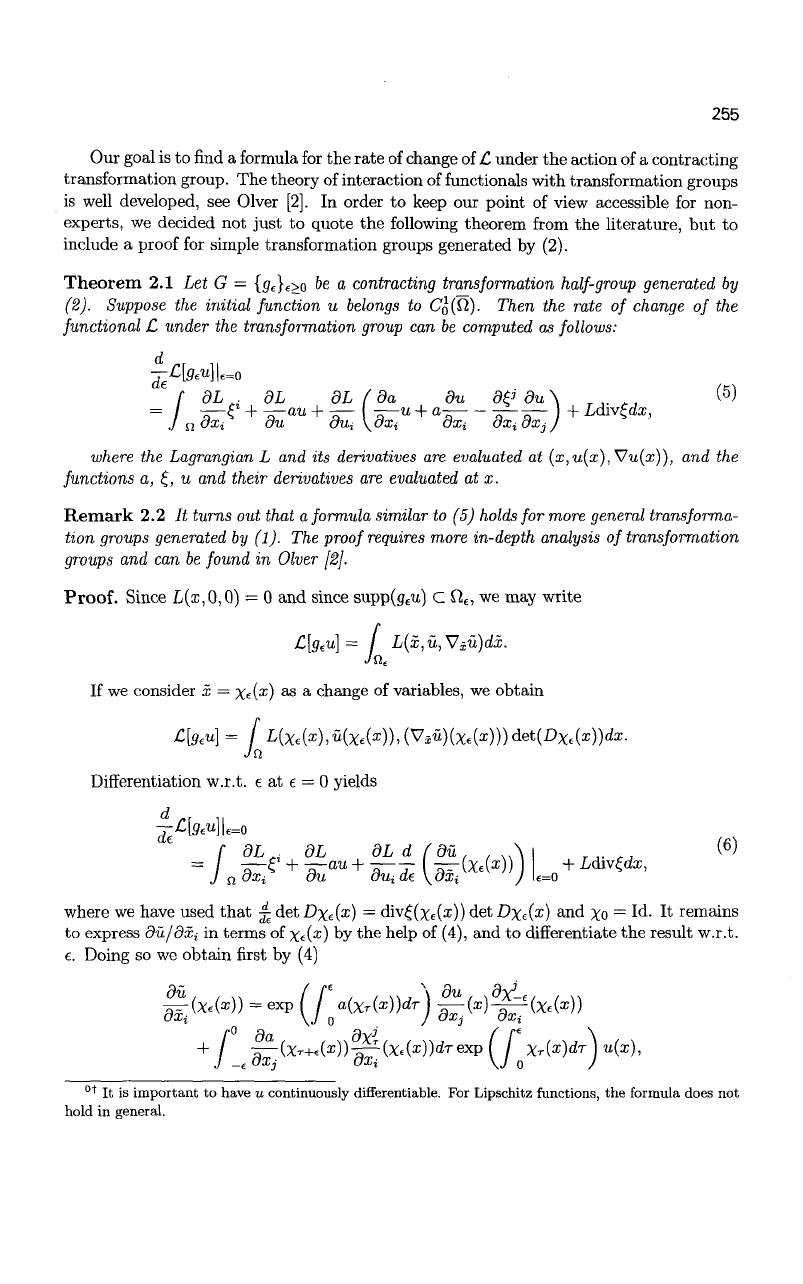
Our goal is to find a formula for the rate of change of C under the action of a contracting
transformation group. The theory of interaction of functionals with transformation groups
is well developed, see Olver [2]. In order to keep our point of view accessible for non-
experts, we decided not just to quote the following theorem from the literature, but to
include a proof for simple transformation groups generated by (2).
Theorem 2.1 Let G =
{g
e
}e>o
be a contracting transformation half-group generated by
(2).
Suppose the initial function u belongs to
CQ(0,).
Then the rate of change of the
functional £ under the transformation group can be computed as follows:
—C[gM\^o
f dL
_.
dh dL (da du d& du\
r
,. ,, (
5
)
= J
a
^
+
Tu^
+
^ fe
M +
fl
&T ft^&J
+ M
^
dX
'
where the Lagrangian L and its derivatives are evaluated at (i,u(i),Vti(i)), and the
functions a, £, u and their derivatives are evaluated at x.
Remark 2.2 It turns out that
a
formula similar to (5)
holds
for more general transforma-
tion groups generated by (1). The proof
requires
more in-depth analysis of transformation
groups and can
be
found in Olver [2].
Proof.
Since L(x, 0,0) = 0 and since supp(g
e
M) c O
e
, we may write
C[g
e
u]
= / L(x,u,Vxu)dx.
If we consider x = Xe(
x
) as
a
change of variables, we obtain
C[g
€
u]
= f L(x
e
(x),u(x,(x)),(V
4
u)(x,(i)))det(£>
Xe
(s))dx.
Jet
Differentiation w.r.t. e at e = 0 yields
j
e
£[geu}\
€=0
f 8L
ri
dL dL d (du , . ,,\ i
T
,.
AJ
(6)
where we have used that ^ det Dx
e
{x) = div£(x
e
(x)) det Dxe(x) and xo = Id. It remains
to express du/dxi in terms of Xe{
x
) by the help of (4), and to differentiate the result w.r.t.
e. Doing so we obtain first by (4)
J|(X.(*))
= exp
(/W))*) -g(*)^(Xe(*))
f° da dx
J
f f
e
\
+
/
faTMT+e{x))-Q^;{Xt{x))dTexp
( /
Xr(x)dr
\ u{x),
°i It is important to have u continuously differentiable. For Lipschitz functions, the formula does not
hold in general.

256
and after differentiation w.r.t. e at e = 0 and using \o = Id, dxb/dxi = 6
t
j we get
A
t)
(JJL
{z)
+
pf
{x
^fjM\ W*
w
„
w
.
(7)
ctey \ axi dxidxi de
IE=O/
cte*
The last relation simplifies since d
2
xb/dxidxi = 0. The result follows by inserting (7)
back into (6). •
Corollary 2.3 Let G = {g
e
}
c
>o be a contracting transformation half-group generated by
(2).
Suppose that the initial function u
belongs
to C
2
(fi)nCQ(f2). Then the rate of change
of the functional under the transformation group satisfies
±C
M
U = J
a
(au - *
•
Vu)£
[u]
dx
+
J J
.U)(L-
£ J£) da, (8)
where
£[u]
= ""^(ffO + f^ i
s
the Euler-operator associated to the functional C. As before,
the Lagrangian L and its derivatives are evaluated at (x, u(x), Vu(i)), the functions a, u,
£ and their derivatives at x.
Proof.
The proof hinges on a formula of E. Noether, which she used in the derivation
of her famous theorem on symmetry and conservation laws, cf. Olver [2], Section 4.4.
Noether's formula expresses the integrand in (5) as a multiple of the Euler-equation plus
a divergence-term, i.e.
dL <9L dL_(da_ ^_^_^_\
T
A-C
dxi du dui \dxi dx
t
dxidxjj
= {au - £
•
Vu)£[u] + 4~ (?L +{au-£- Vu)f^
aXi \ oUi
(9)
Formula (9) is verified by explicit calculation. If we recall that u = 0on
dQ.
and hence
Vu = vdu/dv, an integration by parts results in (8) and completes the
proof.
Remark 2.4 For solutions of the Euler-equation £[u] = 0, the identity between the vol-
ume integral (5) in Theorem 2.1 and the surface integral (8) in Corollary 2.3 is known
as Pohozaev's identity, cf. Pohozaev [3] and Pucci and Serrin [4]. It has long been
noted,
cf. Pucci, Serrin [4] and Van der Vorst
[7],
that some kind of connection between
one-parameter transformation groups, Noether's formula and Pohozaev's identity exists.
For yet another variational
approach
for deriving Pohozaev's identity, which is especially
useful for free-boundary problems, see Wagner [8].
3 Variational sub-symmetries and uniqueness of crit-
ical points
We can now begin to formulate our main result. Loosely speaking it states under mild as-
sumptions on the Lagrangian that a contracting transformation half-group, which reduces
the values of L, implies the uniqueness of the critical point of C.

257
Definition 3.1 Let the functional £[u] = J
n
L(x,u,Vu)dx be defined on C%
A
(Q). A
contracting transformation half-group G
~_{g
€
}
€Z0
generated by (2) is called a variational
sub-symmetry for £ if for all u €
C^
l
(Q)
there exists e
0
(u) > 0 such that £[g
e
u] < £[u]
for all e E (0, e
0
(u)).
Proposition 3.2 Let G = {g
e
}
€
>o be a contracting transformation half-group generated
by (2). Then the condition
~C[g
£
u}\e=o < 0, for all u E C^U), (10)
is necessary and sufficient for G to be a variational sub-symmetry. If (10) holds with
strict inequality for all u E Cg(fi) unless u = 0 then G is called a strict variational
sub-symmetry w.r.t 0.
The importance of the proposition lies in the fact that one can use the explicit formula
for ^£[g
e
u]\
e=0
given in (5) to verify that G is a variational sub-symmetry. We are now
ready to state our main uniqueness result, which follows directly from Corollary 2.3.
Theorem 3.3 Suppose G is a variational sub-symmetry generated by (2) for the func-
tional £[u] =
f
fri
L(x,u,
Vu)dx on the
space
CQ'
1
(ft). Suppose also that L(x, 0, p) is convex
in p € M
n
for all x e dQ. Then either of the following two conditions implies that u
—
0
is the only critical point of £:
(i) G is a strict variational sub-symmetry
w.r.t.
0,
(ii) if u €
C
2
(fi)
n
CQ(Q)
is a solution of £[u] = 0 and
(£
•
u)(L(x, 0, Vu) - !^!^(x,0, V«)) = 0, on dft,
then necessarily u = 0.
Remark 3.4 1.
2.
For a strict variational sub-symmetry the theorem can be understood heuristically:
since a strict variational sub-symmetry strictly reduces the energy £\u] everywhere
except at u = 0 it is only compatible with a (unique) global minimizer at u = 0.
For example, a strict variational sub-symmetry applied to a mountain-pass reduces
the energy everywhere along a minimizing path, which contradicts the definition of
a mountain-pass.
3.
A verification of condition (ii) is usually achieved via a unique continuation princi-
ple, cf. Section J^.l.
258
4 Applications
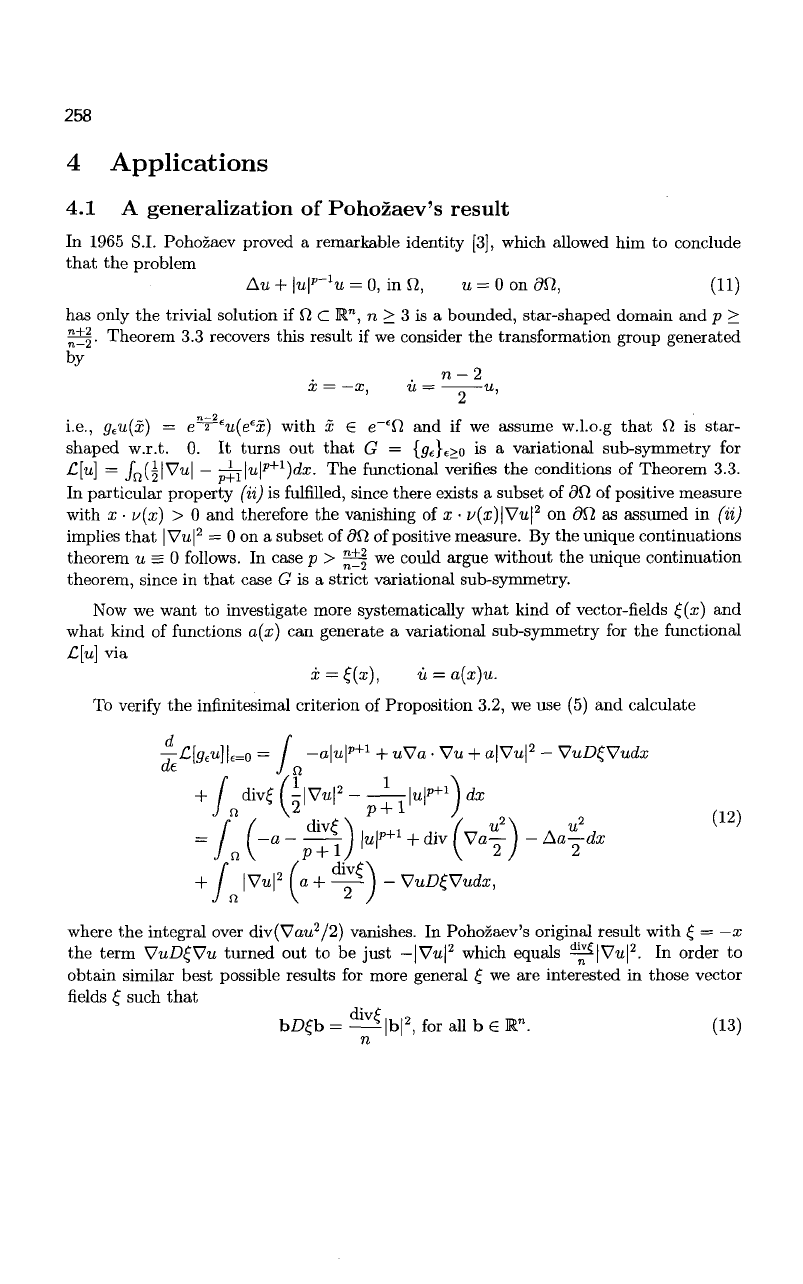
4.1 A generalization of Pohozaev's result
In 1965 S.I. Pohozaev proved a remarkable identity [3], which allowed him to conclude
that the problem
Au + \u\
p
'
l
u = 0, in ft,
M
= 0on<9ft, (11)
has only the trivial solution if ft C R", n > 3 is a bounded, star-shaped domain and p >
jj^§.
Theorem 3.3 recovers this result if we consider the transformation group generated
by
n-2
x = —x, u = —-—u,
i.e., g
e
u(x) = e~f~
c
u(e
e
x) with x €
e
_6
ft
and if we assume w.l.o.g that ft is star-
shaped w.r.t. 0. It turns out that G = {p<J
£
>o is a variational sub-symmetry for
£[u] = /o(ol^
u
l +rl
u
l
p+1
)^
a;
- The functional verifies the conditions of Theorem 3.3.
In particular property (ii) is fulfilled, since there exists a subset of 9ft of positive measure
with x
•
v{x) > 0 and therefore the vanishing of x
•
v(x)\Vu\
2
on 0ft as assumed in (ii)
implies that |Vu|
2
= 0 on a subset of 3ft of positive measure. By the unique continuations
theorem u = 0 follows. In case p >
T
~ we could argue without the unique continuation
theorem, since in that case G is a strict variational sub-symmetry.
Now we want to investigate more systematically what kind of vector-fields £(x) and
what kind of functions a(x) can generate a variational sub-symmetry for the functional
£[u] via
x = t;(x), u = a(x)u.
To verify the infinitesimal criterion of Proposition 3.2, we use (5) and calculate
d
Je
£[ffew]|e=o = / -a\u\
p+1
+ uVa
•
Vu + a\Wu\
2
- VuD^Vudx
J
a
+
/
i)<
.iv
{
(i|v„p-
;r
i
T
i„r)*
+ J \Vu\
2
fa + ^H - VuDiVudx,
where the integral over div(Vau
2
/2) vanishes. In Pohozaev's original result with £ = —x
the term VuD^Vu turned out to be just — |Vw|
2
which equals ^|Vu|
2
. In order to
obtain similar best possible results for more general £ we are interested in those vector
fields £ such that
bD£b = ^
|b|
2
,
for all b 6 K". (13)

259
Lemma 4.1 In R", n > 3 all smooth vector-fields £ which satisfy (13) are (up to a
constant shift) given as linear combinations of the vector-fields
Y
t
j =
XjCi —
x
t
ej, for 1 < i < j < n,
z
i = (
x
i-Y,rfi
x2
j)ei + 2Y,rti
x
i
x
j
e
j, fori =
l...n,
where e; denotes the unit-vector in the x
t
coordinate-direction. The linear combinations
of these vector-fields are called conformal vector-fields.
The proof is obtained by showing first that all component-functions of £ are second-
order polynomials. It is then easy to find the precise form of £. In dimension n = 2 one
can show that the conformal vector-fields are exactly those vector-fields £ = (f
*,
£
2
) such
that ^(x,y) + i£
2
(x,y) is holomorphic in x + iy. Applications of this observation are
discussed in Reichel [5].
We use such a conformal vector-field £ and return to (12) to find
|«u = /
n
(-
fl
-^i)i«r-^
In order to achieve ^C[g
e
u]\
e=0
< 0 it is helpful to choose a = ^f div : £. If we note
that all our conformal vector-fields satisfy Adiv : £ = 0 we finally obtain
S
£
MI- -/
n
(^-
p
-Tl)w
P+ld
^-
Hence the sub-symmetry criterion of Proposition 3.2 will be fulfilled provided div£ < 0
and p > ^|
2
. Uniqueness of the solution « = 0of(ll) now follows from Theorem 3.3.
We sum up our results: .
Definition 4.2 A bounded domain Q C M", n > 3 is
called
conformally contractible if
there exists a conformal vector-field £ such that £(x)
•
v(x) < 0 on dQ with strict inequality
on a subset of 90 of positive measure. The vector-field £ is called an associated vector-
field to fi.
Theorem 4.3 (i) Letp > ^~. IfQ, C R
n
, n > 3 is a bounded conformally contractible
domain with associated vector-field £ and if additionally div£ < 0 in fi then (11)
has only the zero-solution.
(ii) In the special case p = ^| the additional assumption div£ < 0 is not
needed.
Remark 4.4 Part (i) of the theorem holds if
we
replace the non-linearity \u\
p
~
l
u in (11)
by f(u) and assume that F(tu)/t"-i is non-decreasing in t e R for all u e R, where
F(s) =
^f(T)dT.
°* The alternative div : £ > 0 and p < ^| must be discarded for bounded domains since we also
require £
•
v < 0 on d£l. It does however make sense if
Q,
is the complement of a bounded domain.
