Bemelmans J. et. al. (editor) Elliptic and Parabolic Problems: Rolduc and Gaeta 2001

Подождите немного. Документ загружается.

230

det

a>t

- Af,

>jtt

) = 0,

( i t . 1

—

Si i

—

t , \

det A

,i - — A

—*!•

Af,

Jit

= 0,

The computations involve in this result can be found in [13]. It is interesting to

realize that optimal microstructures are second-order rank-one laminates.

6. Concluding remarks

We would like to finish our contribution by briefly comment on some issues.

It is worthwhile to notice that all functions QW(A) given explicitly before are quasi-

convex, and, in particular, the set where they all are finite is a quasiconvex, nonconvex

set, in the sense that weak limits of gradients taking values on it, will also take values

within the same set. In fact, our computations amount to realizing that the polycon-

vexification and the rank-one convexification coincide as is typical in explicit results of

this nature (see for instance [7], [8] among others).

It can actually be shown that the set T can also be determined by using the G-closure

of {al,/91} (1 is the identity matrix). In fact, it is true

r = (A € M

2x2

: there exists 7 e G[al,/31] such that -yA

+ TA

{2)

= o} .

This relationship was noticed in [17] where the idea of introducing a potential to replace

the differential constraint was indeed used and indicated.

Notice how CQW(A, i) is polyconvex in A and convex on t. Joint convexity, however,

is much more than these two separate convexities.

If we further introduce the function

h{A)

= (a + /3)det,4-a^U

(1)

- U<

then the integrand in Theorem 5.2 can also be given by

CQW(A,t) = *_ (p

\A^\

+ |^|

- (at + 0(2 -t)) det A

g(A) > 0, h{A) > 0, n(A) <t<r

(A).

One main objective after having the explicit computation of the previous section is

the numerical approximation of optimal structures via relaxation, i. e. approximate the

optimal solutions of

mini f CQW(VU(x),t(x)) dx

-ff^fi), U

= u

on dtt,

0 <t(x) <1, t(x)dx = \\,

231

and use the local information coming from Theorem 5.2 to build a global picture of

optimal structures all over Q. We hope to address this in the near future ([1]).

Acknowledgements

I would like to thank the organizers of the Fourth European Conference on Elliptic and

Parabolic Problems for inviting me to contribute to this event.

References

[1] Aranda, E., Donoso, A., Pedregal, P., in preparation.

[2] Bellido, J. C, and Pedregal, P., in preparation.

[3] Cherkaev, A., 2000 Variational Methods for Structural Optimization, Springer-Verlag,

New York.

[4] Dacorogna, B. 1989 Direct methods in the Calculus of Variations, Springer.

[5] Fonseca, I., Kinderlehrer, D., Pedregal, P. 1994 Energy functonals depending on

elastic strain and chemical composition, Calc. Var., 2, 283-313.

[6] Grabovsky, Y., 2001 Optimal design problems for two-phase conducting composites

with weakly discontinuous objective functionals, to appear in Advan. Appl. Math.

[7] Kohn, R. 1991 The relaxation of a double-well energy, Cont. Mech. Thermodyn., 3,

193-236.

[8] Kohn, R. V. and Strang, G. 1986 Optimal design and relaxation of variational prob-

lems,

I, II and III, CPAM, 39, 113-137, 139-182 and 353-377.

[9] Le Dret, H., and Raoult, A., 2000 Variational convergence for nonlinear shell models

with directors and related semicontinuity and relaxation results, Arch. Rat. Mech.

Anal., 154, 101-134.

[10] Lipton, R. and Velo, A., 2000 Optimal design of gradient fields with applications

to electrostatics, in Nonlinear Partial Differential Equations and Their Applications,

College de France Seminar, D. Cioranescu, F. Murat, and J.L. Lions eds, Chapman

and Hall/CRC Research Notes in Mathematics.

[11] Murat, F. 1977 Contre-exemples pour divers problemes ou le controle intervient dans

les coefficients, Ann. Mat. Pura ed Appl., Serie 4, 112, 49-68.

[12] Pedregal, P. 1997 Parametrized Measures and Variational Principles, Birkhauser,

Basel.

[13] Pedregal, P. 2001 Constrained quasiconvexification of the square of the gradient of

the state in optimal design, submitted.

[14] Pedregal, P. 2000 Optimal design and constrained quasiconvexity, SIAM J. Math.

Anal., 32, 854-869.

[15] Pedregal, P. 2001 Fully explicit quasiconvexification of the mean-square deviation of

the gradient of the state in optimal design, ERA-AMS, 7, 2001, 72-78.

[16] Pedregal, P. 2000 Constrained quasiconvexity and structural optimization, Arch.

Rat. Mech. Anal., 154, 325-342.

[17] Sverak, V., 1994 Lower semicontinuity of variational integrals and compensated com-

pactness, in S. D. Chatterji, ed., Proc. ICM, vol. 2, Birkhauser, 1153-1158.

232

[18] Tartar, L. 1994 Remarks on optimal design problems, in Calculus of Variations, Ho-

mogenization and Continuum Mechanics, G. Buttazzo, G. Bouchitte and P. Suquet,

eds.,

World Scientific, Singapore, 279-296.

[19] Tartar, L. 2000 An introduction to the homogenization method in optimal design,

Springer Lecture Notes in Math., 1740, 47-156.

A regularity criterion for the angular velocity

component in the case of axisymmetric Navier-Stokes

equations

Milan Pokorny*

Math. Institute of Charles University,

Sokolovska 83, 186 75 Praha 8, Czech Republic

Email: pokorny@karlin.mff.cuni.cz

Abstract

study the instationary Navier-Stokes equations in the entire three-dimensional

space under the assumption that the data are axisymmetric. We improve the regu-

larity criterion for axisymmetric weak solutions given in [10].

1 Introduction

Let us consider the Navier-Stokes equations in the entire three-dimensional space, i.e. the

system of PDE's

•u-Vu-i/Au + Vp = o|

in(0)r)

divu =

(1.1)

u(0,x) = u

(x) inE

where u : (0,T) x R

i-»- K

is the velocity field, p : (0,T) x K

t-> 1R is the pressure,

0 < T < oo, v is the viscosity coefficient, u

is the initial velocity and the forcing term

is,

for the sake of simplicity, considered to be zero. The question of smoothness and

uniqueness of weak solutions to (1.1) is one of the most chalenging problems in the theory

of PDE's. The solution is known to be unique (in the class of all weak solutions satisfying

the energy inequality) if it belongs to the class

(QT)

with ^ + | < 1, r € [2,+oo],

s G [3,+oo] (see [12], [11]). Moreover, if the weak solution belongs to L

) with

- + - < 1, r e [2,+oo], s e (3,+00] and the input data are "smooth enough" then the

solution is smooth. (See [2], [4]).

In the case of the planar flow the weak solution is known to be unique and smooth

as the data of the problem allow (see [8], [5]). Thus a natural question, namely what

'Supported by the Grant Agency of the Czech Republic (grant

No.

201/00/0768) and by the Council

of the Czech Government (project No. 113200007)

233

234

can be said about the axisymmetric flow, appears. The first results in this direction were

obtained in the late sixties for ug = 0 (see [6], [14]) and recently also in [7].

The case ug ^ 0, including the 2-axis, was for the first time considered in the paper

[10] where for u

e L

) with f + f < 1, r e

[2,

+oo], s e

(3,

+co] or for u

£ L

)

with

+ § < £, r e [f

,+oo],

s e [6,+co] and f + | < l _ A,

r e

[10,+oo], s e (f ,6)

the smoothness and thus also the uniqueness in the class of weak solutions satisfying the

energy inequality was obtained. See also [1] where the authors give some more smoothness

criteria for the vorticity components. Note that the criterion on u

probably cannot be

improved by the present technique while the regularity criteria on ug are not optimal from

the scaling argument.

Here we would like to improve the regularity criteria on ug in such a way that it

(almost) undergoes the correct scaling. Our main result is as follows

Theorem 1 Let u be a weak solution to problem (1.1) satisfying the energy inequality

with u

e W

) so that Vu

€ L

) and (u

)gr € L°°(R

). Let u

be axisymmetric.

Suppose further that the angular component ug of u belongs to

I/'"(QT)

for some r €

(2,+oo],

s G (4,+oo],

+ | < 1. Then (u,p), where p is the corresponding pressure, is

an axisymmetric strong solution to problem (1.1) which is unique in the class of all weak

solutions satisfying the energy inequality.

Note that under a axisymmetric solution we understand a pair (u,p) such that in

cylindrical coordinates (r, 0, z), r e

[0,

oo), 9 e [0,27r) and z£f, u

ug and u

, considered

in cylindrical coordinates, are independent of 6, and p, written in cylindrical coordinates,

is also independent of 9.

2 Preliminaries

Denote by (u

,ue,u

) the cylindrical coordinates of the vector field u and by (ui

,uig,ui

)

the cylindrical coordinates of curl u, i.e. u)

= —

^jjf-,

w« = ^

—

^jf- and u

= \-§^(rwo) for

u an axisymmetric field. Moreover, let w = (wi, w

) denote the cartesian coordinates

of curl u.

We will use the standard notation for the Lebesgue spaces Z^R

) equipped with the

standard norm || • ||

PiR

3 and the Sobolev spaces

)

equipped with the standard

norm || •

||fc

lP;H

= (0, T) x R

we denote the anisotropic Lebesgue space

(0,T;

)). If no confusion can arise we skip writing R

and QT, respectively.

Vector-valued functions are printed boldfaced. Nonetheless, we do not distinguish

between L«(R

)

and L«(R

In order to keep a simple notation, all generic constants will be denoted by C; thus C

can have different values from term to term, even in the same formula.

By Du we mean the gradient of u expressed in the cartesian coordinates, while Vu

denotes the derivatives with respect to r and z only (u is axisymmetric). Similarly for u

and u

We will use the following inequalities (for the proofs of Lemmas 1-3 see [10])

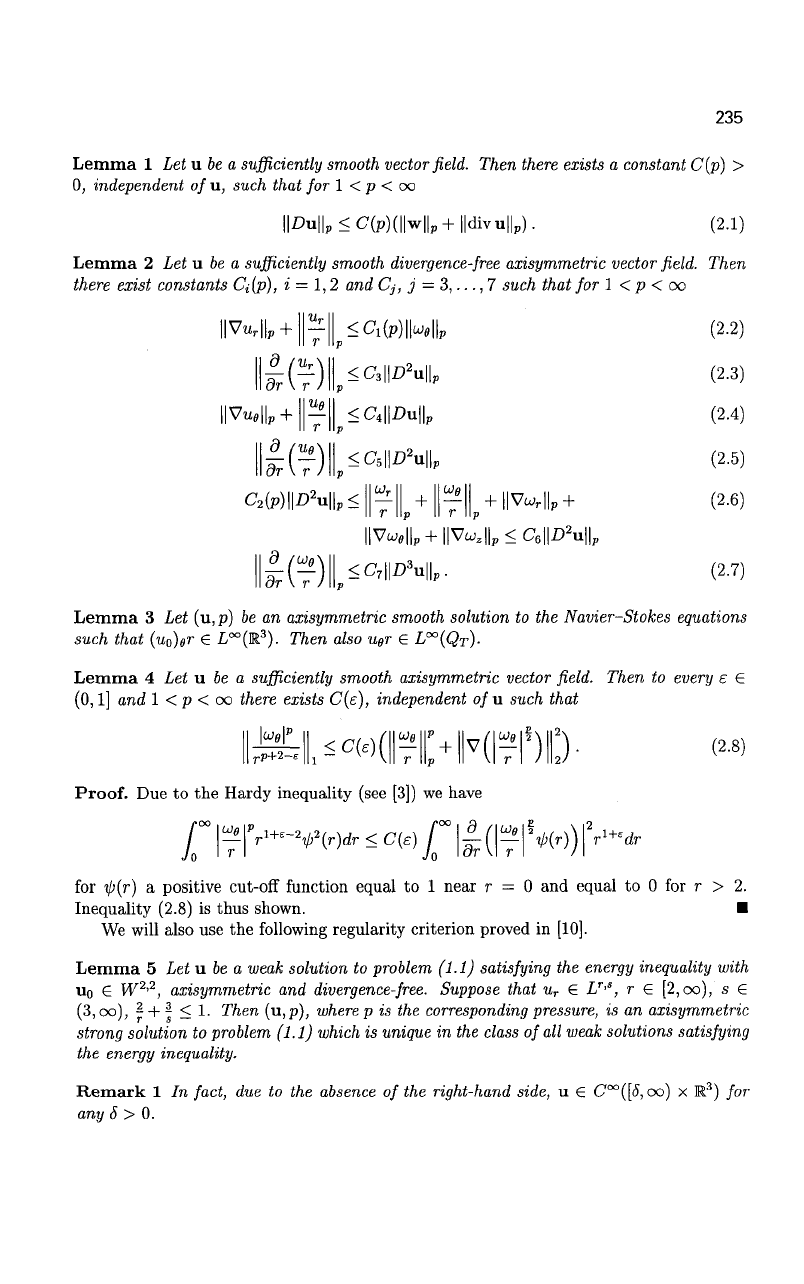
235

Lemma 1 Let u be a sufficiently smooth vector

field.

Then there exists a constant C(p) >

0, independent of u, such that for 1 < p < oo

||Z>u||,<C(p)(||w||, + ||divuy. (2.1)

Lemma 2 Let u be a sufficiently smooth divergence-free axisymmetric vector

field.

Then

there exist constants Ci(p), i = 1,2 and Cj, j =

3,...,

7 such that for 1 < p < oo

llVtirllp+pJ <d(p)N||

(2.2)

ii r lip

I 9 /w

\dr\rJ\\p

)||

< 0.||Z)

u||,, (2.3)

/ lip

HVu,||

+ p|| <C

||-Du||

(2.4)

r \\p

|£(?)|,<<*l|2*%

(2.5)

(p)||.D

u||p<||^|| +p| + ||Vu,

+ (2.6)

II r lip II r lip

||Vw,||, + ||Vw,||

||D

u||

Lemma 3 Let

(u,

p) be an axisymmetric smooth solution to the Navier-Stokes equations

such that (u

)

r e L°°(R

). Then also u

r G

L°°(QT)-

Lemma 4 Let u be a sufficiently smooth axisymmetric vector

field.

Then to every e G

(0,1] and 1 < p < oo there exists C(e), independent o/u s«cft i/iai

<c(

)(|p|r

||v(phin.

(2.8)

i VII r lip II VI r I /

112/

p+2-E

Proof.

Due to the Hardy inequality (see [3]) we have

r^~^(r)dr<C{e) f°

||-(|^|

V(0) |V

rfr

Jo I r I 7

lor

r I /I

for t/)(r) a positive cut-off function equal to 1 near r = 0 and equal to 0 for r > 2.

Inequality (2.8) is thus shown. •

We will also use the following regularity criterion proved in [10].

Lemma 5 Let u be a weak solution to problem (1.1) satisfying the energy inequality with

Uo £ W

, axisymmetric and divergence-free. Suppose that u

6 L

r,s

, r e [2,oo), s e

(3,

oo), - + - < 1. Then (u,p),

where

p is the corresponding pressure, is an axisymmetric

strong solution to problem (1.1) which is unique in the class of

all

weak solutions satisfying

the energy inequality.

Remark 1 In fact, due to the absence of the right-hand side, u €

C°°([5,

oo) x R

) for

any 6 > 0.

236

The improvement of the results from [10] is based on Corollary 1 below. We will use

the following result concerning the Muckenhoupt classes (see e.g. [13] for the definition

and other properties)

Lemma 6 Let r denote the distance of a point in R

from the z-axis. Then r

6 A

for

any 1 < p < oo if

—2

< q < 0.

Recall that for divergence-free functions the gradient can be computed from the vor-

ticity by means of a singular integral operator with properties analogical to the Riesz

transform. For such operators (see e.g. [13]) we have the LP weighted estimates exactly

for the weights from A

classes, 1 < p < oo. Thus we get from Lemma 6

Corollary 1 Let 1 < p < oo and 0 < q < -. Then there exists a constant C(p,q) such

that

llr

9|lp

'\\ri\\

Finally, we will need the following result on the integrability of gradients of u with

some p's less than 2 (see [9] for the proof)

Lemma 7 Let moreover Du

6 L

. Then the weak solution to (1.1) also satisfies Du e

and D

u e IS'

, 1 < p < 2.

3 Proof of Theorem 1

The proof is based on the continuation argument. We have the following (more or less

standard) result

Lemma 8 Let Uo £ W

. Then there exists to > 0 and (u,p), a weak solution to

system

(1.1),

which is a strong solution on the time interval (0,io)- Moreover, if

axisymmetric then also the strong solution is axisymmetric.

Now let u

be as in Lemma 8 (axisymmetric). We define:

t* = sup

t > 0; there exists an axisymmetric strong

solution to (1.1) on (0,t) [

It follows from Lemma 8 that t* > 0. Now let (u,p) be a weak solution to the

Navier-Stokes system as in Theorem 1. Due to the uniqueness property, it coincides

with the strong solution from Lemma 8 on any compact subinterval of

[Q,t*).

There

are two possibilities. Either i* = oo and we have the global-in-time regular solution, or

t* < oo. However, we will exclude this possibility by showing that u

satisfies on (0,£*)

the assumptions of Lemma 5. To this aim we will essentially use both the information

about the better regularity of one velocity component and the fact that the solution is

axisymmetric.

There are also some positive values of q for which r

e A

but we do not need it here.

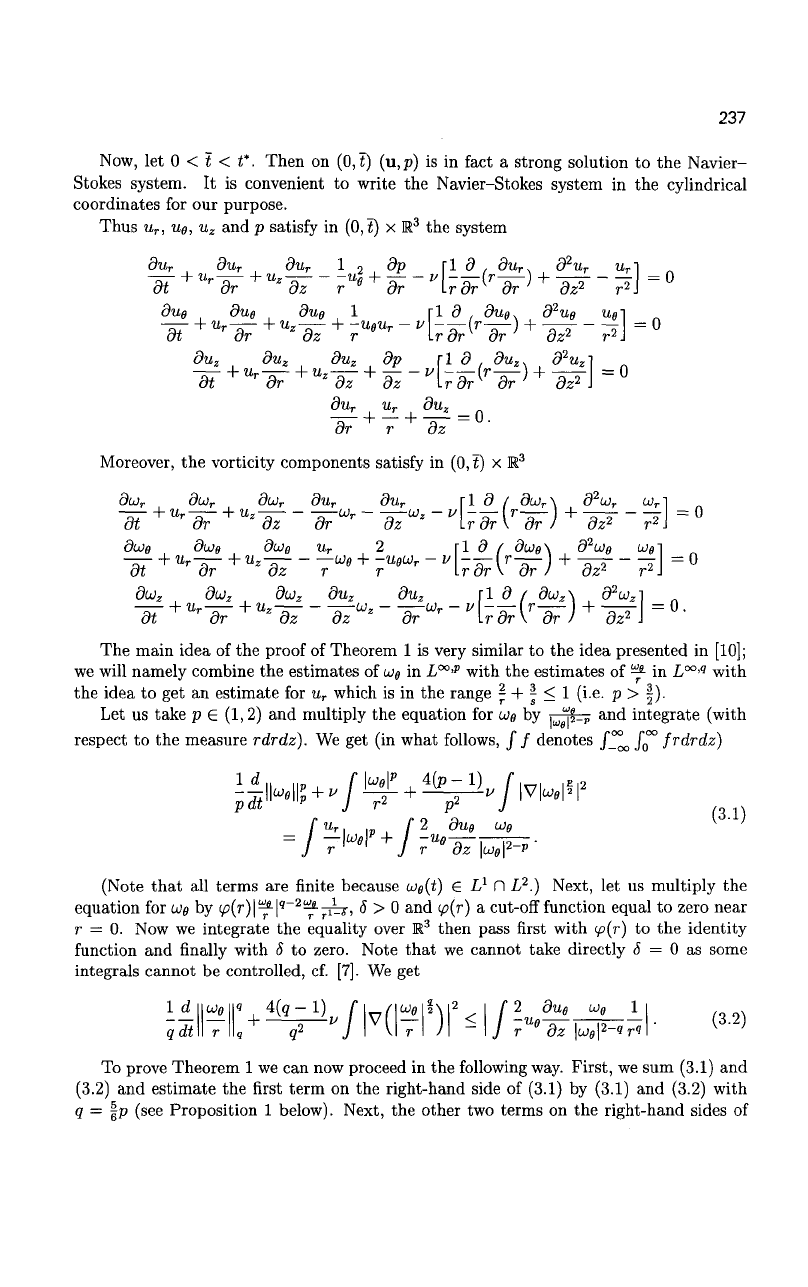
237

Now,

let 0 < t < t*.

Then

on (0,1) (u,p) is in

fact

strong solution

to the

Navier-

Stokes system.

It is

convenient

write

the

Navier-Stokes system

in the

cylindrical

coordinates

for our

purpose.

Thus

, ue, u

and p

satisfy

in (0,t) x R

the

system

^ du

r i

__ du

, dp fl 9, du

„,

"at

dp [1 o . du

6' u

dz r

dr lr dr dr dz

due

due_ due 1 [1 d du

~\ _

dz r

Ir dr dr dz

^£,^P_

1 d

, du

. d

-i _

dz dz lr dr dr dz

du.

r dz

Moreover,

the

vorticity components satisfy

(0,

t) x

duj

dui

rl d (

du>

--k\ =0

ou>

ou)

r / "''M

dt dr

dz dr dz Lr dr\ dr J dz

due , dw

due u

2 rl d ( du

\ , d

ue~\

—- + u

-— + u

— w

-uoU

- v —5-I r-r- + -5-5 5- = 0

dt dr dz r r lr or \ dr 1 dz* r

^£

+ u

^£

+ u

^i_

dUz

^ ^'u -J-^-f

^!£l)

dt dr

dz dz dr lr dr\ dr J dz

The main idea

the proof

Theorem

1 is

very similar

to the

idea presented

[10];

we will namely combine

the

estimates

uje

L°°'

with

the

estimates

of ^ in

L°°'

with

the idea

to get an

estimate

for u

which

is in the

range

* + | < 1 (i.e. p > §).

Let

take

pe (1,2) and

multiply

the

equation

for we by ,J%-

and

integrate (with

respect

to the

measure rdrdz).

We get (in

what follows,

f f

denotes

frdrdz)

>*+»/^

'"'

a (3-1)

,„ / 2 due we

I.. IB ,

/>r

" dz

\we\

-v'

(Note that

all

terms

are

finite because u)e(t)

G L

n L

Next,

let us

multiply

the

equation

for

u>e

tp(r)\

^-r=r,

5 > 0 and ip(r) a

cut-off function equal

zero near

= 0. Now we

integrate

the

equality over M.

then pass first with

ip(r) to the

identity

function

and

finally with

S to

zero. Note that

cannot take directly

S = 0 as

some

integrals cannot

controlled,

cf. [7]. We get

1 dnugw 4(g-l) [\

(\we\l\\

I [2 3a, % ll .

To prove Theorem 1 we can now proceed in the following way. First, we sum (3.1) and

(3.2) and estimate the first term on the right-hand side of (3.1) by (3.1) and (3.2) with

g = |p (see Proposition 1 below). Next, the other two terms on the right-hand sides of

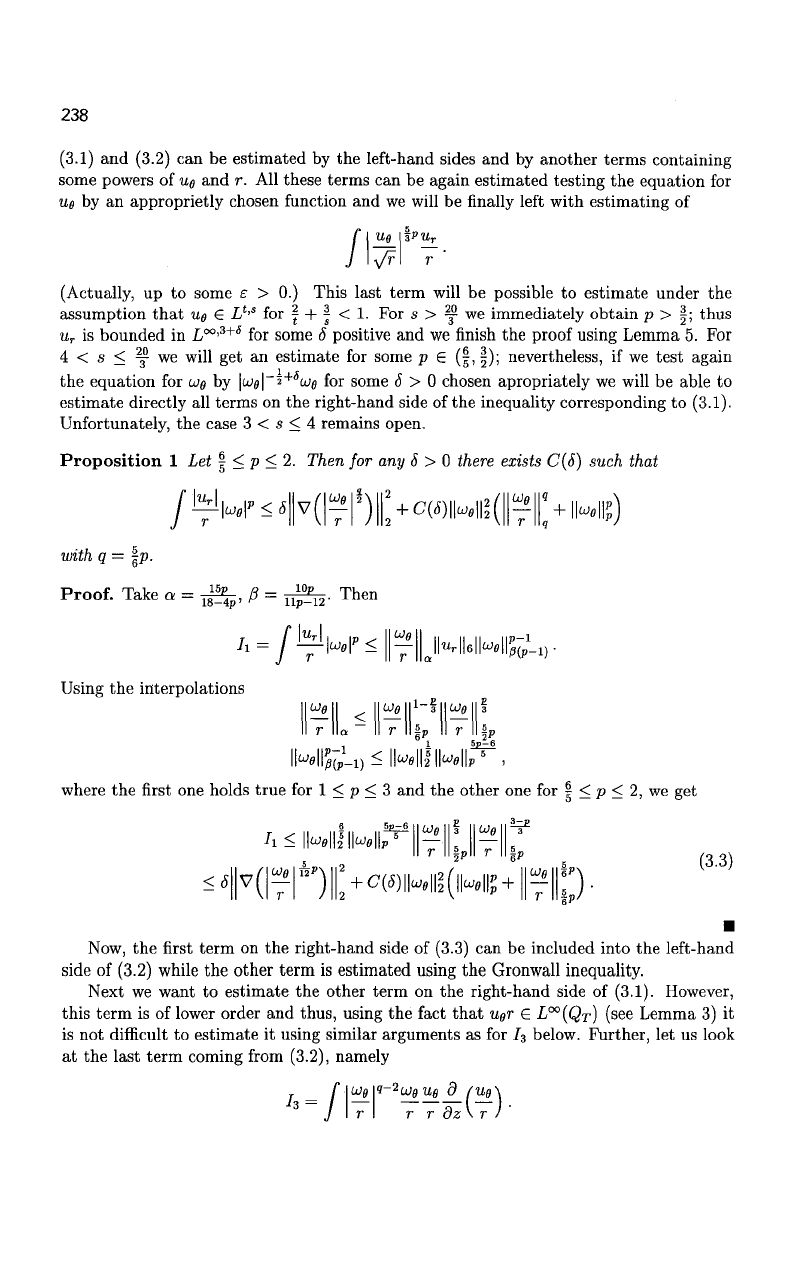
238

(3.1) and (3.2) can be estimated by the left-hand sides and by another terms containing

some powers of

and r. All these terms can be again estimated testing the equation for

ue by an approprietly chosen function and we will be finally left with estimating of

\zfu

y/r\

(Actually, up to some e > 0.) This last term will be possible to estimate under the

assumption that

€ L

for | + | < 1. For s >

™

we immediately obtain p > f; thus

is bounded in L°°'

3+s

for some 5 positive and we finish the proof using Lemma 5. For

4 < s < Y

we wm

estimate for some p e (|, §); nevertheless, if we test again

the equation for

\ue\"^

LUo

for some 6 > 0 chosen apropriately we will be able to

estimate directly all terms on the right-hand side of the inequality corresponding to (3.1).

Unfortunately, the case 3 < s < 4 remains open.

Proposition 1 Let | < p < 2. Then for any S > 0 there exists C(8) such that

with q = |p.

Proof.

Take a = ^, p = ^.. Then

Using the interpolations

£(p-i)'

||W0||

nweiii-f ||W9||I

IIf

II^II^D^INIlllKllp^

where the first one holds true for 1 < p < 3 and the other one for | < p < 2, we get

(3.3)

<S\\

^^MIIWI^ITIIJITI?

Now, the first term on the right-hand side of (3.3) can be included into the left-hand

side of (3.2) while the other term is estimated using the Gronwall inequality.

Next we want to estimate the other term on the right-hand side of (3.1). However,

this term is of lower order and thus, using the fact that ugr G L°°(Q

) (see Lemma 3) it

is not difficult to estimate it using similar arguments as for I

below. Further, let us look

at the last term coming from (3.2), namely

r I r r dz\ r '

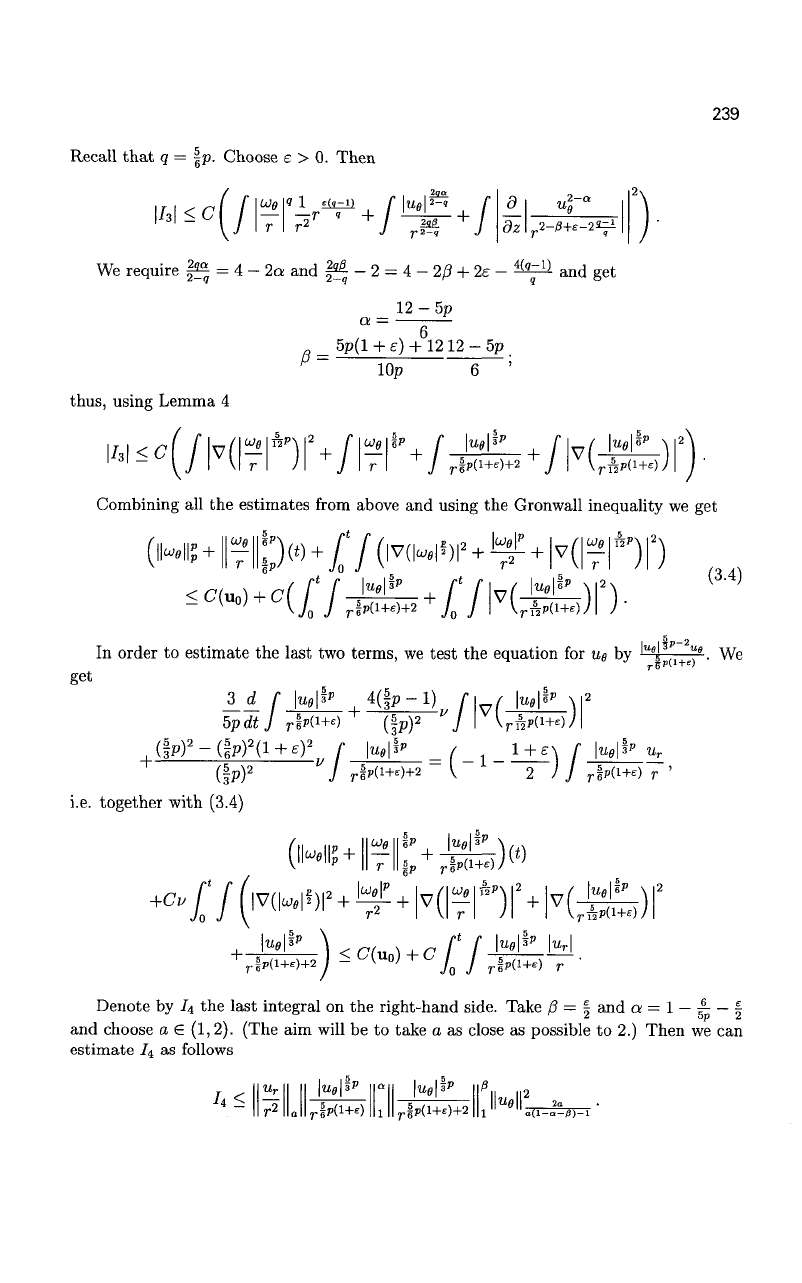
239

I uj

2-ns-23=l

Recall that

q = |p.

Choose

e > 0.

Then

We require

= 4 - 2a and

- 2 = 4 -

2/?

+ 2e - ^zil

d get

12-5p

= —g

5p(l

12 12

- 5p

lOp

6 '

thus,

using Lemma

\r\<r(

[\v(\

\*"M\

" i f\v(

MlP

lhl

{Jr[\v\

J\v\

7i^^

l r\7&w)

Combining

all the

estimates from above

and

using

the

Gronwall inequality we

get

(''"•HI?l>

/7(

<i"'

|!+

KI?l*')f)

, ,

In order

estimate

the

last

two

terms,

test

the

equation

for ua by

^"'J

"". We

get

d f Mi' 4(fp-l) /•• /

|U,|JP

di/

fp(i+o

(|p)2 y I

AP(i+

);l

5pc

(fp)

-(|P)

+ ^ /• l^ft"

/_ i+£\ /• Mb u

|p(i+^)+2

I 2 ) J

fp(i+<0

(b)

i.e. together with

(3.4)

(MHI^'

kl§P

'sP

M*F

|p(l+

)+2

Denote

by 7

the

last integral

on the

right-hand side. Take

= § and a =

—

and choose

a £

(1,2).

(The aim

will

be to

take

a as

possible

to 2.)

Then

we can

estimate

follows

"HI

l"«l

«el

II".,

ILII

|p(i+e) Hi II

|p(i+

)+2

llJ'^^d-^)-!