Bemelmans J. et. al. (editor) Elliptic and Parabolic Problems: Rolduc and Gaeta 2001
Подождите немного. Документ загружается.

240
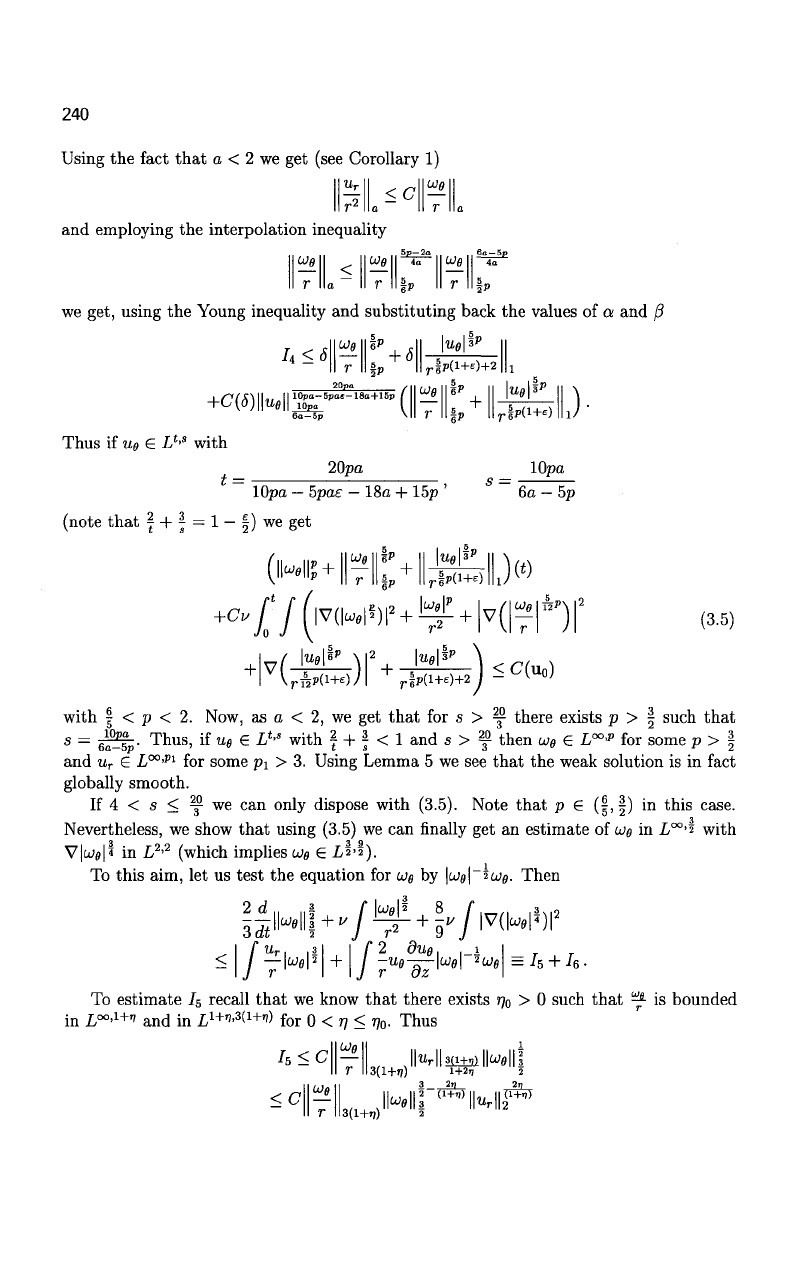
Using the fact that
o < 2
we get (see Corollary 1)
II —II
II T
\\a
and employing the interpolation inequality
—II <c||—II
T
\\a II T \\a
5p-2o
6q-5p
II
r
\\a
M
r
iijfp
II r
II|
P
we get, using the Young inequality and substituting back the values of
a
and
,
r
II
fp ll
r
fj>(i+0+2||i
.
2
°P
a
/lli^llip II biolfP
sr^
VII r
III?
III-IPCH-OIII/
6o-5p
Ml /
II gP
llye^
Thus
if
u
e
e £
M
with
20pa
10pa
t = — :: r-r
T7T-
, s =
Wpa
—
5pas
—
18a
+
15p
'
6a
—
5p
(note that
\ + f =
1
-
§) we get
/'ll,,
||P
i
II
^H*"
i
II
l^l
1
"
II
W
v
IWI?
+
||-||
ip
+
||^pi^)||JW
^/7(|V(Wl)P
+
^
+
|v(|^|
AP
)|
2
(3
.5)
+|
V
V^(T^)J|
+
r
|p(i
+E
)
+
2J ^
C
(
u
°)
with
| < p < 2.
Now,
as a < 2,
we get that
for s > y
there exists
p > |
such that
s
= g^.
Thus,
if
u
B
e L
l
'
s
with
f +
f<lands>f then
u
e
e
L°°'
p
for some
p
>
§
and
u
r
G
L°°>PI
for
some pi
>
3. Using Lemma 5 we see that the weak solution is
in
fact
globally smooth.
If
4 < s < j
we can only dispose with (3.5). Note that
p e (|, |) in
this case.
Nevertheless, we show that using (3.5) we can finally get an estimate of
uig
in
L°°'? with
V]aj0|*
in
L
2
'
2
(which implies
w«
6
iJ'z).
To this aim, let us test the equation for
we
by
\UQ\~IUJO.
Then
-/^
+
^/|v(W!)|
2
2 d
n
nl , fM
3S
M
l
+,
'„
U
r
.
.3
I
I f 2 dug. ._. .
—
N
2
+ /
~
u
0^—N
2W
« \=h + h-
r
\
\
J
r az I
To estimate
Is
recall that we know that there exists
r]
0
> 0
such that
^ is
bounded
in L
00
-
1
"^ and
in
Li+».3(i+i)
for 0 <
?7
<
7y
0
. Thus
/5<C|^||
|K||j
e±sl
||
W
,||J
II
r
Il3(l+lj) l+2l
2
ii,,
II 3 2n 2tj
-
C
|lTll3(l
+
,)
l|W,
'
ll
f
l|Wr
'
12

241
and therefore we can estimate I
5
using the Gronwall inequality. Finally, to estimate I
6
, we
I
l
1+r
>
will use the fact that there exists r?i > 0 such that for all 0 < r\ <
T)\,
p£ is bounded
in
L°°'
2
and its gradient in L
2,2
.
2
Now
T
^
11
IIIIIVT/I
U
» \
l+n
\\\ III
u
<>
sll \\Jr\ /IkllU/r
u
e
\
1+
i\\\ III ue
I
6
'
1
"'''!!?
s/r\ /Il2lll
v
^'l
Hi
and thus 7
6
can be estimated by means of the Gronwall inequality. The proof of Theorem
1 is finished.
References
1] Chae D., Lee J.: On the Regularity of Axisymmetric Solutions to the Navier-Stokes
Equations, (preprint).
2] Galdi G.P.: An Introduction to the Navier-Stokes Initial Boundary Value Problem,
In: Fundamental directions in Mathematical Fluid Mechanics, editors G.P.
Galdi, J.G. Heywood, & R. Ranacher, Birkhauser Verlag (2000).
3] Hardy G.H., Littlewood J.E., Polya G.: Inequalities, Cambridge University Press
(1952).
4] Kozono H., Sohr H.: Remark on uniqueness of weak solutions to the Navier-Stokes
equations, Analysis 16 (1996)
255-271.
5] Ladyzhenskaya O.A.: The mathematical theory of viscous incompressible
flow, Gordon and Breach, New York-London-Paris (1969).
6] Ladyzhenskaya O.A.: On the unique global solvability of the Cauchy prob-
lem for the Navier-Stokes equations in the presence of the axial symmetry,
Zap.
Nauch. Sem. LOMI 7 (1968) 155-177 (in Russian).
7] Leonardi S., Malek J., Necas J., Pokorny M.: On axially symmetric flows in R
3
, ZAA
18 (1999) 639-649.
8] Leray J.: Etude de diverses equations integrates non lineaires et de
quelques
problemes
que pose I'hydrodynamique, J. Math. Pures Appl. IX. Ser. 12 (1933) 1-82.
9] Lions, P.-L.: Mathematical Topics in Fluid Mechanics, Volume I: Incom-
pressible Models, Clarendon Press, Oxford (1996).
[10] Neustupa J., Pokorny, M.: Axisymmetric flow of Navier-Stokes fluid in the whole
space with non-zero angular velocity component, Math. Boh. 126, No. 2 (2001).
2
Actually, we have
1"'J',,
, 6 L
00,2
and its gradient belongs to L
2,2
for some e > 0; but this
information is stronger since the main problems are near r = 0.

242
[11] Prodi G.: Un teorema di unicita per el equazioni di Navier-Stokes, Ann. Mat. Pura
Appl. 48 (1959) 173-182.
[12] Serrin J.: The initial boundary value problem for the Navier-Stokes equations, In:
Nonlinear Problems, ed. Langer R.E., University of Wisconsin Press (1963).
[13] Turesson B.O.: Nonlinear Potential Theory and Weighted Sobolev Spaces,
Springer Verlag, Lecture Notes in Mathematics (2000).
[14] Uchovskii M.R., Yudovich B.I.: Axially symmetric flows of an ideal and viscous
fluid,
J. Appl. Math. Mech. 32 (1968)
52-61.
A Comparison Principle for the p-Laplacian
Arkady Poliakovsky and Itai Shafrir
Department of Mathematics
Technion-I.I.T
32000 Haifa, Israel
1 Introduction
The purpose of this note is to present a quite general comparison principle between sub
and super solutions for singular equations involving the p—Laplacian. We are motivated
by two such results in the case p = 2. The first is the following theorem of Agmon [2,
Theorem 2.7] that we give in a slightly modified form.
Theorem 1.1 (Agmon). Let a(x)
€_L]
0C
(R.
N
\{0}),
R>0 andu,v e C(n
R
)nHl
c
(n
R
),
with Q
R
:= {\x\ > R} and v > 0 on Q
R
, satisfy (in the weak sense),
—At;
—
a(x)v > 0 > —AM
—
a(x)u in Q
R
.
Suppose also that
v(x) > u(x) on {\x\ = R},
and that for some a > 1 we have,
liminfiT
2
/ u
2
= 0. (1.1)
K
^°° J{K<\x\<aK)
Then,
v > u in Q
R
.
Adapting Agmon's method to a different setting, Marcus, Mizel and Pinchover proved
in [5, Lemma 8] the following result (again we slightly modify the statement),
Theorem 1.2 (Marcus-Mizel-Pinchover). Let Q be a proper subdomain ofR
N
with
compact boundary and a(x) e
L}
0C
{Q,).
Set 8{x) = dist(x, dQ) and assume that
/3
> 0 is
such that Sj := {i 6 fl : 5(x) = 6} / 0. Suppose that u,v e C^p U E^) n
H}
0C
{Q,^),
with Qp := {x e
CI
: 6(x) < /?} and v > 0 on ftp
U
E^, satisfy (in the weak sense),
—Av
—
a(x)v > 0 > —Au
—
a(x)u in Up.
Suppose also that
v(x) > u(x) on Ejj,
and that for some a > 1 we have,
liminfr--
2
/ u
2
= 0. (1.2)
r_+0
J{xm-.r<S(x)<ar}
Then,
v > u in Qp.
243

244
In
[9,
Proposition A.l] Shafrir proved
a
generalization
of
Theorem
1.2 for any 1
<
p
<
oo. The purpose
of
this note
is to
present
a
comparison principle which unifies and
generalizes
all the
above mentioned results
for any 1
< p <
oo. We
basically follow
the strategy
of
[9],
but
we make
a
new key observation about
a
choice
of
test functions
which enables
us to
improve
the
known results even
in the
case
p
= 2. In
particular,
this improvement shows that
in
both (1.1)
and
(1.2)
it
is
enough
to
require that
the
corresponding limits are finite (i.e.
not
necessarily zero)
in
order
for the
conclusions
to
hold. We also present some applications
for
uniqueness and Liouville type results.
We begin with some notations and definitions.
In
the sequel
let Q be
a
proper sub-
domain
of R^
whose boundary
dfl is
a
disjoint union
of
two compact sets
T
0
and IV
We assume that
I\ ^
0, but
To may
be
empty. When To 7^
0
we define
the
distance
function
5
a
(x)
=
dist(x, r
0
),
Va; G
M.
N
.
In
case
T
0
= 0 it
will
be
convenient
to set
5
0
=
0. For
p
e
(1,00) we denote by
A
p
u =
div(|Vu\
p
~
2
Vu) the p-Laplacian of u. Given
a(x)
6
L|
oc
(Q) we shall say that
a
function
v
G
W^(Q)
nC(Q) is
a
super solution for the
equation,
-A
p
u-o(x)|u|
p
"
2
u
=
0,
inQ, (1.3)
if
f \Vv\"-
2
VvV4>
-
a{x)\v\<>-
2
v(t>
>
0, for
all 0
<
<j>
e
C
C
°°(Q).
(1.4)
The notions of subsolution and solution are defined similarly by replacing ">"
in
(1.4)
by
"<"
and
"=" respectively. For
0 < a
< b we denote,
Q
K6)
=
{i£fi
:
6
0
(x)
e
(a,b)} and Q
w>)
=
{x
e « :
\x\
6
(a,
6)}.
Consider first
p €
(1,2]
and
a
function
u e
W^{Q). We shall say that
u
satisfies
condition (Co)
if
for
some
0
< a
< 1
there exist
for
each
n >
2,
n
positive numbers
{r<
n)
};?
=1
such that,
(n)
., (n) -, „ 1
r}
+
i^
ffr
j
. J =
l,...,n-l,
with
lim
rj™
=
0
and
n—>oo
i™^i:/
i^)'=o.
(1.5)
For
p
>
2
we replace
in
the definition (1.5)
by
m jff-isjL^®"-'
Condition (C
0
)
is a
growth condition
for v
near To
(it is
trivially satisfied when
F
0
=
0).
We now define analogously
a
growth condition
at
infinity (Coo), in the case of unbounded
Q.. As above, consider first the case
p
G (1,2] and
a
function
u
€
W
lo
f(0). We shall say
that
u
satisfies condition (Co) if for some 0
<
r
<
1 there exist for each
n >
2,
n
positive
numbers {p^'}™
=1
such that,
(n)
^ in) -, _ 1
p)+i<rp)',
j
=
l,...,n-l,

245
with lim p„ = oo and
n—»oo
lim
i^/
w
,„,
(My=o.
(i.7)
For p > 2 we replace in the definition (1.7) by
J5S,
i g
/^M',
(R)'
+
^
g
/
0
<^M->>'
v
"
r
(R)
2
=
°"
(L8)
Our main result is the following.
Theorem 1.3. Consider ft as above, p 6 (l,oo) and a(x) e L^Q). Suppose that
u, v e C(ft
U
Ti) n W,^?(ft) are such that v is a super solution for (1.3), v > 0 onQuTi,
u is a subsolution for (1.3) satisfying condition (C
0
), j/r
0
^ 0, and condition (Coo), */ft
is unbounded. Assume also that
v>u onTi. (1.9)
X/ien,
ii > u in ft. (1.10)
2 Proof of the main result
For the proof we use two basic tools that we shall now recall. The first is a Picone
identity for the p-Laplacian, which is due to Allegretto and Huang [3, Theorem 1.1]. We
summarize it in the next lemma.
Lemma 2.1. Let G be a domain in R
N
. For u, v e C(G) n W^(G) such that u > 0 and
v > 0 in G, denote
L{u, v) = IVu\
p
+ (p- 1)—IVv\" - p—rVwlVv\"-
2
Vv ,
VP v? (2.1)
R(u, v) = |Vu|
p
- v(-^—) \Vv\"-
2
Vv .
Then L{u,v) = R(u, v) a.e. in G. Moreover, L(u, v) > 0 a.e. in G, and L(u, v) = 0 a.e in
G if and only if u = kv for some constant k.
The second tool is given by the following simple inequalities from [9, Lemma A.4].
Lemma 2.2. (i) If p > 2 then
\z
1
+ z
2
\"-\z
1
\'
,
-p\z
1
r
2
z
1
-z
2
< ^^(ki| + \z
2
\)
p
-
2
\z
2
\
2
, Vz
u
z
2
€ R*. (2.2)
(ii) Ifp<2 then there exists a constant j
p
> 0 such that
\zi+z
a
?-\z
1
Jf-p\z
1
JT
2
z
1
-Z2<'y
P
\z
t
^,
V
Zl
,z
2
eR
N
..
(2.3)
246
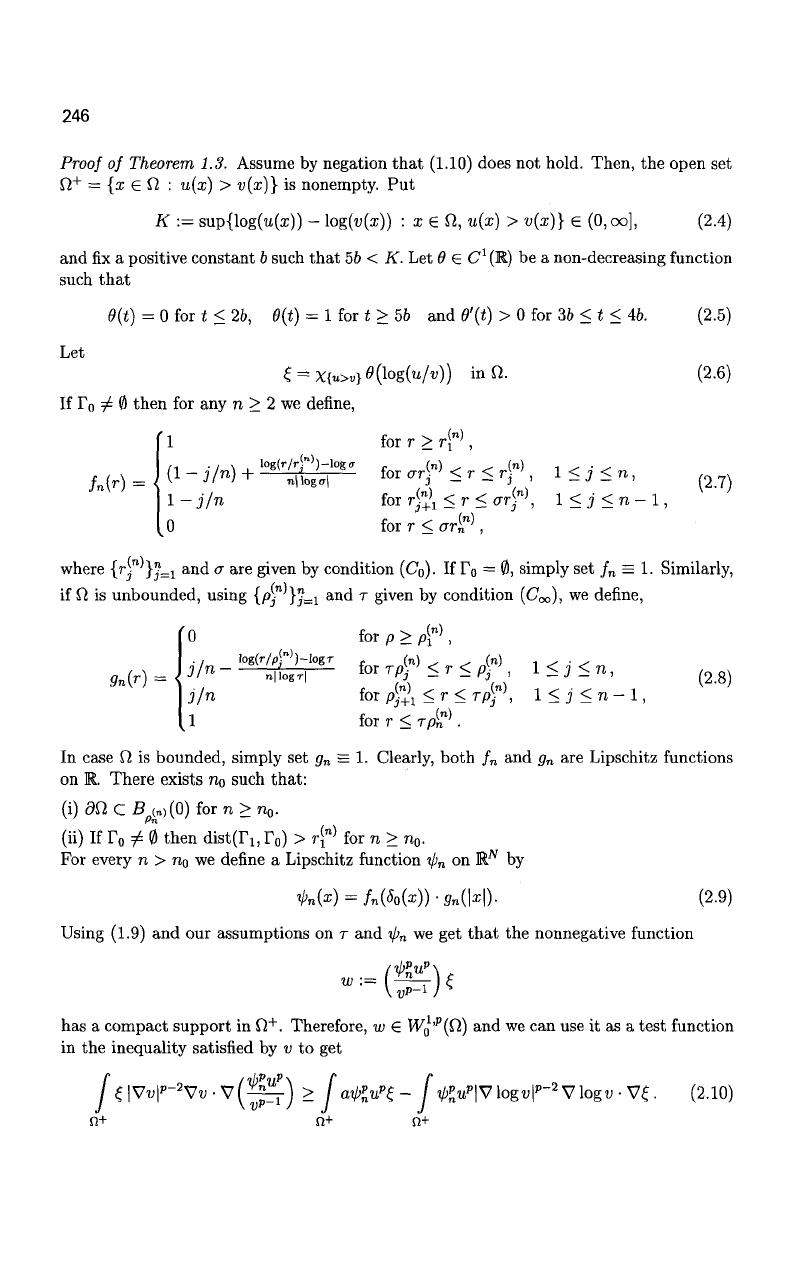
Proof of Theorem 1.3. Assume by negation that (1.10) does not hold. Then, the open set
Q+ = {x e Q : u(x) > v(x)} is nonempty. Put
K := sup{log(u(a;)) - log(u(i)) :i6(l, u(x) > v(x)} 6
(0,oo],
(2.4)
and fix a positive constant
6
such that 56 < K. Let SsC
1
(R)
be a non-decreasing function
such that
9(i) = 0 for t < 26, 9{t) = 1 for t > 56 and 6'{t) > 0 for 36 < t < 46. (2.5)
Let
£ =
X[u>v] &
(log(u/i>)) in O.
If T
0
^ 0 then for any n > 2 we define,
/»M
=
<
1
.W
(l-^
+
^^St^
1 - j/n
0
for r > r\
log(r/r<
n)
)-log<T („) („) .
,' . for crr„- ' < r < rl- , 1 < 7 < n
for r^ < r < ffrf >, 1 < j < n - 1,
for r < or)? ,
(2.6)
(2.7)
where
{ry'}^
=1
and a are given by condition (C
0
). If r
0
= 0, simply set /„ = 1. Similarly,
if
Q.
is unbounded, using {py }™
=1
and r given by condition (Coo), we define,
9n{r) =
<
0
j/n-
j/n
1
for p >
P
W
>
'
og(r/p
r
J)
~'°
gT
for rpf < r < pf , l<j<n,
forp^^r^Tp^, l<j<n-l,
n|logr|
(2.8)
for r < rp.
,(<•)
In case fi is bounded, simply set g
n
= 1. Clearly, both /„ and g
n
are Lipschitz functions
on R. There exists n
0
such that:
(i) 90 C B
oo (0)
for n> n
0
.
(ii) If T
0
+ 0 then dist(r
x
, T
0
) > r[
n)
for n>n
0
.
For every n > rc
0
we define a Lipschitz function ip
n
on R
N
by
^(*) = /n(«o(x))-ft,(N). (2.9)
Using (1.9) and our assumptions on r and ip
n
we get that the nonnegative function
has a compact support in Q
+
. Therefore, w € W
0
1,!>
(fi) and we can use it as a test function
in the inequality satisfied by v to get
[ i\Vv\*-
2
Vv-v(^^j > f a^u"i- [il%u
p
\V\ogv\
p
-
2
VlagvVZ. (2.10)
n+ n+
n+

247
By (2.10) we infer that
0< f L{ij
n
u,v)Z= f RWnU,v)£
Jn+ Jn+
Jn+ Jn+ VW i
J
< [ \V(4>
n
u)n - f o^JuPf
+ I ^
p
u
p
|Vlog«;|
p
-
2
Vlogi>- Vf,
where L(u,v) and R(u, v) are defined in (2.1). Similarly, the nonnegative function
has compact support in fi
+
and therefore z 6
W
0
'
P
(Q).
Testing the inequality satisfied
by u against z yields
0 > / (-div(|V«|
p
-
2
Vu) - a|M|
p
-
2
u)i/<>£
(2.12)
= / £|Vu|
p
-
2
Vw
•
V««) + / V>|Vur
2
Vu-V£ - / <#>
p
£.
Jn+ Vn+ ./«+
Subtracting (2.12) from (2.11) we finally obtain that
f VE«
p
[|Vlogu|'
,
-
2
Vlogu- |Vlogu|
p
-
2
Vlogi;]
•
V£ <
[ [|V(^«)|
p
-|V
W
r
2
V«-V(^»]e. (2.13)
Next we note that
|V(V>n«)|
P
-|V«r
2
VM-V(fr)
= |^„V« + «V^„|
P
-p\^
n
Vu\"-
2
(^
n
Vu)
•
{uV^
n
) - |V>
n
Vu|
p
.
Therefore from Lemma 2.2 we infer that
(7pW
p
|VVn|
p
ifpe(i,2],
|V(^
n
u)|
p
- \Vu\"-
2
Vu
•
V(^» < I
{C(u"\ViP
n
\" + HVV„|)
2
|V«|
p
-
2
) if p > 2.
(2.14)
Assume first that p e
(1,2].
Using (2.13) and (2.14) we infer,
f VS«
P
[|Vlogu|
p
-
2
Vlogu-|Vlogu|
p
-
2
Vlogt;]
•
Vf
<cjr[
u
p
|V(/„(5
0
))|
p
+ CE I. w
W
«
P
|V(p
n
(|x|))|
p
i=i "
/n
(
„i->.rj->) j=i-
/
n
(
'
J
'
Pi
^ C-A /• /|u|y> C A f (\u\y
n
248
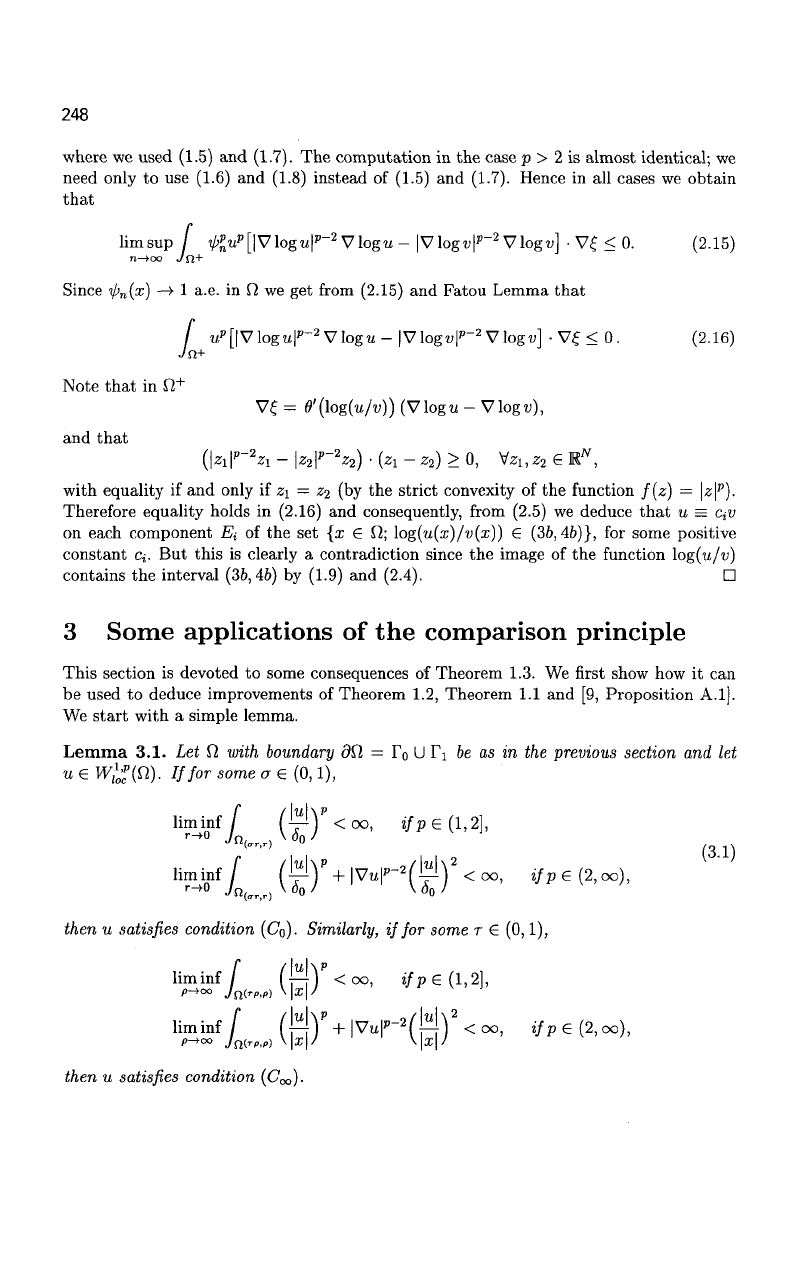
where we used (1.5) and (1.7). The computation in the case p
>
2 is almost identical; we
need only to use (1.6) and (1.8) instead
of
(1.5) and (1.7). Hence in all cases we obtain
that
limsup
/
ipy[\Vlogu\
p
-
2
Vlogu- |Vlogv|
p
-
2
Vlogu]
•
V£
<
0. (2.15)
Since ip
n
(x)
—¥
1 a.e. in Q we get from (2.15) and Fatou Lemma that
/ w
p
[|Vlog«|
p
~
2
Vlog«- |Vlog*;|
p
-
2
Vlogu]
•
Vf
<
0. (2.16)
Note that in 0+
V£
=
6'(\og(u/v)) (Vlogu- Vlogu),
and that
{\
Zl
\"-
2
Zl
-
\z
2
f~
2
z
2
)
•
(
2l
-
z
2
) > 0, Vzi,
z
2
eR
N
,
with equality
if
and only if z\ =
z
2
(by the strict convexity of the function
f(z) =
\z\
p
).
Therefore equality holds in (2.16) and consequently, from (2.5) we deduce that
u
=
CiV
on each component
Ei
of the set
{x €
fi;
\og(u(x)/v(x))
€
(36,46)},
for some positive
constant c;. But this is clearly
a
contradiction since the image of the function log(u/v)
contains the interval (36,46) by (1.9) and (2.4).
D
3 Some applications of the comparison principle
This section is devoted to some consequences of Theorem 1.3. We first show how
it
can
be used to deduce improvements of Theorem 1.2, Theorem 1.1 and [9, Proposition A.l].
We start with
a
simple lemma.
Lemma 3.1. Let
0
with boundary 90
= r
0
U 1^ be as
in
the previous section and let
u
e
W^(Q).
If for some
a e
(0,1),
liminf/
(^)
P
<oo,
j/p 6(1,2],
then
u
satisfies condition (Co). Similarly, if for some
r €
(0,1),
liminf/"
(My<oo,
if
pe
(1,2],
liminf/
(M)
P
+
|
Vur
2(M)
2
<00
, ifpe(2,oo),
l>^°° JiHrp,p)
\\X\J
\\X\J
then
u
satisfies condition (Coo).

249
Proof.
It is enough to prove the first assertion for p 6
(1,2];
all the other cases are similar.
By assumption we can find a sequence {r
n
}'^L
l
such that r
n
\ 0, r
n+1
< ar
n
, Vn and
Jn, ,
V
5
o I
Vn.
Clearly lim,,-^ ^ S?=i
a
n+j
—
0>
so
setting rj™ =
r„
+J
-
for all n > 1 and 1 < J < n we
see that (1.5) is satisfied. •
An immediate consequence is the following improvement of Theorem 1.1.
Corollary 3.1. Let a(x) G iJ
0C
(R
N
\ {0}), R > 0, Q
R
:= {|x| > R} and let u,v e
C(QR)
n W^f(flfl) be respectively a sub and super solutions for (1.3) on 0 = f2
R
. Assume
that v > 0 on QR, U satisfies condition (C^), and i/ta£
«(x) > u(x) on {\x\ = R}.
Then,
v > u in QR. In particular, the result
holds
for u which satisfies for some r G (0,1),
liminf [
0-PrY
<oo, if pe (1,2}
e^™ J{TP<\X\<P} M*K
liminf/
(M)
P
+
|v
ur
=(M)
2
<0O)
,/
p6
(2,oo)
Proof.
The assertions follow easily from Theorem 1.3 and Lemma 3.1 applied with
Q.
=
Q
R
, r
1 =
{|a;| =R} and T
0
= 0. D
In the next proposition we present some common situations in which condition (Co)
is satisfied.
Proposition 3.1. The function u satisfies condition (Co) if either of the following holds:
(i) T
0
is a compact Lipschitz hyper surf ace and for some 0 < r < dist(r
0
,Ti) we have:
u e W
lj,
(n
r
) with Q
r
:= {x G
O,
: S
0
(x) < r} and for some * G C°°(Q) such that $s0
on Q\
Q
T
/2
and f si on O
r
/3, we have \&u G
W
0
'
p
(il
r
).
(ii) To = {^o}
an
d for some 0 < r < dist(x
0
,Ti), and \P G Cf(B
T
(xo)) such that
"9
= 1
on
B
T
/2{x
0
),
we have ^u
G
W
0
'
p
(B
T
(x
0
)
\
{x
0
}).
Proof,
(i) Since Hardy inequality holds for domains with Lipschitz boundary (see [7]) we
have,
for some constant c > 0,
f |V(*u)|" > c f (|*u|A>r. (3.3)
It follows from (3.3) that
U/5Q
is an V function in a neighborhood of T
0
. In case p
G
(1,2]
this clearly implies (3.1) (in this case the limit in (3.1) is actually 0). In case p > 2, we
have by Holder inequality,
/ |V«r
2
(H/5
0
)
2
< ( f |Vu|')
¥
-(/" (\u\/5
0
)»)\
