Bemelmans J. et. al. (editor) Elliptic and Parabolic Problems: Rolduc and Gaeta 2001
Подождите немного. Документ загружается.


210
and
/j,
is to be suitably fixed. Keeping in mind (3.3) and (3.4), is easily verified that
L°
v
H(x,y,t) < (
1
+
£2
)(^ +
^)
+((ci+/jr)ci
_ »)^l + \(
x
,y)\
2
<-\f(x,y,t,v(x,y,t))\,
yjl +
\{x,y)\
2
if /i, i are suitably large. On the other hand, by (3.3),
H\
dp
q
T
> \g\. Therefore, by the
maximum principle, we infer that H ± u > 0 on QT, that is
<=i
\u\<H< 2
Cl
Vl + l(a:,y)|
2
, if T <
A*
Next we prove the estimate for the y-derivative of u. We differentiate equation (3.1) w.r.t.
the variable y and then multiply it by e~
2Xt
d
y
u. Denoting u = (e~
xt
d
y
u) , we obtain
L
E
v
uj
= e'
2Xt
Ll{d
y
u)
2
+ 2\u
= 2
(e-
2Af
(IV^^I
2
+ e\d
vy
uf + d
y
u {d
y
f + djd
y
v)) +
Xto)
> 2 (e~
2Xt
d
y
u {d
y
f + d
v
fd
y
v) +
XCJ)
. (3.6)
Hence, by setting w = a;
—
c
2
, we get from (3.6)
L%w
> 2V^ (- \d
y
f\ - \d
v
vdj\ + Av^)
(by (3.3), (3.5) and by the elementary inequality y^J > 2 (
Cl + s
S
n
(
u;
)\/M))
> V2uj (
Cl
(\ - 2V2c
t
- V2) + \sgn(w)y/\w\)
(for
A
= A(cj) suitable large)
> c^/uj\w\sgn(w), (3.7)
for some positive constant c = c(ci). By contradiction, we want to prove that w < 0 in
QT-
It will follow that
\d
y
u\
< c
x
e
xt
which implies (3.6) if T = T(ci) > 0 is sufficiently small. Let
ZQ
be the maximum of w on
Q
T
. If w(zo) > 0, then
ZQ
6 QT \
O
P
QT,
since by construction w < 0 on
8
P
QT-
This leads
to a contradiction since, by (3.7)
0 > L
c
v
w(z
0
) > cy/ui(z
0
)w(z
0
) > 0.
By a similar technique, we prove estimate (3.5) of the ^-derivatives of u. We set
ui = (e~
xt
d
Xk
u) , w =
u> —
c
2
.
Differentiating equation (3.1) w.r.t. x
k
and multiplying it by e~
2Xt
d
Xk
u, we get
L%w
= e-
2Xt
Ll(d
Xk
u)
2
+ 2Aw = 2 {e~
2Xt
d
Xk
u {d
x
J + d
Xk
vd
v
f) + Aw)
(by (3.3),(3.5), and the estimate of d
y
u just proved)
> V2u (ci fA -
A/2
-
2A/2CI
- 4\/2c
2
) + Asgn(iu)y/\w\)
(if
A
= A(ci) is suitable large)
> c^/uj\w\sgn(w),
for some positive constant c which depends only on c
x
. As before, we infer that w < 0
which yields (3.5). •

211
4 Regularity
Theorems
2.2 and 2.3
rely
on
some representation formulas
for u and its
derivatives
in
terms
of the
fundamental solution
of a
frozen operator.
The freezing method
is a
well-known technique, classically used
to
study
the
regularity
of solutions
to
linear parabolic equations.
In
this case,
the
associated frozen operator
is
simply obtained
by
evaluating
the
coefficients
at a
fixed point. This
new
operator
is, up
to
a
linear change
of
coordinates,
the
heat operator
and its
fundamental solution
can be
considered
as a
parametrix
of
the fundamental solution
of
the non-constant coefficients
op-
erator.
A
much more difficult argument was used
to
prove
the
existence
of a
fundamental
solution
for
Hdrmander type operators (2.3). Indeed
the
frozen vector fields
n
X
i>xo
=
^2Oij(xo)d
Xj
i = 0,
• • •
,p
i=i
commute,
and the
generated
Lie
algebra
has
dimension,
in
general, less than
n. In
this
case
the
operator
E*L-*o,*„
(4-1)
is
not
hypoelliptic,
and it has not a
fundamental solution. Folland
and
Stein
[14]
first
pointed
out
that
the
model operators
in
this case are operators
of
the form (2.3) such that
the
Lie
algebra generated
by
X
0
,...,
X
p
is
nilpotent
and
stratified. Later
on
Rothschild
and Stein introduced
in
[23]
an
abstract
and
very general version
of the
freezing method.
The choice
of the
frozen vector fields
X
i>xo
is
made
in
such
a way
that their generated
Lie algebra
has, at low
orders,
the
same structure
as
Lie(Xo,
...,X
P
).
With this choice
of vector fields,
the
operator
in (4.1) is
hypoelliptic
and its
fundamental solution
r
i0
is
a parametrix
for
(2.3).
As
said above,
we
employ here
a
modification
of
this technique,
introduced
by
Citti
in [6].
We define
a
frozen operator
in
terms
of the
notion
of
"intrinsic" Taylor expansion
of
the coefficients.
We
first consider
L as a
linearized operator
N-l
L
u
= 2^i Xj + Xo,
j=i
where
Xj = d
x
. and X
0
= ud
y
—
d
t
.
Then
we let, for
every zo
= (x
0
, y
0
, t
0
) e R
N+1
,
JV-l
.7
=
1
where Xo
>20
=
(u{z
0
)
+ (x
—
xo)
•
V
x
u(zo))d
v
—
d
t
.
Under
the
assumptions
of
Theorem
2.2,
this choice ensures that
the
frozen operator
L
zo
is a
nilpotent Hormander type operator,
it
has a
fundamental solution
r
zo
and an
associated control distance
d
zo
. For
simplicity
we assume that
/
does
not
depend
on u. We
represent
the
solution
u in
terms
of r
zo
:
«(«) =
/
r
zo
(2,
C)L
zo
u(OdC =
J
r
Z0
(z,
C)/(CR
+
J
r
zo
(z,
Q)K
ZO
{Z,
CR,

212
where
K
zo
(z,()
= (L
u
-
L
Z0
)u(Q
=
(u(C)
- u(z
0
) - (£ - x
0
)
•
V
x
u(zo))d
y
u{Q.
Since
L
u
is a
second order operator, we have
to
consider
the
term
X
0tZo
u
as a
second order
derivative, whereas
Xj is a
first order derivative.
As a
consequence,
the
first order Taylor
polynomial
of u,
with initial point
at z
0
, is
given
by
P
zo
u(z)
= u(z
0
) + (x- x
0
)
•
VZM(Z
0
).
Now,
by
(1.4),
d
y
u is
bounded
so,
when
u € C
1,a
, we
have
K
Z0
{z,0
=
O{d(z
0
,Cy
+a
)
as
d(«b,C)-+0.
By choosing
zo = z,
this estimate allows
us to
differentiate
up to 3
times
the
above
representation formula under
the
integral sign:
D
3
u(*o)
= J
Dr
zo
(z
0
,0-D
2
/(CR
+ /
D
3
r
zo
(z
0
, OK
zo
(z
0
,
CR
and conclude that
u 6 C
3
'
a
. A
rather delicate argument, based
on the use of
some high
order difference quotients allows
us to
iterate this argument
and
conclude
the
proof
of
Theorem
2.2.
The proof
of
Theorem 2.3 follows
the
same lines,
but
there
is
another difficulty. Indeed,
without
the
assumption
of the
Hormander condition (2.5),
the
frozen operator
L
zo
may
not have
a
fundamental solution.
In
this case,
it is
convenient
to
approximate
X
0
by the
vector field
Xo,z
0
=
{u(zo)
+ {x- x
0
)i) d
y
- d
t
,
where
x
x
denotes
the
first component
of the
vector
x.
Then
the
operator
N-l
Lzo
= 2^ Xj + X
0iZ0
does
not
depend
on
V
X
U(ZQ)
and it is
hypoelliptic. Note that, with respect
to X
0>zo
,
this
vector field gives
a
less close approximation
for Xo,
since
X
0
u{z)
-
X
0iZ0
u(z)
= (u{z) - u(z
0
) - (x -
x
0
)i)d
y
u{z)
=
O(d(z
0
,z)),
as
d(z
0
, z)
—>
0,
however
it is
sufficiently accurate
to
prove Theorem
2.2.
References
[1]
ANTONELLI,
F.;
BARUCCI,
E.;
MANCINO,
M.E., A
Comparison result
for
FBSDE with
Applica-
tions
to
Decisions
Theory.
Math.
Methods
Oper.
Res., in
press
(downloadble from
http://www.dm.unibo.it/~pascucci/web/Ricerca/Ricerca.html).
[2]
ANTONELLI,
F.;
PASCUCCI,
A.
On the
viscosity solutions
of
a
stochastic differential utility
problem.
J.
Differential
Equations,
in
press
(downloadble from
http://www.dm.unibo.it/~pascucci/web/Ricerca/Ricerca.html).

213
[3] BARLES,
G., A
weak Bernstein method
for
fully non-linear elliptic equations,
Diff. Int. Eq., 4-2,
(1991),
241-262
[4]
BIAN,
B.;
DONG,
G., The
regularity
of
viscosity solutions
for a
class
of
fully nonlinear equations,
Sci.
China,
Ser. A 34,
No.12, 1448-1457 (1991).
[5]
CABRE,
X.;
CAFFARELLI,
L. A.,
Fully nonlinear elliptic equations, Colloquium Publications.
American Mathematical Society.
43.
Providence,
RI
(1995).
[6] ClTTI,
G., C°°
regularity
of
solutions
of a
quasilinear equation related
to the
Levi operator,
Ann.
Sc.
Norm. Super. Pisa,
CI. Sci., IV. Ser. 23, No.3,
483-529 (1996).
[7] ClTTI,
G.;
PASCUCCI,
A.;
POLIDORO,
S., On the
regularity
of
solutions
to a
nonlinear ultra-
parabolic equation arising
in
mathematical finance,
Diff. Int. Eq. 14-6
701-738 (2001).
[8] ClTTI,
G.;
PASCUCCI,
A.;
POLIDORO,
S.
Regularity properties
of
viscosity solutions
of a non-
Hormander degenerate equation,
3.
Math. Pures Appl.
2001, 80 (9),
901-918 (2001).
[9]
CRANDALL,
M. G.;
ISHII,
H.;
LIONS,
P.-L.,User's guide
to
viscosity solutions
of
second order
partial differential equations, Bull.
Am.
Math.
Soc, New Ser. 27, No.l, 1-67
(1992).
10]
L.
EPSTEIN,
S.
ZIN, Substitution, risk aversion
and the
temporal behavior
of
consumption
and
asset returns:
a
theoretical framework, Econometrica,
57
(1989), 937-969.
[11]
ESCOBEDO,
M.;
VAZQUEZ,
J.L.;
ZUAZUA,
E.,
Entropy solutions
for
diffusion-convection equations
with partial diffusivity, Trans.
Am.
Math.
Soc. 343, No.2,
829-842 (1994).
[12]
W. H.
FLEMING,
H. M.
SONER,
Controlled Markov processes
and
viscosity solutions, Applications
of Mathematics,
25, 1993,
Springer-Verlag.
[13] FOLLAND,
G.B.,
Subelliptic estimates
and
function spaces
on
nilpotent
Lie
groups,
Ark. Mat. 13,
161-207 (1975).
[14] FOLLAND,
G.B.;
STEIN,
E.M.,
Estimates
for the 5(,
complex
and
analysis
on the
Heisenberg
group, Commun. Pure Appl. Math.
27,
429-522 (1974).
[15]
B.
FRANCHI,
E.
LANCONELLI, Holder regularity theorem
for a
class
of
linear non-uniformly elliptic
operators with measurable coefficients,
Ann. Sc.
Norm. Super. Pisa,
CI. Sci., IV. Ser. 10
(1983),
523-541.
[16] HORMANDER,
L.,
Hypoelliptic second order differential equations, Acta Math. 119,147-171 (1967).
[17] JERISON,
D.S.;
SANCHEZ-CALLE,
A.,
Estimates
for the
heat kernel
for a sum of
squares
of
vector
fields, Indiana Univ. Math.
J. 35,
835-854 (1986).
[18]
KRYLOV,
N.V.,
Holder continuity
and L
p
estimates
for
elliptic equations under general Horman-
der's condition, Topol. Methods Nonlinear Anal.
9, No.2,
249-258 (1997).
[19] MOSER,
J., On
Harnack's theorem
for
elliptic differential equations, Comm. Pure Appl. Math.
14,
(1961).
[20]
NAGEL,
A.;
STEIN,
E.M.;
WAINGER,
S.,
Balls
and
metrics defined
by
vector fields
I:
basic
properties, Acta Math.
155,
103-147 (1985).
[21]
PASCUCCI,
A.,
Hlder regularity
for a
Kolmogorov equation, preprint
[22]
PASCUCCI,
A.;
POLIDORO
S., On the
Cauchy problem
for a
nonlinear ultraparabolic equation,
preprint

214
[23] ROTHSCHILD, L.P.; STEIN, E.M., Hypoelliptic differential operators on nilpotent groups, Acta
Math. 137(1976), 247-320 (1977).
[24]
TRUDINGER,
N.S., Holder gradient estimates for fully nonlinear elliptic equations, Proc. R. Soc.
Edinb., Sect. A 108, No.1/2, 57-65 (1988).
[25]
WANG,
L., On the
regularity
theory
of
fully nonlinear
parabolic equations
I and II, Commun. Pure
Appl. Math. 45, No.l, 27-76 and No.2, 141-178 (1992).

Quasiconvexity and optimal design
Pablo Pedregal
ETSI Industrials
Universidad de Castilla-La Mancha
13071 Ciudad Real, Spain
Abstract
A typical optimal design problem in conductivity is examined where the cost functional is the
square-mean deviation of the gradient of the electric potential from a given vector field. Through a
convenient reformulation of the situation as a vector variational problem, we are able to provide a
complete analysis of relaxation including a fully explicit formula for the quasiconvexification of the
resulting integrand and associated optimal microstructures, both under no resource constraints
and under a typical volume constraint. In particular, this analysis opens the gate to the numerical
approximation of optimal structures via relaxation.
To David Kinderlehrer
1.
Introduction
We would like to report on some recent advances on the analysis of some typical optimal design
problems in conductivity where two different conducting materials with conductivities 0 < a < f3
are to fill out in given proportions a regular, simply-connected design domain Cl in R. so as to
achieve a desired goal. One of the new features is the explicit dependence of the objective
functional on derivatives of the underlying electric potential which is related to a given mixture
through the equilibrium equation
div ((ax(x) + 0(1 - x(i)))Vti(i)) = f{x) in O,
together with boundary condition
u =
Uo
on dil.
Here the design field X is a characteristic function of a subset of Q such that
/ x(x) dx =
A
\Q\,
Jn
and A £ (0,1) is given. The integral functional which we want to minimize is
I(X)= f IVu(x) -
F(x)\
2
dx,
Jn
215

216
where
F is a
given target field. Altogether,
the
optimal design problem
we
would like
to
treat
is:
Given
Q C R
2
,
simple-connected
and
regular,
0 < a < /3, A e
(0,1),
F e
L
2
(Q),
f
G
H-^il),
u
0
e H
1
^),
Minimize
I(x) = /
\Vu(x)
-
F(x)\
2
dx,
subject
to
X, a
characteristic function
of a
subset
ofQ, / x{
x
) dx = X \Q\
Jn
div ((a
X
{x)
+
/9(1
-
x(x))) Vu(a:))
= f(x) in SI,
u
=
UQ
on dQ.
Homogenization theory
(see for
instance
[3] or [19]) has
been
the
main tool
in
dealing with
such optimization problems both analytical
and
numerically when cost functionals
do not
depend
explicitly
on
derivatives
of u.
Such problems
are
rather well understood. Further extensions
of
the ideas
of
homogenization
to
tackle
the
dependence
on
derivatives
of u can be
found
in
[6], [10],
[18].
Here
we
would like
to
describe
an
alternative approach based
on a
reformulation
of the op-
timization problem
as a
purely vector variational problem
and see how far we can
reach
in the
analysis
of
such variational principle. This perspective
has
already been explored
in
[16].
As
it
turns
out, the
underlying variational problem
is
non-convex, which
has
been known
since
the
pioneering work [11],
and
therefore
its
analysis proceeds
by
examining relaxation ([4]).
Under
the
volume constraint, this relaxed problem involves
a
convex hull that must take into
account such integral restriction
and
thus ought
to
depend
on a new
scalar variable
in
addition
to
the
usual dependence
on
gradients. What
is
really remarkable
is
that
the
explicit form
of
this
relaxed integrand
can be
computed explicitly
and
given
in
closed form, thus opening
the
gate
to
numerical approximation
of
optimal microstructures
via
relaxation.
This contribution
is
organized
as
follows. Section
2
describes
the
reformulation
of
the problem
as
a
constrained, vector variational problem with some interesting features. Sections
3 and 4
treat
the same optimal design problem without
the
resource constraint
[
X
(x)dx =
\p\.
Section
5
contains some ideas
and
statements
on the
relaxation
of
this non-convex variational
problem under
the
above volume constraint
as
well
as
explicit formulae
for the
appropriate con-
strained convex-hull. Some final remarks
are
included
in
Section
6.
2.
Reformulation
An initial observation relevant
to
simplify
the
computations that will follow
is
that we
can
assume
without loss
of
generality that both
F and / are
identically zero.
The
reason
for
taking
F = 0 is
|V«(x)
-
F(x)\
2
=
\Vu(x)\
2
-
2V«(x)
•
F(x) +
\F{x)\
2
,

217
and only the first term (corresponding toFsO) is non-linear. The reason for taking / = 0 is that
the general case involves a traslation by an auxiliary known function, and thus the computations
are easily derived in the general case from those for / = 0. We thus take from now on F and /
vanishing identically, so that the functional to be minimized is
I(X) = [ |Vu(.
x) I dx,
and the equilibrium equation is
div {{a
X
(x) + P(l - x{x)))Vu(x)) = 0 in O.
The reformulation of our problem starts with the realization that this equilbrium equation
is equivalent (under the assumption of simple-connectedness of
Q.)
to the existence of an stream
function v such that
{a
X
{x) + /3{1 -
X
{x)))Vu{x) + TVv{x) = 0,
where T is the 7r/2, counter-clockwise rotation in the plane. Hence we can alternatively use as
design variables the pair of vector fields («, v) satisfying the additional, important, pointwise
restriction
aVu(x) + TVv(x) = 0 or /3Vu{x) + TVv(x) = 0, (2.1)
for a. e. x € Ci. Notice how we can go from an admissible x to
sucn a
P
3
^ (
u
i ")>
an(
i conversely,
from such a pair (u, v) verifying (2.1) to an admissible X- Om- aim now is to rewrite the optimal
design problem in terms of these pairs (u, v) instead of X-
To this end, collect both u and v in a single vector variable U = (L^
1
', LA
2
') (u is identified
with the first component [/t
1
) while v is identified with £/"'). Define the two densities
by putting
W, V : M
2X2
-> R* = RU {+00} ,
W{A) = \\
AW
\
2
'
XAeAaUAf,^
[.
+oo, else,
V(A) = [
l
<
if
/
6A
«',
(^ +oo, else,
A
7
= JA € M
2x2
: 7AW + TA^ = o|, 7 = a or 0.
Then it is elementary to convince ourselves that the original optimal design problem is equivalent
to the vector variational problem
Minimize J{U) = f W{VU(x)) dx
Jn
UeH
1
^),
U^=u
0
on 90, I V{VV{x))dx = X.

218
The main advantage of this formulation is two-fold. On the one hand, we somehow overcome
the non-local effect associated with the equilibrium equation to the expense of treating a vector
variational problem. But on the other, as such typical vector variational problem, we can utilize
all the accumulated experience with non-convex variational problems. In particular, all ideas
and techniques about relaxation, gradient Young measures, microstructure, etc. But there are
also some new ingredients. We have an additional integral constraint coming from the initial
volume constraint which is important and relevant. Moreover, both integrands W and V are not
Caratheodory functions as they take on the value +00 suddenly. In these notes, we will ignore
this last difficulty as it is, we believe, essentially technical, and focus on how to deal with the first
integral restriction.
3.
Relaxation without volume constraint
In this and the next sections we explore our original optimal design problem, but drop the volume
constraint. The reason is that if this integral restriction is not taken into account our reformulation
of the problem falls into the typical scenario of a vector variational problem.
It is interesting to point out that when some very peculiar adjustment between F, f and a
or /3, takes place, then our optimal design problem admits a unique optimal solution. Indeed, if
/ = a div F or / =
f3
div F the variational problem
Minimize / IVufx) -
F(x)\
2
dx
[
\Vu{x)
-
F(x
subject to u
—
u
0
G
HQ(Q),
admits a unique optimal solution which is a weak solution of the
associated Euler-Lagrange equation
div (Vu) = divF = 7"
1
/, in &,
for 7 equal to a or fl. Therefore our optimal solution, when that close relationship between F
and / occurs, is the solution of the Poisson's equation
jAu = f in Q, U — U
0
€HQ(£Y).
Notice that this choice corresponds to taking x constant to 1 or 0, depending on whether 7 = a
or 7 = /3, throughout fi. When such adjustment between F and / does not happen, then our
analysis becomes significant.
Our main result is the explicit computation of QW and the identification of optimal mi-
crostructures.
Theorem 3.1 Put
g(A) =a
2
p
2
\AW\
4
+ |yl<
2
>|
4
+ (a
2
+ 6a/3 + /3
2
) detA
2
II2II2
II2 II2
-2a/3UW Ll
(2)
-2aj3(a +
P)\A^\
det A - 2(a +/3) \A^\
detA,
h(A)={a + 0)detA-ap\AW\ - U
(2)
l ,
219
and consider the set of matrices
r = {A
G
M
2x2
: h{A) >
0,g(A)
> 0}
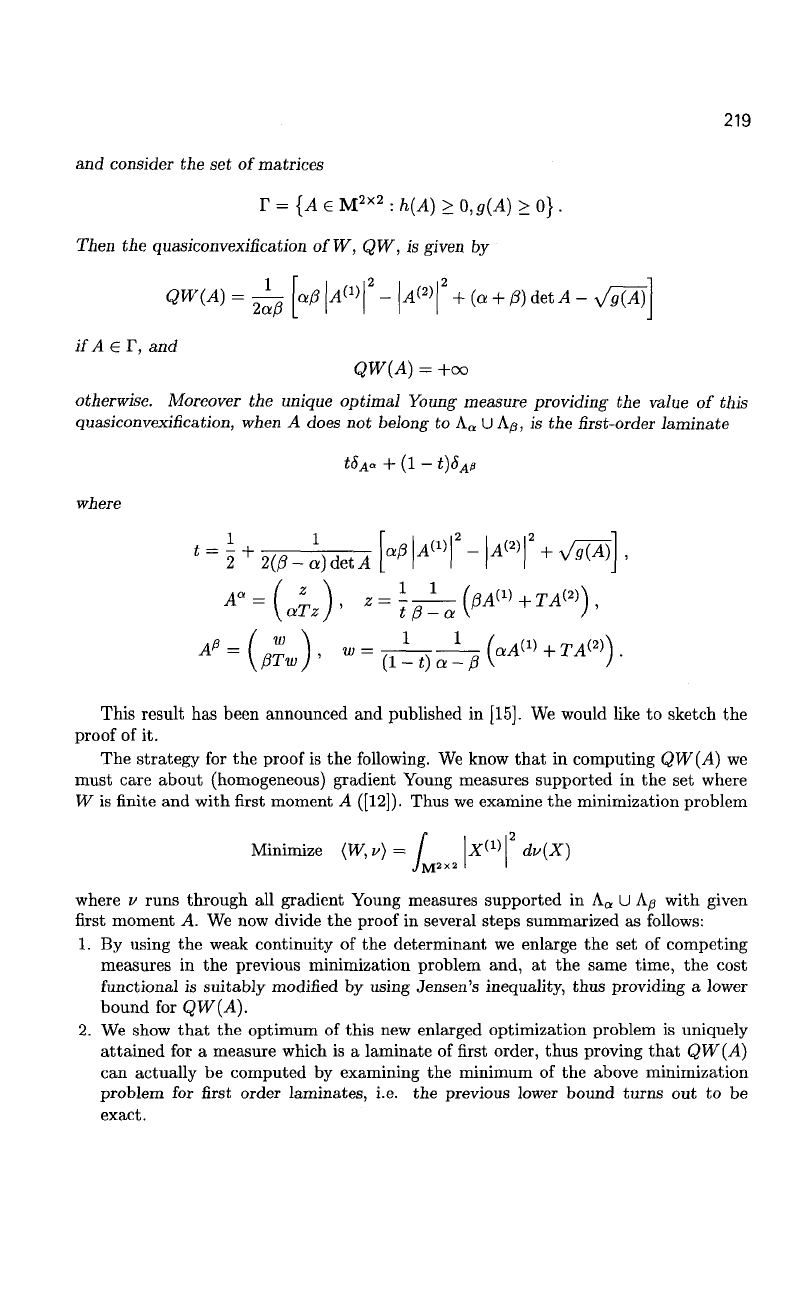
Then the quasiconvexification ofW, QW, is given by
1
QW
^ 2.P
a,flU«| -U
(2)
| +{a
+
/3)detA-y/^(A)
if A e T, and
QW{A) = +oo
otherwise. Moreover the unique optimal Young measure providing the value of this
quasiconvexification, when A does not belong to A
a
UA^, is the first-order laminate
tS
Aa
+
(1
-
t)8
A
e
where
1
t = x +
/aUwf-Urof + VffCA)
2 2(/3-a)detA
This result has been announced and published in [15]. We would like to sketch the
proof of it.
The strategy for the proof is the following. We know that in computing QW (A) we
must care about (homogeneous) gradient Young measures supported in the set where
W is finite and with first moment A ([12]). Thus we examine the minimization problem
Minimize (W» = /" \x
w
\ dv{X)
where v runs through all gradient Young measures supported in A
a
U A^ with given
first moment A. We now divide the proof in several steps summarized as follows:
1.
By using the weak continuity of the determinant we enlarge the set of competing
measures in the previous minimization problem and, at the same time, the cost
functional is suitably modified by using Jensen's inequality, thus providing a lower
bound for QW{A).
2.
We show that the optimum of this new enlarged optimization problem is uniquely
attained for a measure which is a laminate of first order, thus proving that QW(A)
can actually be computed by examining the minimum of the above minimization
problem for first order laminates, i.e. the previous lower bound turns out to be
exact.
