Bemelmans J. et. al. (editor) Elliptic and Parabolic Problems: Rolduc and Gaeta 2001
Подождите немного. Документ загружается.


X
Hyperbolic propagation of singularities in a parabolic system of
shell theory 451
J. Sanchez-Hubert
Singular perturbations going out of the energy space. Layers in
elliptic and parabolic cases 461
E. Sanchez-Palencia
Regularity and uniqueness results for a phase change problem in
binary alloys 475
J.-F. Scheid and G. Schimperna
Bifurcation in population dynamics 485
K. Umezu
Models for shape memory alloys described by
subdifferentials of indicator functions*
Toyohiko Aiki
1
and Nobuyuki Kenmochi
2
1
Department of Mathematics, Faculty of Education
Gifu University
Gifu 501-1193, Japan
2
Department of Mathematics, Faculty of Education Chiba University
1-33 Yayoi-cho, Inage-ku, Chiba, 263-8522 Japan
Email : aiki@cc.gifu-u.ac.jp ; kenmochi@math.e.chiba-u.ac.jp
Abstract
We consider
a
system of nonlinear partial differential equations, which
is
one
mathematical model describing the dynamics of shape memory alloys. We prove
the existence and uniqueness
of a
solution of this one-dimensional model, using
the theory of nonlinear evolution equations governed by subdifferential operators
of time-dependent convex functions on Hilbert spaces, and applying some classical
results dealing with /^-estimates for solutions of parabolic equations.
1 Introduction
The shape memory alloy problem under consideration is to find
a
triplet of functions, the
temperature field 6 := 8(t, x), the stress
a
:— a(t, x) and the displacement
u
:= u(t, x) on
Q(T) := (0,T)
x
(0,1),
0 < T <
oo, satisfying
u
tt
+
^u
xxxx
-
/iu
xxt
- a
x
=
0 in Q(T),
(1)
0
t
-
K-0
XX
=
au
xt
+ jx\u
xt
\
2
in Q(T),
(2)
o-t
-
v(T
xx
+ dl(8, e; a)
3
cu
xt
in Q(T),
(3)
u{t,0)=u(t,l)=u
xx
{t,0)=u
xx
{t,l)
=
0
for0<t<T,
(4)
0
x
{t, 0)
=
0
x
{t, 1)
=
0 for 0
< t
< T,
(5)
a
x
(t, 0)
=
a
x
(i, 1)
=
0 for 0
< t
< T,
(6)
u(0)=u
0
,u
t
{0)
=
v
0
,8(0)=8
0
,a(0)
= a
0
on (0,1),
(7)
*This work
is
partially supported
by
a
grant
of JSPS.
1
2
where e is the shear strain, which is supposed to be defined as e = u
x
, 7,
/J.,
K, V and c
are positive constants, 1(9,
e;
a) is the indicator function of the interval
[/<,(#,
e),f
d
(9, e)},
where f
a
and fd are given functions on K x K, that is :
1(0,e;i
0 Hfa(e,e)<a<f
d
(e,e),
+00 otherwise,
dl(9,e\(r) is its subdifferential with respect to a,
ILQ,
VQ,
OQ
and
<JQ
are given initial
functions.
The above system (l)-(7) is referred to as (P) := (P)(uo,i>o,#o,cro)-
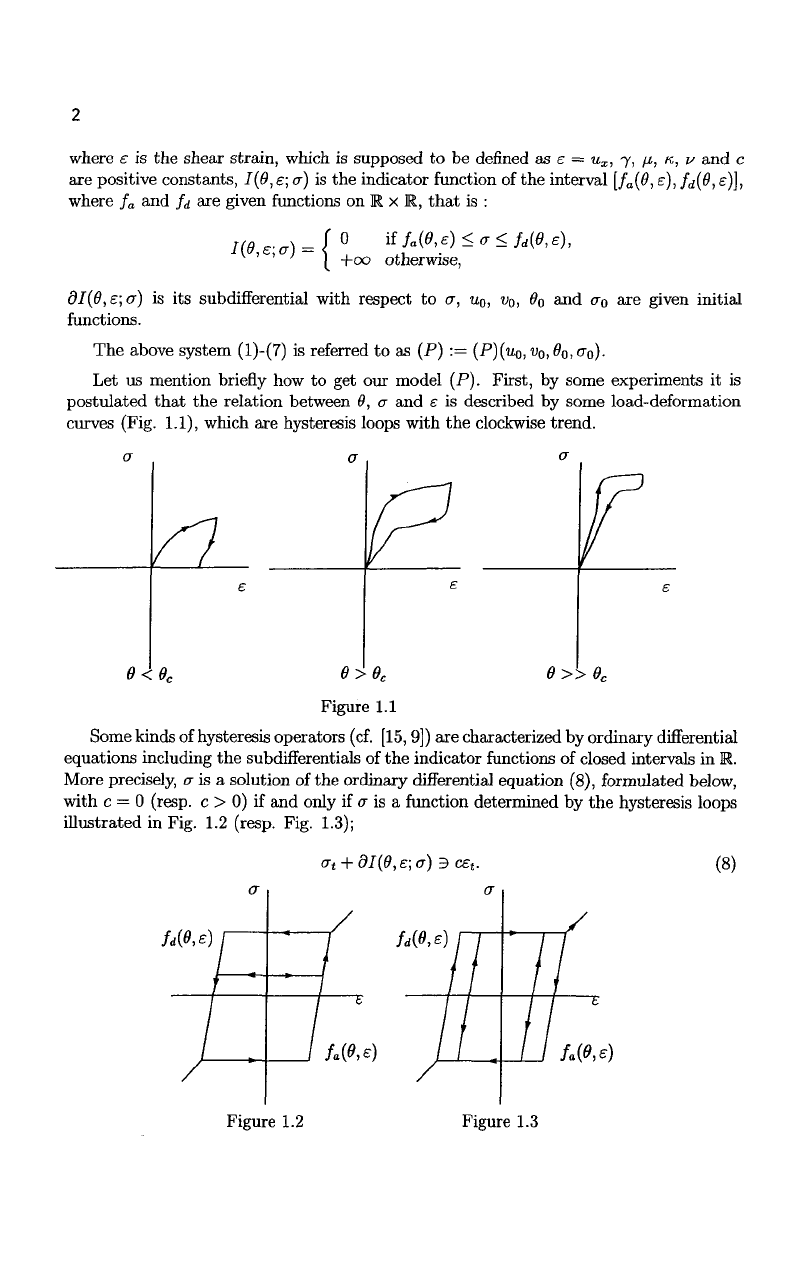
Let us mention briefly how to get our model (P). First, by some experiments it is
postulated that the relation between 9, a and e is described by some load-deformation
curves (Fig. 1.1), which are hysteresis loops with the clockwise trend.
6<0,
9>6
C
Figure 1.1
e»e,
Some kinds of hysteresis operators (cf.
[15,
9]) are characterized by ordinary differential
equations including the subdifferentials of the indicator functions of closed intervals in K.
More precisely, a is a solution of the ordinary differential equation (8), formulated below,
with c = 0 (resp. c > 0) if and only if a is a function determined by the hysteresis loops
illustrated in Fig. 1.2 (resp. Fig. 1.3);
a
t
+ dl(0,
e;
a) 3 ce
t
.
(8)
W,e)
fd(0,e
fa(0,e)
fa(0,e)
Figure 1.2 Figure 1.3

3
We note that the constant c corresponds to the slope of the inside hysteresis loops.
The characterization of hysteresis operators with clockwise trend was discussed by Krejci
[10],
assuming that I is independent of e. In order to get the spatial regularity of a, we
modify (3) by a small viscosity and consider (8) in our model.
Next, the Hamiltonian principle leads to the following equation (see [4, Chapter 5])
utt - &
x
+
^u
xxxx
= 0,
assuming that e = u
x
. Also, according to Falk [5] we have, as the balance law of internal
energy
U
t
+ q
x
- ae
t
- fie
xt
= 0, (9)
where U is the internal energy and q is the heat flux. Here, we use the classical Fourier
law, that is, q =
—K6
X
,
and neglect the last term in the left side of (9). Therefore, from
an elementary approximation U
t
= 8
t
and (9), it follows that
9
t
-K6
xx
=--au
xt
, in Q(T). (10)
Moreover, we add appropriate viscosity terms to the above equations to get u and 9
sufficiently smooth. Thus equations (1) and (2) are obtained. Such an approximation by
means of viscosity terms was studied by Hoffmann and Zochowski [7] and Sprekels, Zheng
and Zhu [14]. In our previous work [2], we discussed the system (l)-(7) with (2) replaced
by (9).
From the physical point of view, (2) is more natural than (10). But, for some mathe-
matical simplicity, we proved in [2] the existence and uniqueness result only for the system
with (10). We remark here that when fi
2
>
A-y,
the decomposition method for fourth-order
partial differential equations is used to get some ZAestimates for functions u satisfying
(1) just as in Pawlow and Zochowski [12].
This kind of models for shape memory alloys originates from the so-called Falk's model
and a lot of one-dimensional models have been discussed, for instance in [13, 4, 1]. In
particular, Pawlow and Zochowski recently established an existence and uniqueness result
for a three-dimensional model [12].
2 Main results
We begin with the precise assumptions for the data.
(Al) /„ and f
d
belong to C
2
(R) n H-^R) and /„ < f
d
on R. We define
L = max{|/
0
|iv2.~(ii), \fd\w^°°(R)}-
(A2) u
0
belongs to H
4
(0,1) with u
0
(0) = uo(l) = u
0xx
(0) = u
0xx
(l) = 0. v
0
belongs to
#(J(0,l)nff
2
(0,l), 9
0
G ^(0,1) and do belongs to ^(0,1). Moreover,
f
a
{9
0
,e
0
)
<
"o < fd(9o,£o) on (0,1), where e
0
= u
0x
.

4
J
(^) = {+CC
othe:
In order to apply the abstract theory of evolution equations in a Hilbert space, we
recall the definitions of indicator functions on L
2
(0,1) and of their subdifferentials. Given
1
G L
2
(0,1) and e G L
2
(0,1) we define a function 1(8,
e;
•) on L
2
(0,1) by:
eK(8,e),
otherwise,
where #(0, e) = {<r
G
L
2
(0,1) |
/„(<?,
e) < a < f
d
(6, e) a.e. on
(0,1)}.
Clearly, 1(8, e; •)
is proper, l.s.c. and convex on L
2
(0,1), £>(/(#,£;•)) = K(9,e), and its subdifferential
9/(0, e; •) is a multivalued operator in I/
2
(0,1) defined by the following way: £ € 9/(0, e; a)
if and only if a G L
2
(0,1) with
f
a
(9,e)
< a <
f
d
(8,e)
a.e. on (0,1) and \ e L
2
(0,1) such
that
[ i(z - a)dx < 0, for any z
G
if
(6,
e). (11)
Jo
We refer to the book of Brezis [3] (or [8]) for the definitions and basic properties of
subdifferentials operators. This variational inequality is equivalent to
i(x) < 0 for a.e. x G (0,1), with a(x) =
f
d
(0,e),
£(x) = 0 for a.e. x G (0,1), with f
a
(8, e) < a(x) < f
d
(6, e),
i(x) > 0 for a.e. x e (0,1), with a(x) =
f
a
(0,e).
Using the above notation, we formulate (P) as follows:
Definition 2.1 We call that a triplet {u, 9, a} of functions u, 6 and a on Q(T) is a
solution of (P) on [0,T], if the following conditions hold :
(51) u G L°°(0, T; tf
4
(0,1)) n
W
1
'
2
^,
T; H
3
(0,1)) n
W
l
-°°(0,
T; H
2
(0,1)).
(52) eeW
l
'
2
(Q,T;L
2
(Q,\))^L'
x
(Q,T;H
1
(Q,l)).
(53) crG
W^^T-L
2
^,!))
(M^^T-H
1
^,!)).
(54) (2) and (3) hold for a.e. (t,x) G Q(T), and there exists £ G L
2
(Q(T)) such that
\(t) 6 dI(8(t),e(t);o-(t)) for a.e. t G [0,7] and
a
t
(t) - v(j
xx
(t) + £(t) = cu
xt
(t) in L
2
(0,1) and for a.e. t G
[0,T],
(12)
and moreover (5)-(7)
hold.
Our main result is stated as follows.
Theorem 2.2 Assume that the above conditions (SI) and (S2)
hold,
and y? >
A"/.
Then,
there exists one and only one solution {u,8,a} of (P)(u
0
,v
0
,8o,ao) on
[0,7].
The proof of the uniqueness is quite similar to that of [2, Theorem 2.1]. Therefore we
omit it in this paper. In the next section, we prove a key lemma giving an lAestimate
for the solutions of (1), under the assumption fi
2
> i"f. This lemma gives the uniform
estimates for approximate solutions. At the end of this paper, we show the proof of the
existence of a solution.
In our existence
proof,
we will use the following approximation of the indicator func-
tion.

5
Lemma 2.3 (cf. [9, Section 4]) For every
A
>
0, let Ix(8,s;o~) be the Yosida approxima-
tion of 1(6,e;a) and I\(6,e;cr) be its subdifferential
:
h(e,e;a)
=
^{\W-f^er\l
2{0tl)
+ \[f
a
(e,s)-a}+\l
H01)
},
dl
x
(e,s;a)
= ^
{[a-/„((?,£)]+-[/.(<?,e)-«T]
+
},
for 6,
s, a £
L
2
(0,1). Our approximate problem
(P)\
with parameter
A
> 0
takes the
form:
m + ju
xxxx
-
/iu
xxt
-cr
x
= 0 in Q(T),
(13)
d
t
-
K9
XX
= au
xt
+
ii\u
xt
\
2
in Q(T),
(14)
<T
t
-
va
xx
+ dl\(6,
e;
a)
=
cu
xt
in
Q(T),
(15)
u(t,0) =u(t,l)
=
0 andu
xx
(t,0) =u
xx
(t,l)
=
0 for0<t<T,
(16)
e
x
{t,0) = 9
x
(t,l) = 0 forO<t<T,
(17)
a
x
{t,0)=a
x
{t,l)=0 forO<t<T,
(18)
u(0) = u
0
,u
t
(0) = v
0
,9{0) = e
0
,a(0) =
a
0
on
[0,1].
(19)
3 //-estimates
The purpose of this section is
to
establish the LP-estimate for solutions
of
(1), using the
classical theory for parabolic equations when
p > 1.
Lemma 3.1 Let
T > 0, 7
and /x be positive constants,
a 6
L
2
(0,T; H
2
(0,1)),
UQ
£
ff
4
(0,1) with «o(0)
=
uo(l)
=
"0^(0)
=
'Uozz(l)
=
0 and v
0
G #o(
0
> 1)
n
#
2
(0, !)• ™en,
ifeere eiisfa
u £
L°°(0, T; fl"
4
(0,1))
n
W
1,2
(0, T; #
3
(0,1))
n
W
1
'
00
^, T; #
2
(0,1)) satisfying
(1),
(4)
and u(0)
= «o
o«d «t(0)
= v
Q
on
(0,1). Moreover,
if
p,
2
>
A'y,
p > 1 and
<J
€
LP^QiT)), then there exists a positive constant
K
p
depending only on
/J,,
7, p
and
T
such that
\lHx\LP(Q(T)) < Kp{\°~\LP(Q(T)) +
|«0*X|W2-2/P,P(0,1)
+ \
V
o\w
2
-Vr,r(o,l))- (
2
0)
This kind of estimates was already obtained for three-dimensional problems in Pawlow
and Zochowski [12]. They decomposed (1) into two parabolic equations. Here, we give
a proof of Lemma 3.1
in a
similar way. First, we recall the classical result for parabolic
equations.
Lemma 3.2
(cf.
[6, Lemma 2.1] and [11, Chapter 2, Section 9]) Let
T > 0, a > 0,
p
> 1, f €
L
2
(0,T-H\0,1)) andw
0
e
W
2
-
2
^"(0,1)
with w
0
(0)
= w
0
(l) = 0.
Then,
there exists w
e
W
1
'
2
^,
T; L
2
(0,1))
n
L°°(0, T;
fl^O,
1)) satisfying
w
t
-
aw
xx
= f
x
in
Q{T),
w{t,0) = w(t,l) = 0 forO<t<T,
tu(0,
x)
=
WQ(X) for 0
< x
< 1.

6
Moreover,
if
f 6
^{QiT)), there exists
a
positive constant
K\
depending only
on
T,
a and
p
such that
\W
X
\LP(Q(T))
<
Kl{\f\Lr>(Q(T))
+
\™o\wi-Vr,r(0,l))-
Proof
of
Lemma 3.1.
The
existence
of
a
solution
u
is
quite standard.
It
is
sufficient
to
show (20).
Let
p
> 1. By the
assumption
p
2
> 47,
there exist positive numbers
Ai and
A2 satisfying
Aj
+
A
2
= n
and
A1A2
=
7
with
\i
>
A
2
. Now,
put
u
t
—
Xiu
xx
=
z
on Q(T).
Then,
it
holds that
z e
W
1
'
2
^,
T; L
2
(0,1))
l~l
L°°(0, T; H^O,1))
and
z
t
-
X
2
z
xx
=
a
x
in Q(T),
z{t, 0)
=
z(t,
1)
=
0
for 0 <
t
<
T,
z(0,
x)
=
v
0
(x)
-
\2Uo
xx
{x)
for 0
< x
< 1.
Hence, Lemma
3.2
implies that
\Z
X
\LP(Q(T))
< KJiiWIwwr))
+
\
v
o\w
2
-VP'P(o,i)
+
I
U
OIXIW2-
2
/P.P(O,I))'
where
K\
is a
positive constant.
Next,
we put
u
t
= y
and A
0
=
\i
-
^-.
Clearly,
A
0
>
0 and we
have
Vt
-
X
0
y
xx
=
{T-Z
X
+ a)
x
in Q(T),
Ai
y(t, 0)
= y(t,
1)
=
0 for 0 <
t
<
T,
2/(0, a:)
= v
0
(x) for 0 <
x
< 1.
Therefore,
by
Lemma
3.2
again,
it
follows that
7
\y
X
\Lr(Q(T))
<
Kl(\o-\
Lr{
Q(
T))
+
—
|Z
X
|LP(Q(T))
+
l«o|lV
2
-
2
/p,P(o,l)),
where A"j
is a
positive constant. Thus
we
have proved Lemma 3.1.
•
4 Existence
of
a
solution
In this section,
we
give
a
short proof
of
the existence
of a
solution
to (P).
This proof
is
a modification
of
that
of
[2, Theorem 2.1].
Proof
of the
existence
of a
solution.
(1st step.)
For
each
A
>
0,
there exist
T\
> 0 and a
unique solution {u\,8\,a\}
of (P)\
on
[0,T
A
].
Banach's fixed point theorem proves this step
in an
easy way.
(2nd step.)
Let
T
> 0 and T\ =
min{T,
T\}.
Then, there exists
a
positive constant
M\
depending only
on T, L, 7, fi, c,
|uo|ff2(o,i), |«o|x,=(o,i)
and
ko|z,2(o,i) such that
\Mt)\h(
0
,i)
+
\^
xx
(t)\h
m
<M
1
fort6(0,f
A
]andAe(0,l],
(21)
\^r
X
{T)\
2
mA
dT
< Mi for
A
€
(0,1],
(22)
I
0
2
°x(t)\h
m
< M
x
for
t 6
(0,T
A
]
and
A
e
(0,1].
(23)

Indeed, multiply (13) by u\
t
(t) and (15) by [<r
A
(i) - L\
+
and
[L
-
<r
A
(t)]
+
,
respectively,
and integrate these resultants over [0,1] for a.e. t €
[0,T\].
Then, these three equations
lead to the estimates (21)-(23).
(3rd step) There exists a positive constant Mi independent of
A
e (0,1] such that
Wx(t,x)\<M
2
for (i,x) e Q(f
A
) and
A
e
(0,1].
We can get this estimate in a similar way to that of [11, Theorem 7.1, Chapter 3] (cf.
[2,
Lemma 4.3]).
(4th step) For p > 1, there exists a positive constant M
P
3 such that
/ * / Wt^dxdt < M
p3
for
A
e
(0,1].
Jo Jo
This is a direct consequence of Lemma 3.1.
(5th step) There exists a positive constant M
4
such that
l^lwi.
2
(o,f
A;
z,
2
(o,i)) + l^lL~{o,f
A;
wi(o,D) -
M/t for A e
t
0
'
1
]-
Taking p = 4 in the 4th step and multiplying (14) by 9
t
(t), we get the above estimate.
(6th step) There exists a positive constant M
5
such that
\
a
^\L\Q(f
x
)) ^
M
s for
x
£ (°>
!]•
Multiplying (15) by <r
A
—
fd{6\,e\) yields the above estimate.
(7th step) There exists a positive constant M
6
such that
!"«.(*)
li»<o,i)
+ l«**»(*)li>(o,i) ^
M
s for 0 < t < f
x
and
A
e
(0,1],
/
Jo
\uxtxx{t)\
2
L
2
{0A)
dt < M
6
for
A
€
(0,1].
We derive the above estimates multiplying (13) by u
Atex
(£)-
(8th step) There exists a positive constant M-j such that
M*)IHI(O,I)
-
Mf
< for 0 < t < f
A
and
A
€
(0,1],
A
/ kxtW||
2(0il)
dt < M
7
for
A
e
(0,1],
Jo
f
X
\dlx(9x(t),ex(t);ax(t))\h
m
dt < M
7
for
A
e
(0,1],
Jo

We multiply (15) by cr
At
(f) and integrate over (0,1). Then we have
\\
a
^\
2
LHo,i) + 1j
t
j
o
\
ax
*W
2dx
+
J
t
J
0
h(0x(t),£x(t);ax(t))dx
< \j \ux
x
t(t)\
2
dx + ±J \Wx{t),ex(t))
t
\\[f
a
{ex{t),ex{t))-*x{t)}
+
\dx
+\f \USx(t),ex(t))
t
\\[ax(t) - f
d
(e
x
(t),ex(t))}
+
\dx
c
2
r
1
< Y/ \
u
x
x
t{t)\
2
dx
(24)
+ f\\fa(9x(t)Mt))t\ + \Wx(t),£x(t))t\)\dh(ex(t),ex(ty,ax(t))\dx.
Jo
Next, multiply (15) by dlx(dx(t),sx(t);crx(t)) and integrate over (0,1). We observe
that
J ax
t
(t)dIx(Ox(t),ex(t);<Tx(t))dx + ^J 15/^(0, £*(*);**(*))
f
Jo
dx
[°x{t)-f
d
{9x{t)Mtm
[f
a
{6x{t),ex{t)) - ax{t)U
C
;j\uUt)?dx
+
.[^^MMMW
dx
fd(0x(t),sx(t))
xx
dx (25)
Jo
1
[A(*A(t),eA(i))-"A(t)]
+
fa(8x(t),£x(t))
X
xdx.
Thanks to Lemma 2.3, it follows from (25) that
jj h{dx{t),ex{t)-ax{t))dx+-
A
J \dIx{ex{t),Ex{t);a
x
{t))\
2
dx
+XK
f \dh(ex(t),ex(t);cTx(t))
x
\
2
dx
Jo
•f
Jo
< AK
2
(\f
a
(ex(t),ex(t))
xx
\
2
+ \f
d
(9x(t),£x(t))
xx
\
2
)dx
(26)
+4
J\\fa(ex(t),ex(t))t\
2
+
\f
d
{ex{t)Mt))t\
2
)dx+
c
^f
\ux
t
,
{t)\
2
dx.
Hence, (24) and (26) with the assumption, fJfia,£o) < cr
0
<
f
d
(9o,£o)
a.e. on (0,1),
imply the assertion of the 8th step. The proof of this step is quite similar to that of [9,
Lemma 4.2].

9
(9th step) For each A e
(0,1],
(P)
x
admits a unique solution on
[0,T].
By the above
uniform estimates, we can extend the solution beyond f
x
if f
x
< T, where [0,7\) is a
maximal existence interval of a solution to (P)
x
. Accordingly, we observe that (P)
x
has
a unique solution on
[0,T].
(10th step) Let A e
(0,1],
T > 0 and {u
x
,6
x
,a
x
} be a solution of (P)
A
on [0,T] ob-
tained in the 9th step. The above uniform estimates with respect to A imply that there
exist a subsequence {A.,} of {A}, with {A
3
} -* 0, and functions u, 0, a and £ on Q(T),
such that u
Xj
-• w weakly* in L°°(0,T; ff
4
(0,1)), weakly in W^O.T; ff
3
(0,1)) and
weakly* in ^^(O.T; #
2
(0,1)), fl
A
. -+ 0 weakly in W^O.T; L
2
(0,1)) and weakly* in
L°°{0,T;
^{0,1)), a
Xj
-> a weakly in W/^TjL^O, 1)), weakly* in L°°(0,T; H\0,1)),
and dI(6
Xj
,£
Xj
;<r
Xj
) -+ £ weakly in L
2
(Q(T)) as j -> oo.
It is easy to see that {u, 9,a} is a solution of (P)(uo, ^o,^o,
o"o)
on
[0,T].
We refer to
[2,
section5] for the detailed proof of this step. •
References
[1] T. Aiki, Weak solutions for Falk's model of shape memory alloys. Math. Methods
Appl. Sci., 23 (2000), 299-319.
[2] T. Aiki and N. Kenmochi, Some models for shape memory alloys. To appear in
Gakuto, International Series Mathematical Sciences and Applications. Vol.17 (2001),
Mathematical Aspects of Modeling Structure Formation Phenomena.
[3] H. Brezis, Operateurs maximaux monotones et semi-groupes de contractions dans les
espaces de Hilbert. North-Holland, Amesterdam, 1973.
[4] M. Brokate and J. Sprekels, Hysteresis and Phase Transitions. Appl. Math. Sci., 121,
Springer, 1996.
[5] F. Falk, Elastic phase transitions and nonconvex energy functions, Free Boundary
Problems: Theory and Applications. I, 45-59, Pitman Research Notes in Mathematics
Series, Vol. 158, Harlow, Longman, 1900.
[6] A. Friedman and J. NeCas, Systems of nonlinear wave equations with nonlinear vis-
cosity. Pacific J. Maths., 135 (1985), 29-55.
[7] K.-H. Hoffmann and A. Zochowski, Existence of solutions to some non-linear ther-
moelastic systems with viscosity. Math. Mech. Appl. Sci., 15(1992), 187-204.
[8] N. Kenmochi, Solvability of nonlinear evolution equations with time-dependent con-
straints and applications. Bull. Fac. Education, ChibaUniv., 30 (1981), 1-87.
[9] N. Kenmochi, T. Koyama and G. H. Meyer, Parabolic PDEs with hysteresis and
quasivariational inequalities. Nonlinear Anal. TMA, 34 (1998), 665-686.
[10] P. Krejci, Hysteresis operators - a new approach to evolution differential inequalities.
Comment. Math. Univ. Carolin., 30 (1989), 525-536.
