Bemelmans J. et. al. (editor) Elliptic and Parabolic Problems: Rolduc and Gaeta 2001

Подождите немного. Документ загружается.

operator equation

for

Stokes boundary value

problems

Chandra

Department

Mathematics,

State University

Malang,

Malang, Indonesia

Graaf

Department

Applied Mathematics

and

Computing Science,

Eindhoven University

Technology,

The Netherlands

Abstract

The Stokes boundary value problems (SBVP)

can be

found

many areas

engineering,

for

example,

fluid mechanics when

the

Reynolds number

small.

This study shows

method

solving

the non

homogeneous SBVP

- Vp = -/, x e CI,

v-v

=-h, x e n, (*)

v(x)

a.(x),

x E 9fi,

by translating

into

operator equation

at the

boundary

dd of the

domain

fi.

First,

show that

the

general solution

of the

system, without boundary condition,

i.e.

f Av

= -/, x e n,

v-u = -h, x e ii,

of the

form

v =a

+Vf +

V{M

(V-

)

(V-

h)+

=-h-V-a

-V-Vf,

with

is a

harmonic extension

of a

which denotes

tangent vector field

at the

boundary

dU, 7 is an

arbitrary

but

fixed function,

and

Af(g),

Vf, 7^ are

respec-

tively defined

AAf(g)

=-g, x S U,

^Af(g)

=0, x e on,

A(£>/)

=-/, x e n,

=0, x e dn,

and

f MHM =o, x e n,

I |;7w =7, a: 6 da

Our operator equation relates the (unknown) tangent vector field a to the given

boundary condition a.

As an application, we present full explicit solutions of SBVP (*) for some simple

domains such as the interior of a disk and of a ball.

1 Introduction

In recent years, a number of studies have been made of finding a method to solve the

Stokes equations

/ nAv - Vp =0,

\ V-v = 0,

{la

which usually emerges in studying the steady flow of an incompressible, viscous fluid at

low Reynolds number. For example, Sheng and Zhong [1] and Padmavathi, et all [2]

mention some solutions such as the Naghdi-Hsu solution, the Papkovich-Neuber solution,

and the Boussinesq-Galerkin solution.

Inspired on the above ideas, we develop a general theory of solving the non homoge-

neous Stokes boundary value problems (SBVP) (see [3, p. 31])

fj,Av -Vp = -/, x G Q,

V-v = -h, x e ft, (2a,b,c)

v(x) = a.(x), x € dQ.,

Briefly, we solve the nonhomogeneous Dirichlet and Neumann problem that leads to an

operator equation at the boundary dQ of Q with the unknown is a tangent vector field a

at the boundary dQ. We obtain the solution of SBVP (2 a-c) can be 'parametrized' by

, the harmonic extension of a to the interior of Q.

Finally, we give some applications of the method to find the solutions of SBVP (2 a-c)

for some simple domains.

2 General Theory

On a fixed open domain Q C R" with piecewise smooth boundary dQ, we consider the

system of Stokes equations

' "

-{'

(3a,6)

V-w = —h.

Here / :

—•

, h : Q

—>

1R are given functions which depend on the position vector

x, which we usually suppress.

In the sequel, we put /j, = 1. For some given boundary condition v = a at dQ, we

want to solve v and p from (3a,6). From [2, 3], we know that the pair (v,p) is a solution

of (3 a, 6) if we put

(v =* + W,

(4 b)

with

(4>,

ip)

any solution of the system

A</>

=-/

A^ + V-tf, =-h.

(

It will turn out that the boundary condition to the system (3 a,

is related to the boundary

condition of (5a,b) by means of an operator equation. For later convenience, we now split

the solution

{4>,4>)

of (5a,b) into several pieces.

2.1 A Dirichlet problem

Consider the Dirichlet problem

Ac/>

=-f, x 6 SI,

=<*,

x e

on,

{6a

where a : dSl

—>

is an arbitrary but fixed tangent vector field at dSl. Next, split

= Vf + oc

, with

•A(vf) =-/,*€ si,

{7ab)

Vf =0, x e 90,

and

= 0, x e SI, ,

ga ft

= a, x e dSl. '

Note that 2?/ and a

can be obtained, at least in principle, by means of Green's function

[4,

pp. 86-87].

2.2 A Neumann problem

Consider the Neumann problem

Aip = -V-<j>-h = -V-a

V-Vf-h,x

e Q,

=7,*

{9a

where 7 : dSl —¥ IR is an arbitrary but fixed function. For this Neumann problem, we

impose the compatibility condition

since

J jda = - I(V-a

+ V-Vf + h) dr = - [(V-Vf + h) dr = 0,

Jan Jn Jn

(10)

/ V-a«dr= / a

nda = / anda = 0. (11)

Jn Jan Jan

Now split f/>=JV(V-a

)-l-A/'(V-X>/-l-/i)+7*Ar, with

AjV(V- a

) = -V- a

, x e SI,

lM(V-a

)

0,xedSl,

{Ua

AAf(V-Vf + h) = -V-Vf-h,xeQ,

and

kin*

= 0, x e Q,

r- an

(

14a

)

^7M

=7, x e aa.

' '

Note that, because of Aa

= 0, one has

A(-ix-a„) = -V-a„, (15)

where a; is again the vector position. The solution for the non homogeneous Neumann

problem (12a,6) can now be written

Af(V-a

) =

-±x-a

+ip(

(16)

where ip^°^ is the solution of the homogeneous Neumann boundary value problem

f AV>

(a)

=0, x e Q,

For any tangent vector field a. : 9f2

—>

IR" and any function 7 : dQ

—•

1R, the pair (v,p)

with

2.3 A parameterized class of solutions

tangent vector field a. : dQ

—>

IR" and any fi

f v =a

+Vf + VM(V-a

) + VM(V-Vf) + VJV(h) + V

^, ,.. .,

{

=4-V.a»-V.D/,

(18a

is a solution to the system (3o,6).

The problem we have to solve now is : How are a and 7 related to a prescribed vector

field a at dQ ?

It will be explained that for a the solution of an operator equation at the boundary

has to be taken. This operator equation involves all the data : a, f, h.

2.4 The operator equation at the boundary dCl.

In this section, we derive an operator equation which relates a : dQ

—>•

to the

prescribed data (a, /, h). From (4a), we obtain the requirement

v = a =

<j>

Vi>,

at dQ, (19)

a + VAf(S7-a

) = a -

lnM

- VM(V-Vf) - VAf(h), at dQ. (20)

If we take at each x g dQ, the inner product of identity (20) and the normal vector n,

we get

7 = an. (21)

Therefore,

obtain

the

operator equation

for an

unknown tangent vector field

a at the

boundary

+ VN

{V-a-H)

a-V{a-n)

-VN

{V-T>f) -VN{h),

at dfl, (22)

where

the

right hand side

is a

tangent vector field

on dfl

that

can, in

principle,

calculated from

a,/, and h. If we can

solve

from

the

operator equation

(22), the

solution

of the non

homogeneous SBVP

{

= -/, x e ft,

V-v = -h, x 6 fi,

(23a,6,c)

D(:E)

o(x),

a; e dfl,

is given

by (18 a,b) or

equivalently

= a

X>/

+ V {-|x-a„ +

(a)

}

VAf(V-Vf)

V.V(/i)

V(a-n)^,

=-h-V-a

-V-Vf.

(24a,6)

Next,

as an

application,

present full explicit solutions

homogeneous SBVP

for

some simple domains such

as the

interior

of a

disk

and of a

ball.

3 Applications

3.1

The

interior

of a

unit disk

Here,

consider

the

homogeneous SBVP with

the

domain

is a

unit disk,

i.e.

' Aw

- Vp =

x £ D =

{(a;,

y)\x

1},

V-v

=0,x£D,

„(*)

= a(x)

=±{a

(

-£$ ) e-

K ( $$> ) e«}

„

e SA

(25a,6,c)

with

= 0 to

satisfy

the

compatibility condition.

According

to the

theory,

have

solve

the

boundary operator equation

(22)

a +VjV(V-a

)

o-V(a-n)„

(26)

since

/ = 0, and h = 0.

Therefore,

first,

study

the

action

of the

operator

i—• a +

VJV(V-a„),

(27)

the

'basic' tangent vector fields

cos(6)

t„=

Sin

),n =

(28)

cos(0)

-sin(fl)

iNg

cos(0)

Using the notation z = x + iy and z = x

—

iy, we obtain the harmonic extension of t

Ki)

+1)+

K~i

)

1),neN

that leads to

\{\)

\{ I

Un =

°'

l[ I

)

2(H+1)

ne:

tn, ?T.

xz .

(29)

VJV(V-t^)|

i =

0,71 = 0,

' 71 t

(30)

We conclude the left hand side of the boundary operator equation (26) is a mapping

a^a +

VAf(V-a

(31)

that maps

(" t

i—>

, n e Z, n ^ 0,

(32)

tn,n = 0.

Therefore, given the boundary condition a, we can solve the boundary operator equation

6) for a

. I

we obtain

cos(#)

(26) for a

. For example, given the 'basic' boundary condition a = I • )a\ \

a-V(a.n)

= U

~»-l(

) z^ (33)

Hence, a, = 2(a - V {a-n)

) =

_n+l

3.1.1 Solution

Since / = 0 and ft = 0, we obtain from (24

o, 6)

the solution of the homogeneous SBVP is

n = Q„+VA/'(V'a

) + V(a-n)

, p=-V-a

. (34)

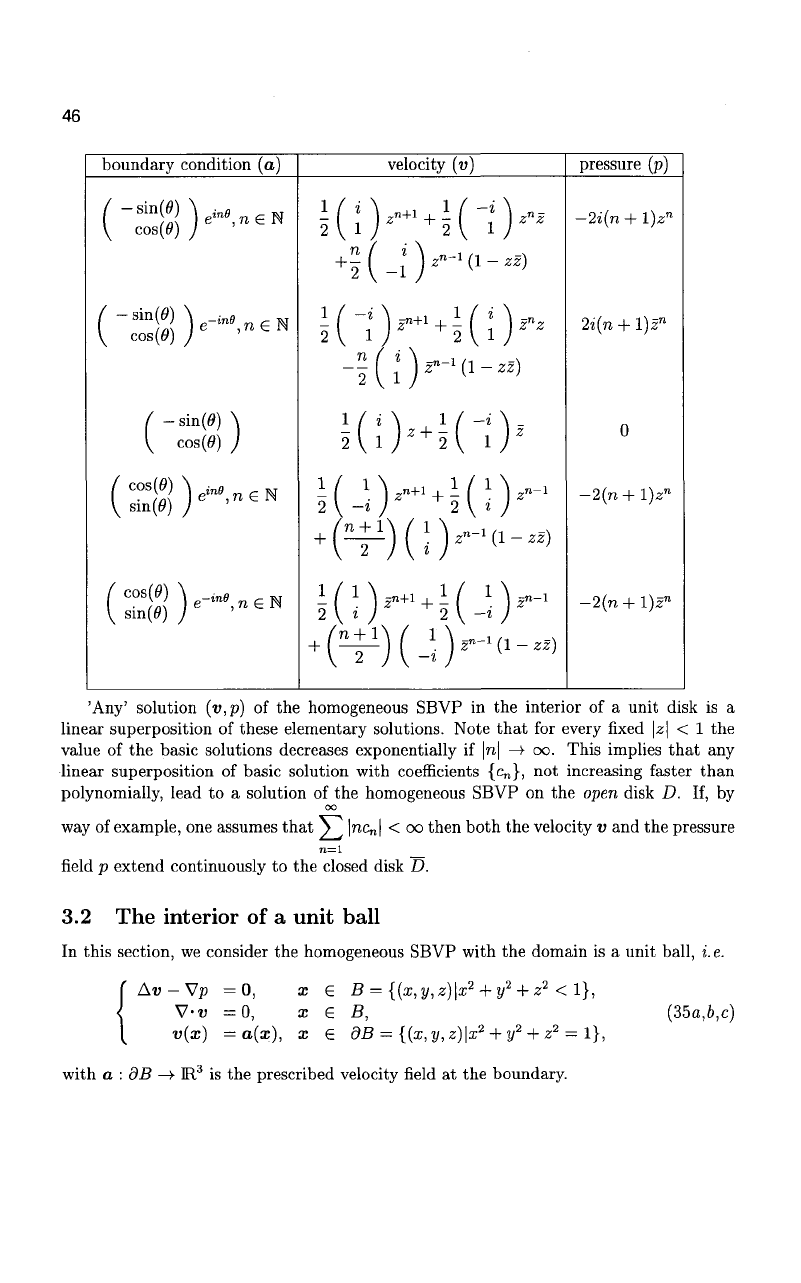
Below, we present a table of solutions for various 'basic' boundary conditions

boundary condition (a)

co8

(

) J

( -sin(fl) \

-in*

neN

(

COS

^)e^,neN

sin

(0)

/ COS(0)

ine

{ sin(0) )

6P<

velocity (w)

!l(-i')*""'<

K0-KD«

(

)(!)'-p->

pressure (p)

-2i(n + l).z

2i(n + l)z"

-2(n + l)z

'Any' solution (v,p) of the homogeneous SBVP in the interior of

unit disk is

linear superposition of these elementary solutions. Note that for every fixed \z\ < 1 the

value of the basic solutions decreases exponentially

\n\ —> oo. This implies that any

linear superposition of basic solution with coefficients {c

}, not increasing faster than

polynomially, lead to

solution of the homogeneous SBVP on the open disk D. If, by

way of example, one assumes that \J \nc

\ < oo then both the velocity v and the pressure

n=l

field p extend continuously to the closed disk D.

3.2 The interior of a unit ball

In this section, we consider the homogeneous SBVP with the domain is a unit ball,

Aw-Vp =0,

x e

B = {(x,y,z)\x

+ y

+ z

< 1},

V-u =0,

x e B,

v(x)

a(x),

x 6

dB = {{x,y, z)\x

+ y

+ z

1},

(35a,6,c)

with

: dB

—•

is the prescribed velocity field at the boundary.

3.2.1 Some notations and preliminaries

In this section, we introduce some notations that will be used later. The closure of B is

denoted by B = B

dB. For a tangent vector field a, one has for all x 6 dB : ax = 0.

We will use fat greek lower case symbols for tangent vector fields. Extensive use will

be made of the usual vector algebra and vector calculus in IR

applying the usual nabla

notations.

V/ = grad/, V-u = divu, V x v = rot v. (36)

Also the Euler operator £ = x • V = x^ +

y-g-

+ z-^, and its resolvents (£ + s)~

s > 0,

given by

(£ + sy

f(x) = f X-'HXx) d\, (37)

play an important role. For vector fields v the operators £v and (£ + s)

v are defined

componentwise. We mention some commutator properties for £ and its resolvents. They

follow from straightforward computation :

V£ = V + 5V, A£ = 2A + £A, V ({£ + s)"

/) (x) = (£ + s + ly

V/(x). (38)

Next, we discuss some lemmas.

Lemma 1 If a continuous vector function

—>

satisfies

A<p(x) =0, x e B,

V-<j){x)

=0, a; G £,

x-</> =0, i 6 9B

then

can

written as

(j)

= xx

V<p,

with Aip = 0 and

is unique if

require

<p(0) = 0.

Proof First we find a necessary condition on V</> by applying 'rotation' on both sides

Vx0 = Vx(« Vy?) = (V-Vtp)x + (V<p-V)x - (V- x)V> - (x -V)V</> = -{£ + 2)Vtp.

(39)

So a candidate for Vip is given by -{£ + 2)

(V x

<p).

We investigate whether —(£ +

(V x 0) = -V x ((£ + 1)

0) has a potential. Calculate

Vx ((£ + 2)-

(Vx</>)) = Vx (Vx (£ + 1)"V) = V (V- ((£ + l)"

*/))) - A(f +

l)"

= V(£ + 2)"

(V-<£)-(£ +3)"^ = 0.

So the vector field -(£ + 2)

-1

(V x

<p)

has indeed a potential ip, say. We write -(£ +

2)"

(V x 0) = V<p. Further note that V-V<p = -V-V x (£ +

l)"

= 0. So ip is

harmonic.

Finally we prove that the vector field u =

+ x x (V x (£ +

l)~

<p)

<p —

x x

V<p

is identically zero. Observe

V x u = 0. See above.

V-w = V-0 + (Vxx)-(Vx (£ + l)"V)-(Vx (V x (£ + l)-

^)^) = 0.

we conclude that u = Va, with

ACT

= 0. However also xu = £a = 0. Expand a

oo oo

in spherical harmonics a = Y^On. Then £a =

VJnff

We conclude that a

= 0 if

n=0

n = 1, 2,

• •

•. Therefore cr must be constant and hence u = 0.

Lemma 2 If a continuous vector function

4> •

—)•

satisfies

A<j>{x) = 0, x € B,

0-x = 0, x e 3S,

i/ien

</>

can be written

(j>

4>a

<Ai>

*"i£/i

<£

= a; x V<£, (40)

*i = Vx-^x

KN'-iJv^

ir^x. (41)

with both if : B

—>

M orad x

;

-B -*• IR harmonic. If we require ip(0) = x(0) = 0, both ip

and x are unique. Moreover x satisfies

[—(£

+ l)

+ | + \{£ + I)"

] X = V-<£ = V-0

Proof First, construct x from V-0, i.e. x = [-(£ + I)

+ | + K

I)"

<£>

and

define (^ according to (41). Put

(f>

<j>

Observe that V-0

= 0. Next, we obtain

x-<p

= (1

—

|a;|

)5 [/

—

|(5 + §)

£] X- Therefore at the boundary, we get

x-<j>

= 0.

Further, since

A(j>

= 0, also

Acj)

= 0. Hence, according to the preceding lemma, we

obtain

= x x Vip. Finally, note that untill now ip and x

are

fixed up to an additive

constant. By taking </?(0) = x(0) = 0, this arbitrariness is removed.

Lemma 3 Let i? : B

—•

IR be continuous and such that Ai9(x) = 0,x E B, i?(0) = 0. //

the vector function w : B -+ IR

satisfies

Aw(x) =0, x e B,

w(x) = a;i5(a;) + ex, x € 9B, c € IR.

T/ierj to can 6e written w = V£~

d + ex. Note that c = ^ /

8fl

tu(x)

-a;

du.

Proof Straightforward computation yield Aw = A(V£

i9 + ex) = 0. Next, by taking

the inner product w(x)-x at the boundary, one finds

x • w = x • V£

$ + x • ex = &(x)

which is equal to x

•

w = x

•

ex = i?(x) + c. Finally, take the inner product w (x)

•

at the boundary, calculate the integral over the boundary, and use the mean value theorem

for harmonic functions, to obtain

/ wxda = x-xi!)(x) da+. exx da = 47n9(0) +

ATTC,

JBB

JdB JdB

-I

4?r J

c = — / w • x da.

47T

In the preceding lemmas, we gave three different recipes for constructing harmonic

vector fields from harmonic functions. For later convenience we introduce a notation for

these recipes.

Definition 4 Let

hj[<p]

: B

—>

IR,

j = 0,1,2, denote the harmonic vector fields defined by

[ip]

hilx]

x i-> x x V(/j(x),

x M. V

- (£*)x +

\{\*\

~ 1)V [(£ + \y

£} x,

x M- Vf

i9.

Remember that

<p,

x, and

-d

are supposed to be harmonic. We gather the recipes of this

section in the following theorem.

Theorem 5 Consider the continuous vector field a

—>

. Let a

: B

—>

be the

harmonic extension of a. Then there exist unique harmonic functions (p,x,$

'•

^>-

TR,

with ip(0) = x(0) =

&(Q)

= 0 such that

[(p]

+ h^x] + hzltf] + ex,

with c =

(ax) da. Note that if d = 0 and c = 0, then a is a tangent vector

field.

Proof The proof is divided into several steps.

Step 1. Split the vector field a into a tangential and a normal component as follows :

a = a

+ a

+ en, with c = ^ J

(a• n) da, o„ = {a-n-c)n and a

= a

—

- en.

Note that a

is tangential.

Step 2. Define $ as the solution of the Dirichlet problem

Ai?(x) =0, x € B,

•d(x) =an

—

xedB.

Step 3. Note that for any x e dB, the vector field a - V£

t?(x)

—

ex is tangential.

Step 4. The harmonic extension x i-> a

(x)

—

'V£~

'd(x)—cx satisfies the conditions

of lemma 2. This means it can be uniquely written as /io[</?] + hi[x] f°

suitable

harmonic

and x-

Note : Keep in mind that h

and hi also depend on an. In our case, V-« = 0 implies

c = 0.

3.2.2 Solution

In a similar way as in the section unit disk, we have to solve the boundary equation (22)

(42)

and at first, we study the operator

ai-^a + VJV(V-a„). (43)

Based on theorem (5), we know that any tangent vector field at the boundary a can be

splitted into half] +

hi[x]-

Therefore,