Bemelmans J. et. al. (editor) Elliptic and Parabolic Problems: Rolduc and Gaeta 2001
Подождите немного. Документ загружается.


480
Moreover, suppose that
d
m
, %eL
4
(0,T;ff),
\M\LH0,T;H)
+
\\
d
t<Pl\\
L
*(0,T;H)
+ \\
Bt
P\ \\
lA(0,T;X)
+
\\
W
2\\^(0,T;V)
^
R
>
for a given R > 0. TTien i/
(<^I,CI,ID
1
,£I)
and
(</S2,C2,
^2,^2) ire couples of solutions to
(17) related to the initial data </9o,i, c^j and
</3o,2,
Co,2 respectively, we have
\\<Pl
-
i
P2\\
L
«.(
0
,T;V)nL
2
(0,T;rr
2
(n)) + ll
C
l
_ C
V\L°°(0,T;V')nL2(0,T;V)
< k
R
(||</3
0
,l - (^0,2||y + ||C0,1 - CoAv) '
7n particular, the solution
(<p,
c,
VJ,£)
provided by Theorem 2.1 is unique.
Remark 2.3 We note that (24)i is surely satisfied if (20) holds.
Theorem 2.4 Let (4), (14)-(15), (18) and (22)
2
hold.
Let us assume that
c
0
e H
3
(ft), <9
n
c
0
= 0, on T, 3£
0
£ V : £
0
e
(3
(c
0
) a.e. in ft. (25)
Then,
there exists
TQ
€ (0,T] such that the solution (ip,c,w,£) given by Theorem 2.1
fulfills the additional regularity
c € H
1
(0,
T
0
; V) n i°°
(0,
T
0
; ff
2
(ft)), , .
»er
(o,
r
0
;
y) n
L
2
(O,
r
o;
#
2
(ft)).
(M)
Moreover, if (22)\ holds, we can choose
TQ
= T.
Remark 2.5 Thanks to the first of
(26)\,
under suitable hypotheses on
<po
(e.g. ipo €
H
3
(ft) and d
n
ifo = 0, on T), further
parabolic
regularity could be deduced also for
(p
by
differentiating (17)\ and multiplying the result by
d
u
<p,
d
t
Btp. We do not enter into the
details, since the procedure is standard.
Theorem 2.6 Let (4), (14)-(15), (18) and
(22)%
hold for two pairs of initial data y?o,i,
(fyi and
>po,2,
c
0>
2
also satisfying (23). Let also To G (0,T] and suppose (y>i,Ci,u)i,£i)
and
(v?2,C2,
^21^2) is a couple of solutions to (17) related to the different initial data and
fulfilling
d
m
, Bn, 8
t
c
u
B
Cl
e L
A
(0,T
0
; H), Vw
2
e L
2
(0,T
0
;L
3
(ft)),
llyi|li
4
(0,ro;ff) + ll
a
*Vlllt
4
(0,To;ff) +
\\
B<
PI\\L*{0,TO;H)
+ ll
C
l
\\L*(0,T
0
;H)
(27)
+ \\dtCi\\
Li{oa
,
0
.
H)
+ IIBdll^jo.r^H) + \\^
w
2\\mo,T
0
;LHn)) < R,
for a given R > 0. Then we have
IIVl
_
f2\\L^(0,To;V)nL^(0,T
0
]H^(n)) + ll
C
l ~~
C
2|lz,»«(0,To;V')nL
2
(0,To;^)
< k
R
(||ipo,i - Vo,21|v + l|co,i - co,2|lv) •
In particular, the solution (p,c,w,£) given by Theorem 2.1 is unique on
[0,To].
(28)
Remark 2.7 As before, (27) are not satisfied in the given regularity setting. However, if
(20) and (25) are assumed, then (27) do hold at least up to a suitably small final time T
0
.

481
3 Proofs of the Theorems
Proof of Theorem 2.2. Set (ip,c,w,£) := {<Pi,ci,Wi,£i)
—
(ip
2
,C2,W2,(,2)-
Then, writing
(17) for the two solutions and substracting the results, we obtain
d
t
tp + Btp = F
x
{ip{)
- F
x
(<p
2
)
+
Cl
(F
2
(
Vl
) - F
2
(<p
2
))
+ cF
2
(<p
2
).
(29)
Doing the same with (17)2,3, we have, respectively
d
t
c +B
vi
w + (B
V1
-B
V2
)w
2
= 0, . .
w = Be + £ + 7 (ci) - 7 (c
2
) + g (fi) - g (p
2
)
•
Then, we test (29) by if + Bip and integrate over
(0,
t) for t < T. By (14)
lj2
, (15) and
Young's inequality, we easily infer
\ llv Wily + II V^li
2(Qt)
+ ^ l|B</>ll£»
W
,) < 2 IIVo.1 " Vo,a||v + k
\\<fi\\l
HQt)
+ fc
\\c\\
2
LHQt)
.
(31)
Indeed, (15) guarantees that
||CI||
LC
„(QX
< 1.
Now, we test (30)
2
by c (which is in V
0
by (23) and (17)e) and (30)i by A/^c =: u,
substract the results and integrate over (0,t). Noting that two terms cancel and using
Young's inequality and the monotonicity of
f3,
we get
/ (d
t
{B
¥
,
1
u),u)dt+ / (fi^)-/j,(<f
2
))Vw
2
- Vudxdtdx + \\Vc"
2
Jo „ J oj n
LHQt) (32)
£
2
(Qt)
T
*• "
G
lli
2
(Qt) •
< k
\\u>\\
2
,
la
,
+ k lie"
2
Let us deal with the first two terms I\ and h on the left hand side of the preceding
inequality. For the first one, by (5), (8) and (22)
2
, we have
h
\( n{^{t))\Vu{t)\
2
dx-
l
-[ fi&
0tl
)\Vu(0)\
2
dx
+1 [ [ v'(<Pi)dm\Vu\
2
dxdt (33)
1
J oi n
> f |V«(i)|
2
-f
IKi-coX'-f/
/ \dm\\Vu\
2
dxdt.
Then, using (11) and a Galiardo-Nirenberg inequality [5, p. 125], we infer
|2
/ / \dtipiWVu\Uxdt
J oJ a
<k f |^i|||Vu||*
t(n)
«tt
< k j \d
m
\
{\\u\\%\
n)
IVu|
1/2
+ IVu|
2
) dt (34)
< kj
\dt
Vl
\
\Vu\
2
dt +
kj^\d
m
\
\Vu\
x
'
2
(|c|
3/2
+ ||Hl5p
(
n) |Vn|
3/2
) dt
< f \d
m
\\Vu\
2
dt + k\\c\\
2
+k f (Ift^il
4
+
|S
Vl
|
4
+ l^l
4
) |V«|
2
dt.
Jo Jo

482
The second term
in
(32) gives, thanks
to the
continuous embedding
H
2
(Q)
C
L°°
(CI)
\h\
<
k
f IML~(n) IVtual |
V«|
dt < | J* (|^|
2
+
\B<p\
2
)
dt + kf
\Vw
2
\
2
\Vu\
2
dt.
(35)
Now, take
the
sum
of
(31)
and
(32)
and
note that (10) can
be
applied with
c in
place
of
(, so
that
the
L
2
-norms
of c on the
right hand sides
of
(31)
and
(32)
are
controlled
by
taking
an
opportune 6. Taking advantage
of
(33)-(35) and recalling assumptions (24),
we
conclude applying Gronwall's Lemma
in the
form
of,
e.g. [3, Lemme A.4,
p.
156],
to the
function
t
i->
||<^
(t)||J.
+
|Vu(i)|
2
.
•
Proof
of
Theorem 2.4. We derive new
a
priori bounds for the solutions
of
(17).
We point
out that,
at
this level, some passages are formal. Anyway, they could
be
made rigorous
by
performing the estimates
at
an approximating level
(cf. e.g.
the Faedo-Galerkin argument
of [4, Sec. 3]). Then, differentiate (17)3 with respect
to
time. This gives
d
t
w
=
d
t
Bc
+
d
t
£,
+ 7'
(c)
d
t
c +
g'
(<p)
dtip.
(36)
Now test (17)2
by d
t
w and (36) by d
t
c and
integrate over (0,i),
t < T.
Noting that
two terms cancel
and
using (6), (14)i
]2
, (22)
2
and the
monotonicity
of
/3,
we get
\\dtVc\\l
2Kt)
+
^\Vw(t)\
2
<^\Vw(0)\
2
+k
J
o
J ((\dM +
\8
t
c\)
\Vw\
2
+ k
\\d
t
<p\\
2
LHQt)
+ k
\\d
t
c\\
2
LHQt]
) dxdt.
Of course,
the
main trouble
is
given
by the
integral term
J on the
right hand side.
Using Sobolev's embeddings,
(11) and the
Gagliardo-Nirenberg inequalities, we infer
J
< kj
(\\dM\
v
+ \\d
t
c\\
v
)\\Vw\\
2
L12/Hn)
dt
<
k f
(\\dM\v
+
\\d
t
c\\
v
)
(\Vwf
2
\\w\\U
2
+
\Vw\
2
)
dt
<
•J
(\\9M\v
+ \\d
t
c\\
v
)
(\Vwf
2
||H|$
(n)
+ |VH
2
)
•
k
f
(\\dM\y
+ \\d
t
c\\
v
)
J
0
(38)
x (|VH
3/2
\d
t
c\
1/2
+ \Vw\
2
(l +
\\<p\\
HHn)
+
llcll^p,,))
dt := J2 Jj,
3=1
where
the
integrals
Jj are
defined
in
this way
:
Ji
:= k [
\\dM\v\VM
i/2
\dtc\
1/2
dt,
J
2
:= k f
\\d
t
c\\
v
\Vw\V
2
\d
t
c\
1/2
dt,
J
3
:= kj
||3^||
v
|V
W
|
2
(l
+
|MI^
(
n))^
J* •= */
||^|lv|Vw|
2
||c|
J
5
:=
kJj\d
t
c\\
v
\Vw\
2
(l + M
H2m
)dt,
J
6
:= kj
||a
(
c||
v
|VH
H
2
(fl)
"*>
C
IIH
2
(SJ)
^*-

483
Now we estimate the Jj's. The term J
3
does not give troubles. Instead, Jj, J
2
, J4, J5,
owing to the third of (21), are bounded as follows:
k [ \Vw\
2
\\d
tl
p\\y
3
dt + k f \d
t
c\
2
dt,
J Q
J 0
h < 6 [ \\d
t
cf
v
dt + k
6
f \Vwfdt,
Jo J 0
-9 ° t (39)
Ji < kj
\Vw\
2
\\c\\*
H2{Q)
dt
+ kJ \Vw\
2
\\dM\fdt,
h < S f \\d
t
cf
v
dt + k
s
[ \Vw\
4
dt.
Jo Jo
The estimation of the latter term J
6
, instead, is more complicated and we proceed
similarly as in [1, p. 497]. More precisely, we collect in the next computation also the first
term resulting from J
4
on the right hand side of
(39)3.
By elliptic regularity :
Je
+ k [ \Vw\
2
\\c\\
l
HHn)
dt
J
0
t t
(40)
< S I
\\d
t
c\\
2
v
dt + ks f (|VH
4
(|c|
2
+ |Bc|
2
) + \Vw\
2
(|c|
4
+ |Bc|
4
)) dt.
Jo Jo
Then, we multiply (17)3 by Be. Owing to the monotonicity of
/3,
to (14)^2, and to the
second of (16)2, we easily infer, a.e. in (0,T)
\Bc\
2
< k (1 + |V«f + |Vc|
2
) < k (1 + |Vu;|
2
)
•
(41)
Then
Je + k J \Vw\
2
\\c\\
4
H2(n)
dt<6 J \\d
t
c\\l dt + ks j
{\Vw\
2
+ \Vw\
6
)dt. (42)
Finally, we have to bound the norm of w (0) in (37). Writing (17)3 for t = 0 (this could
be made rigorous working on the discrete scheme) and recalling (25), we see that
\Vw (0)|
2
< k (l + |h||^
(n)
+
Uof
v
+ \\<p
0
f
v
) < k. (43)
Now, let us take (38)-(43) into account and go back to (37). If (22)
x
holds, the situation
is simple since J
2
= J4 = J5 = Je = 0. Actually, we can apply (10) with
d
t
c, w, tp in place of £, u, v and z, (44)
respectively. Thus choosing 8 suitably small and recalling (21), we see that Gronwall's
lemma applies to the function t i-> \Vw(t)\
2
. This yields Vui € L°°(0,T;H) and also
to the first of (26)i for T
0
= T. The second of (26)i is a consequence of (41). Now the
first of (26)2 would easily follow once we show that w 6 L°° (0,T
0
; H). Due to Neumann
conditions, this is not obvious and has to be proved by suitably adapting the argument
of e.g. [2, Subsec. 5.3]. At this point, also the second of (26)2 is proved since, looking

484
back
at
(17)2,
we can
exploit
(11)
with
the
choices
(44) and
take advantage
of the
third
of
(21) and of the
second
of
(26)i.
Instead,
if
(22) does
not
hold,
we
deduce from (37)-(43) that
liavc|k
wo
+
|v™(t)|
2
<
k +
kj*
(/|VH
2
+
|v
rfi,
where
/ := (l +
||%>||y
3
)
e
1/(0,
T),
for
some
p > 1.
Then, using
a
generalized
Gronwall's lemma
(e.g.
a
slightly modified version
of
[6, Thm. 7.1]
works
for
our
case),
we obtain
the
same relations
as
before, holding
now up
to a
final time To
6
(0,
T].
B
Proof
of
Theorem
2.6.
It is
very similar
to
the
proof
of
Theorem
2.2.
Then,
we
just
give
the
highlights. Proceeding
as in
that proof
(but
choosing
now
t 6
(0,T
0
j),
we see
that
the
last term
in (33) now
depends also
on
d
t
Ci. However,
it
can
still
be
estimated
as
in
(34) by
taking
the
contribution
of
d
t
c\
into account. Thus,
the
last term
in
(34)
will
now depend
on all the
norms
in
(27)2 but the
latter.
The bound
(35)
needs
to
be
modified more carefully. Actually,
we now
have
/
/ (/•'(VijCi)
-M(V2,C
2
))
VM;
2
•
V« dxdt
k
J \M\
L
°°(n)\V™2\\Vu\dt
+
kJ ||c||
L
e
(f!) l|Vw
2
||
L
3(fj) |Vu|
dt.
Thus,
the
first term
can now be
bounded
as
before, while
the
second
one is
less than
^
II
C
IIL
2
(O
T-V)
+
^«
Jo II Vw2||
L
3/m I
V«|
dt, so
that,
on
account
of the
last
of
(27)2,
a
further
application
of
Gronwall's Lemma [3, Lemma A.4,
p. 156]
permits
us
to
conclude.
•
References
[1]
J. W.
Barrett
and
J. F.
Blowey, Finite element approximation
of
the
Cahn-Hilliard
equation with concentration dependent mobility. Math. Comp.,
68
(1999), 487-517.
[2]
E.
Bonetti,
W.
Dreyer,
and G.
Schimperna, Global solution
to
a
viscous Cahn-Hilliard
equation
for
tin-lead alloys with mechanical stresses. Submitted.
[3]
H.
Brezis, Operateurs maximaux monotones
et
semi-groupes
de
contractions dans
les
espaces
de
Hilbert. North-Holland Math. Studies,
5,
North-Holland, Amsterdam, 1973.
[4]
D.
Kessler,
J.-F.
Scheid,
G.
Schimperna
and U.
Stefanelli. Study
of a
system
for
the
isothermal separation
of
components
in
a
binary alloy with change
of
phase. Paper
in
preparation.
[5]
L.
Nirenberg,
On
elliptic partial differential equations.
Ann.
Scuola Norm.
Sup.
Pisa
(3),
13
(1959), 115-162.
[6]
M.
Sassetti
and A.
Tarsia,
On
a
nonlinear vibrating-string equation.
Ann. Mat.
Pura
Appl.
(4), 161
(1992),
1-42.

Bifurcation in population dynamics
Kenichiro Umezu
Faculty of Engineering, Maebashi Institute of Technology
460 Kamisadori, Maebashi 371-0816, Japan
Email : ken@maebashi-it.ac.jp
Abstract
This paper is devoted to the study of a local bifurcation problem for a nonlinear
elliptic boundary value problem arising in population dynamics, having nonlinear
boundary conditions. Necessary and sufficient conditions for the existence of bifur-
cation points are studied. Ecological interpretations of our results are also given.
1 Introduction
Let D be a bounded domain of B.
N
, N > 2, with smooth boundary dD. In this paper we
consider the following nonlinear elliptic boundary value problem:
du
-AM
= \(m(x)
—
au)u, in D, — = b(x)g(u), on dD, (1)
where A is a positive parameter, m e C
e
(D) is a real-valued Holder continuous function
with exponent 0 < 6 < 1 on the closure D of D, and satisfies : m(xo) > 0, at some point
XQ
€ D, a is a given positive constant, b 6 C
1+e
(dD), b > 0 and b ^ 0 on dD, g is a
real-valued sufficiently smooth function near t = 0 which satisfies g(0) = 0, and n is the
unit exterior normal to dD.
It is well-known (cf. [3, 4]) that the equation :
—AM
= \(m(x)
—
au)u, in D, describes
the steady state for the population density of some species which diffuses at the rate
1/A, where m(x) denotes its growth or decay rates at x G D, meaning that the regions
{x € D : m(x) > 0} and {x e D : m(x) < 0} are respectively favorable and unfavorable
for the species, and a represents the crowding effect! The nonlinear boundary condition
du/drx = b(x)g{u) on dD means that the rate of the flux of population into the region D
is governed in a nonlinear way by b(x)g(u) on the boundary dD. From this viewpoint,
the existence of positive solutions is of ecological interest. Here a solution u 6 C
2
(D) of
(1) is called positive if u > 0 on D. We assume here for g that
g(t) is non-negative for all t > 0 close to the origin. (2)
Ecologically speaking, assumption (2) means that only the influx of population can
occur at the boundary, if its density is sufficiently small there.
485

486
In the Neumann case, that is g = 0, Hess proved in [6] the existence, uniqueness and
asymptotic stability of positive solutions and also determined the qualitative behavior of
the unique positive solution when parameter
A
runs, especially when
A
J. 0. By
fJ.i{\),
we
denote the unique principal eigenvalue of the eigenvalue problem
—A<p —
\m(x)if
—
fJ.{\)<p,
in D, — = 0, on dD, (3)
where principal eigenvalues mean eigenvalues with corresponding positive eigenfunctions.
Brown and Lin proved in [2] that there exists a unique and positive Ai(m) such that
/u
1
(Ai(m)) = 0, provided
f
D
mdx
< 0.
Our consideration starts with the following result.
Theorem 1.1 (Hess [6]) Let g = 0. Assume first that J
D
mdx > 0. Then there exists
a unique positive solution u\ €
C
2+e
(D)
of (1), for every
A
> 0, which is globally asymp-
totically stable, and the trivial solution u = 0 is unstable for all X > 0. Moreover, we
have
f
D
m(x)dx
u\-
a\D\
_ —>0, asXIO,
C
2
+
e
(D)
where \D\ is the volume of D.
Assume now that J„ mdx < 0. Then there exists a positive solution ux €
C
2+e
(D)
of
(1) if and only if
A
>
\\{m),
u\ being unique, globally asymptotically stable and such that
II
U
A||
C
2+8(D)
—* °>
as A
i M
m
)-
Moreover, the trivial solution u = 0 is unstable for any A > Ai(m) and globally
asymptotically stable for each 0 <
A
< Ai(m).
Some ecological interpretation of Theorem 1.1 can be given concerning the qualitative
behavior of the globally asymptotically stable, non-negative solution U\ when A J. 0. If
f
D
mdx < 0, then Ux is identically equal to zero for 0 < A < Ai(m). This would say that
the species becomes extinct if he diffuses at the so large rate. If f
D
mdx = 0, then U\
tends to zero as A J. 0, which means that the steady state for the population becomes
small gradually and extinct asymptotically as the diffusion rate goes to infiriity. In the
case J
D
mdx > 0, Ux keeps positive even when A J. 0. This implies that the species is
persistent even if the diffusion rate goes to infinity. To sum up, if the food environment
becomes rich, then the more the species diffuses, the more we find out clearly that it is
favorable for him.
In the case of the nonlinear boundary condition, the uniqueness of positive solutions
does not necessarily hold (see Amann [1, Theorem 2.6] and Pao [7, Theorem 4.6.3] for
criterions for the uniqueness). This suggests that it is quite difficult to obtain always a
globally asymptotically stable solution for (1). For this reason we restrict our attention
to the problem how the stable steady state behaves as
A
J. 0 for the corresponding initial-
boundary value problem
— =
—At;
+ m(x)v
—
av
2
in
(0,
oo) x D,
v(0,x) = u
o
(z)>0 inD, (4)
dv
— = b(x)g(v) on (0, oo) x dD,

487
with sufficiently small initial data u
0
^ 0. For this problem, the following bifurcation
and stability problems arise naturally. Our main interest of this paper is to study the
problem of bifurcation to the right at the origin for (1) of positive solutions, meaning
the problem whether there exist sequences {A^} and
{WA^}
of positive solutions of (1)
for A = \j, such that A.,- J. 0 and
HMAJICCD)
-» 0 as j -» oo, or not, and additionally
to investigate the stability of the bifurcation positive solutions. Our results under the
nonlinear boundary condition are compared with Theorem 1.1 in the framework of the
local bifurcation problem.
In the work [9], we study the behavior of the minimal positive solution of (1) when
A
| 0, under some growth condition on g, mainly for the case J
D
mdx > 0 by the method of
super- and sub-solutions, in which there is the corresponding result for the case J
D
mdx =
0 that is rather weak. In the present work, we focus on the case f
D
mdx = 0 and on the
existence of the local bifurcation branch, which represents the minimal positive solution,
within the scope mentioned above. In the present work, our approach which relies on
bifurcation theory, is different from that used in [9].
2 Main results
Before stating our main results, we remark that the condition g'(0) = 0 is necessary for
the origin (X,u) = (0,0) to be a bifurcation point to the right for (1). Indeed, if
Uj
is a
positive solution of (1) for
A
= Xj such that A., J. 0 and
H^j
Hcrrn)
~~*
0> then we can verify
that the function Vj := Uj/llujllpfm tends to some v
0
e C
2
(D) which satisfies
-Av
0
= 0, in D, -^ = b(x)g'(0)v
0
, on dD.
Now we can formulate our main results. First we have the following result for the case
J
D
mdx > 0.
Theorem 2.1 Suppose that J
D
mdx > 0. // condition (2) is fulfilled, then the origin
(A,u) = (0,0) is not a bifurcation point to the right for (1).
Next we have the following result for the case J
D
mdx < 0.
Theorem 2.2 Suppose that J
D
mdx < 0. // there exists an integer k > 2 such that
g'(0)
= ---=g
ik
-
1)
(0)=0 and ff
w
(0)>0,
then the origin
(A,
u) = (0,0) is a bifurcation point to the right for (1). More precisely, the
set of all non-trivial solutions u of (1) in
A
> 0 and
||W||
C(
B)
both small enough consists
exactly of a unique smooth branch of positive solutions emanating from the origin.
Remark 2.3 In the case J
D
mdx < 0 the origin
(A,
u) = (0,0) is not a bifurcation point
to the right for (1) provided g(t) = 0 for all t > 0 close to the origin, see Theorem 1.1.
This indicates that Theorem 2.2 would be optimal under the additional assumption that g
is analytic at t = 0.

488
Finally we state our results for the case J
D
mdx = 0. Let 70 €
C
2+e
(D)
be the unique
solution of the Neumann boundary value problem
—A70 = m(x), in D, -^— = 0, on dD, /
y
0
dx
= 0.
on J
D
Theorem 2.4 Suppose that J
D
mdx = 0. //
«/(0)=
fl
"(0) = 0, <T(0)>0, (5)
L
b{X)da>V0
'-
=
2
9
'"(*)5
D
\^
0
?dx>
(6)
where da is the surface element of dD. Then the origin (A,u) = (0,0) is not a bifurcation
point to the right for (1). More precisely, there is no non-trivial solution u of (1) for any
A
> 0 and |Mlc(5) both sufficiently small.
On the other hand, we have the following.
Theorem 2.5 Suppose that f
D
mdx = 0, and suppose condition (5) holds. If
b(x)da <
VQ,
(7)
JdL
then the origin (A,u) = (0,0) is a bifurcation point to the right for (1). More precisely,
for any
A
> 0 small, there exists a minimal positive solution ux €
C
2+e
(D)
of (1), which
satisfies that
||"A|IC
2
+
S
(O) —•
0 as
A
J. 0. Additionally if
g
is analytic att = 0, then the set
of all non-trivial solutions u of (1) in
A
> 0 and ||u||c(i5) both sufficiently small, consists
exactly of two smooth branches of positive solutions emanating from the origin.
The following two results are complementary to Theorems 2.4 and 2.5.
Theorem 2.6 Suppose that J
D
mdx = 0, and that g'(0) = 0. Additionally if g"(0) > 0,
then the origin (\,u) = (0,0) is not a bifurcation point to the right for (1).
Theorem 2.7 Suppose that
f
D
mdx
= 0, and that condition (2) holds. Ifg'{0) = g"{0) =
g'"(0)
= 0, then the origin (A,u) = (0,0) is a bifurcation point to the right for (1). More
precisely, problem (1) has a minimal positive solution ux G
C
2+e
(D)
for any
A
> 0 small
such that ||uA|lc
2
+
e
(5) -^ 0 as
A
| 0.
3 Proofs of our main results
In this section we give a sketch of the proofs of our main results. Theorem 2.1 is proved
having a priori lower bounds by a positive constant for positive solutions of (1), which is
a direct consequence of [9, Theorem 3]. For the case f
D
mdx < 0, we can apply the well-
known local bifurcation theory for simple eigenvalues due to Crandall and Rabinowitz [5,
Theorem 1.7] and then Theorem 2.2 follows.
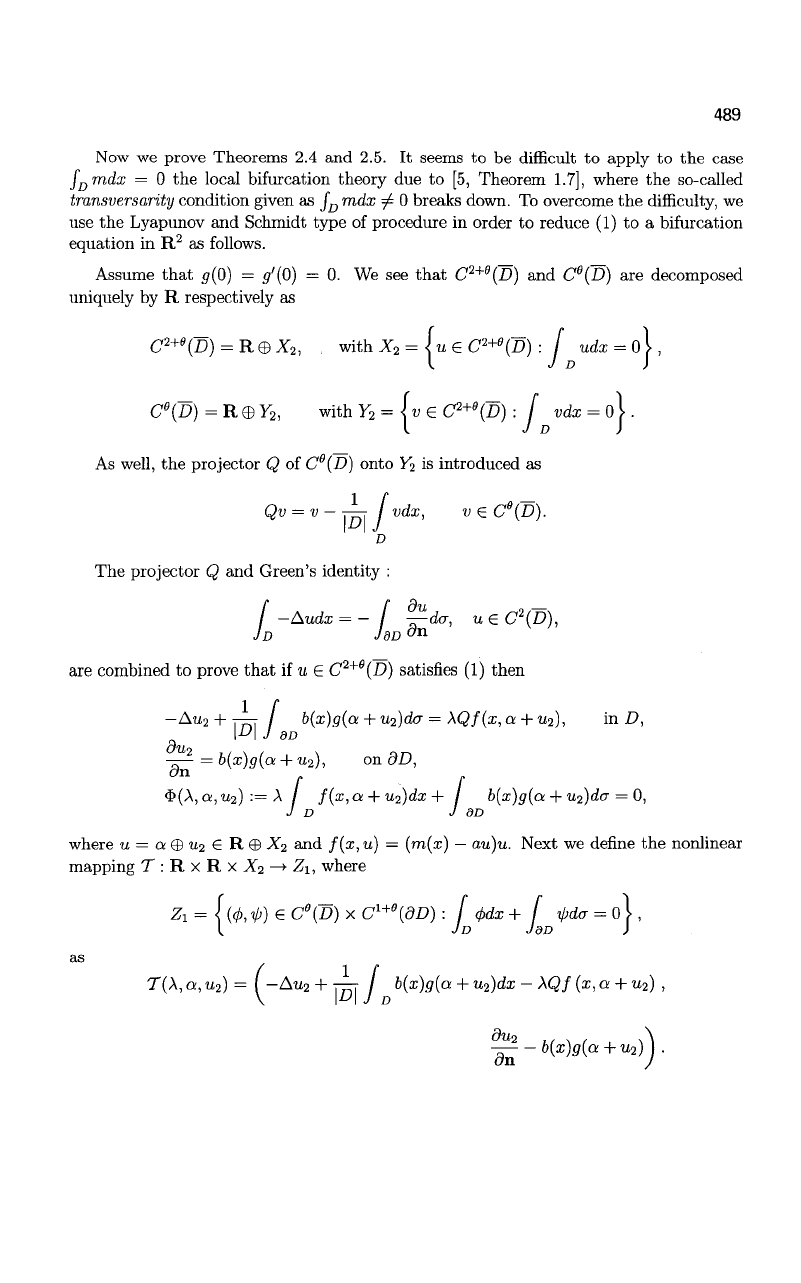
489
Now we prove Theorems 2.4 and 2.5. It seems to be difficult to apply to the case
J
D
mdx
= 0 the local bifurcation theory due to [5, Theorem 1.7], where the so-called
transversarity condition given as J
D
mdx
=/=•
0 breaks down. To overcome the difficulty, we
use the Lyapunov and Schmidt type of procedure in order to reduce (1) to a bifurcation
equation in R
2
as follows.
Assume that g(0) = g'(0) = 0. We see that C
2+e
(D) and C
e
(D) are decomposed
uniquely by R respectively as
C
2+e
(D) = R ffi X
2
, . with X
2
= lue C
2+e
(D) : I udx = o| ,
C(D) = R © Y
2
, with Y
2
= Iv G C
2+e
(S) : J vdx = o\ .
As well, the projector Q of C
6
(D) onto Y
2
is introduced as
Qv = v--^- fvdx, veC
e
(D).
D
The projector Q and Green's identity :
[ -Audx = - J -£-do, u e C
2
(D),
JD
JdD
^n
are combined to prove that if u € C
2+6
{D) satisfies (1) then
-Au
2
+ j--7 b(x)g{a + u
2
)da = \Qf(x,a + u
2
), in D,
l-^l J BD
—^- = b(x)g(a + u
2
), ondD,
$(A,a,u
2
) :=
A
/ f(x,a + u
2
)dx + b(x)g(a + u
2
)da = 0,
J D J 3D
where u = a®u
2
eH®X
2
and f(x, u) = (m(x)
—
au)u. Next we define the nonlinear
mapping T : R x R x X
2
—>
Z\, where
Z
1
=
/(</>,
V) e C(D) x C
1+e
{dD) : f
<f>dx+
f i/ida = o| ,
as
T(A, a, u
2
) = ( -Au
2
+ Tjj; H
x
)9(a + u
2
)dx - XQf (x, a + u
2
) ,
-^ - 6(s)s(a + u
2
]
