Bemelmans J. et. al. (editor) Elliptic and Parabolic Problems: Rolduc and Gaeta 2001

Подождите немного. Документ загружается.

460

0.02

0.015 -

0.01 -

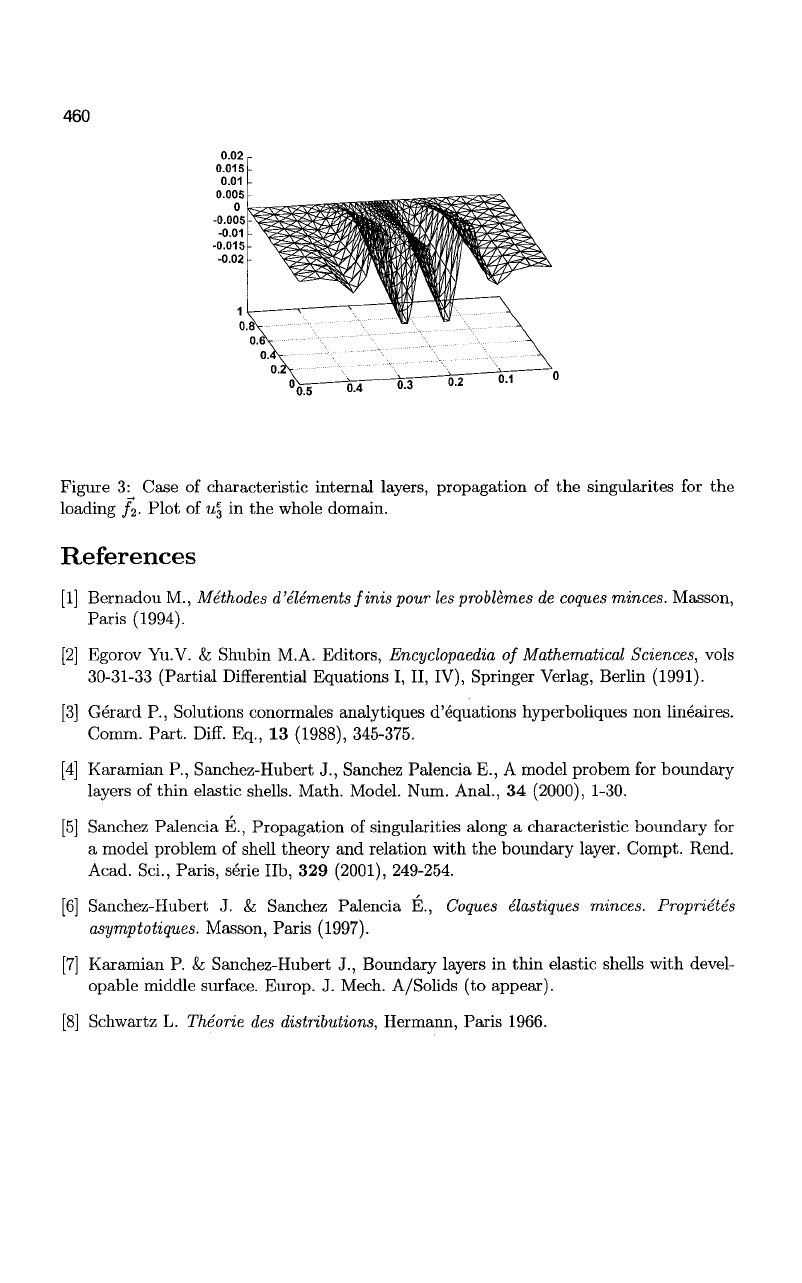
Figure 3: Case of characteristic internal layers, propagation of the singularites for the

loading /

. Plot of u| in the whole domain.

References

[1] Bernadou M., Methodes d'elements finis pour

les

problemes de

coques

minces. Masson,

Paris (1994).

[2] Egorov Yu.V. & Shubin M.A. Editors, Encyclopaedia of Mathematical Sciences, vols

30-31-33 (Partial Differential Equations I, II, IV), Springer Verlag, Berlin (1991).

[3] Gerard P., Solutions conormales analytiques d'equations hyperboliques non lineaires.

Comm. Part.

Diff.

Eq., 13 (1988), 345-375.

[4] Karamian P., Sanchez-Hubert J., Sanchez Palencia E., A model probem for boundary

layers of thin elastic shells. Math. Model. Num. Anal., 34 (2000), 1-30.

[5] Sanchez Palencia E., Propagation of singularities along a characteristic boundary for

a model problem of shell theory and relation with the boundary layer. Compt. Rend.

Acad. Sci., Paris, serie lib, 329 (2001), 249-254.

[6] Sanchez-Hubert J. & Sanchez Palencia E., Coques elastiques minces. Proprietes

asymptotiques. Masson, Paris (1997).

[7] Karamian P. & Sanchez-Hubert J., Boundary layers in thin elastic shells with devel-

opable middle surface. Europ. J. Mech. A/Solids (to appear).

[8] Schwartz L. Theorie des distributions, Hermann, Paris 1966.

Singular perturbations going out of the energy space.

Layers in elliptic and parabolic cases

E. Sanchez-Palencia

Laboratoire de Modelisation en Mecanique

CNRS-Universite Pierre et Marie Curie

4 place Jussieu, F-75252 Paris Cedex 05 France

Email : sanchez@lmm.jussieu.fr

Abstract

We consider singular perturbation problems depending on a small parameter e.

The right hand side is such that the energy does not remain bounded as e

—>

Boundary layers bearing most of the energy and accounting for non-smoothness of

the limit appear. We consider problems which are elliptic for e > 0 in two cases :

the limit problem is elliptic and the limit problem is parabolic.

1 Introduction

Classical singular perturbation theory for symmetric variational problems in real Hilbert

spaces deals with the following situation : let V be a real Hilbert space and a and b two

bilinear symmetric forms on V satisfying :

\a(u,w)\

<C||ii|||H|, Vu,weV (1)

|6(u,w)|<C|H|||w||, \/u,weV (2)

a(w,w)>0, VweV (3)

a(w, w) = 0

=>•

w = 0 (4)

a(w,w) + b(w,w) > c\\w\\

Vw£V,

(5)

where J.|| denotes the norm of V and c, C are constants. Let / e V be the dual of V.

For e > 0 we consider the variational problems P

a{u',w) + e

b{u

,w) = (f,w) Viu e V,

/„,E „„\ _ If „„\ w,„ a T/ (")

461

462

which are well-posed in the Lax-Milgram framework, as the form in the left hand side is

coercive on V (but the coerciveness constant decreases as e

when e

—•

0).

To study the asymptotic behaviour of u

as e

—•

0, we note that (3)-(4) imply that

ll-llv. = «(•>•), (

)

is a norm on V. Let V

be the completion of V with respect to this norm. Clearly

VCV

, 7'CK, (8)

with dense and continuous embeddings. Clearly / e V but it does not necessarily belongs

to V£. When this happens, the "limit problem" P

ueV

()

\a(u,w) =

{f,w),

V»eV„,

is a Lax-Milgram problem. The main result in this theory

([10],

[6]) is given by :

Theorem 1.1 Under the previous hypotheses, let u

be the solution of (6).

i) Iff eVi, then

—>

u strongly in V

, (10)

where u is the solution of 9.

ii) If f i V'

, the energy

i[a(u

) + e

6(«

.«

)]

of the solution u

tends to +oo as e

—•

Proof.

The part i) is classical (see for instance [6]). Its proof is analogous to that of

Theorem 2.4 hereafter. The part ii) is a complement (the proof is easy and may be seen

in [4]) which shows in particular that the condition / 6

is necessary to keep the energy

bounded. •

In this paper we consider examples of singular perturbations with / ^ V'

involving

problems which are elMptic for e > 0 and either elliptic or parabolic for e = 0, i.e. the

limit problem (9) is either elliptic or parabolic, but of course, it does not make sense

as a variational problem as / 0 V'

. The physical motivation for studying such kind of

problems comes from the thin shell theory. In the case when the middle surface, with

the kinematic boundary conditions is geometrically rigid, which furnishes the hypothesis

(4).

In that case the limit problem (9) is elliptic, parabolic or hyperbolic according to the

nature of the points (elliptic, parabolic or hyperbolic) of the middle surface of the shell.

Moreover, in shell theory the space V

is "very large" (going in certain cases out of the

space of distributions, see for instance [4]) so that its dual

is "very small" and "usual

loadings /" do not belong to it.

We consider here several model examples where / ^

because of a singular behavior

along a curve. It then appears a strong layer phenomenon along that curve : energy con-

centrates along it and grows without limit as e

—>

0. In order to study that phenomenon,

463

we perform a dilation of the normal coordinate to the curve ; the problem P

becomes a

new one, noted V

in the new coordinate system. The dilation is obviously anisotropic,

and even if P

and V

are equivalent for s > 0, the asymptotic behaviour of their energies

is different : boundedness deals with different terms of the expression of energy. With

an appropriate choice of the scaling of the dilation, the "new energy" remains bounded,

and we have a "limit problem" Vo of V

. This process, which is specific to each problem

and each loading, may be interpreted in terms of the method of inner and outer matched

asymptotic expansion (see for instance [1] and [5]). P

and V

are then the expressions

of the exact e > 0 problem in the outer and inner variables respectively, whereas

and

Vo are the outer and inner limits respectively. We emphasize that convergence is proved

for V

and Vo- The questions of the convergence of P

and P

is open for / £

In any

case,

if convergence holds, by virtue of Theorem 1.1, its topology is certainly weaker than

the energy one.

In Section 2 we consider an example where the limit problem is elliptic (or microlocally

elliptic along the singular curve, Remark 2.6). Problem P

(6) is variational, whereas P

(9) with / $ V'

is not. Nevertheless, both problems enter in the framework of trans-

position solutions of Lions and Magenes [7]. The limit problem Vo describes the loss of

regularity of the solution as e

—>

0. It is an elliptic problem in the variable transversal to

the singular curve, the tangential variable appearing merely as a parameter. Moreover,

it deals with equivalence classes of functions, denned in some sense "up to the regular

part", in other words, it describes the loss of regularity and nothing more.

In Section 3 we consider an example concerning the equation

-d>)u* = f, (11)

which is elliptic for e > 0 and parabolic for e = 0. We study singularities along the

characteristic a

= 0. We observe that the left hand side of (11) may be factorized so that

the equation is equivalent to the system

(-eA + d

)u = v, , .

(-eA-d

= f,

[U)

so that it amounts to the reiterated solution of two transport-diffusion problems (see for

instance [6] or [5]). We shall solve (11) taking advantage of the variational formulation

of the problem V

. In this case, the equation describing the asymptotic behaviour is a

genuine partial differential equation in yx, yi (the tangential coordinante is no longer a

parameter) accounting for the propagation of singularities along the characteristic. This

point is not considered explicitely here, but may be handled as in [9].

Section 4 is devoted to some complements concerning the case when the singularity

is not interior to the domain, but along a boundary with Neumann non-homogeneous

boundary conditions. The case of equation (11) is then somewhat analogous to that of

[8].

Notations are usual. In particular

(Q),

s € R are the Sobolev spaces (see definition

in [7]) and

Hg(Q.)

are the (closed) subspaces of the functions for which all the traces which

make sense vanish. The summation convention with respect to repeated indices is used.

464

2 Model problem for P

and

elliptic of order 4 and

2 respectively

In this section we consider elliptic problems in the domain

n = (0,7r) x (-1,+1) (13)

of the variable x

—

(x\,x-2). The bilinear forms are

a(u,w)= I diudiwdx, (14)

b(u,w) = / dijudijwdx. (15)

For e > 0 the energy space is

V = #

(fi), (16)

so that the classical formulation of problem P

(6) is :

(-A + £

) u

= / inH, (17)

v? = d

= 0 on 90, (18)

where d

denotes the normal derivative on the boundary dQ.

Obviously, using Poincare's inequality for w and its first order derivates in the bounded

domain

Q,,

we have

C\\w\\

>a{w,w) + e

b(w,w)>ce?\\w\\l, VweF, (19)

so that the coerciveness constant tends to 0 as £ tends to 0. Clearly ||.||

is a norm on V

and the completion of V with respect to this norm is

= H] (fi) (20)

and the classical formulation of P

(9) is

-Au = f in ft, (21)

u = 0 on

dQ,.

(22)

Let us consider the right hand side :

f{x

) = 8'(x

)F{x

), (23)

where 6 denotes the Dirac mass and F e I? (0,7r), so that

(f,w) = -fF(x

w(x

,0)dx

(24)

465

and using

the

trace theorem

\{f,w)\<\\F\\

\\d

w(.,0)\\o<C\W\. *>3/2.

(25)

In addition,

analogous inequality holds

for

< 3/2, so

that

f£H-

{Q)

V • ftIT

(n)

= V

(26)

and

we are in the

framework

Theorem

1.1 ii).

Let

consider,

for

e >

0 the

change

xi=yi

, x

= ey

(x)

=v%y).

(27)

Denoting

= d/d

i =

l,2,

(28)

the problem

(6)

with

(14), (15), (23)

becomes

the new one

Find

e Hi

(0,

TT)

(-1/e,

1/e)

such that

\fw

6 Hi

) : a

,w)

, w) +

, w)

($,

w),

where

(29)

OQ{V,W)

= /

vD2wdy

wdy,

ai(?;,w)

= /

w)dy,

(30)

(v,w)

= /

D\vD\wdy.

{<S>,w)

-jF(y

w(y

,0)dy

(31)

Remark

2.1

Obviously,

and w

(29) may be

extended with values

for | y

1/e,

i/iai

i/iey

are

considered

functions

on Bo

(0,

IT)

xH. As a

consequence, integrals

(30) may be

considered

We are now constructing an energy space adapted to the "limit problem"

(29)

—*

0. We observe that the left hand side

(29) with

only involves the first and

second order derivatives with respect

to y

so that

"ignores" additive functions

of y\.

The same holds for the right hand side. Let us denote by Hl(—l/e, l/e)/R the space of

the functions of class

vanishing for

| y

1/e defined up to an additive constant (i.e.,

for each

w S

Hl(—l/e, 1/e) extended with value zero for

| y

1/e, we consider the set

of functions which differ from

constant, and we consider this set as an element or

equivalence class). We then construct the space

((0, n)

• Hi

(-1/e, 1/e)

/R)

, (32)

466

where U denotes the union and C the completion with respect to the norm (constructed

from ao) :

\\v\\l =

j[{D

vf+{Dlvf]dy.

(33)

It should be noticed that the completion implies loss of the property that the functions

take the same value as y

tends to +00 and —00 (see an example in (44)).

We are now able to define the new limit problem Vo •

Find v eV such that ,„ ..

\/w e V : a

(v,w) = (*,w).

Obviously, by virtue of (30), (33) and Remark 2.1, the bilinear form at the left hand

side of (34) is continuous and coercive oh V. Moreover :

Lemma 2.2 The right hand side of (34) (see (31)) is a continuous functional on V.

Proof.

Immediate, as the functional is independent of additive functions of

y-y.

The

continuity follows from the trace theorem for dw/dy

in (for instance) /f

(—1,+1) and

integration in j/i. •

As a consequence, problem Vo has a unique solution in V (but as a function, it is only

defined up to an additive function of y\).

Remark 2.3 For each element v of

), we may construct its extension with value

zero to the strip

and consider it up to additive functions of y\. The equivalence class

constructed in this way will be denoted by v, and is obviously an element ofV.

We then have the convergence property :

Theorem 2.4 Letv

the solution ofP

(29) andv

the corresponding equivalence class

defined according to Remark 2.3. Then :

—»

v strongly in V, (35)

where v is the solution ofVo (34).

Proof.

Taking in (29) w = v

and using Lemma 2.2, we obtain the a priori estimates :

WWv < C, (36)

ai(v

)

1/2

<Ce-\ (37)

<Ce-

. (38)

Because of (36), there exists v* e V such that (at least for a subsequence)

-> v* weakly in V. (39)

467

Let us check that v* coincides with the solution v of (34). To this end, we fix w

belonging to Hfi (B

) for some E\. Then, after extending it with value zero, it also belongs

to H$ (B

) for e < £i and may be used as test-element in the corresponding V

. Using

(39) and the estimates (36)-(38) it follows from (29) that

ao(v*,w) = (^,w), (40)

but obviously, we may take in (40) w as well as w (see Remark 2.3) and according to (32)

the test-functions so considered form a dense set in V, so that v* is the unique solution

v of (34). In order to prove the strong convergence in (39) (which clearly holds for the

whole sequence), we write the expression

eV (v

, v

) +

6*0,2

, v

) + a

- v, v

- v), (41)

which, using (29) with w = v

, and w = v and (34) with w = v

is equal to :

<$,t/) + (<M)-2(<M

>, (42)

which tends to zero by virtue of (39). In particular, the term do of (41) tends to zero, and

the theorem follows. •

Let us now solve the limit problem (34). Obviously, it is an ordinary differential

equation in y

, with parameter y\. Denoting V = D^v and taking in (34) w obtained

according to Remark 2.3 from w €T> (R), we get

(-D

)

V = F(

)6'(y

(-l + Dl)V = F(

)6(y

) + C, (43)

where C is a constant. But V £ £

(R) is for

> 0 and y

< 0 solution of the differential

equation (43) with F = 0. It follows that C = 0 and V exponentially decreases at infinity.

It then follows from (43) that V(y

) is even and more precisely :

V(y

) =

-^e-M,

so that v, defined up to an additive function of

3/1,

may be represented by the odd function

in 2/2

v (y

) = ±

-'f± (1 - e-lwl) for y

< 0 (resp. y

> 0). (44)

Obviously, it is a piecewise smooth function tending to ±F{y{)/2 as y

tends to ±oo

having at y

= 0 a jump of D\ equal to —F(yi). It should be considered up to an additive

constant.

Remark 2.5 Obviously Theorem 2.4 amounts to a property of convergence of functions

°fyi> 2/2 v,p to an additive function ofyi or, equivalently, a convergence of the first order

derivatives with respect to y

. On account of the change (27) and the form of the limit

468

function in (44) the functions v

(xi,x

/s) converge (always up to an additive function of

Xi) to a step function of intensity —F(xi) at x

= 0. On the other

hand,

the limit problem

-Au = F(

)S'(x

), (45)

enjoys classical elliptic regularity of order 2 inside the domain

Q..

The right hand side

of (45), with F e L

, belongs to

(D,),

s < —3/2, as follows immediately from trace

theorems. Consequently, u locally belongs to

(Q),

r < 1/2, which is consistent with a

step function along x

= 0. Moreover, an asymptotic expansion of singularities analogous

to those used in

[9]

which are based on

[3],

shows that the leading order singularity of the

solution u is effectively a step at x

= 0 of intensity —F(xi). Theorem 2.4 may be seen

as a description of the asymptotic formation of that step as e tends to zero.

Remark 2.6 All the considerations of this section hold in the case where the laplacian

A in (17) is replaced by

/dx\. (46)

In the case (46) the limit problem is no longer elliptic, but the differentiations are transver-

sal to x

= 0, so that it is microlocally elliptic of order two for £ = (0,1), i.e. along the

considered singularity. Microlocal regularity theory then holds classically [2] and all this

section, including Remark 2.5, holds.

3 Model problem for singularities along the charac-

teristics of the limit problem, P

elliptic of order 4,

Po parabolic of order 2

In the same domain Q as in section 2, we now consider

a(u, w) = I d\ud\wdx, (47)

b(u,

UJ)

= / dijudijwdx, (48)

f(x

) = F{x

)ff(x

), (49)

with some F €

(0,n).

For e > 0 the energy space is V = H

(Q) so that P

(6) is :

{-d\ +

)

= F (

) 6' (x

) in fi, (50)

= diM

= 0 on dSL (51)

Clearly ||.||

is a norm on V, and the corresponding completion is

K = L

((-1,+1),

; ^(0,0, (52)

so that the limit problem is P

-dlu

= F{x

)8'{x

) inQ, (53)

469

u = 0 oni! = ±1, (54)

which is elliptic in X\ with parameter x

or equivalently parabolic in (x\, x

) with double

characteristics x

= const. We note that the right hand side (49) is singular along the

characteristic x

= 0. Obviously, / in (49) is not in the dual V'

Let us consider for e > 0, the change

e = rf , x

= y! , x

= ny

, A = d/dy,,

(x) = -v" (y),

(55)

(56)

which is suggested by the fact that the solutions of Po (which are not variational) are of

the form S'(x2)V(xi).

Making the change in P

for the unknown and the test functions, this problem is

equivalent to V,, :

with

Find

£ Hi (B

), 5„ =

(0,TT)

(—1/77,+1/TJ)

such that :

Vw € H§

(Br,)

: a

(v", w) + rj

^ (v", w) + rfa

(v", w) = ($,

(v,w) = / DivDitudy + / D\vD\wdy,

Br,

J B

ai(v,w) = 2/ DiD2vDiD

wdy,

J B,

(v,w) = / DivDiwdy

(57)

(58)

and the right hand side of (57) is again (31). As in Remark 2.3, we always consider

functions of Hi (£?,,) extended with value zero to H

) but this time, we do not consider

equivalence classes. We then construct the space

[Jfl2(B,),

where C denotes the completion with the norm (see (58))

ao(v,vf

We then have :

Lemma 3.1 In V holds the equivalence of norms :

(59)

(60)

llt=(R

V2i

(0,ir)»

)

•

^((CTr^iH^R,,))'

(61)