Bemelmans J. et. al. (editor) Elliptic and Parabolic Problems: Rolduc and Gaeta 2001
Подождите немного. Документ загружается.

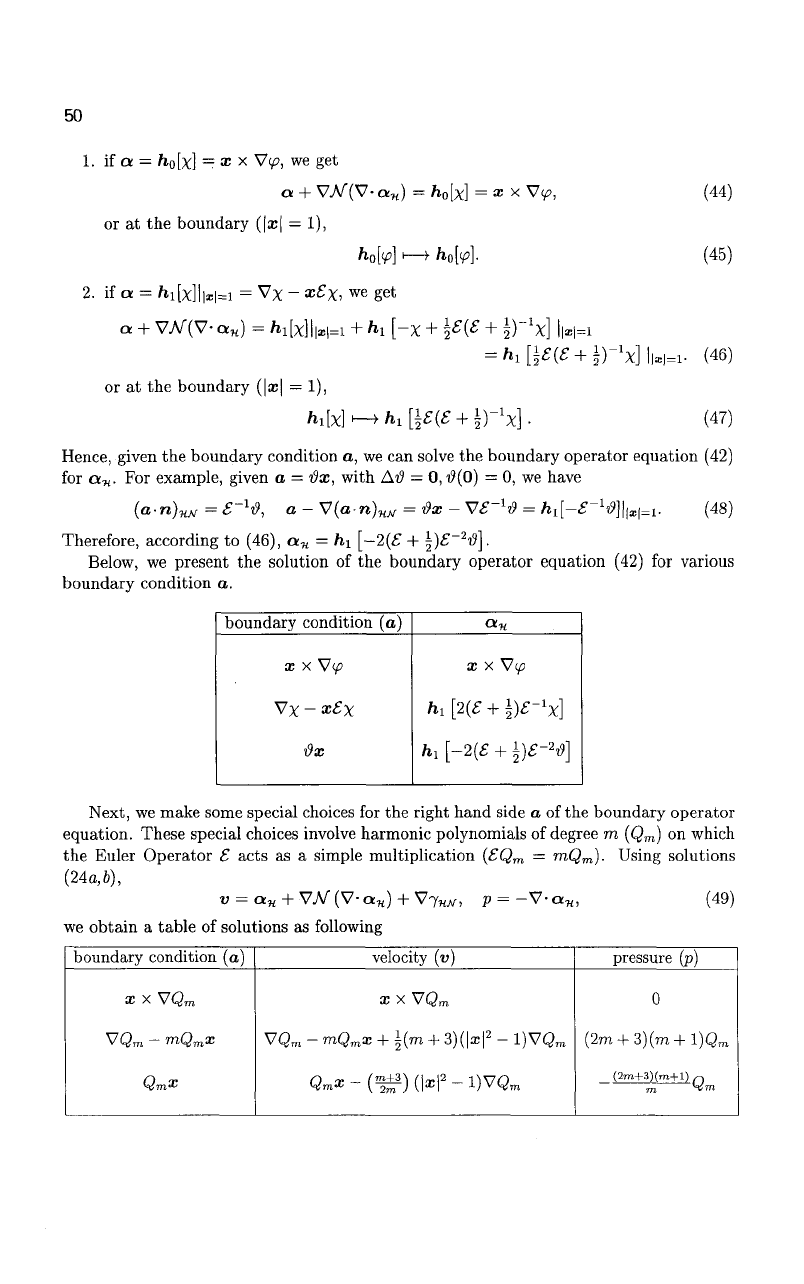
50
1.
if a = h
0
[x] = x x
V<p,
we get
a + VAf(V- a,) = /i
0
[x] = * x Vy, (44)
or at the boundary (|a;| = 1),
h
0
[ip]
i—s-
/i
0
[p].
(45)
2.
if a = /ii[x]||s|=i = Vx - x£x, we get
a + VAA(V-a„) = /»i[x]||»|=i + hi [~X + \£{£ + s)
_1
x]
l|.|=i
= /.i[|£(£ + i)-
1
x]lw=i. (46)
or at the boundary (|ai| = 1),
hxM _>/»![!£(£
+1)"
1
*].
(47)
Hence, given the boundary condition a, we can solve the boundary operator equation (42)
for a
H
. For example, given a = fix, with Afi = 0, i?(0) = 0, we have
{a-n)
nM
= £~
x
fi, a-V(a-n)
nM
= fix-V£-
l
fi = h
1
[-£-
1
fi]\
lxl=1
. (48)
Therefore, according to (46), a
n
= hi [-2(£ + \)£~
2
fi]-
Below, we present the solution of the boundary operator equation (42) for various
boundary condition a.
boundary condition (a)
x x Vip
Vx - x£x
fix
Oi-H
x x Vtp
hi [2(£ + \)£-
l
x
]
h
x
[-2(£ +
\)£~*fi]
Next, we make some special choices for the right hand side a of the boundary operator
equation. These special choices involve harmonic polynomials of degree m (Q
m
) on which
the Euler Operator £ acts as a simple multiplication (£Q
m
= mQ
m
). Using solutions
(24o,6),
v = a
7i
+ VAf{V-a
n
) + Vj
nN
, p = -V-a
M
, (49)
we obtain a table of solutions as following
boundary condition (a)
x x VQ
m
VQ
m
- mQ
m
x
WmX
velocity (v)
x x VQ
m
VQ
m
- mQ
m
x + \{m + 3)(|ai|
2
- l)VQ
m
QmX - (*j£) {\xf - l)VQ
m
pressure (p)
0
(2m + 3)(m+l)Q
m
(2m+3)(m+l) p

51
'Any' solution
(v,p) of the
homogeneous SBVP
in a
ball
is a
linear superposition
of
the elementary solutions. Remarks similar
to
those after
the
table
in
section
3.1.1.
apply
here
as
well.
For neat (functional) analytic considerations (also
for the
general case)
we
refer
to our
forthcoming paper
(see [5]).
References
[1] X.X.
SHENG
and
W.M.
ZHONG,
General Complete Solutions
of the
Equations
of
Spatial
and AxiSymmetric Stokes Flow. Quaterly Journal
of
Mechanics
and
Applied Mathematics
44 (1991), p.537-548.
[2] B.S.
PADMAVATHI,G.P. RAJA
SEKHAR,
and T.
AMARANATH,
A Note on Complete General
Solutions
of
the Stokes Equations. Quaterly Journal
of
Mechanics
and
Applied Mathematics
51 (1998), p.383
- 388.
[3]
R.
TEMAM,
Navier-Stokes Equations. Amsterdam: Elsevier Science Publishers B.V. (1984)
526pp.
[4]
I.
STAKGOLD,
Green's Functions
and
Boundary Value Problem. Toronto: John Wiley
&:
Sons,
Inc. (1998) 689pp.
[5]
J.
DE GRAAF,
D.
CHANDRA,
and R.
DuiTS,
On A
Boundary Operator Equation
For
Stokes
Flow,
(to
appear).

Local stability under changes of boundary conditions
at a far away location
M. Chipot, A. Rougirel
Institut fur Mathematik,
Universitat Zurich,
Winterthurerstr. 190,
CH-8057 Zurich, Switzerland
Abstract
We study the asymptotic behaviour of the solution to lineax and nonlinear
parabolic problems in cylindrical domains becoming unbounded in one or several
directions. In particular we show the local stability of the solutions under changes
of boundary conditions at a far away location. This generalizes a previous work in
which the data depended only of the cross section of the domain.
1 Introduction
In many physical situations the mathematical analysis starts after noticing that the cylin-
drical domain where the phenomenon is taking place being large in one direction, one can
consider only what happens in a cross section reducing the difficulty by one dimension.
We have justified rigorously this reasoning for elliptic and parabolic problems in [3], [4],
[5],
[6], We refer to [2] where others differential equations are involved, for a systematic
treatment of this question.
Let us give a simple example illustrating our results for evolution problems. Suppose
that
n, = (-£,£)x(-l,l) (1.1)
where £ is a number that we will let go to +oo. Let U( be the weak solution to the
parabolic problem
d
t
u
t
- Au
e
= f(t, x
2
) in (0, T) x Q
e
,
u
t
{t, x) = 0 on (0, T) x dtt
e
, (1.2)
u
t
(0, x) = u
0
(x
2
) in tie,
(we denote by x = (£1,2:2) the points in R
2
, dSli denotes the boundary of
Clt).
Our data
/ =
f(t,x
2
),
u
0
= uo(s
2
) are depending only on the section of fi^— i.e. are independent
of Zj. So it is reasonable to suppose that in a neighbourhood of x\
—
0, ui is more or less
52

53
independent of x\. This should be especially true for £ large. Then, if it is the case, in
the neighbourhood of 0, u^ ~ «„, where
Uoc
is the solution to:
d
t
u
x
- aP.tioo = f(t, x
2
) in (0,T) x (-1,1),
u
oo
(f,a;) = 0 on (0,T)
x{-l,l},
(1.3)
Uoo(0,
x) = ^0(2:2) in (-1,1).
More precisely, we have proved (see [4] Theorem 2.1 or [2] Theorem 9.2) that, for any
given £
0
> 0,
u
e
->
Woo
in L°°(0,T;L
2
{Qe
o
)) n L
2
(0,T;H\Ct
lo
)),
with a speed larger than any power of l/£. In this paper, we would like to show in addition
that the independence of the source term and the initial condition with respect to £ and x\
is,
in some sense, not essential. Let us explain this idea reformulating the above problems
in the following manner. Let u\ be the solution to
d
t
u) - AuJ = ft(t, x) in
(0,
T) x Q
t
,
u\{t,x) = 0 on (0,T) x dn
e
, (1.4)
u\(0,x) = uo
t
(x) in Q(,
and uf be the solution to ,
f d
t
u\ - !\u\ = f
t
{t, x) in
(0,
T) x Q
t
,
u
2
{t,x) =
Q
on (0,T)x
{-£,£)
x {-1,1},
d
n
u
2
e
{t,x) =0 on (0,T) x {-£,£}. x (-1,1),
^
u\(0,x) = Mo,(
;r
) in n^.
Then we will prove, under certain assumptions, that
u\ - u? -> 0 in L°°(0,r;L
2
(^
0
)) nL
2
(0,r;if
x
(n
£o
)).
Note that now the source term and the initial condition depend on £ and x\. This setting
generalizes the previous one since, if, in (1.5), fi{t,x) = f(t,x
2
), u
0l
(x) = 110(0:2), it is
clear that uj = u^ where u^ is the solution to (1.3). Moreover this local stability result
is intuitively clear since the problems whose solutions are u\ and u
2
differ only on the
vertical parts of <9fi which are rejected at infinity.
We will study more general problems than (1.4), (1.5). In the next section we will
consider the case of a general parabolic linear problem. In section 3 we will consider a
quasilinear case.
2 The linear case
Let us consider a bounded open set of R" given by
n
e
=(-£,£)P xu) (2.1)
(1.5)

54
where w is a bounded open subset of
R™
p
, 1 < p < n. For x = (x\, ...,x
p
,x
p+
i, ...,a;
n
) €
R" we will set
Xi = (xi,
•••,x
p
),
X
2
= (x
p+
i,
...,x
n
).
We denote by
du>
0
a measurable subset of du and we will assume altough it is not essential
that
|dw
0
| > 0. (2.2)
Then we set
r
0
=
(-e,ey
xduo,
r
1
= r
0
ud(-£,£)
p
xu>
(2.3)
and
H^(Qi;Ti) = {ve H
1
^) ; v = 0 on I\} i = 1,2.
We equip these spaces with the norm (recall (2.2))
IM
2ini
= {j[
\Vv(x)\dx}
1/2
.
(2.4)
Let Vf, Vf be two closed subspaces of
HQ(QI;
F
0
) equipped with the norm (2.4) such that
H^Qf, r\) C V
e
\ V? C Hl(Sl,; T
0
). (2.5)
Note that V} and Vf contain functions not vanishing on (—£,t)
p
xflw\ <9w
0
.
If
fteL^TiH^Slt-.To)'), (2.6)
where ^(fi^; T
0
)' denotes the dual of Hg(Q
e
; F
0
), then for a.e. t e (0,T), the restriction
of ft(t) to V? defines a continuous linear form on VI, i = 1,2. Thus we get a functional
that we still label ft satisfying
f
e
eL
2
(0,T;Vi').
(2.7)
We will need in the following to control the growth of fe at infinity that is why we impose
the condition
lAlLWiffiffW) ^
c
(
r
+ !)
W>0
- (
2
-
8
)
where c and a are positive constants. Remark that (2.7)-(2.8) imply that it holds that
I/*ILW;V?')<
C
(^
+
1
)
W>0,Vt
= l,2. (2.9)
Moreover, as already explain, we allow also a dependence of the initial condition u
0t
with
respect to £ and X\ but we must add the assumption
Kkn
(
<c(£
Q
+ l) W>0. (2.10)
We consider then coefficients
aij = aij(t,x) eL°°((0,T) x R
P
x ui) Vi,j = 1,
...,n.
(2.11)
To simplify the notation we set
A = A(t,x) = (
aiJ
)
iJ=l n
(2.12)

55
and we suppose that for some A, A > 0 it holds that
|A(t,a;)|<A a.e. t 6
(0,T),
a.e. x e R
p
x w, (2.13)
^(*,z)£-£> A|£|
2
a.e. te (0,T), a.e. i6f x w, V£ e R". (2.14)
(|£| denotes the euclidean norm of f, | | the norm of matrices subordinated to the euclidean
norm of K.".) Let us set
ffHCri^.V?') = {ve L
2
(0,T;Vi)
I
«* £ L
2
(0,T;V7')}
•
(2.15)
For /f G L
2
(0, T;
.Hp
(fi<; r
0
)'), u
0l
6 L
2
(f2^) there exists a unique u\ solution to
( uieH\0,T;Vi,Vi>),
f
t
(u\,v)
2
+ J A(x,t)Vu\Vvdx =
(fe,v) mV'(0,T),VveVi,
I u\{0,-)=u
0t
{-) mL
2
{Q
e
).
(We refer the reader to [7], [1] for a
proof.
X>'(0,T) denotes the usual space of distribu-
tions on (0,T), (•, -)
2
the usual L
2
(f^)-scalar product.) Remark that if
du>
0
= dus then
H^QfJi) = H£(£li). Hence, if we choose p = 1, V? =
H^{Q
t
),
V
e
2
= ^(f^To) then
(2.5) holds and for i = 1 (resp. i = 2), (2.16) is the weak formulation of (1.4) (resp.(1.5)).
Then we would like to show
Theorem 2.1 Under the above assumptions, in particular if
(2.5),
(2.8) and (2.10) hold
then,
for any
IQ
> 0, any r > 0, there exists a constant C independent of I such that
Q
\u\
-
U?|L°°(0,T;L2(J1
<0
))
+ \u\ ~ U?Ua(o,T;ffi(n<
0
)) ^ J
r
'
(
2
'
17
)
To prove this theorem we will need several lemmas. Let us first show
Lemma 2.1 (Poincare Inequality) Let v be a function of
HQ(£II;TO).
Then there exists
a constant C
—
C(w) independent of
£
and v such that
\v\
2
,n
t
<C\\V
X2
v\\
2flt
- (2-18)
(V^
2
= {d
x +1
, •••jC'x„).) See [3] for a
proof.
We can now estimate u\. We have indeed
Lemma 2.2 Leti G
{1,
2} andu\
be
the solution to (2.16). Then there exists C = C(\,p)
a constant independent of
£
such that
M
2
L»
(
„,
T;1
»
(nt))
+
[P4\\ln
t
dt
^
C
(
fa
+
!)•
(
2
-
19
)

56
•il^
+ / AVu\Vu\dx = </«(0,«j> < l/J(*)|.||Vujl|
w
J SI/
Proof of Lemma 2.2. We take v = u\ in (2.16). We obtain for a.e. t £ (0,T)
1 d.
where | |» denote the dual norm in VI'. Using (2.14) and the Young inequality we derive
5|I«JIU
+
A||V«JI|^<^I/,I;
+
^|IV«JI|^
Integrating on (0, i) we obtain for a.e. t
\v-m\ln
e
+ *J
o
||V*4l|2,„,d*<
luoM^
+ lflMl^dt- (2.21)
With (2.9), (2.10) we obtain
min(l,
A)
||ui(t)|^ + ^IIV^HU*} ^
C
^ +
1
(2.22)
and the result follows.
Next we show
•
Lemma 2.3 Let u\ be the solutions to (2.16), i = 1,2. Let k be an integer k > 1. Then
there exists a constant C = C{\, A, k,n) independent of I such that
\u\ ~
u
2
e\l°o(o,T;L2(n
l/2k+1
))
+ J
||V(MJ
- u\)\
2
on dt
<T2[\W«-<)\\l
ni/
Jt- (2-23)
Proof of lemma 2.3. In R
p
we consider a smooth function p = p{X{), 0 < p < 1 such that
P=l on
(~\'l)
'
(
2
-
24)
p = 0 outside (-1,1)", (2.25)
\V
XlP
\
< C, (2.26)
where C is some positive constant. Then, for a.e. t, for any t\ € [0,£\, it holds that
x = (X
1;
X
2
) H. (u\ -u
2
e
)(t)p
2
{f) e H^QcT,). (2.27)

57
According to (2.5), this function is a suitable test-function for the problem (2.16) for
i = 1,2. Taking the difference of the equations of u\ and uj with v equal to the above
test-function, we derive
(jM ~
U
^' ^
~~
U
^
p2
)
+
J
AV
^
e
~
U
?)
V
{(
U
< "
u2
e)p
2
}
dx
=
°-
(
2
-
28
)
According to [4], {u\ - uj)p € #H0,T;#
0
1
(^,r
1
),.ff
0
1
(ft6r
1
)') and it holds that (in
L
2
(0,T))
^(uj-u?)p,«) = (-(ui-u?),t;p) Vue^cn/.ri). (2.29)
Thus (2.28) becomes
l_d_
2~dt
\{u\
- u\){t)p\l
ni
+ J AV{u\ - u
2
)V{{u\ - u
2
)p
2
}dx = 0 (2.30)
Jn,
in,
in L
1
(0,T). Performing the derivation in space in the above integral, we get
(u\
- uj)(t)p\l
ni
+ f AV(u\ - u?)V(u] - u
2
)p
2
dx =
Jn,
- / AV{u\ - u\)Vp{u\ - u
2
)pdx. (2.31)
1 Jn,
2d* • .in,
2
In,
Using (2.13), (2.26) and the the Young inequality ab < ^o
2
+ eb
2
, this last quantity
bounded by
2AC
— / \V{u\ - uj)\\u] - uj\pdx < —-5- / \V(uj - uj)\
2
dx + e \u\ - uj\
2
p
2
dx
1 Jn, "1 Jn,, Jn,,
in,
«i Jn
h
Jn
tl
and, with the Poincare inequality of Lemma 2.1, also by
^f / \V{u\-u
2
)\
2
dx + C'ef \V
X2
{u\-u
2
l
)\
2
p
2
dx
"1 Jn
h
.
Jn
tl
where C" is a constant independent of
£—
recall that p is independent of X^. Going back
to (2.31) and using the ellipticity condition (2.14), we obtain choosing e = A/2C"
±-\{u\ - u
2
)(t)p\l
nt
+ X f |V(uJ - uj)\
2
p
2
dx < J / |V(uJ - u
2
)\
2
dx.
at
Jn,
<-i
Jn
h
Integrating this inequality between (0, t), it holds that, since p = 1 on
fi^/2,
H
-
«?)(*)ll,n,
l/a
+
X
f [
l
V
K
-
«/)l
2(fa
^ S f I
l
V
^ " ^l^'
1
Jo
Jn,
l/2
L
i Jo Jn
h
The inequality (2.23) follows easily choosing l\ = £/2
k
. This completes the proof of
Lemma 2.3.
•

58
Proof of Theorem 2.1. From (2.23) we derive
\u\
- u
2
Al°°(o,T;mn,
/2k+1
))+ J ||V(uJ - i
= J
{l««
"
u
2
Al~
{0
,
T
.,
LH
n
t/2k))
+J
o
||V(ui
-
t*?)!!^*]
Iterating this inequality we obtain— using (2.23) at the last step
\\V(
u
l-
u
Mln
lf2k+1
dt
<-
Now, by Lemma 2.2,
jr
T
iiv(«j-«?)ii*
ini
dt<2jf
T
iiv«iii^dt+2jr
T
iivu?ii^dt
<C(^
2a
+ l).
Then we derive from (2.32) that it holds that
f
T
2 l
2a
+1
l«J " u
2
e\l~(o,T;mn
l/2k+l)
) + ^ ||VuJ - u
2
t
\\
2n
^
h+
dt < C-^—^
where C = C(X, A, k,p, n). We select then k such that 2(/c + 1)
—
2a > 2r and we choose
£>1 large enough such that £/2*
+1
> £
0
. We obtain
+ \u
t
- u
t
\
L
2/
0tT
.
H
im )) < -^r (2.33)
C
^lL~(0,T;L2(!!,
0
)) "I" !"£ ~ '"/ lL
2
(0,r
;
if»(«/„)) - ^
where C = C(A, A,p,n). Note that, since uj
—
u| is vanishing on a lateral part of f^
0
, the
L
2
-norm of the gradient is equivalent to the i7
1
(Q£
0
)-norm— see also Lemma 2.1. This
completes the proof of Theorem 2.1.
•
3 The quasilinear case
In this section we consider again
fi,
=
(-«,<fxucr. (3.1)
We set
F
0
= {-£,£)" xdu, (3.2)
Hl(n
e
;
r
0
) = {ue H
l
{U
e
) ; v = 0 on T
0
} (3.3)

59
and we consider
Vl, V
2
two
closed subspaces
of
Hg(Q,f,
T
0
)
equipped with
the
norm
(2.4)
such that
H^Qe)
c V
e
\ Vl C
Hl{Q,
t
- r„).
(3.4)
Let
us
denote
by atj =
aij(t,x,u),
i,j =
l,...,n
Caratheodory's functions
i.e.
functions
such that
(t,
x) i->
a
it
j(t,
x,
u)
is
measurable
VM
G
R, (3.5)
u
H->
a
it
j(t,x,u)
is
continuous
a.e. (t,x)
G
(0,T) xW x
ui.
(3-6)
We write
the
matrix
of
the coefficients under
the
form
(a
h3
)=A = A(t,x,u
)
=^2u))
™
where
Ai is a p x n
matrix
and A
2
is a (n
—
p) x n
matrix.
We
assume that
A
satisfies
the following usual hypothesis,
^••?>A|f|
2
V£GR",VUGR,
a.e. {t,x)
G
(0,T) xW x u, (3.8)
\A(t,x,u)\
< A
VaeR,
a.e.
(i,a;) G
(0,T)
xl'xu,
(3.9)
for
A,
A
> 0.
Furthermore
we
suppose that
\/u,v
G
R, a.e. (t,x) G (0,T)
xfxu
the
following holds
|A(t,s,u)-i4(t,a;,i;)| <Cw(|u-?;|), (3.10)
where w
is a
nondecreasing positive function
on R
+
such that
ds
L
o+
^
2
(s)
(3.11)
Under
the
assumptions (3.5), (3.6), (3.8),
(3.9) one can
show that,
for i = 1,2,
there
exists
u\
solution
to
f uleH'(0,T;Vl,Vl'),
(u'
t
,v)2+
I
A(t,x,u'
e
)Vu'lVvdx
Jn,
i(-i,-,*
• ,^-
v
-.-.-«--f-~
(312)
(f
t
,v) mV'(0,T),Vv€Vi,
{ u\(0,-)=u
0l
(-) mL
2
{n
e
),
for
u
Q[
G
L
2
(Q
e
)
and fe G
L
2
(0,T; ^(fi^To)')-
For an
existence proof
we
refer
for
instance
to
[1].
We
will also assume that (2.8), (2.10) hold
and
that
for
some
A' > 0 it
holds that
for
every
i =
l,...,p,
j,k
—
l,...,n
\d
xt
ai
d
(t,x,u)\<A'
a.e. (t,x)
G
(0,T) x W x u, \/u e R.
(3.13)
Under these assumptions
let us
show
