Bemelmans J. et. al. (editor) Elliptic and Parabolic Problems: Rolduc and Gaeta 2001

Подождите немного. Документ загружается.

Existence of non-steady flows of an incompressible,

viscous drop of fluid in a frame rotating with finite

angular velocity

M. Padula and V.A. Solonnikov

Department of Mathematics, University of Ferrara,

Via Machiavelli 35, 44100, Ferrara, Italy

Emails : slk@dns.uife.it ; pad@dns.uife.it

Abstract

We give an existence theorem of global non-steady solutions of the Navier-Stokes

equations governing flows of a incompressible viscous fluid totally bounded by free

surface. Specifically, we prove that, in the presence of surface tension, if a rigid

rotation admits a stable configuration in the sense of capillarity theory, then to

any suitably small initial perturbation to the shape and to the velocity field there

exists a unique global non-steady solution which eventually goes to the stable rigid

rotation as time goes to infinity, with exponential decay rate. Our proof is based on

a existence theorem of local regular solutions proved by [13], and on some a priori

estimates on the solution, uniform in time for weak norms of the solution in the

wake of [7]. The result does not assume smallness on angular velocity of the drop,

only smallness on initial data!

1 Introduction

Plateau's work inaugurated in 1863 the study of shape and stability of a drop driven by

rotation of surrounding liquid. Plateau's drop was pierced by a shaft and immersed in

a tank of liquid having almost the same density of the drop. The shaft was mounted

vertically and turning it, Plateau could bring the drop into rotation. The drop is driven

to rotate at an imposed angular velocity

UJ.

When increasing the rate of this rotation, the

drop progresses through a sequence of shapes, axisymmetric at first, then ellipsoidal and

two lobed, and then a most remarkable toroidal ring that remains intact for a short time.

Shapes and stability of isolated, self-gravitating masses rotating freely in space have been

much analyzed, e.g. by Maclaurin 1742 (perfect spheroids), Jacobi (1834) (ellipsoids),

and the equilibrium shapes are analogous to those found by Plateau.

1.1 Results on Equilibrium Shapes

In theory, the shape r

of the drop in rigid rotation would have been strictly set by the

balance of the capillary force that comes from surface tension of the curved drop surface,

180

181

and the gyrostatic pressure force which is exerted on the surface by the rotating liquid

owing to ordinary centrifugal force, under the constraint to have constant given volume

\ilt\. Mathematically, this balance is expressed, in spherical coordinates, by the functional

G(R,t) = a\r

\-^j

R5{y/

edS

\, (1)

where a represents the surface tension, p

is a Lagrange multiplier and Si is the unit

surface. For steady flows : G(R, t) = G(R). The basic flow is a steady flow characterized

by a shape f2(,, a gyrostatic pressure

pt,,

and a uniform rigid rotation with angular velocity

cj = u;e

, which is considered as a parameter. The fluid is supposed to be in equilibrium

in the frame rotating with the drop, therefore the shapes are also called equilibrium

shapes. Several equilibrium shapes of rotating liquid drop are theoretically possible. The

equilibrium shape physically realizable is that which renders minimum the potential G.

In capillarity theory, the range of "stability" of the different shapes is investigated when

is varying, and it is shown that rigid rotational stable shapes exist, in correspondence of

a finite value of angular speed w, may be they are not unique ! Some interesting analysis

can be found, e.g., in [3], [1], [2].

The aim of this paper is to prove that the above said configurations, which are stable

in the class of small fluctuations in shape, are also asymptotically stable in the class of

non-steady flows with respect to small initial fluctuations in shape and in velocity inside

the drop.

We quote the papers [4], [6], [7], and [8]- [13] concerning the existence problem for the

full free boundary problem. In particular in [7], it is proved, for the first time, existence

of an unsteady regular motion of rotating liquid drop, exponentially decaying to a rigid

steady configuration corresponding to a given angular momentum. In that paper, it is

assumed smallness on the size of angular momentum. Here we intend to remove such a

restriction, at least for star-shaped domains. Precisely we prove the following.

Theorem 1.1 Let T

= 3fio be given by equation R = Ro (y) with R(y,0) = Ro(y),

y e S

Ro e C^Si), a e (0,1), and letv(x,0) = v

{x), with v

£ C

2+a

Moreover, let

Rf,

(w) satisfy the stability hypothesis of capillarity theory, that is Ri, is an

isolated local minimum for G (R) at fixed

u>,

and set v<, =

x x. Assume additionally

that

satisfies the compatibility condition

x x v

dx = xx v

dx,

J n

and let

|vo - vtlca+a^) + |.Ro - Rb\

*+°(Si) -

> (

)

with e sufficiently small. Then the fluid drop has a unique solution (R,v,p) defined in an

infinite time interval t > 0 and possessing the following properties : R (.,t) €

3+a

(Si),

v(.,t) € C

2+a

(ft

), p(.,t) 6 C

1+Q

(fi

(

), Vt > 0, where T

= dQ

is given by equation

182

.R = R(y,t), y € Si. The solution satisfies the inequality

sup |w

(.,t)|

,„

(n)

+ sup |w(.,t)|

c2+

„

(n)

+ sup \p{.,t)-p

cl+a(n)

te{0,T) te(0,T)

' te(o,T)

+ ™P ^ (••*) " ftlc+«(n,) ^

(no)

+ |#o - ifc|

»+.(s

))

~"»

VT G

(0. °°l.

(3)

where w = v

—

V&, iwrfi some constants 6 > 0 and c independant ofT.

We give the proof for star-like configurations. As it will be clear from the

proof,

our

result heavily relies on the assumption that the basic configuration R

renders minimum

the energy functional of capillarity theory. Therefore, in principle, our proof can be

enlarged to more general equilibrium configurations, like the toroidal ones.

2 Position of the problem

Let a drop of incompressible viscous fluid occupy a star-shaped domain

= {y G R

\y\ < R(y/\y\)}, r = 90. We wish to determine the configuration fit C R

, of prescribed

volume

47r/3,

admissible with a steady rigid rotation of angular velocity u;e

2.1 Equilibrium results

We remind that the energy functional, given by (1), must assume a minimum in R

Denote by Vgj the derivatives along the unit sphere S\, in spherical coordinates, and set

g = R

+ \V

R\ ,

OR 1 OR

~de

+ e

'^e'^^

n = (-V

R,R)

Vsj = e

— + e,

y/R*

+ \V

Also,

it holds :

= J

Ry/gdS\. The condition that R

is a minimum for G

implies, in particular, that it must be a stationary point for G, that is, for R = R

+ tp,

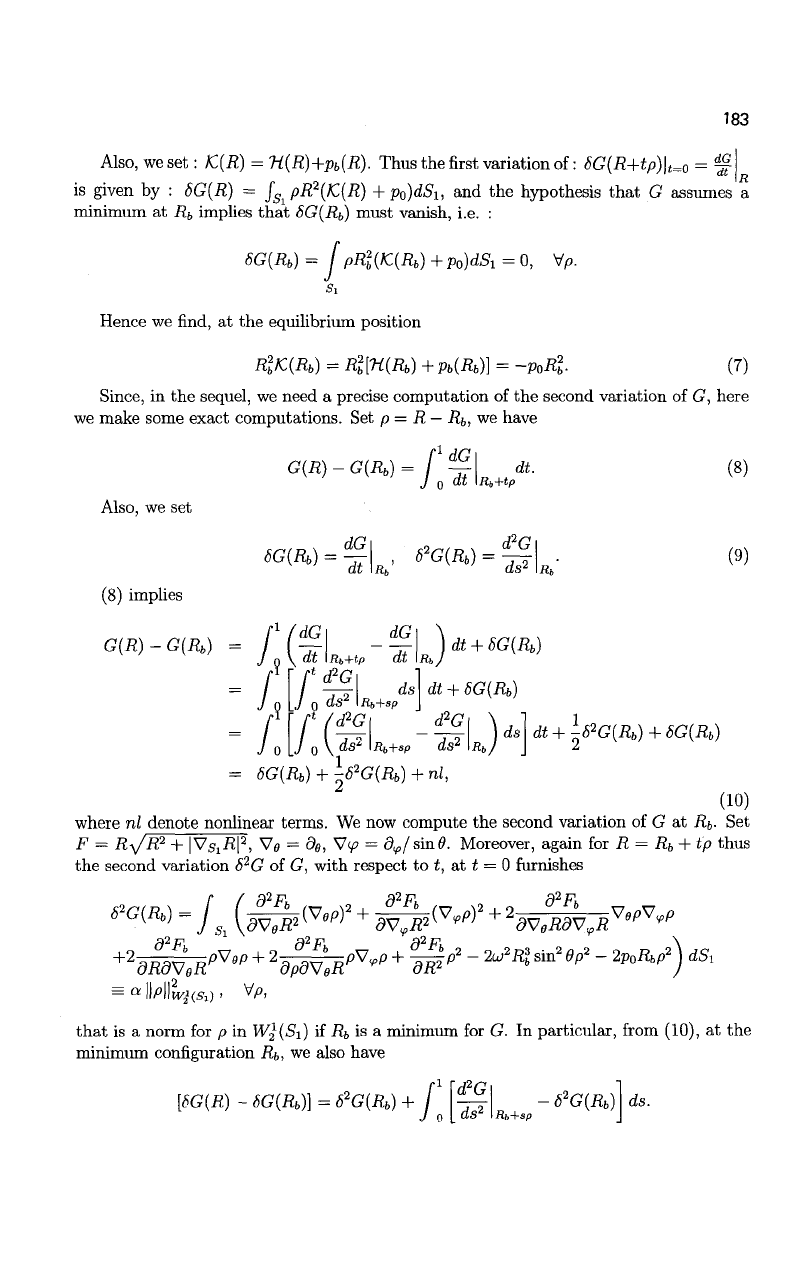
the first derivative of G(R) with respect to t at t = 0 must vanish, i.e. :

0 = 5G(R

) = ^-\ =

f {py/g + ^iRp + VR-VpildStl

\t=o

J sA V9 )

=° (

)

-— / pR*sin

6dSi\ -p

[ pR

\ .

2 J

=° J Si

=°

Integrating by parts, and owing the arbitrariness of p, we deduce the classical eigen-

value problem in unknown R

and po :

H{R

)+p

= 0, (5)

where the double mean curvature of the surface H(R) has the following expression :

1„ (V

R\ 2

™=a**

(-%-)-v?

(6)

183

Also,

weset: K{R) = H(R)+p

{R). Thus the first variation of: SG(R+tp)\

t=0

= ^

is given by : 6G(R) = J

(K.(R) +

)dSi,

and the hypothesis that G assumes a

minimum at R

implies that SG(R

) must vanish, i.e. :

SG{R

)

= J

Rl{K{R

)

)dS

Vp.

Hence we find, at the equilibrium position

RflC(R

) = R

[H{R

)

)]

= -p

(7)

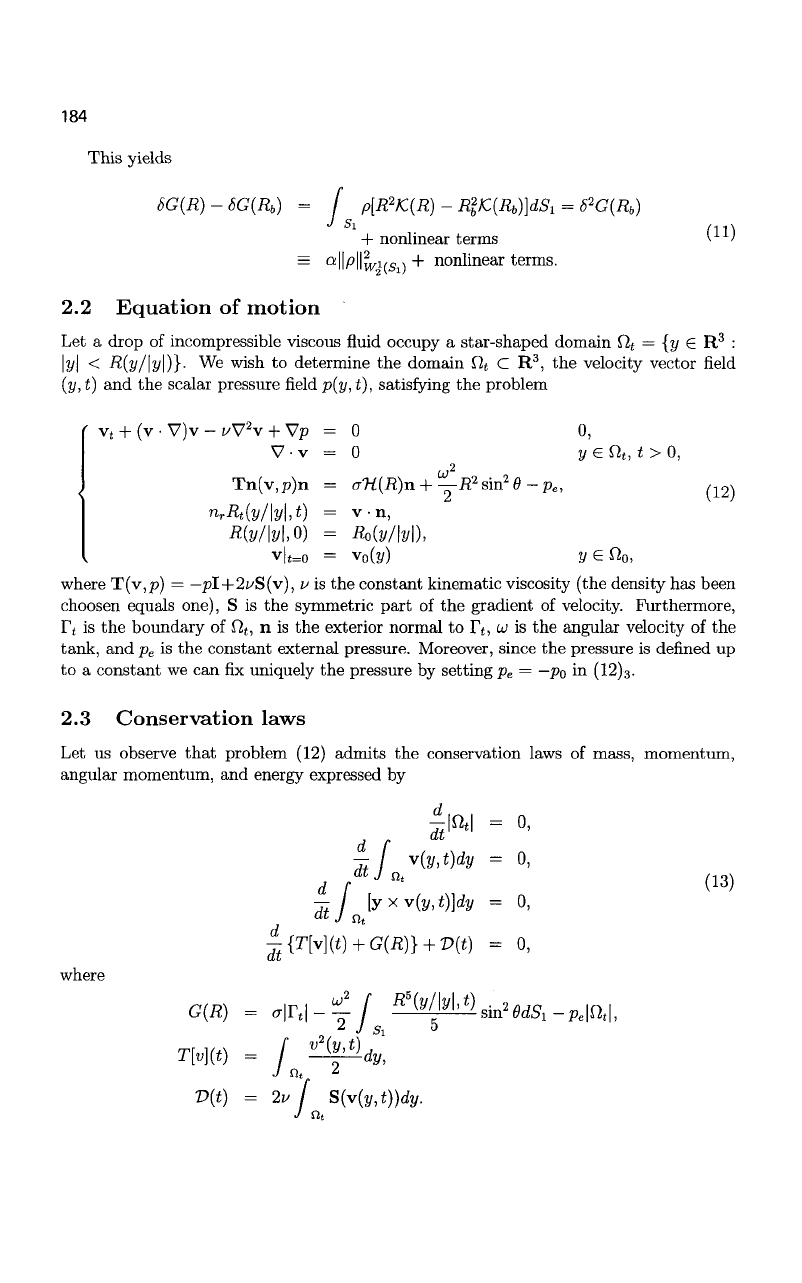
Since, in the sequel, we need a precise computation of the second variation of G, here

we make some exact computations. Set p = R

—

, we have

G(R) - G(R

)

J o

dG\

dt.

Also,

we set

dt \R

+tp

SG(R

)

= ^§\ ,

G(R

)

= ,

dt \R

(8)

(9)

(8) implies

G(R)-G(R

) = J (

fdG\ _dG\

dt \R

+tp dt \R

dt + 5G{R

)

J o

(£2.

o V

ds dt + 6G{R

)

- p J

G\ d

]dg

+sp ds

dt + -8

G{R

) + SG(R

)

= SG{R

) + -8

G{R

) + nl,

(10)

where nl denote nonlinear terms. We now compute the second variation of G at R

. Set

F = R^/R? + \V

, V

= d

, V<p = dy/sw.6. Moreover, again for R = R

+ tp thus

the second variation S

G of G, with respect to t, at t = 0 furnishes

F f)

{avj?^

2 +

WJT

e^R

pVeP + 2

dVeR

pVvP

2Lj2R3b

Sln2 9p2

2poRbp

)

dSl

ORdVeR

,),

Vp,

that is a norm for p in

W^iSi)

if R

is a minimum for G. In particular, from (10), at the

minimum configuration R

, we also have

[6G(R) - SG(R

)\ =

G(R

)

+ / f^?| -

G(R

[

J o

as \ Rb+sp

ds.

184

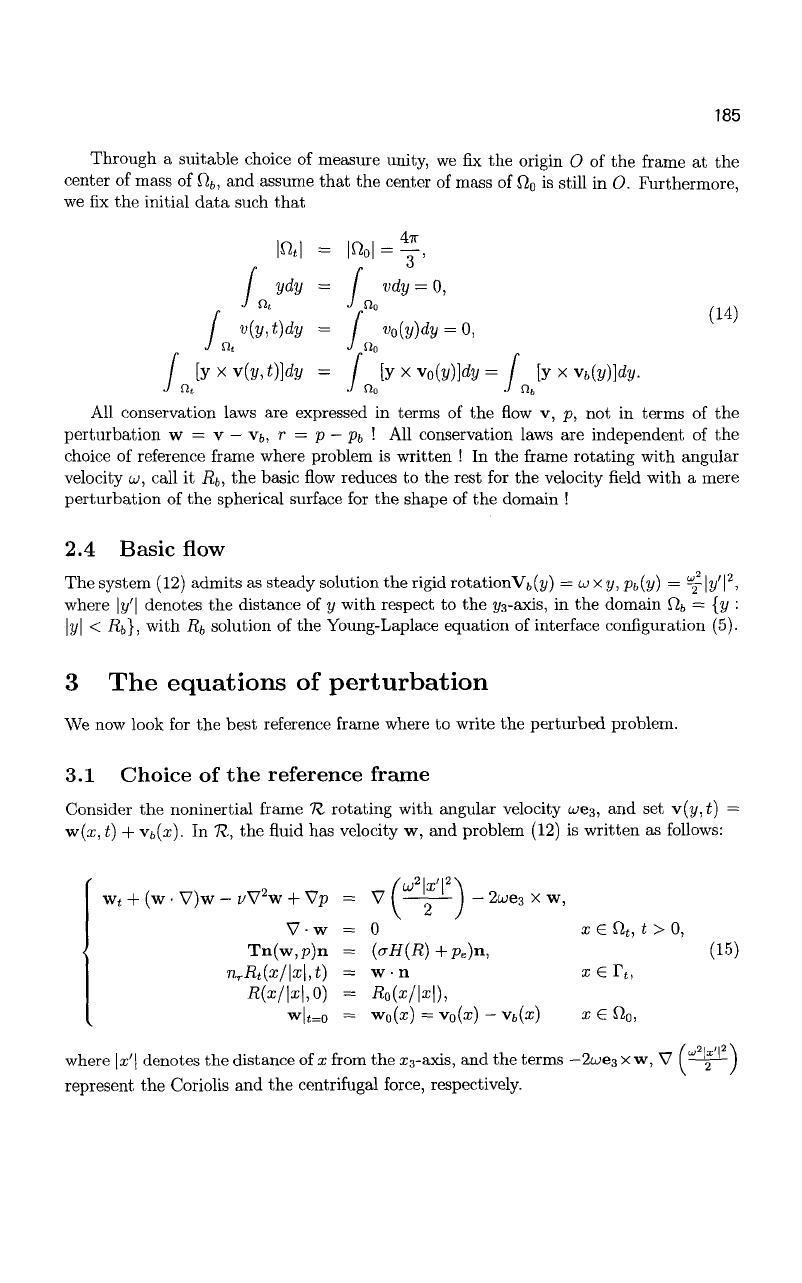
This yields

SG(R)-SG(R

) = /

K.(R) - R

IC(R

)]dS

= S

G{R

)

fill

+ nonlinear terms v /

IIPIIM'1(S

) + nonlinear terms.

2.2 Equation of motion

Let a drop of incompressible viscous fluid occupy a star-shaped domain Q

= {y € R

\y\ < R(y/\y\)}- We wish to determine the domain Q

C R

, the velocity vector field

(y,t) and the scalar pressure field

p(y,t),

satisfying the problem

' v

+ (v

•

V)v - i/V

v + Vp = 0 0,

•

v = 0 y € fit, t > 0,

Tn(v,p)n = aH{R)n + —

sin

6 - p

, ^

Rt(y/\y\,t) = vn,

R(y/\y\,0) =

(y/\y\),

=o = v

(y) y G Q

where T(v,p) = —

pI+2vS(v),

v is the constant kinematic viscosity (the density has been

choosen equals one), S is the symmetric part of the gradient of velocity. Furthermore,

is the boundary of Q

, n is the exterior normal to Tt,

is the angular velocity of the

tank, and p

is the constant external pressure. Moreover, since the pressure is defined up

to a constant we can fix uniquely the pressure by setting p

—po

(12)3.

2.3 Conservation laws

Let us observe that problem (12) admits the conservation laws of mass, momentum,

angular momentum, and energy expressed by

ij^vMv = 0,

[yxv(

= 0,

(13)

where

-{T[y](t) + G(R)} + V(t) = 0,

G(R) = a|r

| - £ j

«M sin'

dSl

TM(t)

= f ^dl

V(t) = 2v I 8(v(y,t))dy.

fit

185

Through a suitable choice of measure unity, we fix the origin 0 of the frame at the

center of mass of to

, and assume that the center of mass of to

is still in 0. Furthermore,

we fix the initial data such that

lai =

l^o|

= y,

««

JJk

(14)

ydy = / vdy = 0,

l v{y,t)dy = / v

(y)dy = 0,

/ [y x v{y,t)]dy = / [yxv

(y)]dy= [yxv

{y)]dy.

J fit J f!

J f!

All conservation laws are expressed in terms of the flow v, p, not in terms of the

perturbation w = v

—

V(„ r = p

—

! All conservation laws are independent of the

choice of reference frame where problem is written ! In the frame rotating with angular

velocity w, call it R

, the basic flow reduces to the rest for the velocity field with a mere

perturbation of the spherical surface for the shape of the domain !

2.4 Basic flow

.'|2

The system (12) admits as steady solution the rigid rotationV

(y) =

Pb(y)

= \

where \y'\ denotes the distance of y with respect to the 3/3-axis, in the domain Q

= {y :

\y\ < R

}, with R

solution of the Young-Laplace equation of interface configuration (5).

3 The equations of perturbation

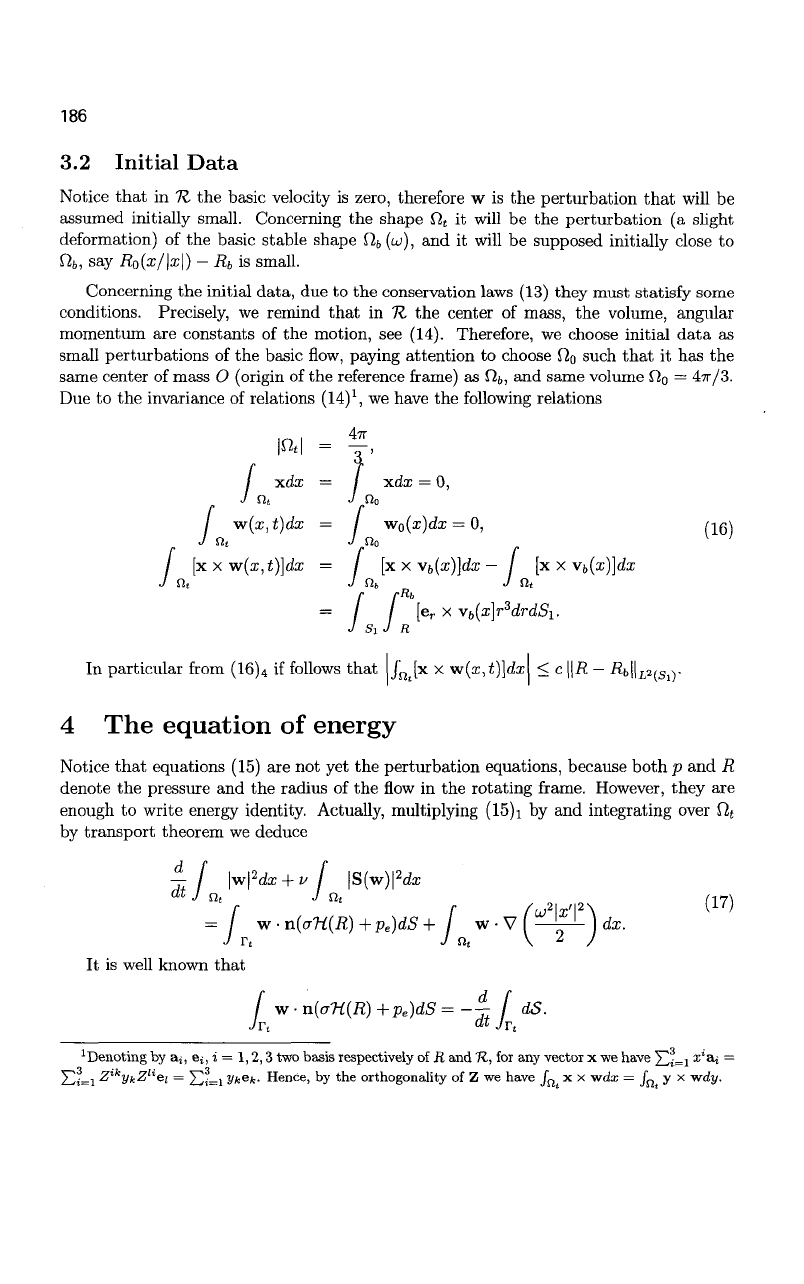
We now look for the best reference frame where to write the perturbed problem.

3.1 Choice of the reference frame

Consider the noninertial frame

rotating with angular velocity o;e3, and set v(j/,t) =

w(x, t) + v

(x). In 7?., the fluid has velocity w, and problem (12) is written as follows:

+ (w

•

V)w - i/V

w + Vp = V ( —~- j - 2toe

x w,

•

w = 0 x € tot, t > 0,

Tn(w,p)n = {aH{R)+p

)n, (15)

{x/\x\,t) = wn leT,,

R{x/\x\,0) = Ro{x/\x\),

t=0

= w

(a;) = v

(x) - V|,(x) x € too,

where \x'\ denotes the distance of x from the a;

-axis, and the terms — 2ue3 x w, V ( "

)

represent the Coriolis and the centrifugal force, respectively.

186

3.2 Initial Data

Notice that in 71 the basic velocity is zero, therefore w is the perturbation that will be

assumed initially small. Concerning the shape Q

it will be the perturbation (a slight

deformation) of the basic stable shape

(ui), and it will be supposed initially close to

fit, say R

(x/\x\) — R

is small.

Concerning the initial data, due to the conservation laws (13) they must statisfy some

conditions. Precisely, we remind that in "R, the center of mass, the volume, angular

momentum are constants of the motion, see (14). Therefore, we choose initial data as

small perturbations of the basic flow, paying attention to choose f2p such that it has the

same center of mass O (origin of the reference frame) as fl

, and same volume f2o =

47r/3.

Due to the invariance of relations (14)

, we have the following relations

47T

xdx = / xdx = 0,

O.0

vt(x,t)dx = / w

(x)dx = 0, fi§\

fit J «o

/ [xx w(x,t)]dx — / [xxv

(x)}dx — / [xxv

(x)]dx

J «

J sib J n

= / [e

x v

(x]r

drdSi.

J SiJ R

In particular from (16)4 if follows that J"

[x x w(a;,t)]d3; < c \\R

—

RbW^ts y

4 The equation of energy

Notice that equations (15) are not yet the perturbation equations, because both p and R

denote the pressure and the radius of the flow in the rotating frame. However, they are

enough to write energy identity. Actually, multiplying (15)i by and integrating over fi

(

by transport theorem we deduce

/ \w\

dx + v [ |S(w)|

= [ w n{oH(R) +

)dS + f w

•

V ( ^L\ d

(17)

It is well known that

/ wn(aH{R)+p

)dS=-4- f dS.

dt J

Denoting by a

, e

i = 1,2,3 two basis respectively of R and

7£,

for any vector x we have Y^i=\

£,a

Yli=i Z

VkZ

'ei = Si=i

Vk^k-

Hence, by the orthogonality of Z we have J

x x wdx = J

y x vfdy.

187

Hence from the identity

.,2

[ ^j-dx + 1 f

dS-

f ^-

sin

edSl + v

f |

(w)|

dx = 0. (18)

* at J

I J

5 J

(17) yields

iwi

d r ._ w

/• R

We obtain easily a norm for the perturbation p = R-R

, to the surface R

= R

(x/\x\)

if we assume that G(R) assumes a local isolated minimum in R

. Indeed,, for regular

function R(x/\x\,t), in a small neighborhood of Rb, it holds

G(R)-G(R

) = \\R-R

wUSi)

. (19)

Finally, since G(R

) is constant in time we deduce the following energy equation for

the perturbation

| {llwllL(ft) + G(R) - G(R

)} + H|S(w)||

2(nt)

= 0. (20)

From Korn's inequality, we know that the gradient of w is increased by S(w) plus the

square of momentum and angular momentum of w. The momentum is zero, cf.

(16)3,

while the angular momentum is increased by R

—

, cf. (16)4. This allows us to state

lli2(nt) ^ l|S(w)||

2(nt)

+ H-R- RbW^Si)-

(21)

Till now we have obtained a classical energy estimate for the perturbation, with a

dissipative term only for the deformation of the velocity field (not even for the velocity

!).

In the next section we shall provide a dissipative term for p = R

—

and for w.

5 Auxiliary equation

In this section we wish to find a "dissipative" term for R

—

, to this end we use the

method introduced in [5] and already applied to free boundary problems in [6], [7]. We

notice that problem (15) can be rewritten as follows

+ (w

•

V)w - i/V

w + Vr = -2we3 x w,

•

w = 0 x e fit, t > 0,

Tn(w,r)n = [a{H(R) - H{R

))

{R)

)]n, (22)

Rt(x/\x\,t) = w-n

x(zT

R(y,o) = Mv) yes

t=0

= w

(i) x S fi

where

r = p-[ —^— =

Pb-

188

We solve an auxiliary problem. Find a solenoidal vector function w in W^fij) satis-

fying the problem

V-v(x,t)

= 0 x e fi

llvllwjtn,) + l|0

v||

i2(

no < c(||p||

(rj)

+ ||w

•

n||

L2(rt)

The compatibility condition is satisfied because the volume is constant. The con-

struction of w can be done by reducing fi

to a fixed domain, thus following the method

outlined in [7]. Furthermore, we select v to satisfy the additional constraint

v-#ote=0, V* = e* x x, i = 1,2,3.

This is possible. Indeed, we choose A = ]T}i=i c'(t)a^(x), with a^ G Cg°(Q.

), and since

fit C -02, where Bi is a ball of radius 2, we can also assume ||ai||w2(

) < 1, J

s^dx = 1.

Then, if V satisfies (23), the vector field V = V +

rotA,

A'S C£°(fi

),'satisfies :

•

tydx = 0, provided that

— / V

•

*dx = / rotA

•

*cte = /

rofr& •

Adx.

Jilt JQt Jilt

In particular, we have

/ rot(ei x x)

•

Adx = —2 / A

= —2a

= / e;

•

(x x V')da;,

which tells us that the coefficients a^t) are bounded by the L

-norm of V, and finally,

V is bounded by \& and w in W^fi). Let us multiply (22)i by V, orthogonal to rigid

motions, and integrate over fi

. Using (23)2, (22)

we get

(w«,

V) + ((w

•

V)w, V) + i/(S(w), VV)

•

n(a{H(R) - H(R

))+

(R) -

))dS = -2(w x w, V),

(24)

where (.,.) denotes the scalar product in L

(fij). We notice that, by transport theorem,

it yields

, V) + ((w

•

V)w,V) 4(w, V) - (V

,w) - ((w

•

V)V,w). (25)

Thus,

we rewrite (24) in the form

±(w,V)-J V-n(a(H(R)-H(R

))+

(R)-

))dS

= -i/(S(w), VV) + ((w

•

V)v, w) + (v

, w) - 2(w x w, V).

Our aim is to increase the right hand side of (26) with terms of the kind either :

(

)lli

(«t)

(

)lli2(r!oll-R

^HwjHsi))

nonlinear terms. Now we notice that, by

189

(23)3,

embeddings theorems and Poincare's inequality, the first two terms at the right

hand side are increased by ||S(w)|||

2(n0

+ ||S(w)||

ia(

)P - Rb\\w}(

), plus nonlinear

terms as we wish. The term F(w) = (vj,w) - 2(w x w,V), presents some problems

since it is increased only by the quadratic term ||Vw||

)||.R - R

y To overcome

this difficulty, we remind that w is orthogonal to rigid motions and that the vector

field w can be always decomposed into a sum of two vectors w' + h with w' such that

'lli

(nt) — ll

(

)ll!

(fit)>

and h a

motion. The linearity of F(yv) implies that

f(w') < ||S(w)|||

2(nt)

+ ||S(w)||

2(nt)

||fl-fl

(Sl)

as we wish. Therefore it remains the

term F(h), with h = (f>xx. This will be handled employing the orthogonahty of w to

rigid motions. Let R

be a reference frame rotating with angular velocity —2u> in

1Z.

Let

us call e; a basis in

TZ,

and a^ a basis in R'. Poisson's formula implies : ^a^ = —2w x aj.

Therefore, since the same vector w has different components V

, W', in the two frames,

related by the identity

V\t)ei

{t)a.j(t)

= V(t), we have

£v-™.-|c*<.M0>-^

-*x<*<.*,M.-|

where §^V, |^V = |^V mean the time derivatives of the same vector w in the different

frames

1Z.

and R', and where ~V = |£V + 2w x V is the derivative in the frame rotating

with angular speed 2u>. In this way, we write

F(h) = ^V + 2(wxV,h)=(^V,*xx

Since we are in Eulerian coordinates, it holds

f - d

—V

• <b

x xdx = - / V

•

—

x xdx + — / V

• <j>

x xdx

(it

J n

dt dt J

- [ (V

• 4>

x x)w ndS = / V

•

[("V

• <j>

x x) w]dx.

ir,

Jn, ^ '

Therefore, F(h) is a nonlinear term. The latter term at left hand side in (24) requires

some calculation because we don't want require smallness on the derivatives of R

. Let

us observe that

•

n{K{R) - K(R

))dS = -/

(IC(R) - KiR^R^gdS,

V' 03 yis (27)

= -/ ^~^(IC(R) - ICiR^dS^

J Si *

Our aim is to prove that (27) is equivalent to the norm of R

—

W^iSi).

hypothesis, R

is a minimum configuration for G(R). Noting that R

— R

= (R

—

Rb){R

+ RRb), since we can choose p = R - R

, by (11), (7), we rewrite (27) as

follows