Bemelmans J. et. al. (editor) Elliptic and Parabolic Problems: Rolduc and Gaeta 2001
Подождите немного. Документ загружается.


170
Let us briefly sketch our basic ideas to establish (5). We start from the simple fact
that, for each a £ R
N
, the rescaled nonnegative global solution w
a
of (10) has to satisfy
/ !"«(«, y)p(y) dy < (p- \)~7^ = K for all s > s
0
,
with
p(y) = (47r)-T
e
-
V
4
. (11)
Since w
a
(s, y) =
WQ{S,
y + ae
S//2
), this can be restated in terms of convolution product
as follows
H/o*
w
0
(s)||oo = sup / w
0
(s,r])p(r]
—
ae
s
'
2
)dn < K for all s > s
0
. (12)
oeR
«
JR."
This estimate leads us to consider the problem (10) in convolution Lebesgue spaces.
Definition 3.1 For all 1 < q < oo and any function f e L]
oc
(R
N
), we set
\\f\\l
P
= IIP* l/l'll^* = f sup j \}{y)\"
P
{a - y) dy)
*
, (13)
where || • ||, denotes the norm in L
q
=
L
q
(R
N
).
The convolution Lebesgue space
L
9
pA
=
L
q
pA
(R
N
)
is then defined by
Ll* = {feLl
c
(R
N
)--\\P*\f\
q
C<™}- (14)
These are Banach spaces with the norm \\-\\*
p
- Also, for q = oo, we define L^ =
L°°(R
W
).
The central part of our arguments is then the analysis of the smoothing properties of
the linear problem
z
s
—
Az - \y
•
Vz in (0,+oo) x R
w
, . .
z(0,y) =
<Hy)
inR
w
, ^
i0j
and of the nonlinear problem (10) in these spaces.
Let us point out that the forward self-similar transformation y =
xt
-1
^
2
,
s = log*
(usually linked to the large time behavior of global solutions) is related with the linear
problem z
s
= Az + \y
•
Vz, and that the corresponding semigroup has nice smoothing
properties in Lebesgue spaces weighted by e'
w
' /
4
. On the contrary, such properties are
not true for equation (15) in Lebesgue spaces weighted by p(y) (see [8] and [11]). The
introduction of convolution Lebesgue spaces enables us to overcome this difficulty.

171
4 Linear
and
nonlinear smoothing effect
in
convolu-
tion Lebesgue spaces
Let
A be the
operator defined
in the
Hilbert space
L
2
by
V {A)
= {/ e
L
2
p
: V/
G
[iff ,
Af-^y-Vf£L
2
in the
distributional sense!
,
A(f)=Af-\yVf,
feV(A),
where L
2
p
is the
weighted Lebesgue space defined
on the
usual
way
corresponding
to the
Borel measure p(y)dy.
The
operator —A
is
self-adjoint with dense domain
in L
2
and it is
the generator
of a
semigroup
of
contractions
on
that space which we denote
by
(5(s))
s
>o-
It
is
easily proved that
(5(
S
)^)(2/)
=
(T(l-
e
-^)(
e
-^)
i 0eL
J,
yeR",
where
T(t) = (G
t
)*
denotes
the
linear heat semigroup
in R
N
and
G
t
(x)
=
(4
7
ri)-?e-
]
^,
t > 0. (16)
Note that
d = p.
We prove that
the
linear semigroup (S(s))
s
>
0
denned
in L
2
admits
a
uniquely, densely
defined extension
to
L* still denoted
by
(<S(s))
s
>o.
In
particular,
if
</>
£
L}
pik
and
<j>
> 0,
then S(s)cj)
> 0.
Moreover,
for
each
1 < q < oo,
(S(s))
s
>o restricts
to a
semigroup
of
contractions
on
L
q
Pii
,.
Our main result
in the
linear theory
is a
smoothing effect
of
the semigroup («S(s))
s
>
0
on convolution Lebesgue spaces
L* .
Theorem
4.1 . Let
<j>
e L*^,
tuifft
1 < g < oo.
Then
S(s)<j>
6 L^ /or all q < r < oo,
and
||S(
S
M|;„
<
(1
-
e-
s
)-^(i-»
||0||;
ip
,
/or a// s > 0.
We should point
out
that this estimate does
not
hold
if
we replace
the
L
q
pik
norms
by
L? norms
(see e.g.
[11]).
Next, we are concerned with the nonlinear problem (10) (where we assume that so
= 0)
with initial data
<j>
e L
9
, 1 < q <
oo. Note that,
if w is a
classical solution
of
(10) (that
is,
w E
C
1
-
2
{{0,oo)
x R>) and w e
L°°{{0,T),L°°(R
N
))
for
each
T > 0),
then
it
solves
the integral equation
w(s)=S(s)<f,+
J'S{s - T)
(\W{T)\
P
-^T)
- ^Yj dr, (17)
for
all s > 0.
An essential ingredient
to the
proof
of our
main result Theorem
2.1 and its
conse-
quences
is the
following smoothing property
for the
equation
(10) in L
9
spaces.

172
Theorem
4.2 .
Let q
c
= N(p
—
l)/2 and let q
>
1 satisfy q
c
< q
< oo. Let
M > 0. For
any
T > 0,
any
<j>
€
L°° with
\\<j)\\
qtP
< M,
and any solution
w e
L°°((Q,T),L'
:o
)
of
(17),
it holds
IMs)IU
<
C8-%M\\*
qiP
,
0
<
8
<min(T,
Sl
),
where
C > 0
depends only on
N, p, q
and Si >
0
depends only on
N, p, q, M.
The proof
of
Theorem
4.2
relies
on the
linear estimates
of
Theorem 4.1. Actually,
from these estimates, we can also derive
a
local existence-uniqueness result
for
problem
(10)
in
LP spaces (in the spirit
of
Theorem
4 in
[4] and based
on
arguments
of
[22]).
5 Universal bounds
and
uniform decay rates
The result
of
Theorem 2.1, case
(i)
(p
<
1
+ 2/N) is a
direct consequence
of
the estimate
(12),
which can
be
restated
in
terms
of
convolution Lebesgue norms
as
lko(s)Hi,p
<« for
all
s > s
0l
and
of
Theorem 4.2 applied with q
=
1
> N(p
—
l)/2.
In view
of
case
(ii) of
Theorem 2.1,
we
also need
to
derive
a
uniform
a
priori
bound
for the
blow-up rate
of
nonnegative solutions
of
(1). More precisely,
for any
(N
—
2)p
< N +
2, we prove that
the
constant
C in (2)
depends only
on the
L°° norm
of
the
initial data
and on an
upper bound
of the
blow-up time
T.
This result
was
already obtained in [18] for the vector-valued problem associated to (1) under the condition
(3N
—
4)p
<
3iV
+
8 (in the scalar problem no sign restriction on the solution was made).
In terms
of
the rescaled problem (10), we prove the following theorem.
Theorem
5.1 .
Assume
p > 1
and
(N -
2)p
< N
+ 2, and let C
0
> 0. Let
<f>
6
L°°
be
nonnegative, satisfying ||</>||oo
<
Co, and such that the solution
w of
(10) (with
SQ
= 0) is
global. Then,
IHs)||oo<C(C
0
,p,iV)
for
qll
s>0.
To conclude the proof
of
Theorem 2.1, case (ii) when
(N
—
2)p <
N,
we then proceed
similarly
as in
[4]. Multiplying
the
equation (10)
by p(y) and
integrating
in
space
on
R
w
and in
time over (s
0
,s
0
+
5), one shows
the
existence
of s
x
e
(0,6/2) such that
J
RJ
v w
p
(si,y)p(y)dy
<
C(p,N,S). Using the fact that the L
q
and L
q
norms are equivalent
in the class
of
nonincreasing nonnegative radially symmetric functions, we obtain
\Hsi)\\;,
P
<C(p,N,6).
(18)
The conclusion then follows by combining (18) with Theorem 4.2, applied for
q
=
p >
N(p
-
l)/2, and Theorem 5.1.
The proof
in the
case
(N
—
2)p
< N
+ 2,
N < 3 is
inspired from the method
of
[19]
and
is
somehow more involved. Beside convolution Lebesgue spaces,
it
relies on rescaling
and contradiction arguments and
on
energy estimates similar
to
those
in
[6]
and
[8].

173
Finally, Theorem 2.2 follows easily from Theorem 2.1. Since the solution u of (1)
exists globally on [0, oo) x R
N
, by applying Theorem 2.1 with e = 1/2 for each T > 0, we
deduce that
K*)l|»<C(JV,p)(T-t)-
1
/<''-
1
\ T/2<t<T<oo. (19)
For each fixed t
0
> 0, applying (19) with T = 2t
0
and t
—
t
0
yields
and the result follows.
References
D.
Andreucci, M. A. Herrero, J. J. L. Velazquez, Liouville theorems and blow up
behaviour in semilinear reaction diffusion systems, Ann. Inst. Henri Poincare 14,
No.
1 (1997), 1-53.
M.-F.
Bidaut-Veron, Initial blow-up for the solutions of a semilinear parabolic equa-
tion with source term, in: Equations aux derivees partielles et applications, articles
dedies a Jacques-Louis Lions. Gauthier-Villars, Paris. (1998) pp. 189-198.
S. Bricher, Blow-up behaviour for nonlinearly perturbed semilinear parabolic prob-
lems,
Proc. Royal Soc. Edinburgh 124A (1994), 947-969.
M. Fila, P. Souplet, F. Weissler, Linear and nonlinear heat equations in L
p
6
spaces
and universal bounds for global solutions, Math. Ann. 320 (2001), 87-113.
V. A. Galaktionov, S. A. Posashkov, The equation u
t
= u
xx
+ u
13
. Localization,
asymptotic behavior of unbounded solutions. Akad. Nauk SSSR Inst. Prikl. Mat.
Preprint 1985, n°97, 30 pp (in Russian).
Y. Giga, A bound for global solutions of semilinear heat equations, Comm. Math.
Phys.
103 (1986),
415-421.
Y. Giga, R. Kohn, Asymptotically self-similar blow-up of semilinear heat equations,
Comm. Pure Appl. Math. 38 (1985), 297-319.
Y. Giga, R. Kohn, Characterizing blowup using similarity variables, Indiana Univ.
Math. J. 36 (1987), 1-40.
C. Gui, W.-M. Ni, X. Wang, Further study on a nonlinear heat equation, J. Differ.
Eq. 169 (2001), 568-613.
A. Haraux, F. B. Weissler, Non-uniqueness for a semilinear initial value problem,
Indiana Univ. Math. J. 31 (1982), 167-189.
M. A. Herrero, J. J. L. Velazquez, Blow up behaviour of one dimensional semilinear
parabolic equations, Ann. Inst. H. Poincare, Anal. Nonlin. 10 (1993), No. 2, 131-189.

174
[12] M. A. Herrero, J. J. L. Velazquez, Explosion de solutions des equations paraboliques
semilineaires supercritiques, C. R. Acad. Sci. Paris, t. 319 (1994), 141-145.
[13] O. Kavian, Remarks on the large time behaviour of a nonlinear diffusion equation,
Ann. Inst. H. Poincare, Anal. Nonlin. 4 (1987), 423-452.
[14] J. Matos, Convergence of blow up solutions of nonlinear heat equations in the super-
critical case, Proc. Royal Soc. Edinburgh 129A (1999), 1197-1227.
[15] J. Matos, Ph. Souplet, Universal blowup estimates and decay rates for a semilinear
heat equation, Preprint.
[16] F. Merle, H. Zaag, Optimal estimates for blowup rate and behavior for nonlinear
heat equations, Comm. Pure Appl. Math. 51 (1998), 139-196.
[17] F. Merle, H. Zaag, Refined uniform estimates at blow-up and applications for non-
linear heat equations, GAFA, Geom. Funct. Anal. 8 (1998), 1043-1085.
[18] F. Merle, H. Zaag, A Liouville theorem for vector-valued nonlinear heat equations,
Math. Ann. 316 (2000), 103-137.
[19] P. Quittner, Universal bound for global positive solutions of a superlinear parabolic
problem, Math. Ann. 320 (2001), 299-305.
[20] P. Quittner, Ph. Souplet, Initial blow-up rates for a nonlinear heat equation, to
appear.
[21] Ph. Souplet, Sur I'asymptotique des solutions globales pour une equation de la chaleur
semi-lineaire dans des domaines non bornes, C. R. Acad. Sci. Paris, Serie I 323 (1996),
877-882.
[22] F. B. Weissler, Local existence and nonexistence for semilinear parabolic equations
in V, Indiana Univ. Math. J. 29 (1980), 79-102.

Asymptotic behavior of curves evolving by forced
curvature flows
Hirokazu Ninomiya
Department of Mathematics and Informatics,
Ryukoku University, Seta, Otsu 520-2194 JAPAN.
The motion of an interface is one of most important and attractive topics in ap-
plied mathematics. Especially, the mean curvature flows have been studied by many
researchers. In the two dimensional case, it is called a curve shortening flow. Asymptotic
behaviors of curve shortening flows are well-known. If a curve is a simply closed one, it
becomes convex eventually and is shrinking to a point (see [7, 8]). If it possesses two
asymptotes, Huisken has proved that it converges to a self-similar solution (see [5, 10]).
In this paper, we consider how the behavior changes, if we add a constant driving force.
First we will define an interface. A pair (T(t),v) is called an interface provided that
there exists a family of connected open sets D(t) in R
N
such that F(i) is a smooth
connected boundary of D(t) and v is a outer normal vector on T(t) pointing from D(t) to
D(t)
c
.
By the definition, an interface is not allowed to have any self-intersection points.
We consider here an interface (T(t),u) which satisfies
V = H + k (1)
where V is a normal velocity in the direction of u, H is the curvature, and k is a given
constant.
This equation appears in the several fields of applied mathematics. For examples,
this system represents the motion of transition layers of the Allen-Cahn equation [6], and
the filamentray vortex of the Ginzburg-Landau equation confined in a plane [4], the BZ
reaction [12].
In the curve shortening flow (i.e., k = 0 and N = 2), the property of non-existence
of self-intersection points preserves in time (see [7, 8]). In the case k ^ 0, unfortunately,
some interfaces may possess some self-intersection points eventually, even if the initial
interface has none. Actually, in the case k > 0, take the initial interface T(t) and D(t) as
in Figure 1, i.e., £>
x
(0) C D(0) C
D
2
{Q),
where
£>!(*) := s((°),-Ri(*))U5((_°
0
),fli(*)).
D
2
(t)
:= B((J),fl
2
(i))UB((~
0
6
),W) ,
175
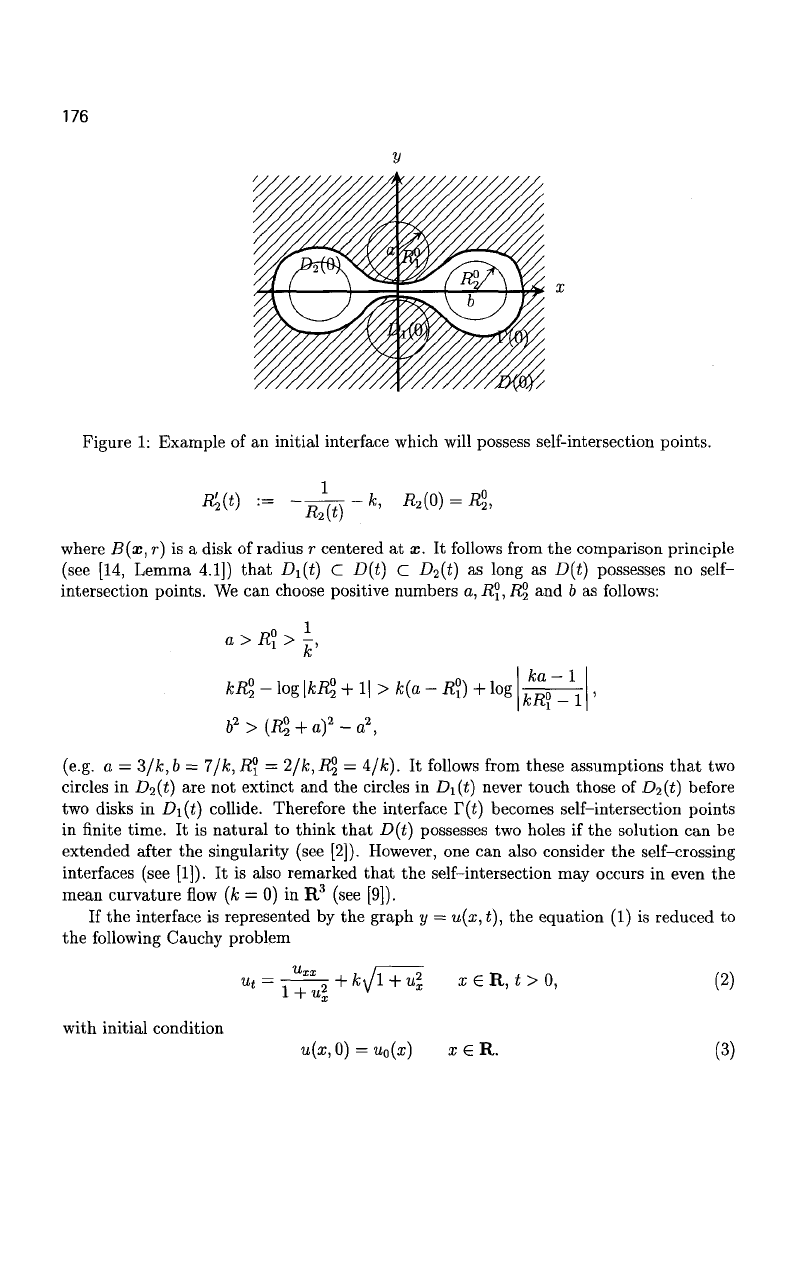
176
§1
VAP*
§L
'<%gm
W
(®?\
y//y/z
IS
Figure
1:
Example
of an
initial interface which will possess self-intersection points.
i^W
==
-J^t)~
k
>
RM = R°,
where
B(x, r) is a
disk
of
radius
r
centered
at x. It
follows from
the
comparison principle
(see
[14,
Lemma
4.1])
that
D
x
{t) C D(t) C D
2
{t) as
long
as D(t)
possesses
no self-
intersection points.
We can
choose positive numbers a, Ri,R%
and b as
follows:
a
> R° > -,
k
kR\
-
log
\kR\ +
1|
>
k{a
-
E?)
+
log
b
2
>(Rl
+
a)
2
-a\
ka
—
1
kRl-
(e.g.
a = Z/k,
b
= 7/k,
Rf(
=
2/fc,
R\ =
4/fc).
It
follows from these assumptions that
two
circles
in D?(t) are not
extinct
and the
circles
in Di(t)
never touch those
of D
2
(t)
before
two disks
in D\(t)
collide. Therefore
the
interface
T(t)
becomes self-intersection points
in finite time.
It is
natural
to
think that
D(t)
possesses
two
holes
if the
solution
can be
extended after
the
singularity
(see [2]).
However,
one can
also consider
the
self-crossing
interfaces
(see [1]). It is
also remarked that
the
self-intersection
may
occurs
in
even
the
mean curvature flow
(k = 0) in R
3
(see [9]).
If
the
interface
is
represented
by the
graph
y =
u(x,t),
the
equation
(1) is
reduced
to
the following Cauchy problem
u
t
l +
ul
+
fc^/l + u\
i£R,i>0,
with initial condition
u(x,
0)
= u
0
(x) iGR.
(2)
(3)
177
The existence and uniqueness of solutions of this equation is studied in [3, 4] (also see
[11,
13]). Note that the maximum principle holds for this equation (cf. [15]).
We will give the definition of the traveling front of (1). As an example, we take
the line y = xta,n9 with v =
t
(—sm6,cos6) where 0 < 9 < ir/2. It is easily seen
that this interface moves not only with speed k in the direction i/, but also with speed
k/cos9 in the direction '(0,1). So, it is natural that the velocity of the traveling front
should be specified. The interface (T(t),v) is called a traveling front with velocity v, if
r(i) = 17(0) + vt. The above example is regarded as the traveling front with velocity
kv or velocity '(0, fc/cos#„). Deckelnick et al in [4] proved the existence of the traveling
curved front and studied the stability of the front under some restricted assumptions for
«o-
The author and Taniguchi relaxed the assumption for the initial data and classified
all the traveling fronts in [13, 14]. They proved the following (see [13, Proposition 1.1 and
Theorem
1.2]).
Theorem 1 Any traveling front of (1) with velocity '(0, c) is one of the three, after ap-
propriate translations,
(i) lines y = xta,u9
t
, and y = —xtan6»
(ii) a traveling curved front T
c
(t) which possesses two asymptotes y = irrtanft,,
(iii) stationary circles with radius l/\k\ only in the case c = 0,
where
9„
= arctan(\/c
2
—
k
2
/k).
Moreover the explicit form of the traveling curved front
T
c
(t) with speed c(> k) is given in
x{9;
c)
y(9;c)
for9e (-0.A)-
The traveling curved front T
c
(t) is "V-shaped", which connects two asymptotes. The
existence of this traveling front is also reported in
[1,4].
The proof of this theorem consists
of two parts. First it was proved that the traveling front with non-zero speed should be
an entire graph (see [13, Lemma 2.4]). Next all the traveling front of (2) are classified
(see [13, Lemma 2.3] for the details).
In the case (ii) where the initial interface possesses two asymptotes, we denote the
exterior angle between two asymptotes by 9", which is equal to
7r —
29,, if their inclinations
are ±tan#„. It is checked that the speed c of the traveling curved front T
c
(t) is uniquely
determined by the angle
9*
(or
9„),
i.e., c = k/ cos
9*.
This traveling front can be observed
in a liquid BZ reaction (see [12]).
The asymptotic stability of the curved traveling front in (2) is discussed in [4, 14].
cVc
k
2
log
1+
1
!"1
c + k 9
/ r tan -
\j
c- k 2
/c + fc 9
/ r tan -
\j
c-k 2
1 fccos9
—
k
•c
l0E
b^r

178
Theorem 2 The traveling curved front is asymptotically stable, if the initial perturbation
is restricted to
BCl := {v e C
X
(R)
sup (|n(o;)| + |v
T
(x)|) < oo, lim v(x) = 0}.
-0O<X<00 |l|->0O
Furthermore, it is shown in [14] that the interface which have two asymptotes with
angle 9* converges to the corresponding traveling curved front as t tends to infinity,
provided that
9*
<
IT.
This means that T
c
(t) is globally asymptotically stable.
If we do not take BCg as the perturbation space, the situation changes. In the class
BC
1
:={ve C^fR) | sup (\v{x)\ + \v
x
{x)\) < oo},
—oo<:r<oo
the traveling curved front is not asymptotically stable. Namely, for any e > 0, there exists
an interface T(i) of (1) such that
dist(r(o),r
c
(o))<4e,
T{t)n
(r
c
(t) +
( ° \\?9, v(t)n
(r
c
(t)-
( °))^
0
for all t > 0 (see [14, Theorem 4.1]). This interface oscillates at infinity around T
c
(i).
The very interesting result [16] by Yagisita should be remarked. He considered nearly-
spherically expanding fronts of the Allen-Cahn equations. Though the front is known
to converge to the sphere after the rescaling of the radius to unity, he proved that the
difference between the front and the expanding sphere does not decay as t tends to infinity.
Next we will explain the case where the angle between the two asymptotes is greater
than n. Using the method of functional analysis, Deckelnick et al also proved in [4] that,
for u
0
(x) = —
|x|tan0*,
(4)
u(x, t) - tQ ( -
as t tends to infinity, where
Q(s) :=
x/F
\s\
tan#*
-
COS0*
+ 0,
\s\
< fcsinft,,
\s\
> ksin9
t
,
(5)
where 9, =
(9*
—
7r)/2. This result indicates that the top order of the solution is tQ{x/t).
We can expect the difference between u and tQ(x/t) does not decay as in [16].
References
[1] P. K.
BRAZHNIK,
Exact solutions for the kinematic model of autowaves in two-
dimensional excitable media, Physica D, 94, (1996) 205-220.
[2] Y.-G. Chen, Y. Giga and S. Goto, Uniqueness and existence of viscosity solutions of
generalized mean curvature flow equations, J.
Diff.
Geom. 33, (1991), 749-786.

179
[3] K.-S. Chou and Y.-C. Kwong,
On
quasilinear parabolic equations which admit global
solutions
for
initial data with unrestricted growth, Calc.
Var.
Partial Differential
Equations 12-3, (2001), 281-315.
[4]
K.
Deckelnick,
C. M.
Elliott,
and G.
Richardson, Long time asymptotics
for
forced
curvature flow with applications
to the
motion
of a
superconducting vortex, Nonlin-
earity
10
(1997), 655-678.
[5]
K.
Ecker,
and G.
Huisken, Mean curvature evolution
of
entire graphs,
Ann. of
Math.
130-3 (1989),
453-471.
[6]
P. C.
Fife, Dynamics
of
Internal Layers
and
Diffusive Interfaces (CBMS-NSF
Reg.
Conf.
Ser.
Appl. Math. 53), SIAM (1988).
[7]
M.
Gage,
and R. S.
Hamilton,
The
heat equation shrinking convex plane curves,
J.
Diff.
Geom.
23
(1986), 69-96.
[8]
M. A.
Grayson,
The
heat equation shrinks embedded plane curves
to
round points,
J. Diff.
Geom.
26
(1987), 285-314.
[9]
M. A.
Grayson,
A
short note
on the
evolution
of a
surface
by its
mean curvature,
Duke Math.
J. 58
(1989), 555-558.
[10] N. Ishimura, Curvature evolution of plane curves with prescribed opening angle, Bull.
Austral. Math. Soc.
52-2
(1995), 287-296.
[11]
O.A.,
Ladyzenskaja,
V.A.
Solonnikov,
and
N.N. Ural'ceva, Linear
and
Quasilinear
Equations
of
Parabolic Type, Translations
of
Mathematical Monographs
24,
Provi-
dence
RI,
(1968), American Mathematical Society.
[12]
V.
Perez-Munuzuri,
M.
Gomez-Gesteira,
A. P.
Munuzuri,
V. A.
Davydov,
and V.
Perez-Villar, V-shaped stable nonspiral patterns, Physical Review
E 51-2
(1995),
845-847.
[13]
H.
Ninomiya
and M.
Taniguchi, Traveling curved fronts
of a
mean curvature flow
with constant driving force,
in
" Free
boundary problems: theory
and
applications,
i"
GAKUTO Internat.
Ser.
Math.
Sci.
Appl.
13
(2000),
206-221.
[14]
H.
Ninomiya
and M.
Taniguchi, Stability
of
traveling curved fronts
in a
curvature
flow with driving force,
to
appear
in
Methods and Application
of
Analysis.
[15]
M. H.
Protter
and
H.
F.
Weinberger, Maximum Principles
in
Differential Equations,
(1984) Springer-Verlag.
[16]
H.
Yagisita, Nearly spherically symmetric expanding fronts
in a
bistable reaction-
diffusion equation,
J.
Dynam. Differential Equations,
13-2
(2001), 323-353.
