Bemelmans J. et. al. (editor) Elliptic and Parabolic Problems: Rolduc and Gaeta 2001

Подождите немного. Документ загружается.

270

Moreover,

follows from (4.3)

and

Lebesgue's convergence theorem that

—>

(0,1) as

—¥

+oo. So by (4.4) and the uniqueness

the limit,

x =

X-

Secondly,

let us

assume (4.1) (resp. (4.2)). Since the constant case

of x is

trivial, we

consider the nonconstant case of x- Here let

x%,k

and

J%,k

be notations c

Xtk

and

Xik

as in (II) of Definition 2.2 with

x =

X- Then, since functions cos(-4j(-)) vary continuously

with respect

K > 0,

is not so difficult to find an open interval

6 C

(#, +oo)

n 9

(resp.

(-co, $) D

and

sequence

|i5e8} such that

X* €

(K(<9),

f) for any i? G 0 and x° ->• X in i

(0,1) as 0 \ d (resp.

-d

/• Q).

For example,

the case

of x

G Sr(ft()?),r; 1),

f =

c^ =

*, and 2^1

= 0, we

can construct them by the following way: ©

(d,

•&

n 0

(resp. (#

—

fl)

n 0 )

with

> 0

satisfying yK($

•

it <

1 (resp.

—

8 > 0), and

-»/

cos

("T^TI

ifxe

[°>

V^WT).

™~ \v«(#)/

-„,

[V^JTT,!],

for any i)£0. Also,

other cases of x, we can construct the positive number

and the

sequence {x*} by

similar way.

Thus we obtain that

dist

(

o,i)(x,Sr(/s(0),f))

|x*

xb(o,i) ->• 0 as

0 \ 0

(resp.

0 /

0).

•

Lemma 4.2 Lei

0, x, T, 0

and

the same as

Lemma 4-1- Let

"*,

and

: 0

—•

(T*!

e a

continuous and strictly increasing function.

(I) dist

,i)(x,5r(«(i?),f(iJ))) ->

0 \

and only

if:

(i) (the case of

f(0)

< r

) x =

const, on [0,1]

or%^

(supp Xi)

(

*)/

fnj (t/ie case

o/r

< f(tf)

< T") X

const on [0,1]

or%^

(supp Xx)

(

"*)-

(II) dist

(0|1)

(x, S

{h(d),f(ti))

/* d if

and only

if:

(i) (the case ofO <

f(d)

)

const,

[0,1] or (supp Xx)

(

^ 0/

(wj (the case of

< f($) <

r* ) x =

const on [0,1]

(supp Xx)

(

*) 7^ $•

Proof.

Definition 2.1,

notice

the

following fact:

for any

nonconstant function

€

ST{K,

with /c

> 0

and

r, < r < r*,

f x

(n)> ifr»<r <^f*S

0C (supp^C

\ / .

(4.5)

Noting (4.5), we can show this lemma by

similar way

as in

the proof of Lemma 4.1.

•

271

Now by making use of Lemmas 4.1 and 4.2, we prove our main theorem. Let $ > 0

and I

- ) be any solution of (P)*. In the proof of Theorem 3.1, the following fact is

important:

i 1

is positive, continuous and strictly increasing on

[0,

). (4.6)

7(0 ' M-)

Proofs of (al) and (bl): In this case, since x? = 1 on

[0,1],

we immediately see that

dtatw ((*|)>

W) = distal) (xf,S[-i,i] (w)'°))

for an

y 0 < ^ < P. Therefore, by

(4.6) and Lemma 4.1 with

= xi<

= [

1]>

^W = ^m

^ ^ = 0, we immediately

have assertions (al) and (bl). •

Proofs of (a2) and (b2): (b2) is obtained just as in (bl). Now we show (a2).

If (

'. ) is above arcwise-connectable, then there are sequences {#j} C ($*,$

) and

(

l'.

€ S{dj), j > 1, such that

•dj

and xf

X?*

in i

(0,1) as j -»• +oo.

Here, by (s3) of Proposition 2.1, |x2

| = Xi , j > 1- So, taking a subsequence if

necessary, we have

|xfl = xfa.e.

on[0)

i].

(4.7)

Combing (4.7) and (s2) of Proposition 2.1, we conclude (xfiXiT)

[0,1]-

Conversely, if (Xi'.xD

-^o on

[0,1],

then it follows from (s3) of Proposition 2.1

that

dis%

((

i'.),S{ti)) =0foranytf* <

•&

-d

Proofs of (a3) and (b3): If (

) is above (resp. below) arcwise-connectable, then

distal) (xf, S

(^j, ^P)) -»• 0 as 0 \ 9 (resp. 0 S $)•

So,

by (4.6) and (i) of (I) (resp. (II)) in Lemma 4.2 with

= X?, T =

[0,1],

K(I3)

= ^,

and f ($) = (^, we obtain

f y? = const, on [0,11 or

Al l J

(4.8)

1 0 / (supp (

f),)

C (xf)-

(0) (resp. (supp (

?)*)

C (x?)"

^) * «)•

Conversely, if (4.8) holds, then applying (i) of (I) (resp. (II)) Lemma 4.2 again, we

find an open interval 0

C (#,i?

) (resp. ($*,^)) and a sequence {xf | i? G 0o} such that

X? 6 Sp,!]^,^) for demand

Xf -»• xf in £

(0,1) as tf \ # (resp. tf /> #).

272

Here since

\x%\

= xi

[0,1],

it is not so difficult to find a sequence {xt \ fl G Oo} C

(0,1) such that

J, e S{§) for

•d

e 6

and xl -»• X2

(°- 1) as

(resp.

•d

/> 1?).

Thus we conclude that (

* ) is above (below) arcwise-connectable. •

Proofs of (a4) and (b4): (a4) (resp. (b4)) is shown just as in the proof of (a3) (resp.

(b3)) by applying statements (ii) of (I) (resp. (II)) with x = xt,T =

[0,1],

re(tf) = ^

References

[1] E. Bonetti, Global solution to a Fremond model for shape memory alloys with thermal

memory, to appear in Nonlinear Analysis.

[2] N. Chemetov, Uniqueness results for the full Fremond model of shape memory alloys,

Anal. Anwendungen, 17 (4), 877-892, 1998.

[3] X. Chen and C. M. Elliott, Asymptotics for a parabolic double obstacle problem,

Royal Soc. London, Proc. Math. Phys. Sci. Ser. A444 (1994), 429-445.

[4] P. Colli, M. Fremond and A. Visintin, Thermo-mechanical evolution of shape memory

alloys, Quart. Appl. Math., 48, 31-47, 1990.

[5] P. Colli, P. Laurengot and U. Stefanelli, Long-time behavior for the full one-

dimensional Fremond model of shape memory alloys, Contin. Mech. Thermodyn.

12 (6), 423-433, 2000.

[6] P. Colli and J. Sprekels, Global solution to the full one-dimensional Fremond model

for shape memory alloys, Math. Methods Appl. Sci., 18, 371-385, 1995.

[7] M. Fremond, Materiaux a memoire de forme, C. R. Acad. Sci. Paris Ser. II Mec.

Phys.

Chim. Sci. Univers Sci. Terre, 304, 239-244, 1987.

[8] M. Fremond, Shape memory alloys. A thermomechanical model, Free Boundary Prob-

lems: Theory and Applications edited by K. H. Hoffmann and J. Sprekels, editors,

Number 185 in Pitman Research Notes Math. Ser., pp 295-306, Longman, London,

1990.

[9] M. Fremond and A. Visintin, Dissipation dans le changement de phase. Surfusion.

Changement de phase irreversible, C. R. Acad. Sci. Paris Ser. II Mec. Phys. Chim.

Sci.

Univers Sci. Terre, 301, 1265-1268, 1985.

[10] K. H. Hoffmann, M. Niezgodka and S. Zheng, Existence and uniqueness to an ex-

tended model of the dynamical developments in shape memory alloys, Nonlinear

Analysis, 15, 977-990, 1990.

273

[11] N. Shemetov, Existence result for the full one-dimensional Premond model of shape

memory alloys, Adv. Math. Sci. Appl., No. 8, Vol. 1, 157-172, 1998.

[12] K. Shirakawa and U. Stefanelli, Structure of steady-state solutions for one-

dimensional Premond models of shape memory alloys, in preparation.

Lagrangian coordinates in free boundary problems for

multidimensional parabolic equations

Sergei I. Shmarev*

Departamento de Matematicas,

University of Oviedo,

33007 Oviedo, SPAIN

shmarev@orion. ciencias. uniovi.es

Abstract

We study the Cauchy problem for nonlinear degenerate parabolic equation

p(x)u

= div(uV/(u) + uq(u)) +uh(u), (x,t) €R

x (0,oo),

in the space dimension N > 1. It is assumed that /(0) = 0, f(s) > 0 and f'(s) > 0

for s > 0, and that p(x) is strictly positive on every compact subset of K.

. Equa-

tions of this class possess the property of finite speed of propagation of disturbances

from the initial data which gives rise to the interfaces (free boundaries) separating

the regions where the solution is positive from those where it is equal to zero.

We describe and justify a non-local coordinate transformation that renders sta-

tionary the support of the nonnegative solution of the Cauchy problem. We present

the explicit formulas which define the solution of the original Cauchy problem and

its interface through the solution of the auxiliary problem posed in the time inde-

pendent domain.

1 Introduction

The aim of this note is to justify the use of a non-local coordinate transformation in the

study of free-boundary problems for degenerate parabolic equations.

We consider the Cauchy problem for the degenerate parabolic equation

p(x)u

= div(tiV/(u) +tiq(n)) + uh(u) (1.1)

under the assumptions

/ e C(R+), /(0) = 0, f(s) > 0 and f'(s) > 0 for s > 0. (1.2)

It is assumed that

•The author was partially supported by DGICYT Project BFM2000-1324, Spain

274

275

p(x) is strictly positive on every compact subset of R^. (1.3)

Let the initial function u

(x) be nonnegative and compactly supported in

TSt

Under

convenient assumptions on q and h the weak continuous solution of the Cauchy problem

for equation (1.1) is also nonnegative and its support is compact for every t > 0. Thus,

the solution exhibits free boundaries or interfaces that separate the regions where the

solution is positive from those where it is identically zero.

The study of the free boundaries lends itself to various methods. In this note, we

describe and justify the method of Lagrangian coordinates which allows one to substitute

the original free-boundary problem for a single equation by a system of nonlinear equations

posed in a time independent domain. The solution of the original free-boundary problem

is then explicitly represented through the solution of the auxiliary one. In particular,

the interface T(t) = <9{suppu(:r,£)} is obtained as a one-to-one mapping from T(0) =

9{suppit

(a;)},

which gives certain advantage in the study of its properties.

The idea of using Lagrangian coordinates in free-boundary problems for evolution

equations was independently proposed in [1, 2, 3] and then developed by several authors.

A survey of results obtained via this method can be found in the monograph [4] and

papers [6, 7].

2 Lagrangian coordinates

In this section we introduce a system of Lagrangian coordinates generated by a solution

of a parabolic equation.

Let u(x,t) be a solution of equation (1.1). Throughout the section we assume that

the solution is as smooth as is needed to perform all the requested transformations. It is

convenient to rewrite equation (1.1) in the form

= ug(p) (2.1)

under the notation

d = pu, p = /(«), w(s) = q(/-

{s)), and g(s) = hif'^s)), s > 0.

Let us start with reformulation of the Cauchy problem for equation (2.1) viewed as

the mathematical description of the process of propagation of a polytropic gas in a porous

medium. There are two possible ways to describe the motions of continua. The first one,

usually referred to as the Euier

method,

consists in viewing the characteristics of motion

(such as velocity, density, etc.) as functions of time t and some Cartesian coordinate

system xi,...,x

not connected with the medium. The alternative description is due

to Lagrange. In this method all magnitudes describing the motion are considered as

functions of time and the initial state of the continuum.

Let S7(0) and H(t) C R

be the domains occupied by a polytropic gas at the moments

t = 0 and t > 0. This correspondence defines the mapping

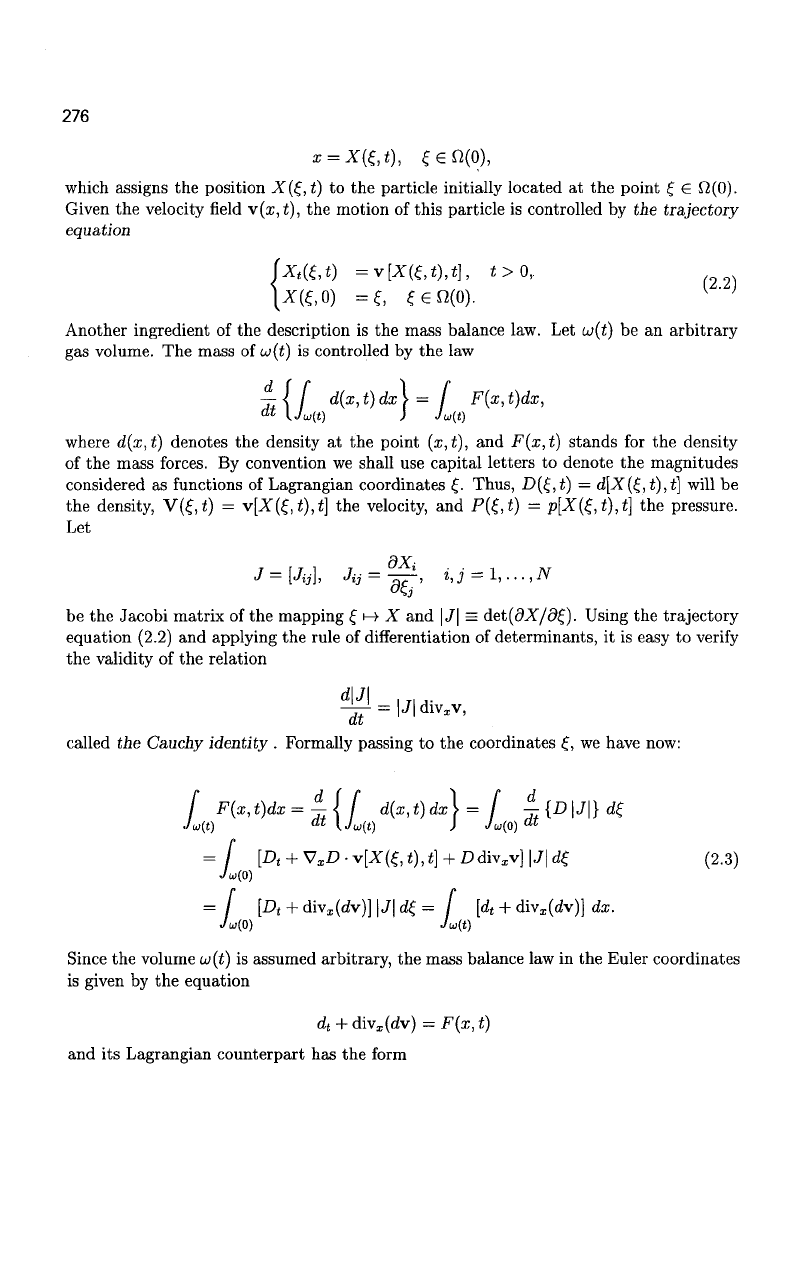
+ div

Vp + w(p)

276

x =

X((,t),

f€fi(0),

which assigns the position X(£,t) to the particle initially located at the point f G fi(0).

Given the velocity field v(x,i), the motion of this particle is controlled by the trajectory

equation

((,t) =v[X(t,t),t], *>0

X(t,0) = £, {6fi(0).

Another ingredient of the description is the mass balance law. Let u(t) be an arbitrary

gas volume. The mass of uj(t) is controlled by the law

XW d(x,t)dx)=[ F(x,i)dx,

dt (J wit) J J wit)

where d(x,t) denotes the density at the point

(x,t),

and F(x,i) stands for the density

of the mass forces. By convention we shall use capital letters to denote the magnitudes

considered as functions of Lagrangian coordinates f. Thus, D(£,t) = d[X{(,,t),t\ will be

the density, V(£,t) = v[X(£,t),t] the velocity, and P(£,i) = p[X(t;, t),t] the pressure.

Let

be the Jacobi matrix of the mapping £

H->

X and | J| = det(9X/df). Using the trajectory

equation (2.2) and applying the rule of differentiation of determinants, it is easy to verify

the validity of the relation

-^ = |J|div

called the Cauchy identity . Formally passing to the coordinates £, we have now:

/ F{x,t)dx=±{[ d(x,t)dx\=f ^-{D\J\}dC

Jw{t)

Uw(t) )

Jw(p)

= [ [D

D-v[X(Z,t),t]

+ Ddiv

v)\J\dZ (2.3)

Ja(0)

= [ [D

+ div,(dv)] \J\ d£ = f

[dt

+ div

(dv)] dx.

Jw{0) Jui(t)

Since the volume w(t) is assumed arbitrary, the mass balance law in the Euler coordinates

is given by the equation

dt + div

(dv) = F(x,t)

and its Lagrangian counterpart has the form

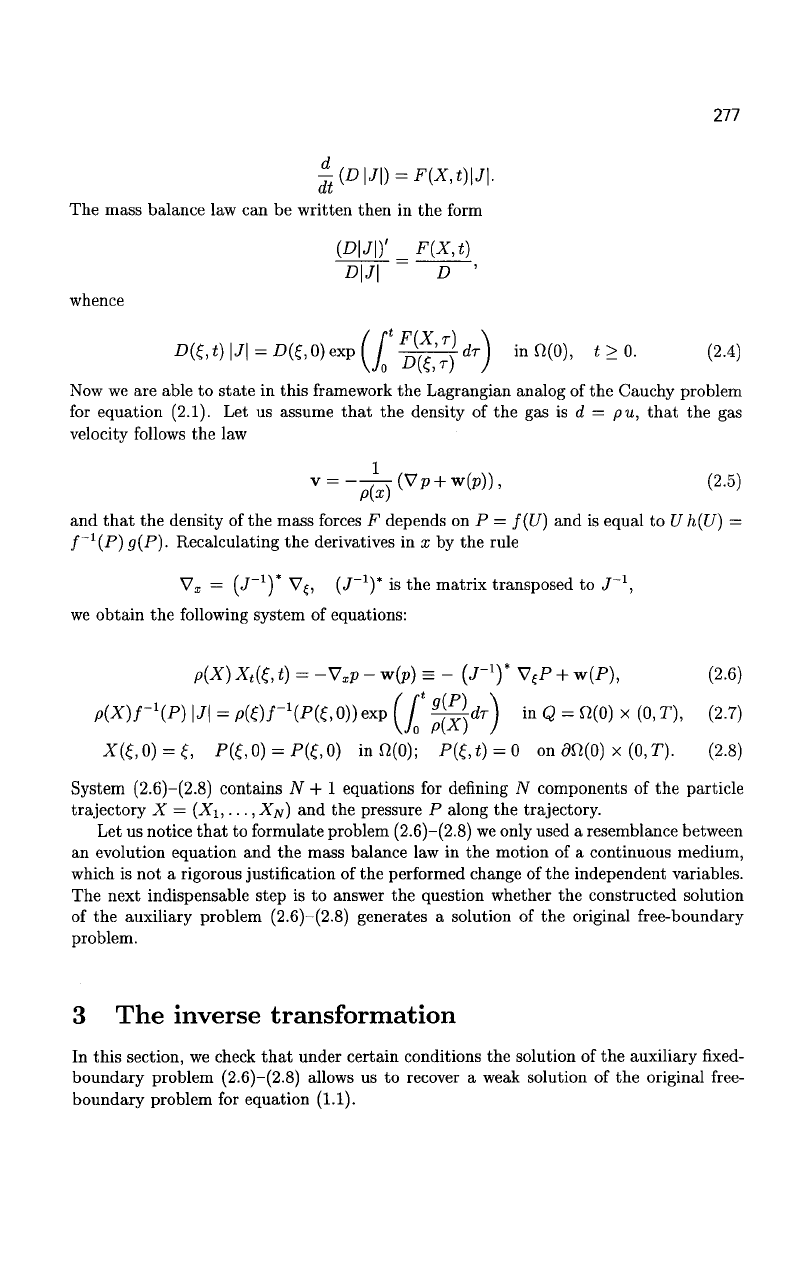
277

(D\J\)

F(X,t)\J\.

The mass balance law can be written then in the form

{D\J\)'

F(X,t)

D\J\ D '

whence

£>(£, t) \J\ = £>(£, 0) exp [J ^^ dr) in J1(0), t > 0. (2.4)

Now we are able to state in this framework the Lagrangian analog of the Cauchy problem

for equation (2.1). Let us assume that the density of the gas is d = pu, that the gas

velocity follows the law

v = -^y(Vp + w(p)), (2.5)

and that the density of the mass forces F depends on P = f(U) and is equal to U h(U) =

-1

(P) g{P). Recalculating the derivatives in x by the rule

Vi = (</

) V^, (>/

)* is the matrix transposed to J~

we obtain the following system of equations:

p{X)XM,t) = -V,p-w(p) = -

(J"

P + w(P), (2.6)

{x)r\p)

\J\

p(orW,o))ex

)

Q =

(°)

(°>

(

)

X(Z,0) = {, P(f,0) = P(f,0) inJ7(0); P(f,t) = 0 on fln(0) x (0,T). (2.8)

System (2.6)-(2.8) contains N + 1 equations for defining JV components of the particle

trajectory X = (Xi,..., X

) and the pressure P along the trajectory.

Let us notice that to formulate problem (2.6)-(2.8) we only used a resemblance between

an evolution equation and the mass balance law in the motion of a continuous medium,

which is not a rigorous justification of the performed change of the independent variables.

The next indispensable step is to answer the question whether the constructed solution

of the auxiliary problem (2.6)-(2.8) generates a solution of the original free-boundary

problem.

3 The inverse transformation

In this section, we check that under certain conditions the solution of the auxiliary fixed-

boundary problem (2.6)-(2.8) allows us to recover a weak solution of the original free-

boundary problem for equation (1.1).

278

A weak solution of the Cauchy problem for equation (1.1) is understood in the following

sense.

Definition 3.1 A function u(x,t) is said to be weak solution of the Cauchy problem for

equation (1.1) in the strip S

—

R" x (0,T) if

u(x, t) is bounded, non-negative, and continuous in S;

for every test-function

r](x,

t) € C^S) such that

TJ(X,

t) = 0 when t = T and \x\ > R

with some R > 0, the following identity holds:

/ p(x)u(x, 0)rj(x, 0) dx

+ / (p(x)t]

u-u'V

r]-V

p + u'w(p) •Vr) + unh(u))dxdt = 0, p = f(u).

Let the pair (X(£,£),P(£,i)) be a solution of problem (2.6)-(2.8). Let us assume that

P(£,£) is non-negative, bounded and continuous in Q, that P = 0 on 9Q(0), and that

M/'H-P))

and

|(^

-1

P + w| are bounded in Q. Define the

mapping

x = X(£,t) for(£,i)eQ, *(£,(>) = £ for£en(0),

and assume that for every t e (0,T) it is a bijection of 17(0) onto

Cl(t),

and that \J\ ^ 0

il(t).

Set

X(£,t)=t- f

{{J-

P + w(P)) dr, $ e 0(0), (3.1)

and

(x,t) =

{

ifx =

JC(C,*)

with

ei^y,

(0 otherwise.

The functions p(x,t) and u = /

(p) defined in this way are non-negative because of

(2.7),

bounded and continuous in R" x

[0,T],

assume the initial values by continuity and

satisfy equation (1.1) in the weak sense. Indeed, given an arbitrary function tp{x,t) 6

x [0,T]), vanishing for large |a;| and for t = T, the following relations hold:

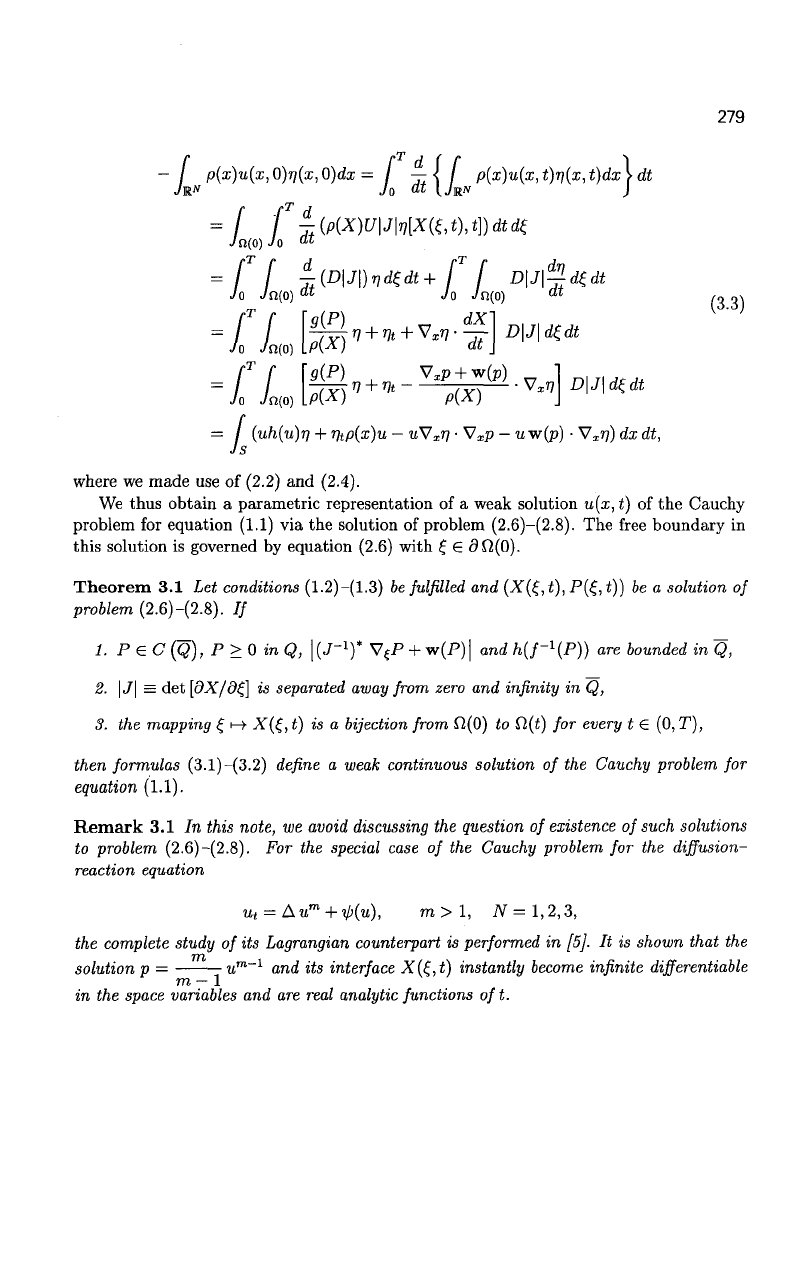
279

- / p(x)u(x,0)r](x,0)dx = / —

/ p(x)u(x,t)r)(x,t)dx \ dt

JRN

Jo at [J

N )

= f f ±(p{X)U\J\Ti[X(t,t),t])dtdt

Jn(o) Jo

= f f £(D\J\)ridtdt+ [

[ D\J\%d£dt

Jo Jn(o)

Jo Jrt{o)

-JT/„[^'

--T]

I«*

D\J\d£dt

(3.3)

r f \g(P)

VXP + W(

) '

(uh{u)rj + r]

p(x)u

—

•

—

u w(p)

•

V^jy) cfc dt,

where we made use of (2.2) and (2.4).

We thus obtain a parametric representation of a weak solution u(x, t) of the Cauchy

problem for equation (1.1) via the solution of problem (2.6)-(2.8). The free boundary in

this solution is governed by equation (2.6) with £ € 9O(0).

Theorem 3.1 Let conditions (1.2)-(1.3)

fulfilled and (X(£,t),P(£,t)) be a solution of

problem (2.6)-(2.8). If

P eC(Q), P>0 inQ,

-1

P + w(P)| andh{f-

(P)) are bounded in Q,

\J\ = det [dX/d£] is separated away from zero and infinity in Q,

the mapping £

>->•

X((, t) is a

bisection

from fl(0) to

Cl(t)

for every t €

(0,

T),

then formulas (3.1)-(3.2) define a weak continuous solution of the Cauchy problem for

equation (1.1).

Remark 3.1 In this note, we avoid discussing the question of existence of such solutions

to problem (2.6)-(2.8). For the special case of the Cauchy problem for the diffusion-

reaction equation

= Au

il)(u),

m>l, JV = 1,2,3,

the complete study of its Lagrangian counterpart is performed in [5j. It is shown that the

solution p = «

m_1

and its interface X(t;,t) instantly become infinite differentiable

m—1

in the space variables and are real analytic functions of t.