Teodorescu P.P. Mechanical Systems, Classical Models Volume I: Particle Mechanics

Подождите немного. Документ загружается.

MECHANICAL SYSTEMS, CLASSICAL MODELS

280

Replacing in (4.2.62), (4.2.62') and translating the axis

Ox so that the origin does

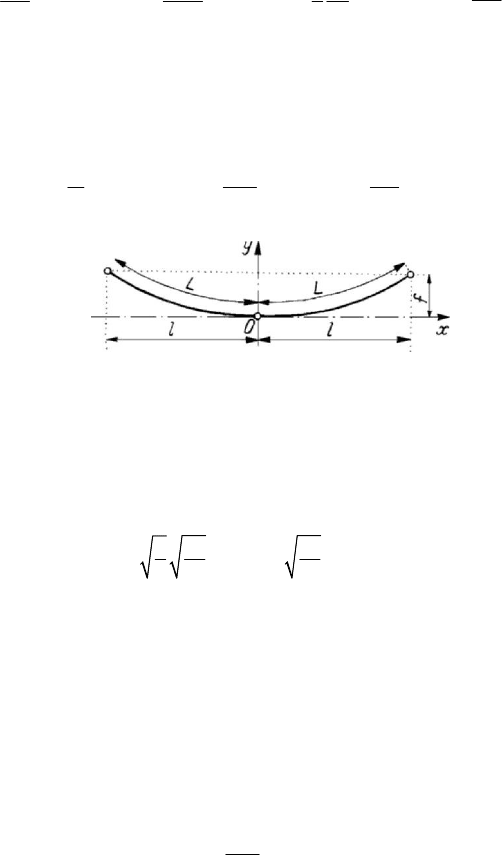
coincide with the vertex of the catenary curve (which is now a parabola), we obtain

(Fig.4.49)

Lx x x

⎛⎞

=+ = +

⎜⎟

⎝⎠

TH x H

=+ ≅

(4.2.63)

Assuming that the points

P and

P have the same ordinate ( 0h

), equal to the

deflection

of the thread, and noting that

lll

, we may also write (

/2al f= )

= ,

=+ ,

≅=

(4.2.63')

Figure 4.49. Parabolic curve.

We remark the great influence of a small variation of the length

of the thread on

the deflection

(due – for instance – to dilatation or contraction), the distance

between the points of suspension remaining constant. If

Ll l

+Δ , then the second

relation (4.2.63') leads to

1, 225

=≅

;

(4.2.64)

thus, for a variation of the length

2L given by / 0, 0001ll

= (e.g., 21lΔ= cm for

2100l = m), we obtain 0, 01225

(hence, 61

≅

cm).

Analogously, one can study the case of a thread fixed at the extremities and acted

upon by a distributed load of constant direction, proportional to the length of the

projection of the element of thread on a plane normal to the direction of the force; using

the same frame of reference as in the previous case (Fig.4.49), we may introduce a

uniformly distributed load of intensity

by the relation ddps qx

. The equation

(4.2.60) holds further and becomes

wherefrom, by integration,

Statics

281

()

=−

()

yy xx

−= −

By a translation of the co-ordinate axes, we find again the results of the preceding case

of the catenary curve with a relatively small deflection, in the form

= ,

222

()Tx H qx=+.

(4.2.65)

Hence, the funicular curve is a parabola; these results are used – for instance – in the

case of threads acted upon by loads laid down by the snow.

2.2.3 Threads acted upon by concentrated loads

If the external load ()sp is no more distributed, the thread being acted upon by one

or several concentrated loads, the equation of equilibrium (4.2.57) can no more be

applied in the usual manner. In this case, noting that a thread acted upon by only two

concentrated forces at two distinct points of it is in equilibrium if and only if the two

forces have the same modulus, the same support and opposite directions; the

configuration of equilibrium of this portion of thread is a segment of a line which

coincides with the common support of the two forces. Thus, the thread may be

assimilated to an open articulated system, the problem of equilibrium being thus

reduced to the problem of determination of the funicular polygon of the external given

forces; various conditions which may be imposed to this polygon, function of the given

limit conditions, have been discussed in Subsec. 2.1.9. Analytically, the study of such a

thread can be made writing the equations of equilibrium for each point of application of

a concentrated load.

To pass over these difficulties of computation, as it was shown by W. Kecs and P.P.

Teodorescu, one may use the methods of the theory of distributions. Thus, we can admit

that the equation (4.2.57) maintains its form in distributions,

()sT and ()sp being

distributions, while the differentiation is made in the sense of the theory of

distributions; besides, this equation may be obtained directly in distributions, in an

analogous manner. Using the formulae (1.1.50), (1.1.51) and assuming that the tension

()sT is a regular distribution, we may write

()

d() d()

()

−

=+Δδ−

∑



(4.2.66)

where “tilde” indicates the derivatives in the usual sense, while

()

T are the jumps of

the tension at the points of discontinuity

1,2,..., 1in

−

. We decompose the

external load in the form

()

() ()

ss ss

−

=+δ−

∑



pp F

(4.2.66')

MECHANICAL SYSTEMS, CLASSICAL MODELS

282

where

()s



are forces in the usual sense (distributed loads), while

F are concentrated

forces, applied at the points

1,2,..., 1in

−

. In this case, the equation (4.2.57)

can be decomposed in the form

d()

()



(4.2.67)

()

Δ+=TF0, 1,2,..., 1in

− ;

(4.2.67')

the equation (4.2.67) coincides with the classical one, while the

1n −

relations

(4.2.67') must take place for the points of application of the concentrated forces.

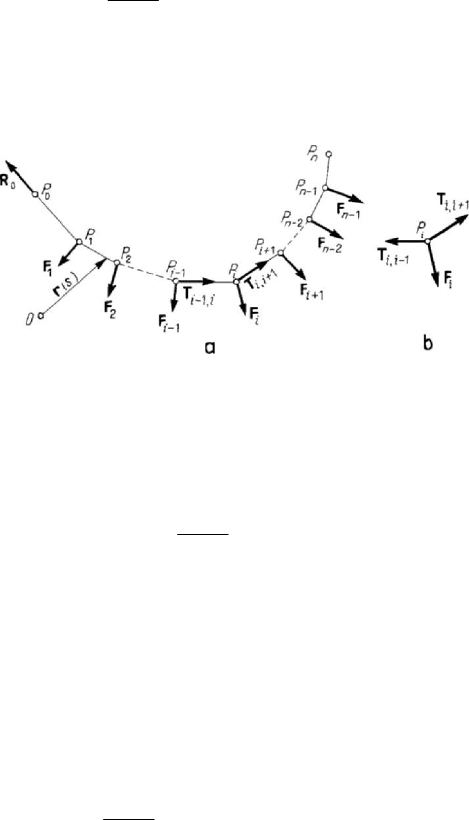
Figure 4.50. Thread acted upon by concentrated forces (a). Local equilibrium at a vertex (b).

Let thus be a thread acted upon only by the concentrated forces

F ,

1,2,..., 1in=−, applied at the points

12 1

...

ss s

−

<< ; the equation (4.2.67)

becomes

d()



wherefrom we see that the tension

()sT is piecewise constant, so that the funicular

curve is a funicular polygon (Fig.4.50,a). On the other hand, we notice that

,1 1,

()

ii i i

−

Δ= −TT T,

1, , 1ii ii−−

=TT 0;

hence, the equation (4.2.67') corresponds to the equilibrium of the point

P , subjected

to the action of the concentrated force

F and of the tensions

,1ii−

T and

,1ii+

(Fig.4.50,b). Integrating the equation

()

d()

−

δ− =

∑

we get

()

sss

−

=− θ − −

∑

TF R;

(4.2.68)

Statics

283

we put thus in evidence the tension at each point of the thread, excepting the points of

discontinuity, but at these points appear the corresponding jumps. We denote by

=RT the constraint force applied at the point

P . If ()sr is the position vector of

a point of the thread and if we take into account the formula (4.1.9), then we obtain

()

d()

() ()

ssss

sT T

−

⎡

⎤

== =− θ−+

⎢

⎥

⎣

⎦

∑

TFR

where

()

Tss

−

⎡

⎤

== + θ−

⎢

⎥

⎣

⎦

∑

TR F .

(4.2.68')

Noting that the unit vector of the tangent to the curve of equilibrium has only

discontinuities of the first species and taking the origin at the point

P , we get, by

integration,

()

(1)

()

sssss

≥

−

=−+−

⎡⎤ ⎡⎤

⎢⎥ ⎢⎥

⎣⎦ ⎣⎦

∑∑

∑

∑∑

(4.2.68'')

for

1kk

sss

≤≤

, 0,1,2,..., 1kn=−, with

and

, where

is the

total length of the thread; as well, it was denoted

. We have thus obtained the

vector equation of the searched funicular polygon.

2.2.4 Threads constrained to stay on a surface

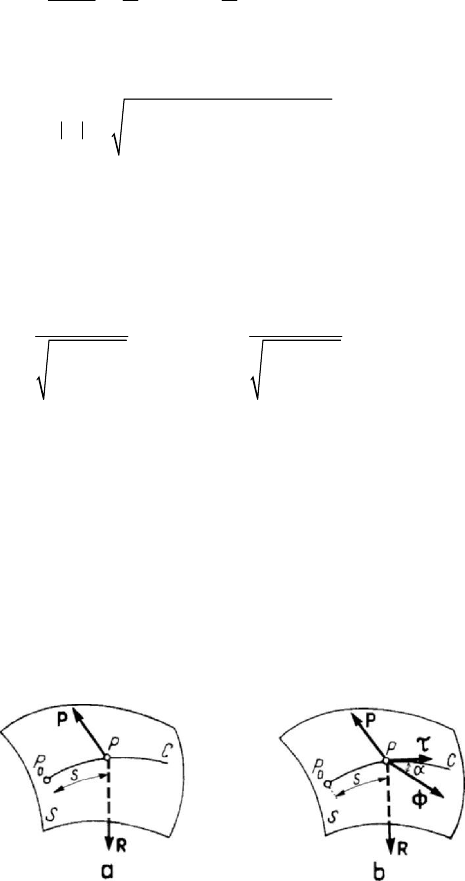
We consider first of all a thread C staying on a smooth surface S , supposing that

the external forces which act on it are distributed; in the case of concentrated forces,

one can adapt the results of the preceding subsection. Let be

(

)

123

,, 0

xxx

(4.2.69)

Figure 4.51. Equilibrium of a thread on a frictionless surface (a)

or on a surface with friction (b).

MECHANICAL SYSTEMS, CLASSICAL MODELS

284

the equation of the surface, with respect to a right-handed orthonormed frame of

reference

Ox ,

1, 2, 3i =

. An element ds of the thread is acted upon by a given

external force

()dssp and by the constraint force ()dssR , where

grad

λ=R

is along the normal to the surface), λ being an indeterminate scalar (Fig.4.51,a). The

equation of equilibrium is written in the form

d()

() ()

pR 0

(4.2.70)

or, in projection on the co-ordinate axes,

(

)

Tpf

+=, 1, 2, 3i

(4.2.70')

Thus, the equations (4.2.69), (4.2.70') and the relation (4.2.59') allow the determination

of the unknowns T and λ , and of the co-ordinates

123

,,xxx as functions of s .

Projecting the equation

d() d()

() () () ()

Ts s

sTs s s

++ =

τ pR 0

on the axes of the intrinsic Darboux’s trihedron and taking into account the first

formula (4.1.25), we get the equations

+=,

(4.2.71)

In particular, if the thread is not acted upon by an external given load (

=p0), then

constT = , 1/ 0ρ = and /

RTρ

− , the thread staying along a geodesic line of

the surface (case mentioned in Subsec. 2.2.1); if

, then the thread is not

extended, its form being arbitrary.

If the surface

S is rough, then its reaction on an element of thread will have, besides

the normal component

()dssR , a tangential component ()dss

too, called force of

sliding friction. The equations of equilibrium in Darboux’s trihedron become

cos 0

Φα++ =, sin 0

Φα

+=, 0

+=,

(4.2.72)

where

Φ , while α is the angle made by the force

with the unit vector τ

(Fig.4.51,b), tangent to the thread; according to Chap. 3, Subsec. 2.2.12, one must have

RΦ

≤

(4.2.72')

for equilibrium, where

is a Coulombian coefficient of friction (of sliding). It follows

that, for the same external load

()sp , the configurations of equilibrium C of the thread

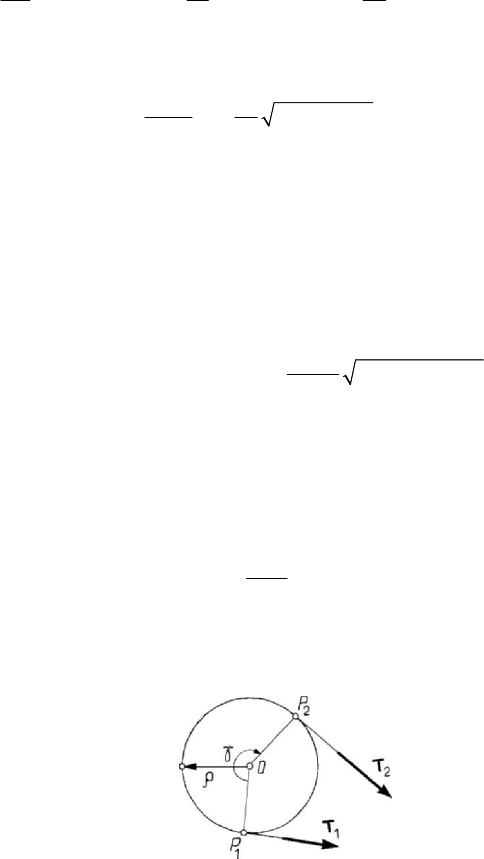
Statics

285

on the surface

S will be contained between two limit curves

C and

C . In the limit

case (the sign “=” in the relation (4.2.72')), assuming that the thread is not acted upon

by external loads (

=p0), we may write

cos 0

α+=

, sin 0

= , 0

= .

(4.2.73)

Eliminating

R and α between these relations, we obtain the differential equations

ext

tan d

=± −

for the extreme values of the tension

T , where we took into account the formulae

(4.1.19), (4.1.21),

θ being the angle formed by the normal n to the surface S with the

principal normal

ν to the line C . We obtain thus Euler’s inequalities

max

min

()TTsT

≤

≤ ,

(4.2.74)

with

min

eTT

χ−

= ,

max

eTT

= ,

tan ( )d

()

fχθσσ

ρσ

=−

∫

(4.2.74')

where

is the tension at the point

, origin of the considered co-ordinates.

Denoting

tan

ϕ=

, where ϕ is the angle of sliding friction, we notice that, for

equilibrium, one must have

θϕ

≤

. If the thread is along a geodesic line of the surface,

then

0θ =

, so that

()

ρσ

∫

(4.2.75)

where, on the basis of Meusnier’s formula (4.1.19),

ρρ

, ρ being the radius of

curvature of the curve

C .

Figure 4.52. Thread wrapped up a circular cylinder along a director curve of it.

In particular, we consider the case of a thread wrapped up a circular cylinder, along

a director curve of it (a geodesic line of the cylinder), case which appears often in

MECHANICAL SYSTEMS, CLASSICAL MODELS

286

practice. Let

P and

P be two extreme points of the thread on the rough cylinder

(Fig.4.52). We may calculate

()

χψγ

ρσ

===

∫∫

so that Euler’s relation

γγ−

≤≤

(4.2.76)

where

γ is the arc, measured in radians, covered by the thread, must hold for

equilibrium. Because

increases rapidly with γ , for great values of this argument,

one may equilibrate a force of great intensity, which acts at an extremity of the thread,

by a force of a relative small intensity, applied at the other extremity; for instance, for

0.25

= , a contact of the thread on half of the circumference ( γπ

) or on a whole

circumference or on two or four circumferences, we are led to an amplification of the

tension in the thread

2.19 times or 4.81 times or 23.14 times or 535.49 times,

respectively.

A geodesic line of the cylinder is – in general – a helix, and we can make an

analogous study in this case too.

Chapter 5

KINEMATICS

Kinematics deals with the motion of mechanical systems in time, without taking into

account their masses and the forces that act upon them; thus, its object of study is the

geometry of motion. We consider the kinematics of the particle, developing the notions

of velocity and acceleration; as well, the kinematics of rigid solids and the kinematics of

mechanical system – in general – are dealt with, emphasizing the relative motion too.

1. Kinematics of the particle

We consider the motion of a particle (material point) with respect to a fixed frame of

reference, emphasizing thus its trajectory, velocity and acceleration; the results thus

obtained are particularized for some important cases.

1.1 Trajectory and velocity of the particle

In what follows, we define the trajectory and the velocity of a particle, as well as the

horary equation of motion; we specify then the velocity in curvilinear co-ordinates and

in some particular systems of co-ordinates.

1.1.1 Trajectory. Horary equation of motion. Velocity

We have introduced in Chap. 1, Subsec. 1.1.4 the notion of frame of reference with

respect to which the motion is studied, using arbitrary curvilinear co-ordinates or, in

particular, spherical co-ordinates, cylindrical co-ordinates, or orthogonal Cartesian

co-ordinates. As well, in Chap. 1, Subsec. 1.1.5, we have shown that a particle

describes a trajectory

C (Fig.1.5), of vector equation (1.1.6) or of parametric

equations (1.1.16')-(1.1.16

), which define the law of motion. The functions which are

involved must be continuous and bounded in modulus for

[

]

,tT tt

∈

≡ ; they must

be differentiable too, excepting – eventually – a finite number of moments,

distinguishing thus between a continuous motion (the functions are everywhere of class

C ) and a discontinuous motion. If the trajectory is a rectifying curve, the mapping

(1.1.17) (or (1.1.18')) is the horary equation of motion.

By analogy with the relation (1.1.18'), a particle

P verifies the law of motion

(

)

;t=rrr,

(

)

;

iii

xxtx=

1, 2, 3i

(5.1.1)

287

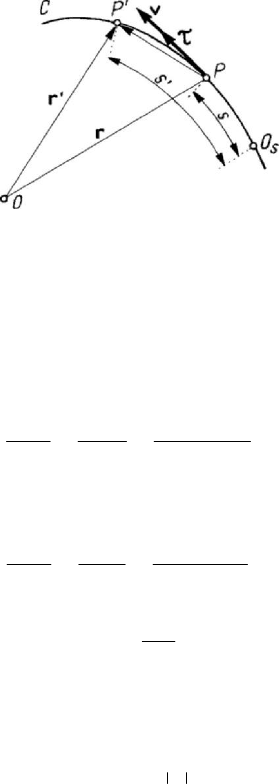
MECHANICAL SYSTEMS, CLASSICAL MODELS

288

where

x=ri corresponds to the position

P of the particle P at the initial moment

Figure 5.1. Trajectory and velocity of a particle.

It was also shown that the first derivative



is an invariant with respect to a change

of fixed frame of reference. To emphasize the mechanical significance of this

derivative, we will study how the particle

P moves on the trajectory. Let P and P

′

the positions of the particle at time

t and time t

′

, respectively; the mean velocity of

the particle in the interval of time

′

−

is defined in the form (Fig.5.1)

mean

() ()tt

tttt tt

′

′′

−

== =

′′ ′

−

−−

JJJG

(5.1.2)

Analogously, the mean magnitude of the velocity is given by

mean

() ()st st

PP s s

tttt tt

′

′′

−

===

′′ ′

−

−−

;

(5.1.2')

we notice that this magnitude coincides with the modulus of the mean velocity only by

identifying the arc

′

with the chord PP

′

. Passing to the limit, we obtain the

instantaneous velocity (the velocity at the point

P )

mean

lim

′

→

=vv,

mean

lim

′

→

(5.1.3)

supposing that these limits exist; in this case,

v . It follows that the velocity of a

particle

P is given by (for the sake of simplicity, we renounce to the index P )



vr, (5.1.4)

and is expressed by the derivative of the position vector with respect to time

(corresponding to the formulae (1.1.20')). We notice that the instantaneous velocity

(which, as a matter of fact, will be used) is an ideal notion, because only mean

velocities can be practically measured; the approximation is the best if one considers, in

the series of the mean velocities, terms which correspond to as small as possible

Kinematics

289

difference

′

− , tending to the instantaneous velocity. The components of the velocity

in orthogonal Cartesian co-ordinates are given by

 , 1, 2, 3i

(5.1.4')

x being the velocities of some particles situated in the projections of the point P on

the three axes of co-ordinates. It results that the modulus of the velocity is given by

ii ii

vvv xxs

== ,

(5.1.5)

where we took into consideration the relations (1.1.18). The velocity

v of the particle

on the trajectory (which is a scalar) is thus the modulus of the velocity v (vector

quantity); the horary equation of motion is obtained by the integration of the differential

equation

d()dsvtt

(5.1.5')

obtaining the formula (1.1.18'). If

() constvt v

= , the motion is called uniform; in

this case

svts

+ . (5.1.6)

Passing to the limit in formula (5.1.3) we notice that the oriented segment

′

JJJG

situated along the chord

′

tends to the tangent to the trajectory at the point P , the

velocity v enjoying this property. In intrinsic co-ordinates (after Frenet’s trihedron) we

may thus write

, 0vv

= , (5.1.7)

so that

. (5.1.7')

We notice that the velocity vector is applied at the point

P , its direction

corresponding to the direction of the motion.

1.1.2 Velocity of a particle in curvilinear co-ordinates

Let be a system of curvilinear co-ordinates

123

,,qqq, linked to the position vector

and to the orthogonal Cartesian co-ordinates by relations of the form (A.1.32), (A.1.33).

Taking into account the formulae (A.1.35) and the notation (A.1.36), the velocity of a

particle is expressed in the form

iiiii

qq v

∂

===

∂



vee,

(5.1.8)