Salby M.L. Fundamentals of Atmospheric Physics

Подождите немного. Документ загружается.

390 12

Large-Scale Motion

are the horizontal components of vector vorticity ~" = (~:, r/, ~'). Then the

budget for the vertical component of vorticity becomes

+ f) ew ew)

= -(st + f)V~

. v h + \ 3x + rl--~y + (Vzp

x V~a).

(12.36)

wherein the Lagrangian derivative includes full three-dimensional advection.

According to (12.36), the absolute vorticity of a material element can change

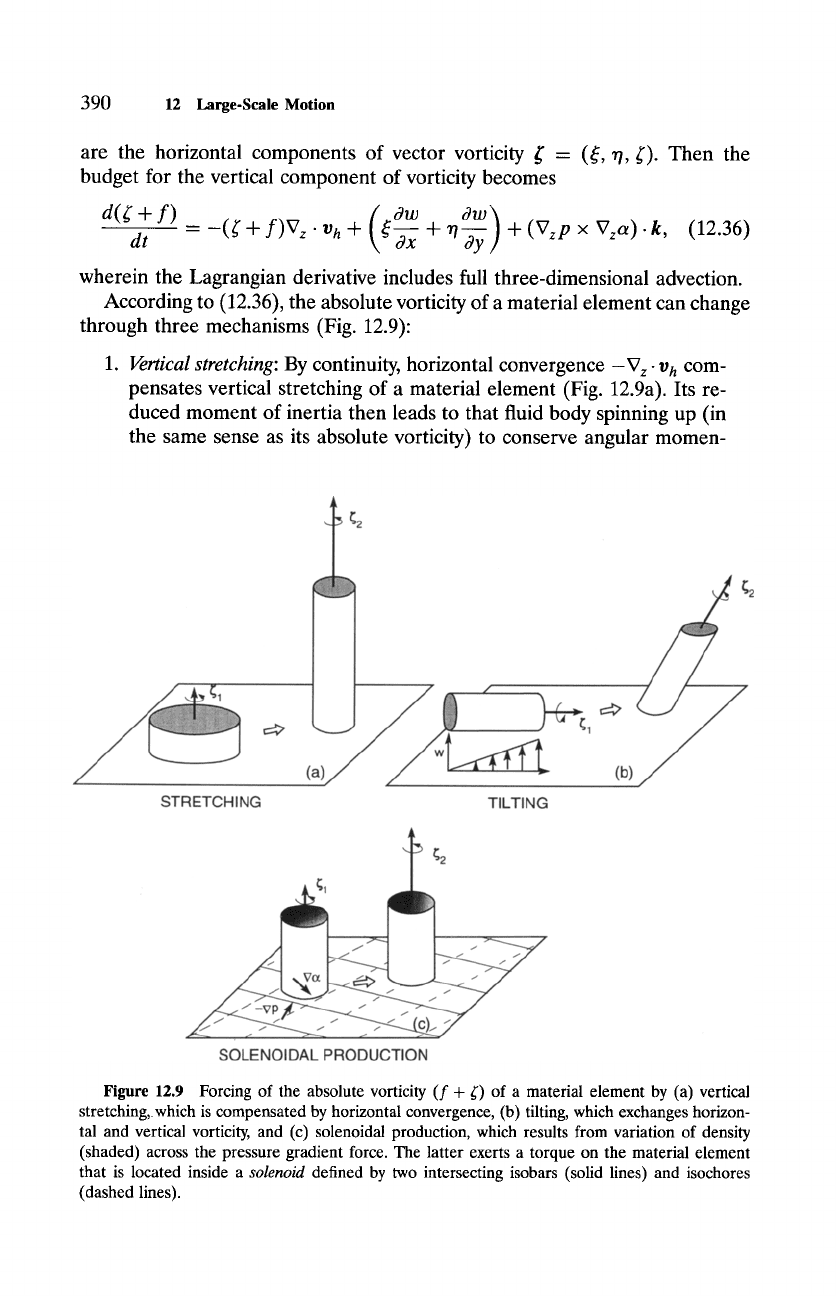
through three mechanisms (Fig. 12.9):

1. Vertical stretching:

By continuity, horizontal convergence

-Vz.V h

com-

pensates vertical stretching of a material element (Fig. 12.9a). Its re-

duced moment of inertia then leads to that fluid body spinning up (in

the same sense as its absolute vorticity) to conserve angular momen-

,.:3 r~2

(a)

(b)

STRETCHING TILTING

SOLENOIDAL PRODUCTION

Figure

12.9 Forcing of the absolute vorticity (f + ~') of a material element by (a) vertical

stretching, which is compensated by horizontal convergence, (b) tilting, which exchanges horizon-

tal and vertical vorticity, and (c) solenoidal production, which results from variation of density

(shaded) across the pressure gradient force. The latter exerts a torque on the material element

that is located inside a

solenoid

defined by two intersecting isobars (solid lines) and isochores

(dashed lines).

12.5 Weakly

Divergent Motion

391

tum. Horizontal divergence leads to the material element spinning

down to conserve angular momentum.

2. Tilting:

Horizontal shear of the vertical motion

Vzw

deflects a ma-

terial element and its vector angular momentum (Fig. 12.9b). This

process transfers vorticity from horizontal components into the verti-

cal component. Strong vertical shear of the horizontal motion (e.g.,

as accompanies a sharp temperature gradient) magnifies the compo-

nents s c and 77, which then make tilting potentially important as a

source of vertical vorticity.

3. Solenoidal production:

Under baroclinic stratification, isobars on a

surface of constant height do not coincide with isochores. Two sets

of intersecting isobars and isochores then define a

solenoid,

across

which pressure varies in one direction and density varies in another

(Fig. 12.9c). Variation of density across the pressure gradient force

then introduces a torque, which changes the angular momentum of

the material element occupying a solenoid. Under barotropic stratifi-

cation, density is uniform across the pressure gradient, so the torque

and solenoidal production of vorticity V~p • V~c~ vanish.

Each of these forcing mechanisms reflects a rearrangement of angular

momentum~not dissipation, which has been excluded by considering adiabatic

and inviscid conditions. Scale analysis indicates that vertical stretching domi-

nates the forcing of absolute vorticity in large-scale motion (Problem 12.44).

Because other mechanisms on the right-hand side of (12.36) follow from baro-

clinic stratification, this is another reflection of the nearly barotropic nature

of the large-scale circulation.

Under adiabatic conditions, tilting and solenoidal production disappear for-

mally when the vorticity budget is expressed in isentropic coordinates. Making

use of vector identities in Appendix D allows the horizontal momentum equa-

tion (11.92.1) to be expressed

&v h 1 8Vh

-- -V0XI*, (12.37)

at ~- -2 v~ (Vh " Vh) + (~0 + f)k x Vh + oo0 ao -

where st0 - (V 0 x

Vh). k

is the relative vorticity evaluated on an isentropic

surface. Under adiabatic conditions, the term representing vertical advection

disappears. Applying k. V0• to (12.37) then obtains the vorticity budget

a( o + f)

+ Vh.

V0( 0 + f) = +

f)Vo'Vh

d((o + f)

= --(~0 -+- f)Vo'Vh,

(12.38)

in which the Lagrangian derivative involves only horizontal advection. Because

vertical motion vanishes identically, so does the tilting apparent in physical co-

ordinates (12.36). Likewise, solenoidal production vanishes because the ver-

tical vorticity if0 is evaluated on an isentropic surface (Problem 12.48). The

392

12 Large-Scale

Motion

continuity equation in isentropic coordinates (11.92.3) reduces to

dt --~ + --~ V ~ " v h = O

under adiabatic conditions. Combining this with (12.38) yields

1 d(~+f)_ 1 d {dp~

(~ + f) dt - {ap] dt ~ ] -~ '

where

(12.39)

~o+ f

Q = (12.40.2)

1( 0)

defines the

potential vorticity,

also termed the

Ertel potential vorticity

(Ertel,

1942) and the

isentropic potential vorticity.

A generalization of the conservation principle for barotropic nondivergent

motion, (12.40) asserts that the potential vorticity of an air parcel is conserved

under inviscid adiabatic conditions. The parcel's absolute vorticity (st0 + f)

can change, but in direct proportion to the vertical spacing

-(1/g)ap/aO

isentropic surfaces (11.85), which are material surfaces under adiabatic condi-

tions. Despite its name, the conserved property Q does not have dimensions

of vorticity, the denominator in (12.40.2) introducing other dimensions.

Potential vorticity is a dynamical tracer of horizontal motion. Under the

same conditions that Q is conserved, vertical motion is determined by varia-

tions in the elevation of an isentropic surface, on which Q is evaluated. Unlike

chemical tracers, potential vorticity is not advected "passively" by the circula-

tion. Rather, the vorticity at a point induces motion about it, which makes Q

and the motion field completely dependent on one another. According to the

so-called

invertibility principle

(Hoskins

et al.,

1985), the distribution of Q at

some instant uniquely determines the circulation. The conserved property Q

can therefore be regarded as being self-advected.

In practice, friction and diabatic effects are slow enough away from the sur-

face and outside convection for Q to be conserved over many advection times.

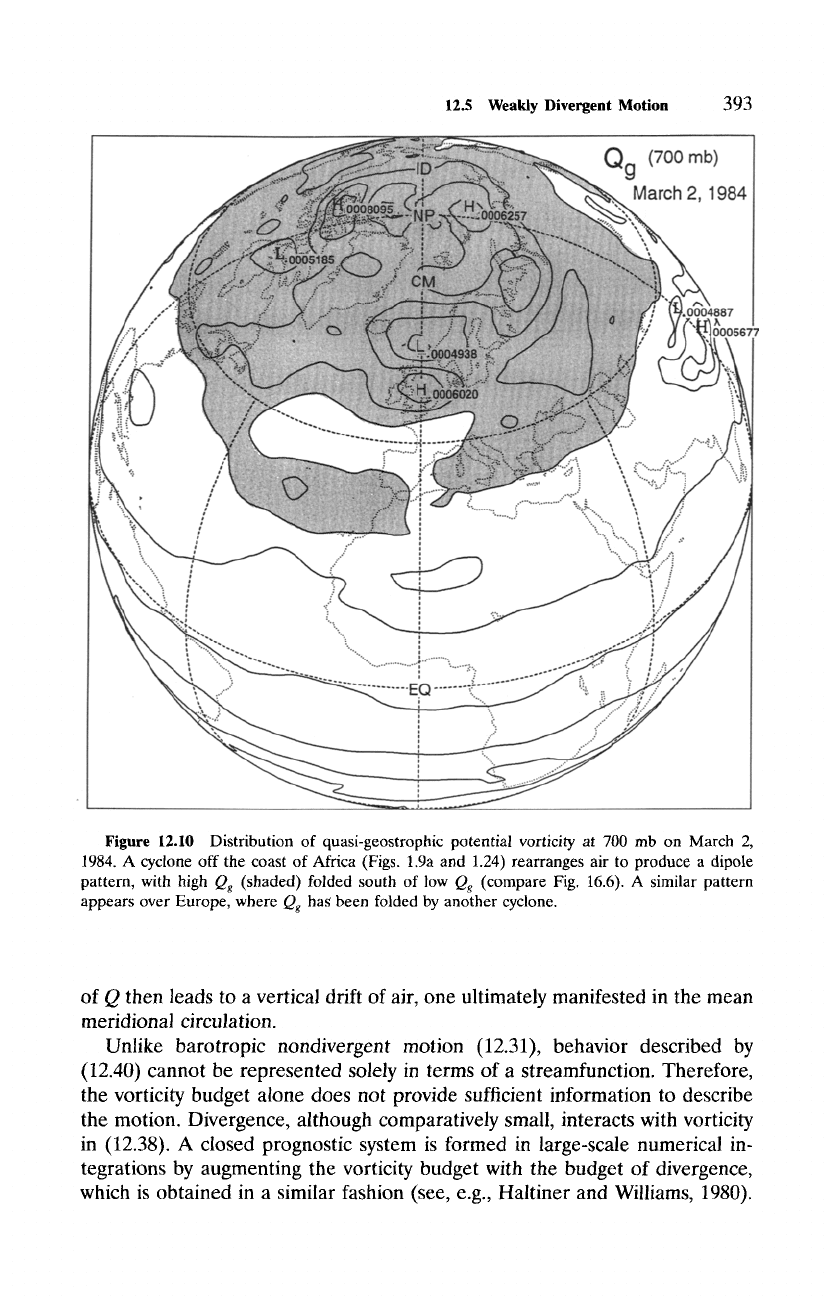
Synoptic disturbances are marked by anomalies of potential vorticity (see Fig.

12.10). Hence, forecasting such disturbances amounts to predicting the distri-

bution of Q, which is controlled primarily by advection. Over timescales much

longer than a day, dissipative processes lead to production and destruction

of Q (e.g., inside an individual air parcel). Nonconservative effects associated

with turbulence, radiative transfer, and condensation eventually render the

behavior of an air parcel diabatic, which introduces motion across isentropic

surfaces (Sec. 3.6.1). Irreversibility associated with production and destruction

= 0, (12.40.1)

12.5 Weakly Divergent Motion 393

(700 mb)

March 2, 1984

~:..

"..:.

\',,!

:":"" ~

-: ,

-( j

--:.. ~'~. ,~____..~2~~

.... i... .........

-. ....................

, :

...... :-..

"'~ ...... EQ; ....... .--.. ~i

...}

"-....

~i~

--.'..

~--. ..... ~ .....:...:..,

... ......... 9 ......................... ~ ....

; ........ ;....-......

"..... ",..

.:p

", ..... -.~-...." i ,

0005677

Figure 12.10 Distribution of quasi-geostrophic potential vorticity at 700 mb on March 2,

1984. A cyclone off the coast of Africa (Figs. 1.9a and 1.24) rearranges air to produce a dipole

pattern, with high Qg (shaded) folded south of low

(compare Fig. 16.6). A similar pattern

appears over Europe, where

has been folded by another cyclone.

of Q then leads to a vertical drift of air, one ultimately manifested in the mean

meridional circulation.

Unlike barotropic nondivergent motion (12.31), behavior described by

(12.40) cannot be represented solely in terms of a streamfunction. Therefore,

the vorticity budget alone does not provide sufficient information to describe

the motion. Divergence, although comparatively small, interacts with vorticity

in (12.38). A closed prognostic system is formed in large-scale numerical in-

tegrations by augmenting the vorticity budget with the budget of divergence,

which is obtained in a similar fashion (see, e.g., Haltiner and Williams, 1980).

394

Large-Scale Motion

12.5.3 Quasi-Geostrophic Motion

An approximate prognostic system governing large-scale motion, which is use-

ful for many applications, can be derived via an expansion in

Rowas

was

used to introduce the diagnostic description of geostrophic motion. For the

resulting system to remain prognostic, the expansion must then retain terms

higher order than those in (12.2) and, in particular, it must retain horizontal

divergence that forces changes of absolute vorticity (12.38).

We consider motion with meridional displacements that are

O(Ro)

and

hence sufficiently narrow to ignore the earth's curvature. Analogous to the f-

plane development, spherical geometry is approximated in a Cartesian system

tangent to the earth at latitude 4'0 (Fig. 12.3). For consistency with other terms

in the equations, the variation with latitude of the Coriolis force must now be

retained to order

Ro.

Expanding f in a Taylor series in y (which reflects the

meridional displacement of an air parcel) and truncating to first order yields

the

~-plane approximation

f = fo +/3y, (12.41.1)

y=O

(12.41.2)

where f0 and y are given by (12.3). The motion is also presumed to satisfy the

so-called

Boussinesq approximation,

in which variations of density are ignored

except where accompanying gravity in the buoyancy force. The Boussinesq

approximation neglects compression of an air parcel introduced by changes

of pressure, retaining only density variations that are introduced thermally.

Vertical displacements of air must then be shallow (e.g., compared to H).

Following the development in Sec. 11.4, all variables are nondimensional-

ized by characteristic scale factors. Dependent variables are then expanded

in a power series in Rossby number. The dimensionless horizontal velocity

becomes

O h : ~)g + ROy a -+-...,

(12.42)

where t~g satisfies geostrophic balance with f = f0 and the

ageostrophic velocity

where o a defines an

O(Ro)

correction to it. Both are O(1), as are all dependent

variables in a formal scale analysis (see, e.g., Chamey, 1973), in which magni-

tudes of various terms are contained in dimensionless parameters like

Ro.

streamline the development, we depart from the formal procedure by absorb-

ing dimensionless parameters other than

into the dependent variables, the

magnitudes of which can then be inferred from the ensuing equations. This

allows the dimensionless equations to be expressed in forms nearly identical

to their dimensional counterparts.

12.5 Weakly Divergent Motion

395

In log-pressure coordinates, the horizontal momentum equation exclusive

of friction becomes

Ro ~

+ (llg _qt_

Rov~ +...). V~

9 (Vg +Roy a +...) +Row3

gz(Vg + Rov +...)

(12.43)

+ (fo + Ro[3y)k x (Vg -+- Roy a +...)

= --Vz(dPg + Ro~ a +...),

where z denotes log-pressure height (11.65) and factors such as 13 are under-

stood to be dimensionless and O(1). Because vg satisfies (12.2), the Ro ~ bal-

ance drops out of (12.43). Under the Boussinesq approximation, w is smaller

than O(Ro) [denoted o(Ro)], so vertical advection of momentum also drops

out to O(Ro). Then the momentum balance at O(Ro) is given by

dg l~g

d---7- + f~

x 11 a

+ flyk x Vg

- -Vzf~a,

(12.44.1)

where

dg d

= + Vg. Vz (12.44.2)

dt 3t

is the time rate of change moving horizontally with the geostrophic velocity.

According to (12.44), first-order departures from simple geostrophic equilib-

rium provide a time rate of change or tendency to Vg, which is absent at

O(Ro~

The continuity equation (11.72.3) becomes

oo oz (p~ - . + Rov +...)

= -RoV z . v a, (12.45)

so w follows directly from v a. The ageostrophic velocity introduces a secondary

circulation, which, unlike the O(Ro ~ geostrophic velocity, involves vertical

motion. Under adiabatic conditions, the thermodynamic equation (11.72.4)

becomes

d (~g + Ro~a + .) +

+ (Vg q-Rov a +...). V z -~z ""

N2w

dt 3z ] + w - O, (12.46)

where N 2 is evaluated with the basic stratification T 0. The Boussinesq approx-

imation requires N 2 to be large enough to render vertical displacements small,

so the second term in (12.46) must be retained to leading order.

Equations (12.44), (12.45), and (12.46) describe quasi-geostrophic motion

on a fi plane. Accurate to O(Ro), they have dimensional counterparts that

396

12 Large-Scale Motion

are nearly identical. By expressing the momentum budget as in (12.28) and

applying k. V z x, we obtain the vorticity equation

-1-- U~ --[-- s V z . V a = 0

dg(~g + f)

= --fOVz "lIa,

(12.47.1)

1 2 (12.47.2)

= --foVz~g

where

is the geostrophic vorticity. This is similar to the full vorticity equation in

isentropic coordinates (12.38), but the conditions of quasi-geostrophy make

the relative vorticity Srg negligible compared to the planetary vorticity f0 in the

divergence term. With the continuity equation, (12.47) can be written ~

d(srg + f) 1

dt = f~ -~z

(poW) .

Then applying the operator

(1/po)O/Oz(po)

to the thermodynamic equation

and eliminating w yields the

quasi-geostrophic potential vorticity equation:

dO,

= 0, (12.48.1)

where

[

Qg = V2z ~ + fo + flY + --

(12.48.2)

is the

quasi-geostrophic potential vorticity.

Since

dg/dt

involves only

Vg,

(12.48)

can be expressed in terms of the single dependent variable q~

o o ,o oq, o oo =o,

(12.49)

which is a generalization of the barotropic nondivergent vorticity equation

(12.31) to weakly divergent motion.

Equation (12.49) constitutes a closed prognostic system for the streamfunc-

tion 0. Like (12.31), it is quadratically nonlinear and can be integrated for the

motion field's evolution with suitable initial and boundary conditions. The con-

served property

is an analogue of isentropic potential vorticity (12.40.2),

4Under fully Boussinesq conditions, the stretching term simplifies to

foaW/az--the

same as

under incompressible conditions. With the density weighting retained, the approximation is re-

ferred to as

quasi-Boussinesq

and accounts for vertical structure associated with the amplification

of atmospheric waves (Chapter 14).

Suggested Reading

An Introduction to Dynamic Meteorology (1992) by Holton gives a complete

treatment of gradient wind solutions and provides an excellent introduction

to the concepts of vorticity and circulation. An advanced treatment of those

concepts is presented in Geophysical Fluid Dynamics (1979) by Pedlosky.

An Introduction to Fluid Dynamics (1977) by Batchelor contains a formal de-

velopment of vorticity and its relationship to angular momentum. The inter-

dependence of vorticity and stratification is discussed in The Ceaseless Wind

(1986) by Dutton.

Hoskins et al. (1985) provide an overview of isentropic potential vorticity and

different applications. Comparisons with quasi-geostrophic potential vorticity

are given in Atmosphere-Ocean Dynamics (1982) by Gill and in Middle Atmo-

sphere Dynamics (1987) by Andrews et al.

Introduction to Circulating Atmospheres (1993) by James discusses vertical mo-

tion in large-scale flows and contains a concise summary of quasi-geostrophic

relationships.

398

12 Large-Scale Motion

Problems

12.1. With the scaling in Sec. 11.4, perform an asymptotic series expansion of

dependent variables in the horizontal momentum equations to obtain

(12.1) accurate to

O(Ro ~

and then to

O(Rol),

where drag may be

regarded

O(Ro ~

inside the boundary layer and smaller elsewhere.

12.2. Recover the geostrophic velocity (12.2.2) from the vector equations of

motion.

12.3. Obtain expressions for the geostrophic velocity in (a) height coordinates

and (b) isentropic coordinates.

12.4. Write down explicit expressions for the geostrophic velocity components

in terms of geopotential for (a) Cartesian coordinates, (b) spherical

coordinates, and (c)vertical cylindrical coordinates.

12.5. Discuss why the Coriolis force is not apparent for laboratory-scale flow

in the reference frame of the earth.

12.6. For geostrophic motion in spherical geometry and isobaric coordinates,

(a) determine an expression for the vertical vorticity, (b) determine an

expression for the horizontal divergence, and (c) interpret the signif-

icance of the divergence in part (b) in light of observed circulations,

wherein divergence is strongly correlated to lows and highs.

12.7. The bullet train moves over straight and level track at 40 ~ latitude.

If the train's mass is 105 kg, how fast must it move to experience a

transverse force equal to 0.1% of its weight?

12.8. Interpret the velocity potential in relation to the general concept of a

potential function introduced in Sec. 2.1.

12.9. Demonstrate that the Helmholtz theorem reduces to (12.7.1) for a two-

dimensional vector field.

12.10. Consider a steady vortex with circular streamlines and azimuthal veloc-

ity v. (a) Determine the form of the velocity profile

v(r)

that possesses

no vorticity for r > 0. (b) Calculate the

circulation

F(r)

= f v(r). ds =

f2~ v(r)rd ck

about the vortex. (c) Use Stokes' theorem to interpret the

distribution of vorticity.

12.11. (a) Discuss the time during which a fluid body may be regarded as a

compact closed system (e.g., a finite-dimensional air parcel) in relation

to its size and deformation (see Problem 10.10). (b) After sufficient

time, what additional factors intervene to invalidate the description of

part (a)?

12.12. Show that geostrophic motion on an f plane automatically satisfies

conservation of mass.

12.13. (a) Determine the components of frictional geostrophic motion tangen-

tial and orthogonal to contours of height, in terms of the correspond-

ing geostrophic velocity. (b) Construct an expression for the angle 6 by

Problems

399

12.14.

12.15.

12.16.

12.17.

which

deviates from height contours. Estimate 6 for a characteristic

wind speed of 5 m s -a at a latitude of (c) 45 ~ and (d) 15 ~

(a) Derive an expression for the divergence of frictional geostrophic

motion on an f plane to show that, in the presence of Rayleigh friction,

V.Vh

is proportional to the V2~. (b) From this expression, infer how

vertical motion over a surface low depends on its latitude.

Consider a surface low that is narrow enough for motion about it to

be treated on an f plane. For Rayleigh friction with a timescale of

1 day, determine the latitude at which horizontal divergence inside the

boundary layer becomes comparable to vertical vorticity.

The surface motion beneath a cyclone is described by the stream func-

tion and velocity potential

qJ(x, y)- ~ { l-expI-(x2 +

]}

X(x

y)= Xexp[ -(x2

_+_ y2)]

' L 2 ,

where q~ and X are constants. Determine (a) the horizontal velocity,

(b) the horizontal divergence, and (c) the vertical vorticity. (d) Sketch

Vh(X, y)

and discuss it in relation to q~(x, y) and

X(x,

y). (e) Indicate

the horizontal trajectory of an air parcel and discuss it in relation to

three-dimensional air motion.

(a) Express the vertical velocity oJ in isobaric coordinates in terms of

the geometric vertical velocity w under the conditions of large-scale,

steady motion above the boundary layer. (b) Estimate the geometric

vertical velocity that corresponds to oo = 100 mb day -1 at 500 mb.

12.18. The 500-mb surface has height

z 00-z 00

zs00 - _ _ Y \

500 500 ~,a

12.19.

( x2+y )]

COS 7/" L2 (x 2 -k-y2)89 _< L

(x 2 + y2)~ >/~

where the constants 2500, 2500, and zs00 reflect global-mean, zonal-

mean, and perturbation contributions to the height field, respectively,

a is the radius of the earth, and y is measured on midlatitude f plane

centered at latitude ~b 0. (a) Provide an expression for the 500-mb

geostrophic flow vs00. (b) Sketch zs00(x, y) and vs00(x, y), presuming

z~00 < < 25oo. Discuss the contributions to vs00, noting how the flow is

influenced by the thermal perturbation. (c) Provide an expression for

the absolute vorticity at 500 mb and discuss its contributions.

(a) Determine the geostrophic motion accompanying the upper-level

depression in Problem 6.7. (b) Plot the vertical profile of horizontal

motion at (h, ~b) = (0, ~b 0 - L).