Potter T.D., Colman B.R. (co-chief editors). The handbook of weather, climate, and water: dynamics, climate physical meteorology, weather systems, and measurements
Подождите немного. Документ загружается.

extended to include other free energies affecting condensation or sublimation such as
solution effects, curvature effects, to process of chemical equilibrium and so on.
REFERENCES
Dutton, J. (1976). The Ceaseless Wind, McGraw-Hill.
Emanuel, K. A. (1994). Atmospheric Convection, Oxford University Press.
Hess, S. L. (1959). Introduction to Theoretical Meteorology, Holt, Reinhardt, and Winston.
Iribarne, J. V., and W. L. Godson (1973). Atmospheric Thermodynamics, D. Reidel Publishing
Co.
Sears, F. W. (1953). Thermodynamics, Addison-Wesley.
Wallace, J. M., and P. V. Hobbs, (1977). Atmospheric Science: An Introductory Survey,
Academic Press.
206
PHYSICAL ATMOSPHERIC SCIENCE
CHAPTER 16
MOIST THERMODYNAMICS*
AMANDA S. ADAMS
1 LATENT HEATS — KIRCHOFF’S EQUATION
Consider the effect resulting from mass fluxes between constituents of the parcel
within the system. In particular, mass fluxes between the three possible phases of
water are considered. We can express these phase transformations by:
dH ¼ dQ þ VdPþ
P
k
@H
k
@n
k
dn
k
ð1Þ
where @H
k
=@n
k
represents the energy per mole of the n
k
constituent of the system.
We let the system of moist air be compo sed of n
d
moles of dry air, n
v
moles of vapor
gas, n
l
moles of liquid water and n
i
moles of ice. Then
dH ¼ dQ þ VdPþ
@H
v
@n
v
@H
l
@n
l
dn
v
þ
@H
i
@n
i
@H
l
@n
l
dn
i
ð2Þ
Handbook of Weather, Climate, and Water: Dynamics, Climate, Physical Meteorology, Weather Systems,
and Measurements, Edited by Thomas D. Potter and Bradley R. Colman.
ISBN 0-471-21490-6 # 2003 John Wiley & Sons, Inc.
*The derivations, concepts, and examples herein are based partially on course notes from the late
Professor Myron Corrin of Colorado State University. Other derivations are taken from the Isbane and
Godson text, the Emanuel (1994: Atmospheric Convection, Oxford University Press) text, and the Dutton
(The Ceaseless Wind ) book.
207
We define latent heats to be the differences in enthalpy per mole between two phases
at the same temperature and pressure and is expressed as:
L
lv
@H
l
@n
l
@H
v
@n
v
L
il
þ
@H
i
@n
i
@H
l
@n
l
L
iv
L
il
þ L
lv
ð3Þ
L
lv
; L
il
, and L
iv
are the latent heats of condensation, melting, and sublimation
defined as positive quanti ties with units of joules per mole. Employing these defini-
tions, Eq. (2) can be written:
dH ¼ dQ þ VdP L
iv
dn
v
þ L
il
dn
i
ð4Þ
In order to find Kirchoff’s equation, we write Eq. (4) for three adiabatic homogenous
systems consisting of vapor, liquid, and ice held at constant pressure:
C
pv
T ¼
@H
v
@n
v
;
C
l
T ¼
@H
l
@n
l
; and
C
i
T ¼
@H
i
@n
i
:
ð5Þ
where C
pv
; C
l
, and C
i
are the heat capacity of vapor at constant pressure, the heat
capacity of liquid, and the heat capacity of ice. Applying (5) to (3) and differencing
with respect to temperature we obtain Kirchoff’s equation:
@L
lv
@T
¼ C
pv
C
l
;
@L
il
@T
¼ C
l
C
i
; and
@L
iv
@T
¼ C
pv
C
i
:
ð6Þ
208 MOIST THERMODYNAMICS
In the ‘‘enthalpy per mass’’ for m, Kirchoff’s equation is:
@l
lv
@T
¼ c
pv
c
l
;
@l
il
@T
¼ c
l
c
i
; and
@l
iv
@T
¼ c
pv
c
i
:
ð7Þ
Given heat capacities determined observationally as a function of temperature, we
can determine the variation of latent heat with temperature. Kirchoff’s law can also
be used to study reaction heats of chemical changes.
2 GIBBS PHASE RULE
For the case of a simple homogenous gas, we found that there were three indepen-
dent variables, being the pressure, temperature, and volume assuming the number of
moles was specified. Once we require that the gas behave as an ideal gas, the
imposition of the equation of state (and the implicit assumption of equilibrium)
reduces the number of independent variables by one to two.
If we now look at a system consisting entirely of water, but allow for both liquid
and gas forms, and again assume equilibrium, then not only must the vapor obey the
ideal gas law, but the chemical potential of the liquid must equal that of the vapor.
This means that if we kn ow the pressure of the vapor, only one water temperature
can be in true equilibrium with that vapor, i.e., the temperature that makes the liquid
exert a vapor pressure equal to the vapor pressure in the air. Hence by requiring
equilibrium with two phases, the number of degrees of freedom is reduced to one.
If we allow for all three phases, i.e., vapor, liquid, and ice, then there is only one
temperature and pressure where all three states can exist simultaneously, called the
triple point.
Now consider a two-component system such as one where we have water and air
mixed. Consider a system allowing only liquid but not the ice phase of water. Now
we have to consider the equilibrium as is applied to the dry air by the ideal gas law in
addition to the equilibrium between the liquid and ice water. For this case, the water
alone had one independent variable and the dry air had two, for instance, its partial
pressure and temperature. If the vapor pressure is specified, then the tem perature of
the water is specified and, for equilibrium, so is the air temperature. Hence adding
the additional component of air to the two-phase water system increased the number
of independent variables to two.
A general statement concerning the number of independent variables of a hetero-
geneous system is made by Gibbs phase rule, which states:
n ¼ c f þ 2; ð8Þ
2 GIBBS PHASE RULE 209
where n is the number of independent variables (or degrees of freedom), c is the
number of independent species, and f is the number of phases total. Hence for a
water–air mixture, allowing two phases of water and one phase of dry air, there is
1 degree of freedom. So if we specify vapor pressure, then liquid temperature is
known, air temperature is known, and so air pressure is fixed assuming the volume
of the system is specified.
3 PHASE EQUILIBRIUM FOR WATER
Figure 1 shows where equilibrium exists between phases for water. Note that equili-
brium between any two phases is depicted by a line, suggesting one degree of
freedom, while all three phases are only possible at the triple point. Note that the
curve for liquid–ice equilibrium is nearly of constant temperature but weakly slopes
to cooler temperatures at higher pressures. This is attributable to the fact that water
increases in volume slightly as it freezes so that freezing can be inhibited some by
applying pressure against the expansion.
Note also that at temperatures below the triple point there are multiple equilibria,
i.e., one for ice–vapor and another (dashed line) for liquid–vapor. This occurs
because the free energy necessary to initiate a freezing process is high, and local
equilibrium between the vapor and liquid phase may exist.
Note that at high temperatures, the vapor pressure curve for water abruptly ends.
This is the critical point ( p
c
) where there is no longer a discontinuity between the
liquid and gaseous phase. As we showed earlier, the latent heat of evaporation l
lv
decreases with increasing temperature. It becomes zero at the critical point.
Since the triple point is a well-defined singularity, we define thermodynamic
constants for water at that point, and these values are given in Table 1. At the critical
point, liquid and vapor become indistinguishable. Critical point statistics are given in
Figure 1 Phase-transition equilibria (from Iribarne and Godson, 1973).
210
MOIST THERMODYNAMICS

Table 2. These phase relationships can also be seen schematically with an Amagat–
Andrews diagram (Fig. 2). Note how the isotherms follow a hyperbola-like pattern at
very high tem peratures as would be expected by the equation of state for an ideal
gas. However, once the temperature decreases to values less than T
C
, an isothermal
process goes through a transition to liquid as the system is compressed isothermally.
The latent heat release and the loss of volume of the system keep the pressure
constant while the phase change occurs. After the zone of phase transition is crossed,
only liquid (or ice at temperatures below T
t
) remains, which has very low compres-
sibility and so a dramatic increase in pressure with further compression.
Phase equilibrium surfaces can be displayed also in three dimensions as a p-V-T
surface and seen in Figure 3. Here we see that at very high tem peratures, the region
where phases coexist is lost. Note how the fact that water expands upon freezing
results in the kink backward of the liquid surface. Note that we can attain the
saturation curves shown in Figure 1 by cutting cross sections through the p-V-T
diagram and constant volume or temperature (Fig. 3).
We can use these figures to view how the phase of a substance will vary under
differing conditions, since the phases of the substance must lie on these surfaces.
Figure 4 shows one such system evolution beginning at point a. Note the liquid
system exists at point a under pressure p
1
. The pressure presumably is exerted by an
atmosphere of total pressure p
1
above the surface of the liquid. Holding this pressure
constant and heating the liquid, we see that the system warms with only a smal l
volume increase to point b where the system begins to evolve to a vapor phase at
TABLE 2 Critical Point Values for Water
Variable Symbol Value
Temperature T
c
647 K
Pressure p
c
2:22 10
7
Pa (218.8 atm)
Specific volume vapor a
c;t
3:07 10
3
m
3
=kg
TABLE 1 Triple Point Values for Water
Variable Symbol Value
Temperature T
t
273.16 K
Pressure p
t
610.7 Pa, 6.107 mb
Ice density r
i;t
917 kg=m
3
Liquid density r
l;t
1000 kg=m
3
Vapor density r
v;t
0.005 kg=m
3
Specific volume ice a
i;t
1:091 10
3
m
3
=kg
Specific volume liquid a
l;t
1:000 10
3
m
3
=kg
Specific volume vapor a
v;t
2:060 10
2
m
3
=kg
Latent heat of condensation l
vl;t
2:5008 10
6
J=kg
Latent heat of sublimation l
vi;t
2:8345 10
6
J=kg
Latent heat of melting l
il;t
0:3337 10
6
J=kg
3 PHASE EQUILIBRIUM FOR WATER 211
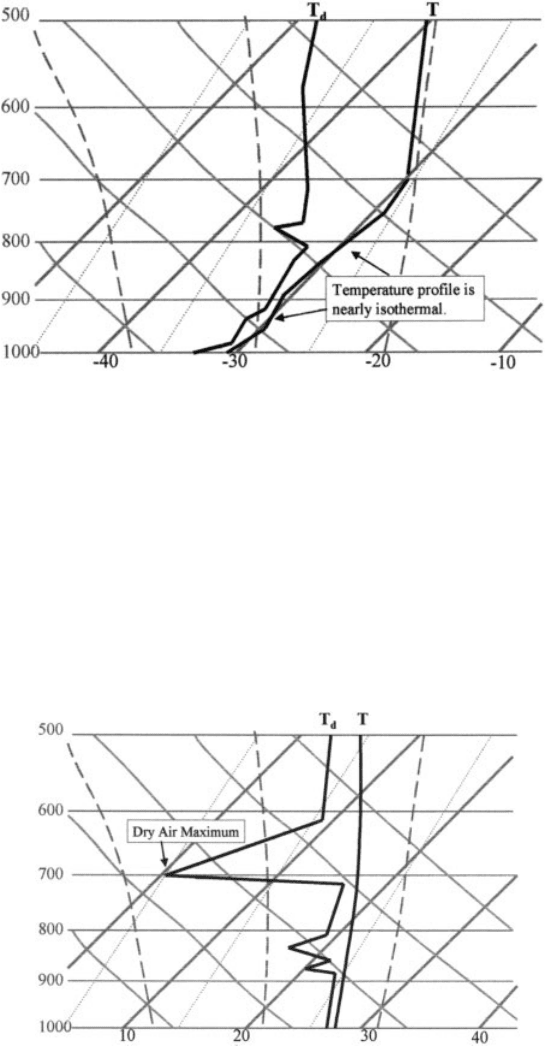
much higher volume as the temperature holds constant. The evolution from b to c is
commonly called boiling and occurs when the vapor pressure along the vapor–liquid
interface becomes equal to the atmospheric pressure. Hence we can also view the
saturation curves as boiling point curves for various atmospheric pressures.
Alternatively, if the system cools from point a, the volume very slowly decreases
until freezing commences at point d. There we see the volume more rapidly decrease
as freezing occurs. Of course, for water the volume change is reversed to expansion
and is not depicted for the substance displayed on this diagram.
Figure 3 Projection of the p-V-T surface on the p-T and p-V planes. (Sears, 1959).
Figure 2 Amagat–Andrews diagram (from Iribarne and Godson, 1973).
212
MOIST THERMODYNAMICS
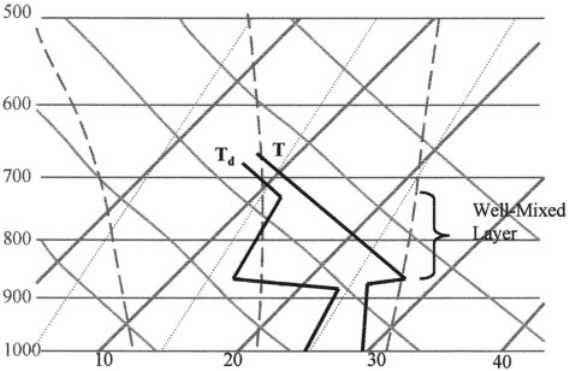
4 CLAUSIUS–CLAPEYRON EQUATION
We will now express mathematically the relation between the changes in pressure
and temperature along an equilibrium curve separating two phases. The assumption
of equilibrium between two systems a and b, requires that at the interface between
the systems:
g
a
¼ g
b
;
m
a
¼ m
b
;
T
a
¼ T
b
; and
u
a
¼ u
b
:
where we will assume that a and b are of different phases. For equilibrium, we also
require infinitesimal changes in conditions along the interface to preserve equili-
brium. Hence,
dg
a
¼ dg
b
;
dm
a
¼ dm
b
;
dT
a
¼ dT
b
; and
du
a
¼ du
b
:
ð9Þ
The Gibbs free energy was defined to be
g ¼ u þ pa Ts; ð10Þ
¼ h Ts: ð11Þ
Figure 4 Projection of the p-V-T surface on the p-T and p-V planes. (Sears, 1959).
4 CLAUSIUS–CLAPEYRON EQUATION 213

By virtue of Eq. (11),
dg
a
¼ðdu
a
Þs
a
dT þðTds
a
þ pda
a
Þþa
a
dp
¼ðdu
b
Þs
b
dT þðTds
b
þ pda
b
Þþa
b
dp ¼ dg
b
where the terms in parenthesis drop out [because of Eq. (9) and the first law] to give:
ðs
b
s
a
Þ dT ¼ða
b
a
a
Þ dp; and
s
b
s
a
a
b
a
a
¼
dp
dT
ð12Þ
From Eq. (11) it follows that along the interface,
g
b
g
a
¼ h
b
h
a
T ðs
b
s
a
Þ¼0; ð13Þ
and since, l
ab
¼ h
b
h
a
, we can write
l
ab
¼ Tðs
b
s
a
Þ: ð14Þ
Hence, we can rewrite Eq. (12) as:
dp
dT
¼
l
ab
Tða
b
a
a
Þ
ð15Þ
which is the general form of the Clapeyron equation.
The physical meaning of this equation can be illustrated by the process depicted
in Figure 5. Consider the four-step process shown. Beginning at T, P in the lower left
point of the cycle we can move to the point T þ dT and p þ dp in either of the two
paths shown. Since g is a state function, the path does not matter for the change in g
and hence the chang e in g along both paths can be equated, yielding the equation.
Hence the equation defines how the equilibrium vapor pressure must vary with
temperature based on the value of latent heat.
The Clapeyron equation can be applied between any two systems having differing
phases to produce expressions for the variations of equilibrium vapor pressure with
temperatures. Its integrated solution leads directly to expressions for the value of
Figure 5 Cycle related to Clapeyron equation (Hess, 1959).
214
MOIST THERMODYNAMICS

equilibrium vapor pressures with respect to a particular phase change for any
temperature.
Equilibrium Between Liquid and Solid Clapeyron Equation
Applying the phase equilibrium to a phase transition between liquid and ice yields
de
dT
¼
l
il
Tða
i
a
l
Þ
ð16Þ
where we are using the symbol e for pressure of the liquid and ice, and we will
always take the latent heat l
il
as the positive definite difference in enthalpy between a
liquid and frozen phase where the liquid phase has the greater enthalpy. For a
freezing process considered here, the enthalpy change passi ng from liquid to ice
will be negative. Hence, the negative sign appears on the right-hand side (RHS) of
Eq. (16). Since the volume of the ice is greater than that of the liquid, the change in
vapor pressure with temperature is negative. This is evidenced in Figure 1 as a
negative slope to the liquid–ice phase equilibrium curve. Note also that the slope
of the ice–liquid curve is very large resulting from the relatively small volume
change between liquid and ice phases [denominator of Eq. (16)].
Equilibrium Between Liquid and Vapor: Clausius–Clapeyron Equation
Applying the phase equilibrium to a phase transition between liquid and vapor under
equilibrium conditions yields
de
s
dT
¼
l
lv
Tða
l
a
v
Þ
ð17Þ
where e
s
is the vapor pressure of the equilibrium state, and the latent heat of
vaporization l
lv
is defined as the absolute value of the difference in enthalpies
between the liquid and ice phase. Since the enthalpy of the vapor state is higher
than that of the liquid, the negative sign appears in front of Eq. (17). Since a
v
a
l
,
we can drop the specific volume of liquid water compared to that of vapor. Hence,
applying the equation of state to the vapor specific volume:
d ln e
s
dT
¼
l
lv
R
v
T
2
: ð18Þ
This is the Clausius–Clapeyron equation and we will use it for a number of
applications. The equation tells us how the satur ation vapor pressure varies with
temperature. Hence given an observation point, such as the triple point, we can
determine e
s
at all other points on the phase equilibrium diagram.
4 CLAUSIUS–CLAPEYRON EQUATION 215
