Mrinal K Pal. Power system stability
Подождите немного. Документ загружается.


SYNCHRONOUS MACHINES
5-9
Several other transformations have been reported in the literature in an attempt to avoid this
problem, and also to render the transformation power invariant, in which the expression for
power is of the same form in both the original and transformed variables. The transformations
differ only in the constants used. It may be noted that in order for the transformation to be power
invariant the transformation matrix must be orthogonal (i.e.,
TT
′
=
−1
). The original
transformation used by Park has been retained here, since the same effects can be achieved by a
suitable choice of per unit system, noting that for balanced operation e
o
= i
o
= 0.
In the basic equations currents and voltages have been expressed as instantaneous values. In the
case of stator sinusoidal quantities, these have been expressed in terms of the peak values of the
sine wave. In the choice of the base quantities, once voltage, current and frequency bases are
picked, the bases for the remaining variables or circuit parameters such as flux-linkages,
inductance, etc. are automatically set, such as:
basebase
base
base
base
base
base
base
base
i
e
i
L
e
ω
ψ
ω
ψ
==
=
Also, in deriving the per unit quantities, the variables should be divided by their appropriate
bases. For instance, if the value of voltage is a peak value, in order to express it in per unit it
should be divided by the peak voltage base.
The flux linkage equations (5.21) and (5.23) can be expressed as
=
q
d
q
d
q
d
I
I
Λ
Λ
Ψ
Ψ
(5.27)
where
=
M
d
fd
d
d
1
ψ
ψ
ψ
Ψ ,
=
M
M
q
q
q
1
ψ
ψ
Ψ ,
=
M
d
fd
d
d
i
i
i
1
I ,
=
M
M
q
q
q
i
i
1
I ,
−
−
−
=
OMMM
L
L
L
dfdda
dfffdafd
daafdd
d
LLL
LLL
LLL
1111
1
1
2
3
2
3
Λ , and
−
−
=
OMM
OMM
LL
LL
qqa
qaq
q
LL
LL
111
1
2
3
Λ

SYNCHRONOUS MACHINES
5-10
The base quantities are defined as follows:
i
ao
= peak rated phase current (amps)
e
ao
= peak rated phase voltage (volts)
Z
ao
= e
ao
/i
ao
(ohms)
ω
o
, f
o
= rated frequency (rad/sec, cyc/sec)
L
ao
= Z
ao
/
ω
o
(henries)
X
ao
= r
ao
= Z
ao
=
ω
o
L
ao
ψ
ao
= L
ao
i
ao
= e
ao
/
ω
o
= stator base flux linkage (weber turns)
3
φ
VA base = VA
o
= (3/2)e
ao
i
ao
= (3/2)
ω
o
L
ao
i
ao
2
= (3/2)
ω
o
ψ
ao
i
ao
ψ
fdo
= base field flux linkage
ψ
xdo
= base flux linkage for additional d axis rotor windings
ψ
xqo
= base flux linkage for additional q axis rotor windings
I
fdo
, I
xdo
, I
xqo
are the base currents of the corresponding rotor windings.
It is not possible to assign physical values to the rotor base quantities at this stage.
In order to express equation (5.27) in per unit form, a diagonal matrix
Ψ
o
of base flux linkages
and another
I
o
of base currents are defined and these are operated on (5.27) to give
=
−
pu
pu
o
1
o
pu
pu
q
d
q
d
q
d
I
I
I
Λ
Λ
Ψ
Ψ
Ψ
which, after removing the subscript pu for convenience, can be written in short as
IΛΨ
=
(5.28)
For the per unit system of equations to have reciprocal mutual inductances, the matrix
Λ in the
above equation must be made symmetrical. Equating the corresponding off diagonal elements
and rearranging, the following relations are obtained.
ω
o
ψ
fdo
I
fdo
= (3/2)
ω
o
ψ
ao
i
ao
= VA
o
(5.29)
ω
o
ψ
xdo
I
xdo
= (3/2)
ω
o
ψ
ao
i
ao
= VA
o
(5.30)
ω
o
ψ
xqo
I
xqo
= (3/2)
ω
o
ψ
ao
i
ao
= VA
o
(5.31)
This shows that in order to have reciprocal mutual inductances the volt-ampere bases of all the
rotor circuits must be the same as the machine volt-ampere base.
Since with the rated frequency used as the base frequency, per unit inductance becomes the same
as per unit reactance, it is customary to use the reactance symbol
x to denote inductances in the
per unit machine equations.
Also, since
θ
=
ω
t ±
θ
o
,
ω
θ
=
dt
d
Thus the per unit equation (5.28) can be written in expanded form as
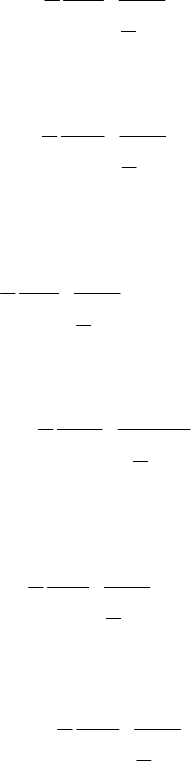
SYNCHRONOUS MACHINES
5-11
−
−
−
−
−
=
M
M
OMM
L
L
OMMM
L
L
L
M
M
q
q
d
fd
d
qaq
qaq
dfdad
dfffdfad
daafdd
q
q
d
fd
d
i
i
i
i
i
xx
xx
xxx
xxx
xxx
1
1
111
1
1111
1
1
1
1
ψ
ψ
ψ
ψ
ψ
(5.32)
where all the quantities are in per unit.
x
d
, x
afd
, etc. can be shown, using equations (5.29) – (5.31),
to be given by
==
o
o
o
2
3
2
3
a
fd
a
afd
fadafd
i
I
L
L
xx
(5.33)
==
o
o
o
1
11
2
3
2
3
a
xd
a
da
adda
i
I
L
L
xx
(5.34)
etc.
2
o
o
o
2
3
2
3
=
a
fd
a
ffd
ffd
i
I
L
L
x
(5.35)
==
2
o
oo
o
1
11
4
9
2
3
a
fdxd
a
df
fddf
i
II
L
L
xx
(5.36)
etc.
2
o
o
o
11
11
2
3
2
3
=
a
xd
a
d
d
i
I
L
L
x
(5.37)
2
o
o
o
12
2112
2
3
2
3
==
a
xd
a
d
dd
i
I
L
L
xx
(5.38)
etc.

SYNCHRONOUS MACHINES
5-12
==
o
o
o
1
11
2
3
2
3
a
xq
a
qa
aqqa
i
I
L
L
xx
(5.39)
2
o
o
o
11
11
2
3
2
3
=
a
xq
a
q
q
i
I
L
L
x
(5.40)
etc.
oa
d
d
L
L
x =
(5.41)
oa
q
q
L
L
x =
(5.42)
These are the conversion formulae for converting the impedances from normal units to per unit
values. The form in which the expressions have been written is to be particularly noted. Each
expression has been written in terms of base current ratios. The base current ratios have been
taken as the ratio of the base rotor circuit currents to 3/2 the base stator current. Also each of the
original impedance values has been multiplied by 3/2. This is necessary to give uniform
conversion formulas for all the self and mutual impedances while converting the original non-
reciprocal system of equations to a reciprocal per unit system of equations. The selection of base
current ratios is equivalent to the selection of stator rotor turns ratio. In other words, all the
quantities are first referred to the stator using the specially formed turns ration and multiplying
by 3/2 and then the stator base quantities are used to find the per unit values. As there is no
unique choice of selecting the turns ratio there is no unique value for the per unit impedance that
has been derived by applying the turns ratio. The base rotor currents are usually selected so as to
make all per unit values of self and mutual impedances of the same order of magnitude. One
choice of selecting base rotor currents is discussed later.
Dividing through the individual voltage equations in (5.5) and (5.26) by the respective base
quantities and manipulating a little, the per unit form of the equations can be expressed in matrix
form as
pupupu
o
pu
1
rrrr
dt
d
IRΨV +=
ω
(5.43)
puopupuo
o
puo
o
puo
1
dqdqdqdq
r
dt
d
iΨSΨe −+=
ω
ω
ω
(5.44)
where the per unit quantities are given by
=
o
o
o
pu
2
3
a
fd
a
fd
fd
i
I
e
e
e
(5.45)

SYNCHRONOUS MACHINES
5-13
=
o
o
o
pu
2
3
a
fd
a
fd
fd
i
I
ψ
ψ
ψ
(5.46)
2
o
o
o
pu
2
3
2
3
=
a
fd
a
fd
fd
i
I
r
r
r
(5.47)
2
o
o
o
1
pu1
2
3
2
3
=
a
xd
a
d
d
i
I
r
r
r
(5.48)
etc.
o
pu
a
r
r
r =
(5.49)
o
pu
a
d
d
e
e
e =
(5.50)
etc.
dqd
xxx
′′′′′
,, are pu impedances (defined later) as seen from the machine terminals and so they
must be independent of the base rotor currents and will be dependent only on the machine volt
ampere and voltage base.
dqd
xxx
′′′′′
,,
, as derived later, are given by, considering one additional rotor circuit on each axis,
2
111
2
111
2
11
2
dfffdd
daffdafddadfafdd
dd
xxx
xxxxxxx
xx
−
+−
−=
′′
(5.51)
q
qa
qq
x
x
xx
11
2
1
−=
′′
(5.52)
ffd
afd
dd
x
x
xx
2
−=
′
(5.53)
Substituting the values of
dd
xx
11
, etc. as found previously,
2
111
2
111
2
11
oo
2
1
2
3
dfffdd
daffdafddadfafdd
aa
d
d
LLL
LLLLLLL
LL
L
x
−
+−
−=
′′
(5.54)
q
qa
aa
q
q
L
L
LL
L
x
11
2
1
oo
1
2
3
−=
′′
(5.55)

SYNCHRONOUS MACHINES
5-14
ffd
afd
aa
d
d
L
L
LL
L
x
2
oo
1
2
3
−=
′
(5.56)
dqd
xxx
′′′′′
,, are thus invariant when expressed in per unit of the machine volt ampere and voltage
base.
Choice of rotor base currents
Several definitions of rotor base currents are available. The base field current which is most
commonly used is that current which will induce in each stator phase a voltage equal to
ω
o
L
ad
i
ao
or
X
ad
i
ao
. L
ad
is the mutual component of the stator self-inductance L
d
, i.e., the component of L
d
corresponding to the flux linkages which link the rotor. Defining a leakage component
L
1d
corresponding to the flux linkages which do not link the rotor,
L
d
= L
ad
+ L
1d
Similarly,
L
q
= L
aq
+ L
1q
The leakage components in the
d and q axes are generally assumed equal and labeled L
l
. Let base
currents of other rotor circuits be similarly defined. With these definitions
L
afd
I
fdo
= L
ad
i
ao
L
a1d
I
xdo
= L
ad
i
ao
L
a1q
I
xqo
= L
ad
i
ao
, etc.
Multiplying both sides of the first equation by
oo
o
2
3
2
3
aa
fd
Li
I
, we have
o
oo
o
2
3
2
3
fdafd
aa
fd
IL
Li
I
=
o
oo
o
2
3
2
3
aad
aa
fd
iL
Li
I
or
x
afd
I
fdo
= x
ad
I
fdo
or
x
afd
= x
ad
Similarly, from the second and third equation
x
a1d
= x
ad
, x
a1q
= x
aq
Thus this definition makes
x
afd
and x
a1d
equal to x
ad
. It cannot, however, be said that x
f1d
is also
equal to
x
ad
, although due to the proximity of the rotor circuits to the air gap this is very nearly
true, and in machine analysis it is often assumed that in per unit form all the direct axis mutual
inductances are equal and all the quadrature axis mutual inductances are equal. Therefore we can
write

SYNCHRONOUS MACHINES
5-15
M
L
LL
dadd
fdadffd
qaqaaq
dfdadaafdad
xxx
xxx
xxx
xxxxx
111
21
121
+=
+=
==
=
=
=
=
=
=
(5.57)
laqq
ladd
qaqq
xxx
xxx
xxx
+=
+=
+
=
M
111
To find the base field current, let
I
fo
be the field current which generates rated stator voltage on
open circuit at rated speed as found from the air gap line. Then
I
fo
×
ω
o
L
afd
=
ω
o
L
ao
i
ao
Also
I
fdo
× L
afd
= L
ad
i
ao
From these
I
fdo
/ I
fo
= L
ad
/L
ao
= x
ad
or
I
fdo
= x
ad
× I
fo
(5.58)
The per unit equations can be summarized as follows:
Per unit voltage equations
Stator
−
−
+
=
o
oo
o
00
1
i
i
i
r
dt
d
e
e
e
q
d
d
q
q
d
q
d
ψ
ψ
ω
ω
ψ
ψ
ω
(5.59)
Rotor
+
=
M
M
M
O
O
O
M
M
M
M
M
M
q
d
fd
q
d
fd
q
d
fdfd
i
i
i
r
r
r
dt
d
e
1
1
1
1
1
1
o
1
0
0
ψ
ψ
ψ
ω
(5.60)
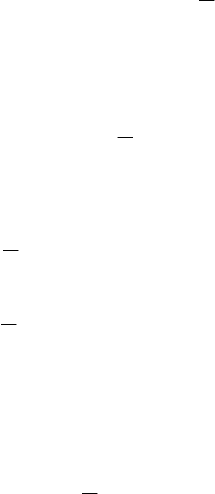
SYNCHRONOUS MACHINES
5-16
Per unit flux-linkage equations
Stator
+
−=
M
M
L
L
q
d
fd
qa
daafd
q
d
q
d
q
d
i
i
i
x
xx
i
i
i
x
x
x
1
1
1
1
ooo
ψ
ψ
ψ
(5.61)
Rotor
+
−=
M
M
OM
L
OMM
L
L
M
M
M
M
q
d
fd
q
ddf
dfffd
q
d
qa
da
afd
q
d
fd
i
i
i
x
xx
xx
i
i
x
x
x
1
1
11
111
1
1
1
1
1
ψ
ψ
ψ
(5.62)
In the above equations all quantities are in per unit except time which is in seconds, and
ω
and
ω
o
, which are in rad/sec.
Power and Torque
The instantaneous power measured at the machine terminals is given by
P = e′
abc
i
abc
(5.63)
In terms of
dqo components
ooo
11
o
2
1
1
2
3
][
dqdqdqdq
P ieiTTe
′
=
′′
=
−−
(5.64)
Under normal balanced condition
e
o
= i
o
= 0
)(
2
3
qqdd
ieieP +=
∴
(5.65)
Substituting for
e
d
and e
q
from equation (5.26)
[]
qqdqddqd
iriiriP )()(
2
3
−++−−=
ωψψωψψ
&&
[]
)()()(
2
3
22
qddqqdqqdd
iiriiii +−−++=
ψψωψψ
&&
(5.66)
The above equation can be interpreted as: Net power output = (rate of decrease of armature
magnetic energy) + (power transferred across air-gap) – (armature resistance loss). The air-gap
torque is obtained by dividing the second term (air-gap power) by rotor speed. Thus
)(
2
3
dqqde
iiT
ψψ
−= (5.67)

SYNCHRONOUS MACHINES
5-17
For balanced operation with zero armature resistance, e
d
= −
ωψ
q
and e
q
=
ωψ
d
, and the torque is
)(
1
2
3
qqdde
ieieT +=
ω
which checks with equation (5.65)
Expressed in per unit of three phase power base, equation (5.65) becomes
qqdd
ieieP
+
=
(5.68)
Similarly, the expression for per unit torque is
dqqde
iiT
ψ
ψ
−
= (5.69)
Steady State Operation
Consider a synchronous machine operating as a generator, supplying power to an infinite bus.
Let the machine phase voltages be
)120(cos
)120(cos
cos
o
o
+=
−=
=
tee
tee
tee
c
b
a
ω
ω
ω
(5.70)
Let the phase currents supplied by the generator be
)120(cos
)120(cos
)(cos
φω
φω
φ
ω
−+=
−−=
−
=
o
o
tii
tii
tii
c
b
a
(5.71)
We apply the
dqo transformation to the voltages using equation (5.20), noting that in matrix T,
θ
is the angle between the direct axis and the axis of phase
a. In the steady state the rotor electrical
speed
ω
is the same as the frequency of the voltage and current waves. If we assume that at t = 0
the
d axis lags phase a by
θ
o
, then
θ
=
ω
t −
θ
o
. Substituting the expressions for the phase
voltages in (5.20),
o
o
sin
cos
θ
θ
ee
ee
q
d
=
=
(5.72)
Similarly, for the current,
)sin(
)cos(
o
o
φθ
φ
θ
−=
−
=
ii
ii
q
d
(5.73)
Equations (5.72) and (5.73) show that for balanced steady state operation,
e
d
, e
q
, i
d
and i
q
are
constant dc quantities.
Under steady state conditions all
d and q axes flux-linkages are constants, i.e., their rates of
changes are zero. Therefore, since under steady state
ω
=
ω
o
, we obtain from equations (5.59) to
(5.62)

SYNCHRONOUS MACHINES
5-18
qdq
dqd
ire
ire
−=
−−=
ψ
ψ
(5.74)
0....
11
===
=
qd
fdfdfd
ii
ire
(5.75)
fdffddafdfd
qqq
fd
fd
ad
ddfdadddfdafdddd
ixix
ix
e
r
x
ixixixixix
+−=
−=
+−=+−=+−=
ψ
ψ
ψ
(5.76)
From (5.74) and (5.76) we obtain
qddq
dqqd
irixEe
irixe
−−=
−=
(5.77)
where
fd
fd
ad
fdad
e
r
x
ixE ==
E is introduced for convenience and may be considered as the field excitation measured in terms
of the terminal voltage that it would produce on open-circuit, normal-speed operation.
Observation of equations (5.77) shows that it is possible to consider the voltages and currents as
phasors in a plane having
d and q coordinate axes mutually perpendicular and oriented in exactly
the same way as the
d and q axes of the machine itself as shown in Figure 5.1. (Note that these
axes rotate in space at the electrical speed of the rotor, and in this sense they are conceptually
related to the phasor representing an ac quantity which rotates at synchronous speed.)
Complex voltage and current may be defined as
qd
qd
jiii
jeee
+=
+
=
ˆ
ˆ
(1) (5.78)
and from (5.72) and (5.73)
)sin()cos(
ˆ
sincos
ˆ
oo
oo
φθφθ
θ
θ
−+−=+=
+
=
+=
jiijiii
jeejeee
qd
qd
(5.79)
Note that these equations were derived from a definition of
e
a
= e cos
ω
t and i
a
= i cos(
ω
t-
φ
), and
θ
=
ω
t −
θ
o
, the angle between the direct axis and the axis of phase a. At t = 0, the d axis lags
phase
a by
θ
o
. Hence, expressing e
a
on the d-q axes plane is equivalent to lining up the e
a
phasor
with
e as given by equation (5.79), from which it follows that e
a
leads the d axis by
θ
o
. Note also
that the phase displacement between
e and i is the same as that between e
a
and i
a
.
(1) a “^” over a symbol is used to distinguish a phasor from a scalar quantity. This will, however, be omitted when
there is no chance of confusion.
