Massoud M. Engineering Thermofluids: Thermodynamics, Fluid Mechanics, and Heat Transfer
Подождите немного. Документ загружается.

272 IIIa. Fluid Mechanics: Single-Phase Flow Fundamentals
termining the flow rate of fluids. However, we assumed ideal conditions such as
frictionless venturi as well as steady, inviscid, and incompressible fluid flow. To
account for non-ideal conditions, the above flow rate is reduced by a discharge co-
efficient as discussed in Section IIIb.4.2.
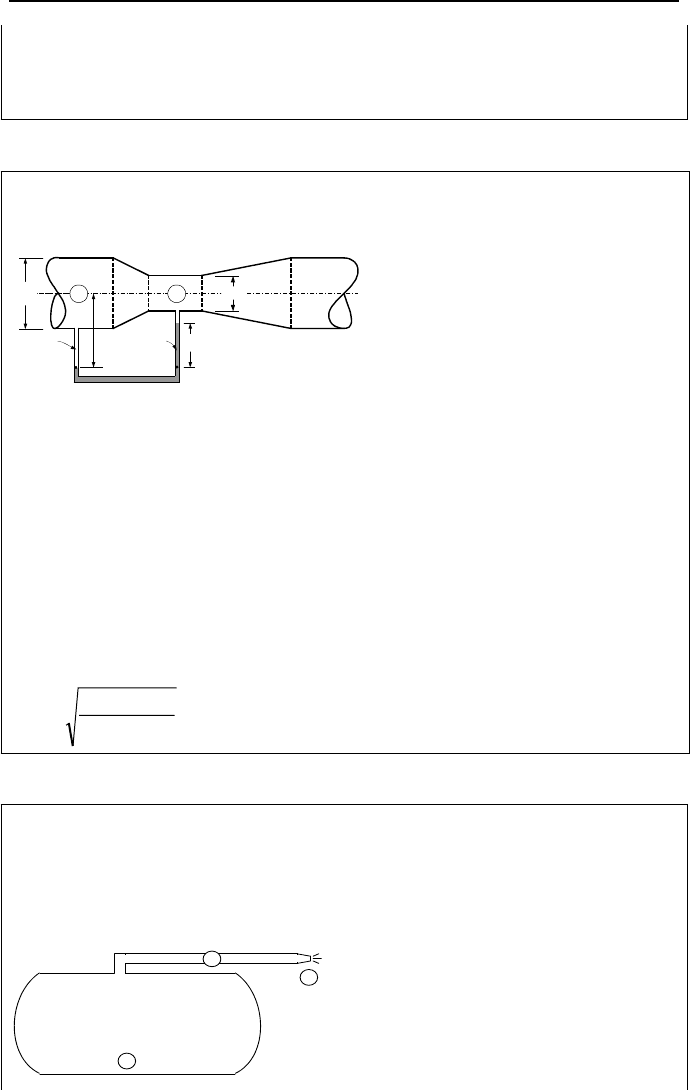
Example IIIa.3.19. For the venturi shown in the figure, derive a relation for flow
velocity, V
1
in terms of h, ȕ = D
1
/D
2
, and ȡ* = ȡ
m
/ȡ
f
where ȡ
m
is the density of the
manometer liquid.
21
ρ
m
ρ
f
h
h'
AB
D
1
D
2
Solution: Applying Equation IIIa.3.33 between points 1 and 2, noting equal ele-
vations (Z
1
= Z
2
) gives
(
)
(
)
2/2/
2
22
2
11
VPVP
ff
ρρ
+=+
From Equation IIa.5.3, velocity V
2
can be expressed in terms of V
1
as V
2
= V
1
(D
1
/D
2
)
4
. Substituting, we get:
()
()
42
112
/2 1
f
g
VPP
ρβ
−=−
We now express P
1
and P
2
in terms of h. To do this, we know that P
A
= P
B
. But
P
A
= P
1
+
ρ
f
h’g and P
B
= P
2
+
ρ
f
(h’ – h)g +
ρ
m
hg. Therefore, P
1
– P
2
= gh(
ρ
m
–
ρ
f
).
Substituting, we obtain:
()
1
1*h2
4
1
−
−
=
β
ρ
g
V
IIIa.3.49
Example IIIa.3.20. A large pressurized tank filled with air discharges into the
atmosphere. The flow path is a short and frictionless smooth pipe connected to a
discharge nozzle. Find a) the flow rate of air and b) pressure in the pipe for the
given data. Ignore all frictional losses, including head losses at the entrance to the
pipe, at the bend, and at the nozzle.
3
P
Tank
(kPa): 110.0
D
Pipe
(m): 0.040
d
Nozzle
(m): 0.015
Air
2
1
T
atm
(C): 20
P
atm
(kPa): 101
3. Conservation Equations 273
Solution: a) To find the flow of air we need to find
33
V AV=
where point 3 is
taken at the nozzle exit. Having A
3
=
π
d
2
/4 = 3.14(0.015)
2
/4 = 1.77E-4 m
2
, we
find V
3
by using the Bernoulli equation between points 1 and 3:
3
2
3
31
2
1
1
22
gZ
V
PgZ
V
P
ρ
ρ
ρ
ρ
++=++
We note that P
3
= P
atm
, Z
1
≈ Z
3
, and V
1
≈ 0. We ignored the elevation head here
primarily because the working fluid (air) has low density. We assume that air ve-
locity at point 1 is negligible because the tank is large. Hence;
31
2
3
2
PP
V
−=
ρ
We now need air density, 3.1)]27320(9.286/[3E110/
11
=+== RTP
ρ
kg/m
3
.
Substituting;
1183.1/)000,101000,110(2/)(2
313
=−=−=
ρ
PPV m/s
Therefore,
33
V AV=
=118×1.77E-4 = 0.021 m
3
/s. We now should calculate the
speed of sound in air to ensure that V
3
< 0.3c. Assuming air is an ideal gas:
1.4 286.9 (20 273) 1085 m/scRT
γ
==××+=
Therefore application of the Bernoulli equation is valid here.
b) To find pressure in the pipe, we write the Bernoulli equation between points 1
and 2:
2
2
2
12
V
PP
ρ
−=
But we do not have V
2
. We can calculate it from the continuity equation:
22
V AV=
=
33
AV
Since A
2
= 3.14(0.04)
2
/4 = 1.257E-3 m
2
therefore, V
2
= 0.021/1.257E-3 = 16.7
m/s. So that P
2
= 110,000 – [1.3× (16.7)
2
/2] = 109.82 kPa.
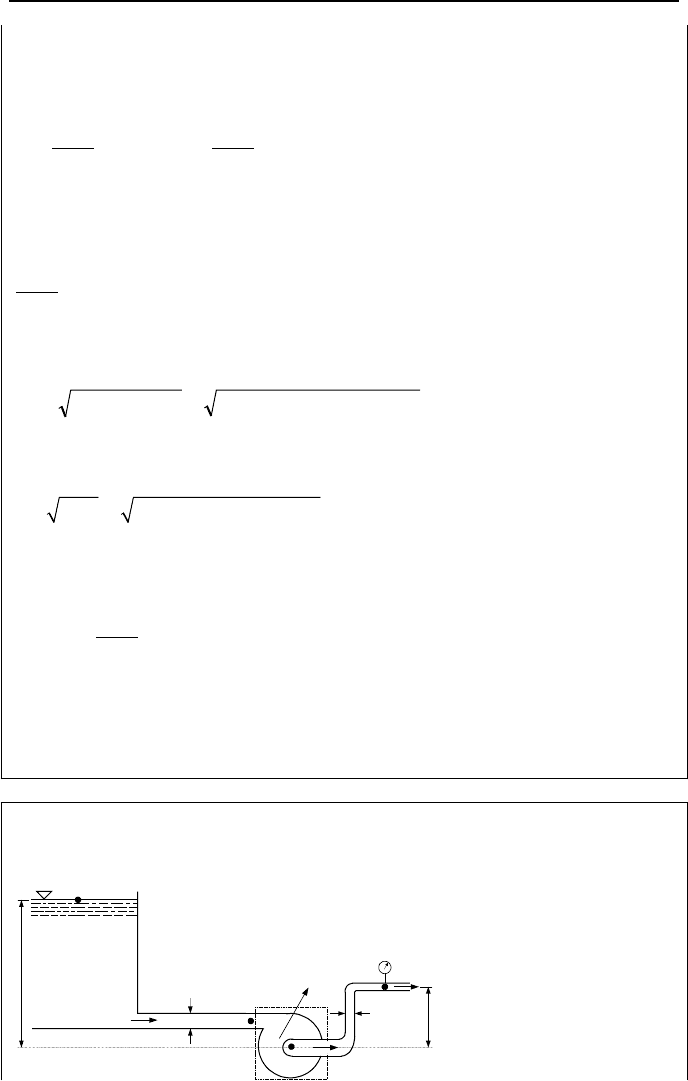
Example IIIa.3.21. Find the maximum power developed by the turbine. Data:
H
1
= 37 m, H
4
= 2. m, D
2
= 56 cm, D
4
= 35 cm, V
4
= 8.5 m/s, P
4
= 200 kPa, P
atm
≅ 100 kPa,
ρ
= 1000 kg/m
3
.
H
1
D
2
D
4
1
2
3
4
H
4
Turbine
V
4
P
4
Lake
h
s
274 IIIa. Fluid Mechanics: Single-Phase Flow Fundamentals
Solution: Since the Bernoulli equation does not apply here, we use Equation
IIIa.3.31. The maximum power is obtained when we ignore all frictional losses.
Equation IIIa.3.31 is applied between locations 1 and 4 noticing that V
1
§ 0 and
there is a turbine on the path:
)
2
(hh)
2
(
4
2
4
4s1
2
1
1
gZ
V
PgggZ
V
P
f
ρ
ρ
ρρρ
ρ
+++=−++
where we ignored velocity at point 1. Solving for h
s
, we find the head developed
by the turbine as:
=−−+−=
2
)()(h
2
4
4141s
V
PPZZgg
ρ
ρρ
1000 × 9.81(37 – 2) – (200 – 100) ×
1000 –
2
5.81000
2
×
s
h
g
ρ
= 343.35E3 – 100E3 – 36.125E3 = 207.23 kPa
The volumetric flow rate is
[
]
82.05.84/)100/35(V
2
≅×==
π
VA
m
3
/s. Turbine
power is then found as:
3
S
( h )V 207.23 kPa 0.82 m /s 170Wg
ρ
== ×≅
kW ≅ 228 hp
QUESTIONS
− What is continuum and why do we use such a concept in thermodynamics and
fluid mechanics?
− Is pressure a body force or a surface force?
− What is the difference between fluid static, fluid kinematics, and fluid dynam-
ics?
− Consider an ideal gas going through an isentropic process. Does the speed of
sound in this gas increase as the gas temperature increases?
− Is blood a Newtonian fluid?
− Why does the rate of shearing strain in viscous fluids increase with the increase
in shear stress?
− For what type of fluid is a yield stress defined?
− What approach best describes watching a school of fish while paddling in a ca-
noe?
− Is Equation IIIa.3.14 the Eulerian or the Lagrangian description of the conserva-
tion of momentum?
− What is the significance of the Reynolds number?
− What is the d’Alembert’s paradox?
− What is the contribution of Prandtl to the field of fluid mechanics?
− Is flow external or internal in a wind tunnel where objects of study are placed in
a controlled flow of air?

Questions and Problems 275
− What is an unrecoverable pressure drop? Is there also a recoverable pressure
drop?
− The Navier-Stokes equations are highly non-linear partial differential equations.
Can we get rid of the non-linear terms if we use an inviscid fluid?
− What is mechanical energy? Is it correct to say that there is no conversion of
mechanical energy to internal energy for the incompressible inviscid flow?
− What is vorticity and for what condition is the vorticity of a fluid flow zero?
PROBLEMS
Section 1
1. A fluid element in a flow field at time t is identified with the three components
of its location vectorgiven as: r
x
= 1 cm, r
y
= -4 cm, and r
z
= 2 cm. Find a) the
magnitude and b) sketch the direction this fluid element is heading. [Ans.: a) 4.58
cm].
2. Use Table A.IV.4(SI) to find the dynamic viscosity of air at the temperatures of
100, 300, 400, 600, 800, and 1000 K. Air is the atmospheric pressure. Plot the
viscosities versus temperature and describe the trend.
3. Use Table A.IV.5(SI) to find the dynamic and kinematic viscosities of satuaretd
water at 300, 400, 500, and 600 K. Plot the viscosities versus time and describe
the trend. Compare the results for water with the results for air in Problem 2 and
explain the difference.
4. The velocity vectors of three flow fileds are given as
1
(1 )Vaxibx tjtk=+++
K
K
KK
,
2
(1 )Vaxyibx tj=++
G
GG
, and ktbzyiaxyV
K
K
K
)1(
3
+−= where coefficients a and b have
constant values. Is it correct to say that flow field 1 is one-, flow filed 2 is two-, and
flow filed 3 is three-dimensional? Are these flow fields steady or unsteady?
5. Two large flat plates are sparated by a narrow gap filled with water. The lowr
plate is stationary and the top plate moves at velocity V
f
. The velocity profile in
water is linear
iyVV
f
K
K
)h/(= where y is the vertical distance. Find the shear
stress at the wall. T
Water
= 20 C, h = 5 mm, and V
f
= 0.5 cm/s.
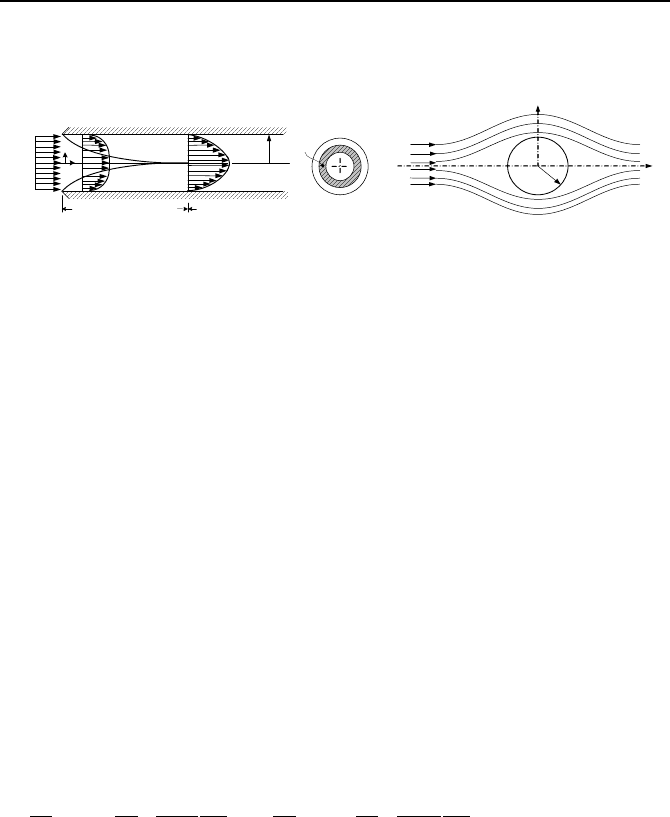
6. Water is flowing between two stationary parallel plates. For laminar flow, the
velicty is a parabolic function of the vertical distance y as shown in the figure.
Find a) the force applied on the lower plate and b) the average flow velocity in the
fully developed region of the flow field. Data: V
max
= 0.1 m/s, h = 0.8 cm, plate
length is 1 m and the plate width is 10 cm.
y
2
max
h
2
1
¸
¹
·
¨
©
§
−=
y
V
V
h
x
V
max
276 IIIa. Fluid Mechanics: Single-Phase Flow Fundamentals
7. The velocity profile for the laminar flow of water in a pipe is V
x
(r) = (V
x
)
max
[1 –
(r/R)
2
]. Find velovity at r = R/8, r = R/4, and r = R/2. [Ans.: V
x
(r = R/8) =
0.015625(V
x
)
max
].
V
x
(r)
r
x
Fully DevelopedEntrance Length
R
(V
x
)
f
dA
a
V
f
x
y
Problems 7 and 8 Problems 9 and 22
8. In the above problem find the average flow velocity and the volumetric flow
rate if the maximum velocity at the pipe centerline is 2 m/s and pipe diameter is 8
cm. [Ans.: V
av
= (V
x
)
max
/2 = 1 m/s, 5E-3 m
3
/s].
9. The components of the velocity vector for the flow of an ideal fluid over a
circular cylinder are:
V
r
= V
f
(1 – a
2
/r
2
)cos
θ
and V
θ
= –V
f
(1 + a
2
/r
2
)sin
θ
where a and V
f
are the radius of
the cylinder and the velocity of the approaching flow, respectively. Find a) the
magnitude of velocity at
θ
=
π
/4 for fluid particles at r
1
= 1.1a, r
2
= 1.2a, r
3
= 1.3a
and b) the maximum velocity and its location. [Ans.: V
2
= 1.22V
f
].
10. Fluid is flowing between two large plates. Flow enters the gap between the
two plates at x = 0 and leaves the gap at x = L, where L = 5 m. The two paltes are
not parallel so that the flow area at x = 0 is twice the flow area at x = L. The flow
enters the gap at x = 0 at a veloxity of V
1
= 1 m/s. Find a) the flow velocity at x =
L and b) the velocity vector.
11. In Cartesian coordinates, the Eulerian description of an unsteady, two-
dimensional velocity field is given as
jeietyxV
ytxt
K
K
K
+=),,( . Find the velocity of
a particle located at x = 2 and y = 3 at time t = 1.
12. In the polar coordinate system, as defined in Chapter VIIc, r and
θ
are related
to x and y as r
2
= x
2
+ y
2
and tan
θ
= y/x. a) Use the chain rule for differentiation to
show that
θ
θ
θ
∂
∂
+
∂
∂
=
∂
∂
r
r
x
sin
cos
and
θ
θ
θ
∂
∂
+
∂
∂
=
∂
∂
rry
cos
sin
b) Take the derivative of f(r,
θ
) = rsin(2
θ
) in the Cartesian coordinate system.
13. Use the chain rule for taking the derivative of composite functions and find
dG/ds where G = F[f
i
(s), s]. Next, find the acceleration of a fluid particle for a
case in which G = V, s = t, and f
1
(s) = x(t), f
2
(s) = y(t), and f
3
(s) = z(t). [Ans.:
dG/ds =
Σ
i
(∂G/∂f
i
)df
i
/ds + ∂G/∂s].
Sections 2
14. Find the fluid acceleration if the veoclity profile is given as a)
iyVV
f
K
K
)h/(=
and b) iytVV
f
K
K
)h/(= .
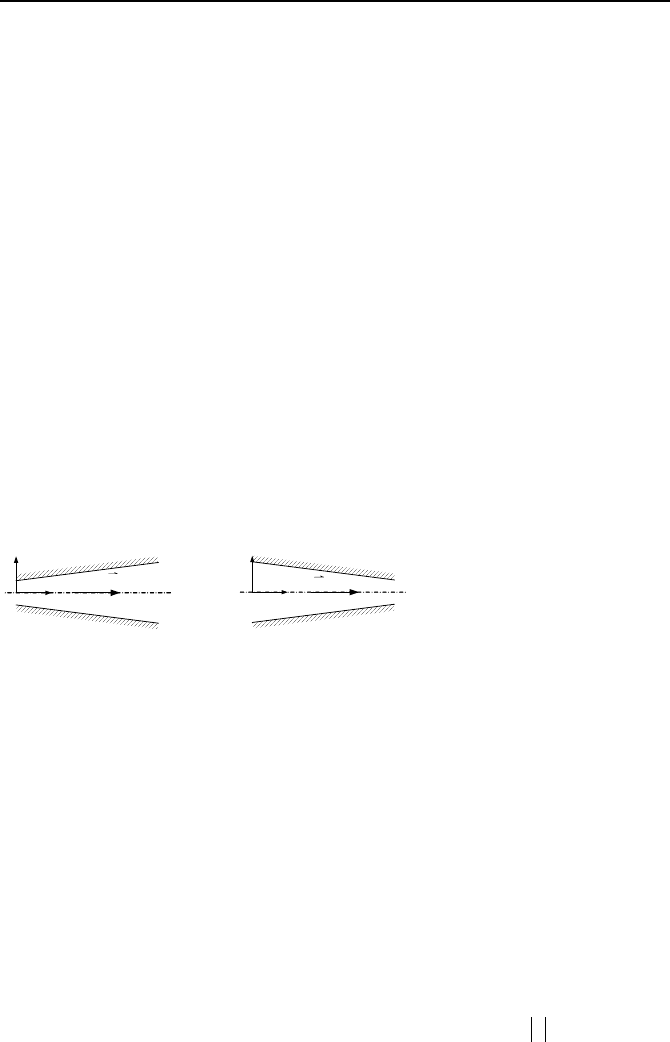
Questions and Problems 277
15. The three components of a velocity vector of a fluid particle in the Cartesian
coordinate system are V
x
= x
2
yt
2
, V
y
= –xy
2
(1 + t)t, and V
z
= 2xyt. Find the
acceleration of a fluid particle located at x = y = z =1 at t = 1s.
16. Consider a high-rise building. Balloons are being released steadily from each
floor of the building such that the balloon population at any elevation z is given by
N = N
o
[1 + (z/H)] where N
o
is the number of balloons released at the ground and H
is the elevation of the last floor. Find the observed rate of change of the balloon
population as seen by a) an observer on the first floor looking up and b) an ob-
server traveling upward in a hot-air balloon at velocity V
o
.
[Ans.: 0 and V
o
N
o
(2z/H
2
)].
17. Specify the framework that best describes the following situations; a) a
helicopter pilot following a Police chase, b) a hunter targeting a bird in a flock of
birds, c) a lion chasing a wilderbeast in a herd, d) a geologist watching lava
flowing from a volcano, e) studying blood flow in the arteries by using suitable
dyes.
18. Air flows in a duct with a rectngular flow area. At the enterance, the rectangle
is 1 m by 0.5 m and at the exit, the recangle is 1.5 m by 0.8 m. The duct is 10 m
long and the volumetric flow rate of air in the duct is 1 m
3
/s. Find the acceleration
of air halfway through the duct. Is flow accelerating or decelerating? [Ans.:
0.114 m/s
2
].
x
y
x
2
= Lx
1
= 0
x
y
V
x
1
= 0
x
2
= L
V
Problem 18 Problem 19
19. The velocity vector for a one-dimensional, steady, incompressible flow in the
channel shown below is given as
iLxVV
K
K
)]/(1[
1
+= . Find a) the x-component of
the acceleration vector and b) the position vector of a fluid particle located at x = 0
and time t =0. [Ans.: a) a
x
= LVLx /)/1(
2
1
+ and b) )1(
/
1
−=
LtV
eLx ].
20. A velocity vector is given as
jytxixytV
K
K
K
22
−= . Find a) the local
acceleration vector. b) the convective acceleration vector. [Ans. for part a:
jytxixya
local
K
K
K
2
2−= ].
21. A position vector is given as
kRjRiRR
zyx
K
K
K
K
++= where R
x
= 0.334xy
2
t
3
+
1.5zt
2
, R
y
= 0.5xyt
2
– x
2
t, and R
z
= –(0.334y
2
zt
3
– 0.25z
2
t
2
. Find the velocity and the
acceleration vectors. Calculate the acceleration of a point located at x = 1 m, y = –
1 m, and z = 1 m at time t = 2 s. [Ans.:
kjia
K
KK
K
5.47 −−= and 38.8=a
K
m/s].

278 IIIa. Fluid Mechanics: Single-Phase Flow Fundamentals
22. Use the components of steay flow velocity around a cylinder as given in
Problem 9 and find the acceleration of a fluid element located at r = 2a and
θ
=
π
/2. [Ans.: -(V
f
)
2
/(4a)].
Section 3 (Continuity Equation)
23. Do the following vectors represent the velocity vector of an incompressible
flow?
kytxtzjxyztixyztV
K
K
K
K
)(5.0)()(
222
1
−+−= and
kytxtzjxyztixyztV
K
K
K
K
)(5.0)()(
222
2
−+−=
24. In a two-dimensional incompressible flow, the component V
x
of the velocity
vector is given as V
x
= –x
2
y. Find V
y
. [Ans.: V
y
= xy
2
+ C].
25. In a three dimensional incompressible flow, V
x
and V
y
are given as V
x
= 3x
2
+
xy
2
, V
y
= xzy. Find V
z
. [Ans.: V
z
= –(6x + y
2
)z – 0.5xz
2
].
26. Two components of a two-dimensional flow in polar coordinates are given as
V
r
= V
o
cos
θ
[1 – (a/r)
2
] and V
θ
= –V
o
sin
θ
[1 + (a/r)
2
]. Is this a steady incom-
pressible flow?
27. The velocity vector in a flow field is represented by
ϕθ
uuuV
r
K
K
K
K
432 ++=
where
ϕθ
uuu
r
K
K
K
and,, are the unit vectors of the spherical coordinate system.
Does this represent an incompressible flow field?
28. The velocity vector of a steady, two-dimensional, incompressible flow in polar
corrdinates is shown as
θθ
iViVV
rr
K
K
K
+= . If the r-component is given as V
r
=
(
θ
/2
π
r) + V
o
cos
θ
, find the
θ
-component. [Ans.: V
o
sin
θ
].
29. Density of a two-dimensional steady compressible flow field in the Cartesian
coordinate system is given as
ρ
= xy. If the component V
x
of the velocity vector is
V
x
= x
2
y
2
, find the differential equation from which V
y
can be determined. [Ans.:
dV
y
/dy +V
y
/y +3xy
2
= 0].
30. A velocity vector is given as
kytxtzjxyztixyztV
K
K
K
K
)(5.0)()(
222
−+−= . Find
the vector representing the applied differential force on a unit mass of the fluid
(i.e.,
dmFd /
K
).
[Hint,
)///()/(/ zVVyVVxVVtVdmFd
zyx
∂∂+∂∂+∂∂+∂∂=
K
K
K
K
K
].
31. Show that the velocity of an incompressible flow has zero divergence.
32. Velocity profile for turbulent flow in smooth circular pipes may be
empirically expressed as V(r) = V
max
(1 –
η
)
m
. The value of the exponent m
depends on the flow condition and varies from 1/6 to 1/10, with 1/7 being used for
wide ranges of turbulent flow. Find the ratio of the average to the maximum
velosity in the flow field
)(/
max
mfVV = . [Ans.: )2)(1/[(2/
max
++= mmVV ].
Questions and Problems 279
33. Derive the relation between
ρ
, V, and A for one-dimensional flow under steady
state condition.
[Ans.:
0=++
A
dA
V
dVd
ρ
ρ
].
34. Use the Gauss divergence theorem to obtain Equation IIIa.3.13 from IIIa.3.2.
35. Two infinitesimal elements of the same fluid in the Cartesian coordinates
taken in a flow field are shown in the figure. One is used as a control volume
through which fluid flows to derive the conservation equation of mass. The other
is used as a differential fluid element in a free body diagram to relate the net ap-
plied forces to the acceleration of the fluid element. Specify which of these deri-
vations uses the Eulerian and which uses the Lagrangian approach. b) Specify the
conservation equation of mass in the Lagrangian approach.
∆y
∆z
∆x
∆y
∆z
∆x
C.V. for
conservation of
mass
C.V. for
conservation of
Momentum
36. The general form of the continuity equation that is independent of the coordi-
nate system and applies to steady or unsteady, compressible or incompressible,
viscous or inviscid flow is given by Equation IIIa.3.13. Which term of this equa-
tion represents the mass flux? Show the units of the mass flux term. Apply this
equation to a compressor, which is steadily delivering compressed air to a reser-
voir.
37. Apply Equation IIIa.3.13 to a flow field identified with the velocity vector
given as
iaxV
K
K
= and density of
ρ
= b + ce
–sx
cos
ω
t where coefficients a, b, c, and
s are constants.
38. Equation IIIa.3.13 for steady flow simplifies to
0=⋅∇ V
KK
ρ
. What other con-
dition should exist to be able to further simplify this equation and obtain
0=⋅∇ V
KK
?
39. Regarding the differential formulation of the continuity equation, match num-
bers with letters:
1:
()
0=⋅∇+
∂
∂
V
t
K
K
ρ
ρ
, 2:
(
)
0=⋅∇ V
K
K
, 3:
()
0=⋅∇+ V
Dt
D
K
K
ρ
ρ
, 4:
(
)
0=⋅∇ V
K
K
ρ
a: Lagrangian form, b: Steady flow, Eulerian form, c: Incompressible flow, d: Eul-
erian form
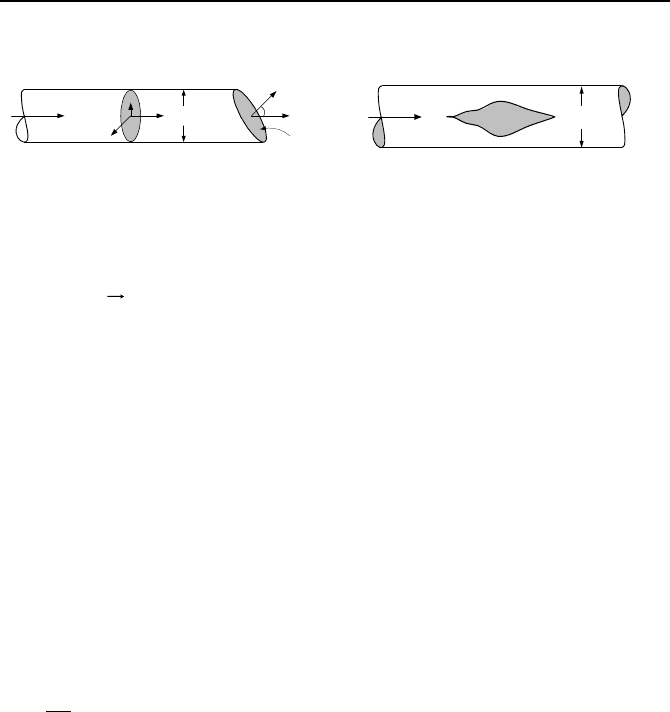
40. Water flows steadily in a pipe of inside diameter D. Flow area at exit, S is at
an inclined angle
α
with the horizontal plane. Find
α
in terms of the pipe diameter
and exit flow area. [Ans:
α
= cos
–1
(
π
D
2
/4S)].
280 IIIa. Fluid Mechanics: Single-Phase Flow Fundamentals
41. Find water flow rate at steady state condition in the above pipe for V = 3 m/s,
S = 100 cm
2
, and
α
= 30
o
.
x
n
α
S
D
V
V D
Problems 40, 41, and 42 Problem 43
42. Water is flowing steadily in a 4 in diameter pipe at a velocity of 5 ft/s. Flow
area at the exit (surface S) is at an inclination angle of 30 degrees with the horizon-
tal plane. a) Find the velocity vector as well as the vector representing surface S.
b) Use the two vectors you obtained in part a to find the volumetric flow rate as:
³
S
SVdsV
K
K
K
⋅=⋅=V
43. Water at 7 ft/s and 65 F flows in a 10 in schedule 40 pipe (inside diameter
from Table A.III.1(SI) is 254.5 mm or 10.02 in from Table A.III.1(BU)). The pipe
now bursts with an effective rupture area of twice the flow area of the pipe (A
Rupture
=
π
D
2
/2). Find the mass flow rate of water through the ruptured area. Assume
that the flow velocity remains at 7 ft/s after the pipe ruptures. [Ans.: 239 lbm/s].
44. Consider a cylinder, equipped with a piston. The cylinder contains a gas, hav-
ing a density of 20 kg/m
3
when the piston is L
o
= 20 cm from the closed end of the
cylinder. We now pull the piston away from the closed end at a velocity of V
piston
= 15 m/s. Find the gas density at t = 0.5 s after the piston is pulled. Assume that
V
gas
= V
piston
(x/L). Note that at time zero, L = L
o
. [Ans.: 14.5 kg/m
3
].
Section 3 (Momentum Equation)
45. For the flow of fluids, Newton’s second law of motion can be expressed as:
»
»
»
¼
º
«
«
«
¬
ª
+
»
»
»
¼
º
«
«
«
¬
ª
∂∂
∂∂
∂∂
»
»
»
¼
º
«
«
«
¬
ª
=
»
»
»
¼
º
«
«
«
¬
ª
z
y
x
zzyzx
yzyyx
xzxyx
z
y
x
g
g
g
z
y
x
V
V
V
Dt
D
ρ
σττ
τστ
ττσ
ρ
/
/
/
a) Is this equation applicable to non-Newtonian fluids?
b) Draw the free body diagram and show all the forces applied on the infinitesimal
fluid element.
c) By taking moments about axes passing through the center of the infinitesimal
element, show that the shear stresses become
τ
xy
=
τ
yx
,
τ
yz
=
τ
zy
, and
τ
xz
=
τ
zx
(this is
an implication of the Stokes hypothesis).
d) The constitutive equations for the normal stresses are
σ
x
= –P +
τ
xx
,
σ
y
= –P +
yy
τ
,
σ
z
= –P +
z
z
τ
where
VxV
xxx
K
K
⋅∇+∂∂=
λµτ
)/(2 , VyV
yyy
K
K
⋅∇+∂∂=
λµτ
)/(2 ,
and
VzV
zzz
K
K
⋅∇+∂∂=
λµτ
)/(2 where
λ
= –2
µ
/3. Use the Stokes hypothesis to
show that:
P = –(
σ
x
+
σ
y
+
σ
z
)/3
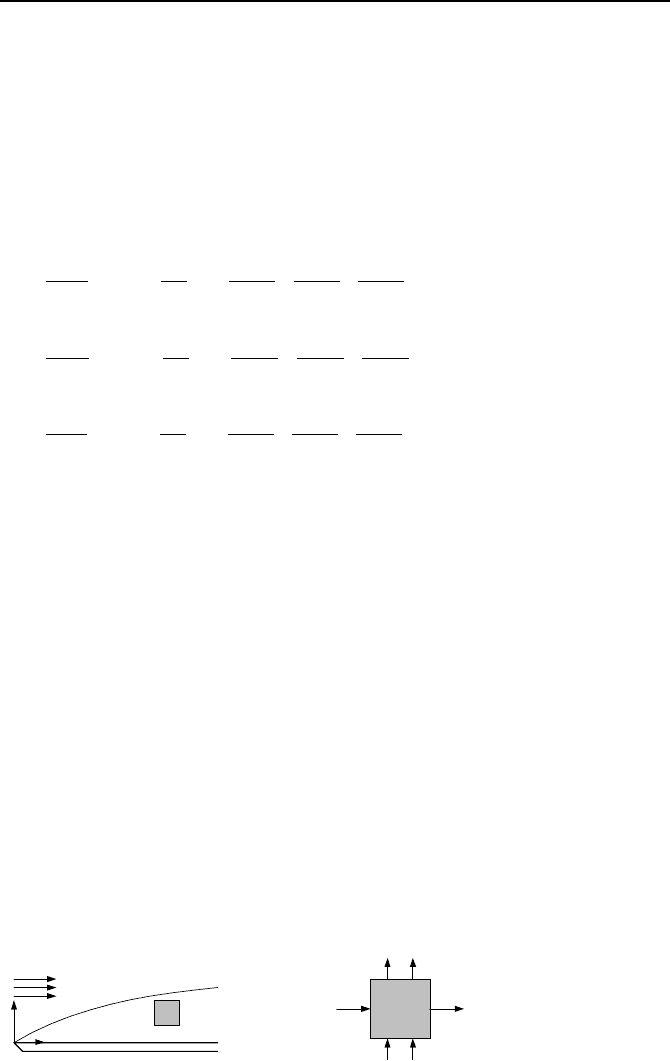
Questions and Problems 281
Thus, pressure at a point in a fluid is a compressive stress, having an absolute
value equal to the average value of the three normal stresses applied at that point.
46. Consider the case of an ideal fluid flow in the conduit of Problem 19. Find
pressure at the exit of the conduit in terms of the flow density and velocities at the
inlet and the outlet. [Ans.:
)(
2
1
2
212
xx
VVPP −−= ].
47. Show that the Navier-Stokes equations for a three dimensional, incompressi-
ble, and Newtonian fluid are:
¸
¸
¹
·
¨
¨
©
§
∂
∂
+
∂
∂
+
∂
∂
+
∂
∂
−=
2
2
2
2
2
2
z
V
y
V
x
V
x
P
g
Dt
DV
xxx
x
x
µρρ
¸
¸
¹
·
¨
¨
©
§
∂
∂
+
∂
∂
+
∂
∂
+
∂
∂
−=
2
2
2
2
2
2
z
V
y
V
x
V
y
P
g
Dt
DV
yyy
y
y
µρρ
¸
¸
¹
·
¨
¨
©
§
∂
∂
+
∂
∂
+
∂
∂
+
∂
∂
−=
2
2
2
2
2
2
z
V
y
V
x
V
x
P
g
Dt
DV
zzz
z
z
µρρ
48. Two components of a two-dimensional flow are given as V
x
= x
2
– y
2
and V
y
=
–2xy. Use the result of Problem 47 to find the condition for this velocity vector to
be the solution to the Navier-Stokes equations.
49. The velocity components of a two dimensional steady ideal fluid flow (fric-
tionless and incompressible) are given as V
x
= axy and V
y
= –by
2
where coefficients
a and b are constants. Find the pressure gradient in terms of a, b, x, and y. Find
the value of the pressure gradient for a = b = 1 at point x = y = 1.
50. Assuming air behaves as an ideal gas use the equation of state and Equation
IIIa.3.22 to find the air pressure in the upper atmosphere as a function of elevation.
Use the result to find air pressure at an elevation of 1 km (3280.8 ft) from the sea
level. In a winter night temperature at this elevation is measured as –16 C. [Ans.:
)/( TRMzg
s
u
ePP
−
= , 88.7 kPa].
Section 3 (Energy Equation)
51. Solve Example IIIa.3.13 by direct derivation. For this purpose, consider the
elemental control volume inside the boundary layer as shown in the figure. Write
a steady state energy balance for this control volume by considering the net con-
vection in the flow direction, net conduction perpendicular to the flow direction,
and viscous work.
dx
dy
x
y
V
f
Convection
Conduction Viscous work
