Leray J. Lagrangian Analysis and Quantum Mechanics: A Mathematical Structure Related to Asymptotic Expansions and the Maslov Index

Подождите немного. Документ загружается.

184

I I I,§ 1,4-III,§2,0

au = 0,

whose unknown u:E3\{0} -+ C and its gradient have to be square in-

tegrable (III,§4).

§2. The Lagrangian Equation aU = 0 mod(1/vs)

(a Associated to H, U Having Lagrangian Amplitude > 0

Defined on a Compact V)

0. Introduction

Summary.

In §1, we studied problem (1) (introduction to chapter III),

that is, a system of three lagrangian equations. In §2, we study the first of

these three equations and, more precisely, problem (2) (introduction to

chapter III).

Each solution of problem (1) is a solution of problem (2), by theorem 3

of §1. In the exceptional case of a hamiltonian H independent of M (for

example, Schrodinger and Klein-Gordon without a magnetic field), a

rotation in E3 acting simultaneously on x and p obviously transforms

solutions of problem (1) into solutions of problem (2) that are no longer

solutions of problem (1). Without considering this exceptional case,

theorem 1 of §2 constructs solutions of problem (2) that are not solutions

of problem (1): a solution of problem (2) defined on a torus T(l, m, n) (theorem

3 of §1) is not necessarily unique up to a constant multiplicative factor.

On the other hand, theorem 2 shows that even if H depends on some

parameters, these tori T(l, m, n) are, in general, the only compact lagrangian

manifolds V on which solutions of problem (2) are defined. Moreover,

theorem 3.1 specifies that in the Schrodinger and Klein-Gordon cases

problems (1) and (2) define the same energy levels: the classical levels.

Remark. Theorem 1 of §2 does not have any physical significance: it takes

into account the condition that some numbers, which in the Schrodinger

and Klein-Gordon cases are measurements of physical quantities, take

rational values.

CONCLUSION.

Let us call a problem that when posed for the Schrodinger-

Klein-Gordon equation has the essential features of the classical problem

(4) (introduction to chapter III) a well-posed problem. Problem (1) is well-

posed by theorem 4.2 of §1. From the preceding remark, the main result of

§2 is that problem (2) is not well-posed.

III,§2,1 185

1. Solutions of the Equation aU = 0 mod(1/v2) with Lagrangian

Amplitude -> 0 Defined on the Tori V [L0 , Mo)

In section 2 of §1 we chose H and defined the manifolds

W:H=0,

V[L0,Mo]:H=L-Lo=M-Mo=0.

Any compact lagrangian manifold V in W is a union of characteristics K

of H (whose paramter t varies from - cc to + oo); V is thus a union of

compact closures K of such characteristics.

Properties of a characteristic K of H with compact closure K. By (2.13)

of §1, such a characteristic K stays on a torus V[L0, Mo] and is given by

the equations

K:`Y-To+).[L0,M0,t]=(D -(o+p[L0,M0,t]=0

(0o, `1'o constants)

on this torus. Recall that the coordinates of a point of V[L0, M0],

(`1', 4), t),

are defined

(mod 2n, mod 2n, mod co), where co = c[L0, Mo].

More explicitly, let R3 be given the coordinates (`Y, D, t) and let Z3 be

the additive group of triples of integers (S, q, () acting on R3 as follows:

Z3 -3 ((1, q, C): (P, 4), t) i -+ (P + 2nd, 1) + 2n7, t + c0S);

the quotient of R3 by this group Z3 is V[L0, Mo]: there exists a natural

mapping

R3 -' R3/Z3 = V[Lo, M0].

(1.2)

Given a function

F: (L,

M, t

M, t].

Obviously

A,R=A,Q=0.

186 III,§2,1

By the definitions (2.8) of 0 and (2.9) of N in §1,

O0[L, M, t] = 2nN[L, M];

then, by (1.3) and §1,definition (2.10) of

and

2ndN[L, M] = AA [L, M, t] dL + A,u[L, M, t] dM;

that is,

A,,l = 2nNL,

0,u = 2nNM.

Let

NL,, = NL[LO, MO],

NMo = NM[L,, MO]

We can now describe K.

LEMMA 1.1.

1°) Assume NL° and NM° are rational:

NL° _

-L1

NMo =

Ml,

N,' N,

where (L,, M, N1) E Z3,

G.C.D.(L,, M1, N,) = 1 (1.6)

(that is, L,, M,, and N, are integers with greatest common divisor 1). Then

K = K is a closed curve given by the equations (1.1).

More precisely, the equations (1.1) define an open curve R (that is, a

curve homeomorphic to R) in R3. By (1.5) and (1.6), the subgroup 2 of Z3

generated by (L1, M,, N,) leaves ft invariant; we have

K=K=R/Z.

(1.7)

2°) Assume that NL° and N,M° are connected by a unique affine relation

L, NL,, + M, NMO = N,

(1.8)

where (L,, M,, N,) E Z3, G.C.D.(L,, M,, N,) = 1.

Then K is the 2-dimensional torus T 2 defined in V [L., M0] by the equation

L,{`P - To + 2[L0, M0, t]} + M,{0 - b0 + u[L0, M0, t]} = 0.

(1.9)

More precisely, equation (1.9) defines a surface R2 in R3 homeomorphic

to R2. By (1.5) and (1.8), the subgroup

22 of Z3 given

by the equation

Z:LjI +M,q+N1 = 0

(1.10)

III,§2,1 187

acts on 1l2. We have

T2 = It2/22.

(1.11)

In order to describe the generators of Z2, let

L2 = G.C.D.(M,, N1),

M2 = G.C.D.(L1, N1),

N2 = G.C.D.(L,, M1).

(1.12)

M2 and N2 divide L1i G.C.D.(M2, N2) = 1 by (1.8)3; then there exists an

integer L3 and similarly integers M3 and N3 such that

L1 = L3 M2 N2,

M1 = L2 M3 N2 ,

Ni = L2 M2 N3.

(1.13)

Z2 is generated by its three elements

(0, M2N3, -M3N2),

(-L2N3, 0, L3N2),

(L2M3, -L3M2, 0),

which evidently are connected by the relation

L3(0, MZN3, -M3N2) +

M3(-L2N3, 0, L3N2)

+ N3(L2M3, -L3M2, 0) = 0.

(1.14)

(1.15)

3°) Suppose there is no affine relation with integer coefficients connecting

NL° and NMO. Then K is the torus V[L0, Mo].

Proof.

The subset of R3 whose natural image in V[L0, Mo] is K is

defined by the condition

C ` I ' - To +.[LO, MO, t]b - 0o + u[Lo, MO, t]

E G,

2rc

where G is the image of Z 3 in the additive group R 2 under the morphism

Z33(

b)~'(S + NLOS,fl + NM00ER2.

Then K is the natural image in V[L0, Mo] of the closed subset of R3

defined by the condition

C`I'

- To +2 [Lo, M0, t]

(D - .bo + 2[Lo, Mo,

where G is the closure of G; G is a closed subgroup of R2.

Three cases occur: (1°) G is discrete, (2°) dim 6 = 1, (3°) 6

= R2.

188 III,§2,1

1°) G is discrete, that is, C = G.

This is the case if and only if NL° and

NM° are rational (use the rapidity of convergence to an irrational number

of its rational approximations given by its continued fraction develop-

ment), that is, if and only if (1.6) holds. Then K = K; the elements of the

subgroup Z of Z3 leaving invariant the curve R c R3 given by the

equations (1.1) are the

rl, l;) in Z3 such that

N1 = L1C,

N,tl = M1 C-

By (1.6)4, N1 divides C. Thus Z is generated by (L1, M1, N1) E Z3.

2°) dim G = 1.

Then G is the set of (0, t) e R2 satisfying a condition

of the form

L10 + M1t E Z,

where L1, M1 E R; (1.17)

by the definition of G, G is the subgroup (1.17) of R2 if and only if (1.8) is

satisfied and G is not discrete, that is, by (1°), if and only if NL° and NM°

are connected by a unique affine relation with integer coefficients, which

is (1.8).

Assuming this hypothesis, by definition (1.16) of k and definition (1.17)

of G, K is the image under (1.2) of the manifold R2 in R3 given by equation

(1.9); obviously (1.10) defines the subgroup Z2 of Z3 that leaves A2

invariant; and (1.10) implies by (1.8)3 and (1.12) that

L2, M2, and N2 divide , ri, and t;, respectively. (1.18)

By (1.13), the three elements (1.14) are contained in Z2. On one hand,

(1.14)1 generates the subgroup of Z2 given by the equation

=0,

because G.C.D.(M2N3, M3N2) = 1 by (1.12)1, (1.13)2, and (1.13)3. Thus

G.C.D.(N3, M3) = 1, which implies that the values taken by

in the

subgroup of 22 generated by the elements (1.14)2 and (1.14)3 are all the

multiples of L2. Thus, by (1.18), the three elements (1.14) of Z2 generate

3°)

R2.

By (1°) and (2°), G = R2 if and only if NL° and NM° are

not connected by any affine relation with integer coefficients. If G = R2,

then K is the image of R3 under (1.2); thus K = V[L0, M0].

Invariant measures on V[L0, M0].

Recall that V[L0, M0] has a measure

> 0 that is invariant under the characteristic vector K of H [(3.2) of §1] :

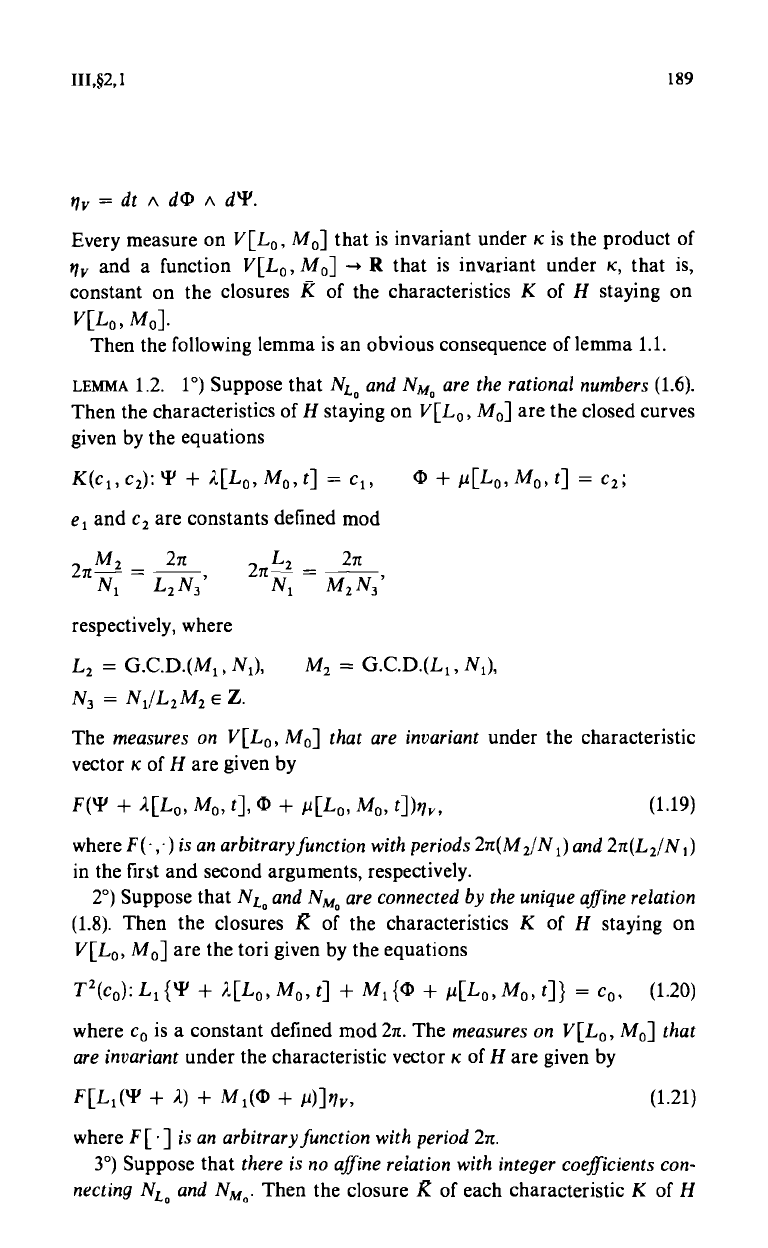
III,§2,1

189

tlv = dt A dO A dW.

Every measure on V [LO, M0] that is invariant under K is the product of

tlv and a function V[L0, Mo] -+ R that is invariant under K, that is,

constant on the closures k of the characteristics K of H staying on

V[Lo, Mo].

Then the following lemma is an obvious consequence of lemma 1.1.

LEMMA 1.2.

1°) Suppose that NL° and NM,, are the rational numbers (1.6).

Then the characteristics of H staying on V[L0, Mo] are the closed curves

given by the equations

K(c1, c2): `P + %[Lo, Mo, t] = c1,

b + p[Lo, Mo, t] = c2;

el and c2 are constants defined mod

M2 27c

= L2N3,

M2 N3,

respectively, where

L2 = G.C.D.(M1, N1),

M2 = G.C.D.(L1, N1),

N3 = N1/L2M2 E Z.

The measures on V[L0, Mo] that are invariant under the characteristic

vector K of H are given by

F(`f' + )[Lo, Mo, t], cD + p[Lo, Mo, t])r)v,

(1.19)

where is an arbitrary function with periods 27r(M2/N1) and 27t(L2/N,)

in the first and second arguments, respectively.

2°) Suppose that NL, and NM,, are connected by the unique affine relation

(1.8). Then the closures K of the characteristics K of H staying on

V[L0, Mo] are the tori given by the equations

T2(co): L1 {`P + ).[Lo, Mo, t] + M1 {db + p[Lo, Mo, t]} = co,

(1.20)

where co is a constant defined mod 27t. The measures on V[L0, Mo] that

are invariant under the characteristic vector K of H are given by

F[L1(`P + A) + M1(b + u)]'lv,

(1.21)

where F[ ] is an arbitrary function with period 2n.

3°) Suppose that there is no affine relation with integer coefficients con-

necting NL,, and NM,. Then the closure K of each characteristic K of H

190

111,§2,1-11 1,§2,2

staying on V[L0, Mo] is V[LO, Mo]. Every measure on V[L0, Mo] that is

invariant under the characteristic vector x of H is given by

const. g v .

(1.22)

The following theorem is an easy consequence.

THEOREM 1.

1°) The tori V[L0, Mo] on which a lagrangian solution U of

the equation

aU = 0 mod

(a is the operator associated to H)

with lagrangian amplitude >,0 may be defined are defined by the condition

(3.11), (3.12) of §1: there exist three integers

1,rn,n

such that

Iml 1<n,

Lo = h(l + i),

Mo = hm,

Lo + N[LO, Mo] = hn.

2°) A necessary and sufficient condition for the solution U defined on such

a torus to be unique up to a constant factor is that the derivatives of N,

NL[LO, Mo],

NM[LO, Mo],

are not connected by any affine relation with integer coefficients.

Proof.

By theorem 6 of II,§3, the condition that there exist such a solu-

tion on V[LQ, Mo] is Maslov's quantum condition. The condition that it

be unique is the condition that the invariant measure nv on V[L0, Mo]

be unique (up to a constant factor). In section 3 of §1, Maslov's quantum

condition is formulated as (3.11)-(3.12). The condition that the invariant

measure be unique is given in lemma 1.2.

2. Compact Lagrangian Manifolds V, Other Than the Tori V [Lo , Mo],

on Which Solutions of the Equation a U = 0 mod(

I/v2) with Lagrangian

Amplitude >,0 Exist

We shall show that such manifolds V exist only exceptionally.

The calculation of their Maslov index (lemmas 2.3 and 2.4) uses the

following properties.

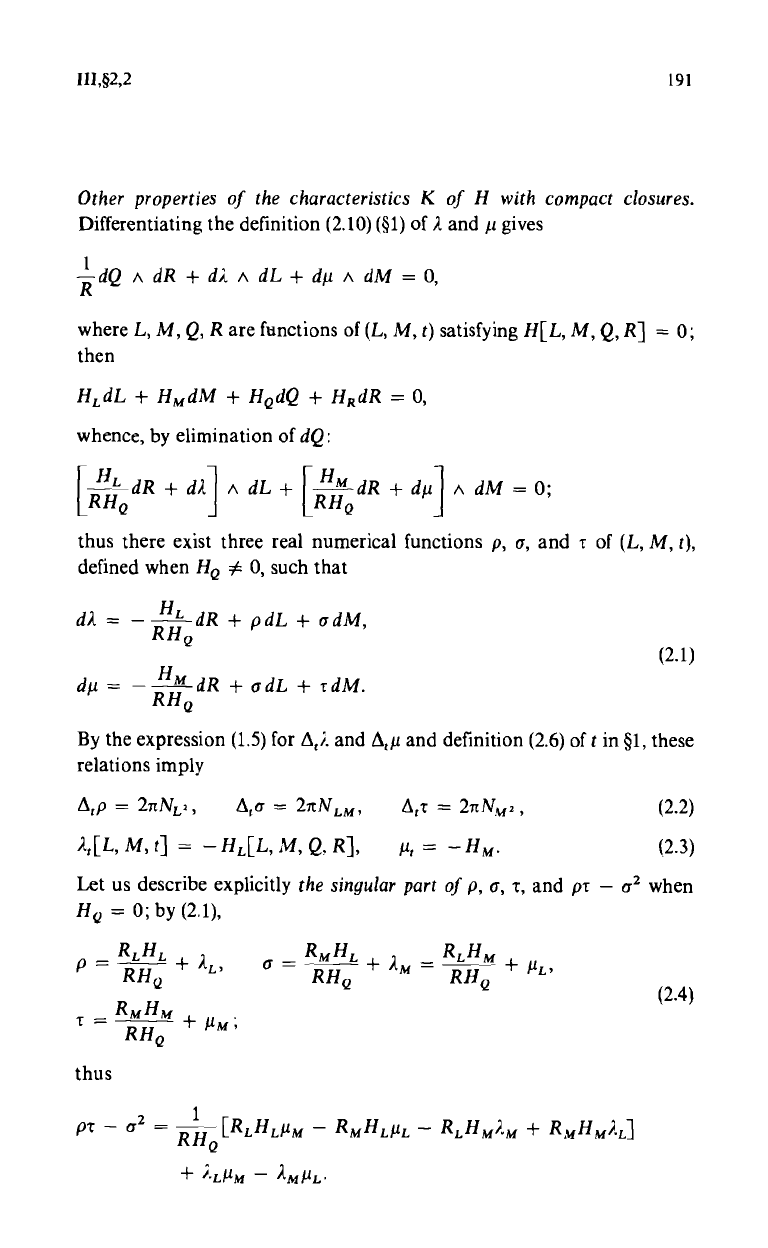
III,§2,2

191

Other properties of the characteristics K of H with compact closures.

Differentiating the definition (2.10) (§1) of A and p gives

R dQ n dR + dJ n dL + dp n dM = 0,

where L, M, Q, R are functions of (L, M, t) satisfying H[L, M, Q, R] = 0;

then

HLdL+HMdM+HQdQ+HRdR=0,

whence, by elimination of dQ:

[j_dR+d1]AdL+[!jy-dR+dp]AdM=o;

R Q

thus there exist three real numerical functions p, a, and r of (L, M, t),

defined when HQ * 0, such that

dR + p dL + a dM,

dA _ -

RHQ

,u = -

dR + a dL + T dM.

By the expression (1.5) for A. and O,u and definition (2.6) of tin §1, these

relations imply

A,p = 21tNL1,

A,a = 21tNLM,

O,t = 21tNMz, (2.2)

A,[L, M, t] _ -HL[L, M, Q, R],

p, _ -HM.

(2.3)

Let us describe explicitly the singular part of p, a, t, and PT - a2 when

HQ = 0; by (2.1),

p =

RLHL

+ L,

a =

RMHL

+ M = RLHM + AL,

RHQ

T =

RMHM

+ AM;

RHQ

thus

pt - a2 = RH [RLHLPM - RMHLPL - RLHMI.M +

+ i.L/M - MAL-

192

III,§2,2

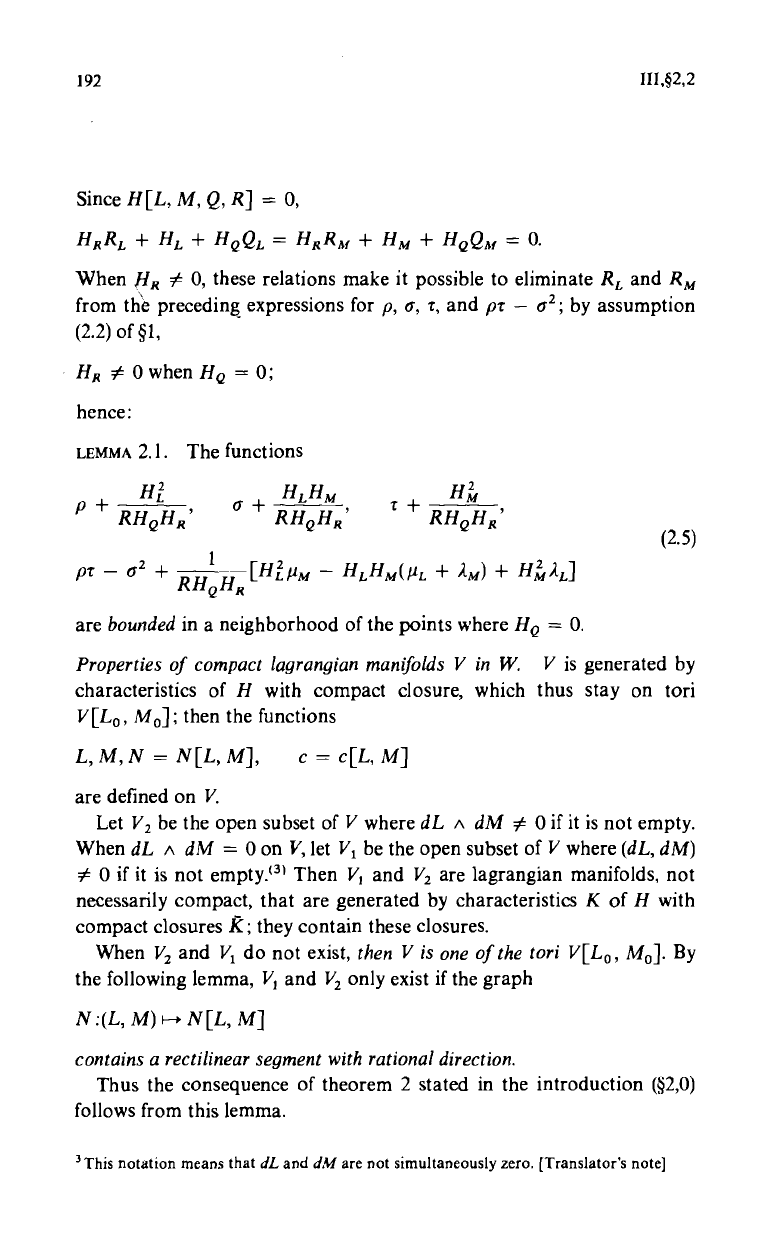
Since H[L, M, Q, R] = 0,

HRRL+HL +HQQL=HRRM+HM+HQQM=O.

When HR :j6 0, these relations make it possible to eliminate RL and RM

from the preceding expressions for p, a, T, and pr - 62; by assumption

(2.2) of §1,

HR # 0 when HQ = 0;

hence:

LEMMA 2.1.

The functions

2 2

HLHM

RHQHR,

RHQHR'

PT -

1 _

RH H

[Hi 12M - HLHM(uL + AM) + H2 AL]

Q R

are bounded in a neighborhood of the points where HQ = 0.

Properties of compact lagrangian manifolds V in W. V is generated by

characteristics of H with compact closure, which thus stay on tori

V[L0, Mo]; then the functions

L, M, N = N [L, M], c = c [L, M]

are defined on V.

Let V2 be the open subset of V where dL A dM 96 0 if it is not empty.

When dL A dM = 0 on V, let Vt be the open subset of V where (dL, dM)

: 0 if it is not empty

t3) Then Vt and V2 are lagrangian manifolds, not

necessarily compact, that are generated by characteristics K of H with

compact closures k; they contain these closures.

When V2 and Vt do not exist, then V is one of the tori V[L0, Mo]. By

the following lemma, Vt and V2 only exist if the graph

N : (L, M) i--+ N [L, M]

contains a rectilinear segment with rational direction.

Thus the consequence of theorem 2 stated in the introduction (§2,0)

follows from this lemma.

'This notation means that dL and d.41 are not simultaneously zero. [Translator's note]

III,§2,2

193

LEMMA 2.2.

1 °) On V2, N is an aff ne function of (L, M). More precisely,

L1dL + M1dM + N1dN = 0,

(2.6)

where (L1, M1, N1) E Z3,

G.C.D.(L1, M1, N1) = 1.

V2 is defined in W by the datum of a function F of two variables and by the

equations

`P + I[L, M, t] + FL[L, M] _'V + µ[L, M, t] + Fr,[L, M] = 0.

(2.7)

More precisely, in the space R5 with the coordinates (L, M, `P, 'V, t),

equations (2.7) define a 3-dimensional manifold P2. By (1.5), where

NL = -L1/N1,

NM = -M,/N,,

the elements q, c) of the subgroup Z of Z3 generated by (L1, M, , N,) E Z3

act on V2 as follows:

(5, n, C): (L, M, T, (D, t) i-- (L, M, `P + 2irS,'V + 2nq, t + c [L, M] S ). (2.8)

We have

V2 = V2/Z

(2.9)

V2 has a measure that is invariant under the characteristic vector of H,

given by

qv = dL A dM A dt.

2°) On V1, L, M, and N are affine functions of a single variable s. More

precisely,

dL -dM=dN=ds

L1 M1 N1

(2.10)

where (L1, M1)

(L1, M1, N1) E Z3,

G.C.D.(L1, M1, N1) = 1.

V, is defined in W by the datum of three functions of s-the affine functions

L and M satisfying (2.10) and F-and by the equations

L = L(s), M = M(s),

L1{`P + %[L, M, t]} + M1{dV + u[L, M, t]} + F,(s) = 0.

(2.11)

More precisely, equations (2.11) define a 3-dimensional manifold V1 in R5