Leray J. Lagrangian Analysis and Quantum Mechanics: A Mathematical Structure Related to Asymptotic Expansions and the Maslov Index

Подождите немного. Документ загружается.

164 III,Introduction

characterize the equations having solutions (thus the energy levels) and

also the solutions. These are Schrodinger's three quantum numbers. The

lagrangian manifolds on which these solutions are defined are 3-dimen-

sional tori T(l, m, n) [see theorem 7.1, 2°)], given by the equations

T(l, m, n): H(x, p) L2(x, p) - const. = M - const. = 0.

These constants have the same values as in (1) and depend on (1, m, n).

In §2, we determine solutions of the lagrangian equation

a U = 0 mod

(2)

that are defined mod(]/v) on a compact lagrangian manifold V and that have

lagrangian amplitude > 0. This amounts to a formal problem analogous to

the boundary-value problem whose study constitutes the classical study of

the Schrodinger equation; the condition concerning behavior at infinity in

the classical problem is now replaced by the condition that V is compact.

Always, the condition of existence is the same: it is characterized by a triple

of quantum integers; but the solution corresponding to such a tripel is not

necessarily unique.

In §3, we determine solutions defined on a compact lagrangian manifold

of the lagrangian system

aU = (aL2 - const.)U = (aM - const.)U = 0,

(3)

where the constants are formal numbers that are real mod(]/v2) and H

is the hamiltonian of the relativistic or nonrelativistic electron; then a, aLs,

and aM commute. Here theorem 7.2 is applied. Solutions again are charac-

terized by a triple of quantum integers (1, m, n). Solutions of problem (1) are,

mod(]/v), those of problem (3).

In §4, we recall the problem classically posed regarding the Schrodinger

and Klein-Gordon equations: to find a function

u:E3-C,

that is square integrable-as is its gradient-and that satisfies the partial

differential equation

au = 0.

(4)

In §4, we recall the resolution of this problem in order to show how it

III,Introduction

165

differs essentially from that of the preceding problems. We observe that all

these problems define the same energy levels, but we do not explain why this

happens.

The difficulties encountered in §2 and the length of the calculations

used in §3 and §4 contrast with the simplicity of §1; §1 justifies the following

conclusion.

CONCLUSION

Applying the Maslov quantization (I1,§3,6 and 7) to an

atom with one electron placed in a constant magnetic field gives the ob-

servable quantities the same values as does wave mechanics. However, the

Maslov quantization is directly related to corpuscular mechanics, hence to

the old quantum theory. Nevertheless it does not have the shortcomings of

the old theory. It has a logical justification (chapters I and II); it does not

require the determination of the nonquantized trajectories of the electron,

but only the knowledge of the classical first integrals L and M of Hamilton's

system, which defines these trajectories.

The probabilistic interpretation of this quantization is the following:

In the state defined by a choice of a triple of quantum integers (1, m, n),

the point (x, p), representing both the position x and the momentum p of the

electron, belongs to a 3-dimensional torus T(l, m, n) in the 6-dimensional

space Z(3) = E3 O+ E3; the probability that (x, p) belongs to a subset of

T(l, m, n) is defined by the invariant measure q on this torus T(1, m, n)

[see §1,(3.16)].

Remark. Let

(1, m, n) 0 (1', m', n')

be two distinct triples of quantum integers. They define (§1) tori whose

intersection is empty:

T(l, m, n) n T(1', m', n') = 0.

Let U and U' be two lagrangian functions defined on T(1, m, n) and

T(1', m', n'), respectively. Then their scalar product is [II,§2,theorem

3.2,3°)]

(UI U') = 0.

Historical note.

V. P. Maslov did not give this application of his quanti-

zation. He only studied the case of quantum numbers tending toward

infinity, that is, the "correspondence principle" in quantum mechanics.

166

III,§1,1

§1. A Hamiltonian H to Which Theorem 7.1 (Chapter II, §3)

Applies Easily; the Energy Levels of a One-Electron Atom, with

the Zeeman Effect

Theorem 7.1 of II,§3 assumes that

I functions on 0 c Z(1) are given

that are pairwise in involution. In chapter III, we choose I = 3 and choose

a classical triple of such functions.

1. Four Functions Whose Pairs Are All in Involution on E3 m E3

Except for One

Let X = X* = E3 denote 3-dimensional euclidean space. Let us apply

chapter II to

1=3,

Z(3)=E3rE3,

thus making a choice of a frame Ro of Z : theorem 5 of II, §3 spares us

the use of another frame.

But in E3 we use both a fixed orthonormal frame (I,, 12, 13) and a

moving orthonormal frame. Let

xeX=E3,

PEX*=E3;

let (x,, x2, x3) and (pl, P2, p3) be the coordinates of x and pin (1,, I2113):

P = Y_ Pj1j'

J=1

Let us define five functions of (x, p):

R(x) = Ixl, P(p) = I pI, Q(x, p) = <p, x>,

L(x, p) = Ix A PI,

M(x, p) = x1p2 - x2 p, (third component of x A p);

they are connected by the obvious relations

L2 + Q2 = P2R2,

IMI _< L, 0 _< P, 0 <, R.

(1.2)

Then the vector 13 has a priviledged role: it will be, for example, the

direction of the magnetic field producing the Zeeman effect.

In E 3 Q E 3, the characteristic system of d< p, dx) is

dx = dp = 0.

Every function E3 m E3 -+ R is a first integral of this system. Thus the

III,§ 1,1

167

Poisson bracket ( , -)(definition 2 of II,§3) of two such functions is defined.

By formula (3.14), of II,§3,

(L, M) = (L, Q) = (L, R) = (M, Q) = (M, R) = 0,

(Q, R) = R. (1.3)

From theorem 2 (E. Cartan) of II,§3, a quadrature locally defines four

real numerical functions f

o, f 1, f 3, f 5 on

E 3 (D E 3 such that

<p,dx> = dfo + f1dL + f3dM + f5dR.

(1.4)

Let us now define the functions ff explicitly. Let (J1i J2, J3) be the

orthonormal moving frame, defined for L 0, such that

x=RJ1i

x n p=LJ3,

which implies

p = QR-1J1 +LR-'J2,

P 0 0, R 0.

Let w1, w2, and w3 be the infinitesimal components of the displacement

of that frame relative to itself (G. Darboux-E. Cartan); these are the

pfaffian forms

wl = <J3, dJ2> = -<J2, dJ3>,

w3 = <J2,dJ1>

such that

dJ1 = w3J2

dJ3 = w2J1

w2 = <J1, dJ3>,

dJ2 = ())1J3 - w3J1,

- w2J3,

- w1J2.

Recall that exterior differentiation of these relations gives the equations

dw1 = 0)3 A w2, d()2 = w1 A w3, dw3 = w2 A w1

(which are the structural equations of the orthogonal group).

By (1.7), differentiating (1.5)1 gives

(1.8)

dx = (dR)J1 + Rw3J2 - Rw2J3,

(1.9)

from which follows

< p, dx> = QR-1 dR + Lw3 (1.10)

by (1.6).

In order to transform this formula into a formula of the form (1.4),

168

III,§ 1,1

let us introduce the Euler angles CD, `P, and O; these are the parameters

of the frame (J1, J2, J3) that are defined as follows for J3

±13, that

is, IMl < L:

0 is the angle between 13 and J3 ; 0 < 0 < it;

a rotation by CD around 13 transforms (11,12, 13) into (I1, 12, 13) such

that

12sinO = 13 A J3;

a rotation by 0 around 12 transforms (Ii,12, I3) into (I' l, I2, J3);

a rotation by `P around J3 transforms (Il, I2, J3) into (J1, J2, J3).

It is obvious that

M = L cos 0, (1.11)

CD and `P are defined mod 2n. We have the classical formulas:

J1 = (cosCDcos`Pcos0 - sin (D sin `P)I1

+ (sin CD COST cos 0 + cos CD sin T)12 - cos `P sin 013 ,

J2 = (- cosCsin`PcosO - sin (D cos')11

(1.12)

+ (- sin Csin `PcosO +cos(D cos'F)12 + sin `P sinO13,

J3 = cos(1) sin 011 + sin CDsin 012 + cos013;

wl = - cos`PsinOdC + sin `PdO,

cot = sin `P sin 0 dC + cos `P dO, (1.13)

cos = cos 0 dC + d'.

By (1.11) and (1.13)3, the explicit expression we sought for formula

(1.10) is

p, dx> = QR

+ Ld`P + MdC.

(1.14)

This fundamental formula is of the form (1.4), in agreement with E. Cartan's

theorem, which is our guide.

Complementary formulas.

By (1.7), differentiating (1.6) gives

dp = [d(QR-')

- LR-1w3]J1 + [d(LR-1) + QR-'0)3]J7.

+ [LR-1co1 -

QR-'w2]J3.

(1.15)

1II,§ 1,1

169

By (1.9), d3x = dx1 A dx2 A dx3 is given by

d3x = R2(dR) A a)2 A (A3;

hence, by (1.15),

d3x A d3p =

A dQ A dL A (01 A

(02 A (J)3,

(1.16)

or, replacing the o )j

their expressions (1.13) and eliminating O by means

of (1.11),

d 3x A d 3 p= dL A dM A dQ A

A dD A d`P.

(1.17)

Let 516 be an open subset of Z(3) = E3 Q E3 on which

IMI <L;

we can use the coordinates

L, M, Q, R, D, `P

on 516, where 4) and `I' are defined mod 2n.

Remark 1 . L O on S16 ; thus by (1.2)1, P

O, R

LEMMA 1.

Let V be a lagrangian manifold in 06 (dim V = 3). Let us

replace each of the preceding functions and differential forms by its

restriction to V.

1°) The apparent contour ER. of V is the surface in V where

ER°:dR A w2 A w3 = 0 on V. (1.18)

2°) In a neighborhood of a point of ER°, there exists a differential form

m on V of degree 3, that is nowhere zero, such that on ER.

dQ A cot A 0)3/m > 0,

dL A dR A cot/rw >1 0,

dRAco3Awl/w>, 0,

(1.19)

where the three left-hand-side functions do not vanish simultaneously.

There exists a constant c such that, in a neighborhood of this point of V,

the Maslov index MR. has the value

when dR A 2 A (D3/rv < 0

MR°

jl (1.20)

1 + c when dR A cot A (J)3/0 > 0.

170

III,§1,1-III,§ 1,2

Proof of 1°).

Use expression (1.16) for d'x.

Proof of 2°).

Let us calculate the jump of mR. across ERo by applying

theorem 3.2 of I,§3. By (1.9), (1.15), and (1.18), the components d;x and

dap of dx and dp in the frame (J1, J2, J3) satisfy on V, at the points of

2:R0

dtp A d2x A d3x = R2[d(QR-') - LR-'w3] A w2 A w3

=RdQAw2Aw3,

d1x A d2p A d3x = -RdR A [d(LR-') + QR-'w3] A w2

=dLAdRAw2,

d1x A d2x A dap = (dR) A 0-)3 A [Lw1 - Qw2]

=LdRAw3Awl.

The existence of w satisfying (1.19) and (1.20) follows by this theorem

(theorem 3.2, of I,§3).

2. Choice of a Hamiltonian H

Let

be an infinitely differentiable function in involution with L and M, that is,

by (1.14), a composition with the functions L, M, Q, and R:

H(x, p) = H[L(x, p), M(x, p), Q(x, p), R(x)]. (2.1)

H[ ] is an infinitely differentiable function defined on an open subset 04

of the space R4 with the coordinates L, M, Q, R. Assume that, on 524

0 < R,

I MI < L, (HQ, HR) (0, 0) when H = 0.

(2.2)

HQ denotes the function aH[L, M, Q, R]/8Q.

By (1.3), the three functions H, L, and M of (x, p) are pairwise in involu-

tion, so that the results of II,§3 can be applied explicitly to the lagrangian

operator a associated to H.

From theorem 2 (E. Cartan) of II,§3, a quadrature locally defines four

real numerical functions on 526,

90,91+92,93,

111,§ 1,2

171

such that

< p, dx> = dgo + g1 dL + 92 dM + g3 dH.

(2.3)

We shall use this formula when H = 0; it is made explicit by lemma 2,

assuming

H = 0.

According to (1.14), the quadrature is the definition of the function 0 by

(2.8).

Notation. Let W be the hypersurface in 06 given by the equation

W : H(x, p) = 0

(Compare II,§3). Let (Lo, M0) be a pair of real numbers such that IM01

< Lo; let V[L0, M0] denote any connected component of the subset of

W given by the equations

V [LO, MO] : H(x, p) = 0,

L(x, p) = Lo,

M(x, p) = Mo.

(2.4)

W is the union of the V [La, M0]. By theorem 7.1, of II,§3, V [LO, M0] is

a lagrangian manifold. It is the topological product of

the 2-dimensional torus with the coordinates D and T mod 27r,

a connected curve I [Lo , M0] in the open half-plane with the coordinates

Q, R > 0.

The equation of this curve is

T [L0, MO]: H[L0, MO, Q, R] = 0.

(2.5)

By (2.2)3, this curve does not have any singular points.

Let us define a real numerical function t of [L, M, Q, R] on W up to the

addition of a function of (L, M) by the condition

dt = RHQ[L,IM,

Q, R]

RHR[L,dM,

Q, R]

on F [L, M];

(2.6)

when the curve T[L, M] is a closed curve, then t is defined modc[L, M],

where

c [L,

_ dQ . (2.7)

[L'

M] _

fr[L,M]

RHQ

-fr[L,M]

RHR'

172

III,§1,2

t is monotone on F; (L, M, t, (D, `P) forms a system of local coordinates on

Let us define another real numerical function S2 on W up to the addition of

a function of (L, M) by

A2 = QRon F[L, M];

(2.8)

when the curve F[L, M] is

a closed curve, then

defined

mod2nN[L, M] where

1 dR

> 0

M] =

N[L

r[LM]

S2 is not monotone on F. Let its differential be denoted by

dc2[L, M, t] = Q R + 1 [L, M, t] dL + µ[L, M, t] dM.

(2.10)

The properties of the functions ) and µ thus defined will be specified in §2.

Now we can give the explicit expression of the restriction of (2.3) to W.

LEMMA 2.

1°) The restriction of the pfaffian form

w=<p,dx>

to Wis

cow=d(S2+L`P+MD)-O +`P)dL-(µ+(D)dM.

(2.11)

2°) The characteristic system of dww is Hamilton's system:

HH(x, P)

H,,(x, p)

H(x, p) = 0. (2.12)

Its first integrals are compositions with the functions

L, M,2+`P,µ+ b;

(2.13)

L and M are in involution; 2 + `P and p + (D are also in involution.

3°) On the solution curves of this system, that is, the characteristic curves

of W, we have

_ --

_ dR _ dP

_ d(D

dt =

H,(x,

HH(x, p)

RHQ[L, M, R, Q]

HL[' ]

HM[' ]

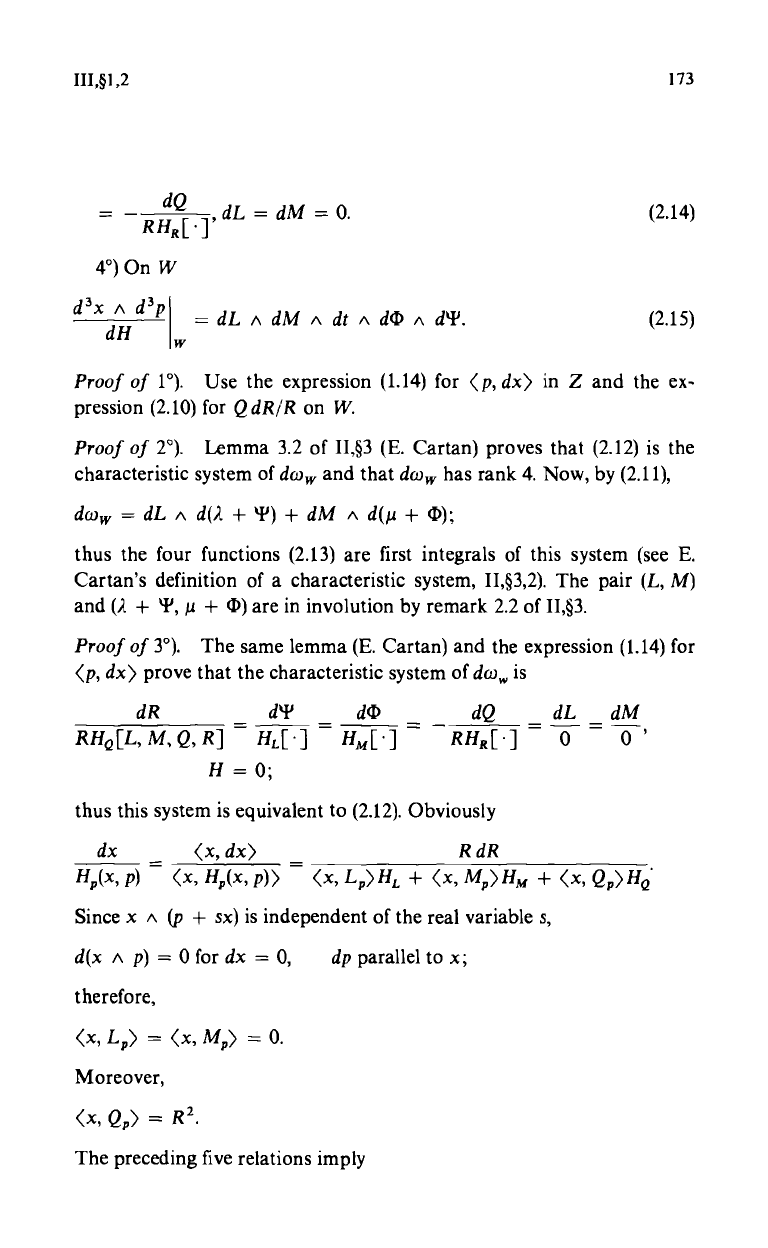
III,§1,2

dQ ,dL=dM=0.

RHR[-]

4°) On W

d 3x A d 3

P I

_ dL n dM n dt n d(D n d`)'.

173

(2.14)

(2.15)

Proof of 1°). Use the expression (1.14) for <p, dx> in Z and the ex-

pression (2.10) for Q dR/R on W.

Proof of 2°).

Lemma 3.2 of II,§3 (E. Cartan) proves that (2.12) is the

characteristic system of dww and that dww has rank 4. Now, by (2.11),

dww, =dL n d(.1 +`Y)+dM n d(µ+(D);

thus the four functions (2.13) are first integrals of this system (see E.

Cartan's definition of a characteristic system, II,§3,2). The pair (L, M)

and (2 + `F, p + 1) are in involution by remark 2.2 of II,§3.

Proof of 3°).

The same lemma (E. Cartan) and the expression (1.14) for

<p, dx> prove that the characteristic system of dw,, is

d`P

dD dQ _ dL

_ dM

RHQ[L,M,Q,R]

HL[

HM[']

RHR[']

0 0

H = 0;

thus this system is equivalent to (2.12). Obviously

dx _

<x, dx>

R dR

HP(x, p)

<x, HP(x, p)>

<x, LP>HL + <x, MP>HM + <x, QP>HQ

Since x A (p + sx) is independent of the real variable s,

d(x A p) = 0 for dx = 0,

dp parallel to x;

therefore,

<x, LP> = <x, MP> = 0.

Moreover,

<x, Q> = R2.

The preceding five relations imply