Leray J. Lagrangian Analysis and Quantum Mechanics: A Mathematical Structure Related to Asymptotic Expansions and the Maslov Index

Подождите немного. Документ загружается.

xii

Preface

are the images of the sources of light; nevertheless geometrical optics

holds beyond the caustics.

V P. Maslov introduced an index (whose definition was clarified by

I. V. Arnold) that described these phase jumps, and he showed by a con-

venient use of the Fourier transform that these amplitude singularities are

only apparent singularities. But he had to impose some "quantum con-

ditions." These assume that v has some purely imaginary numerical value

vo, in contradiction with the previous assumption about v, namely, that

v is a parameter tending to i oo. The assumption that v tends to i oo is

necessary for the Fourier transform to be pointwise, which is essential for

Maslov's treatment. A procedure, avoiding that contradiction and guided

by purely mathematical motivations, that makes use of the Fourier trans-

form, expressions of the type (1), Maslov's quantum conditions, and the

datum of a number vo does exist, but no longer tends to define a function

or a class of functions by its asymptotic expansion. It leads to a new

mathematical structure, lagrangian analysis, which requires the datum of a

constant vo and is based on symplectic geometry. Its interest can appear

only a posteriori and could be quantum mechanics. Indeed this structure

allows a new interpretation of the Schrodinger, Klein-Gordon, and Dirac

equations provided

vo = ii = 2h1, where h is Planck's constant.

Therefore the real number 2ni/vo whose choice defines this new mathe-

matical structure can be called Planck's constant.

The introductions, summaries, and conclusions of the chapters and

parts constitute an abstract of the exposition.

Historical note. In Moscow in 1967 I. V. Arnold asked me my thoughts

on Maslov's work [10, 11]. The present book is an answer to that question.

It has benefited greatly from the invaluable knowledge of J. Lascoux.

It introduces vo for defining lagrangian functions on V (chapter II, §2,

section 3) in the same manner as Planck introduced h for describing the

spectrum of the blackbody. Thus the book could be entitled

The Introduction of Planck's Constant into Mathematics.

January 1978

College de France

Paris 05

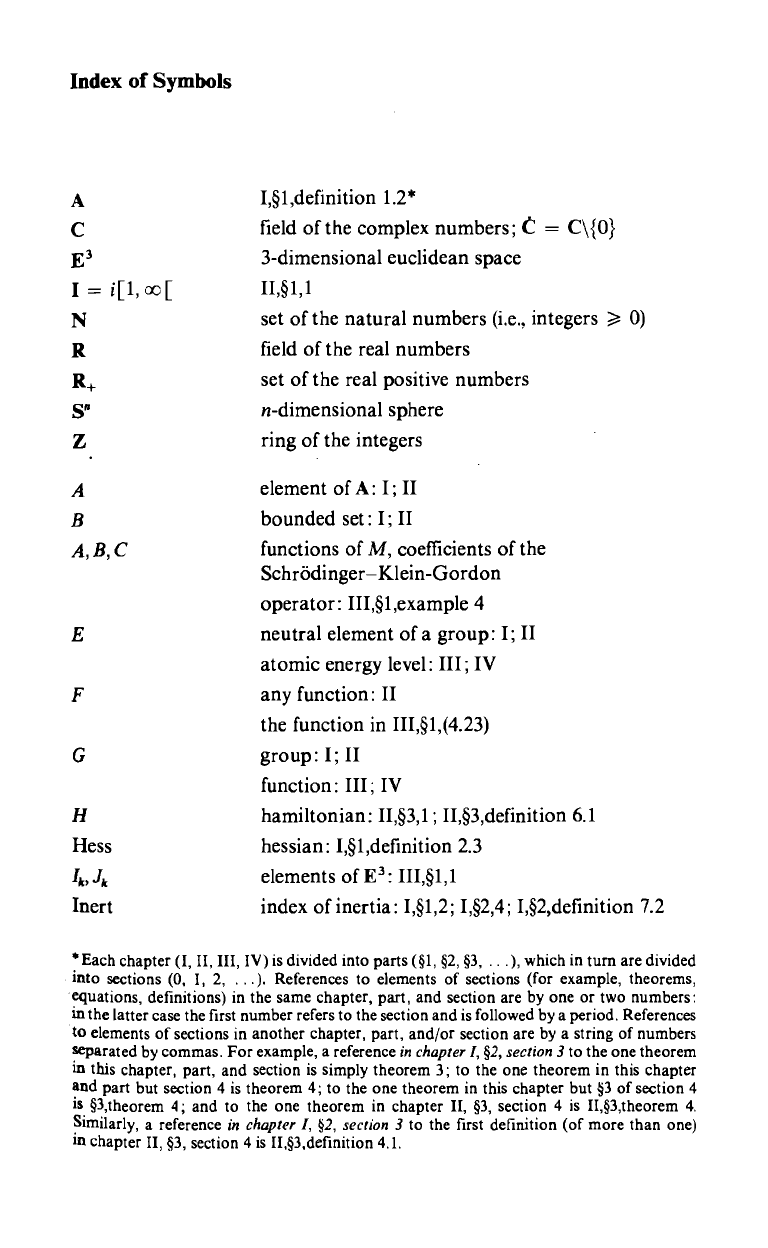
Index of Symbols

A I,§1,definition 1.2*

C field of the complex numbers;

= C\{0}

E3 3-dimensional euclidean space

II,§1,1

N set of the natural numbers (i.e., integers > 0)

field of the real numbers

R+

set of the real positive numbers

n-dimensional sphere

Z ring of the integers

element of A: I; II

bounded set: I; II

A, B, C

functions of M, coefficients of the

Schrodinger-Klein-Gordon

operator: III,§1,example 4

neutral element of a group: I; II

atomic energy level: III; IV

any function: II

the function in III,§1,(4.23)

group: I; II

function: III; IV

H hamiltonian: II,§3,1; II,§3,definition 6.1

Hess hessian: I,§1,definition 2.3

Ik,Jk

elements of E3: III,§1,1

Inert

index of inertia: I,§1,2; I,§2,4; I,§2,definition 7.2

* Each chapter (1, II, III, IV) is divided into parts (§l, §2, §3, ... ), which in turn are divided

into sections (0, I, 2, ... ). References to elements of sections (for example, theorems,

equations, definitions) in the same chapter, part, and section are by one or two numbers:

in the latter case the first number refers to the section and is followed by a period. References

to elements of sections in another chapter, part, and/or section are by a string of numbers

separated by commas. For example, a reference in chapter !, §2, section 3 to the one theorem

in this chapter, part, and section is simply theorem 3; to the one theorem in this chapter

and part but section 4 is theorem 4; to the one theorem in this chapter but §3 of section 4

is §3,theorem 4; and to the one theorem in chapter II, §3, section 4 is II,§3,theorem 4.

Similarly, a reference in chapter !, §2, section 3 to the first definition (of more than one)

in chapter II, §3, section 4 is II,§3,definition 4.1.

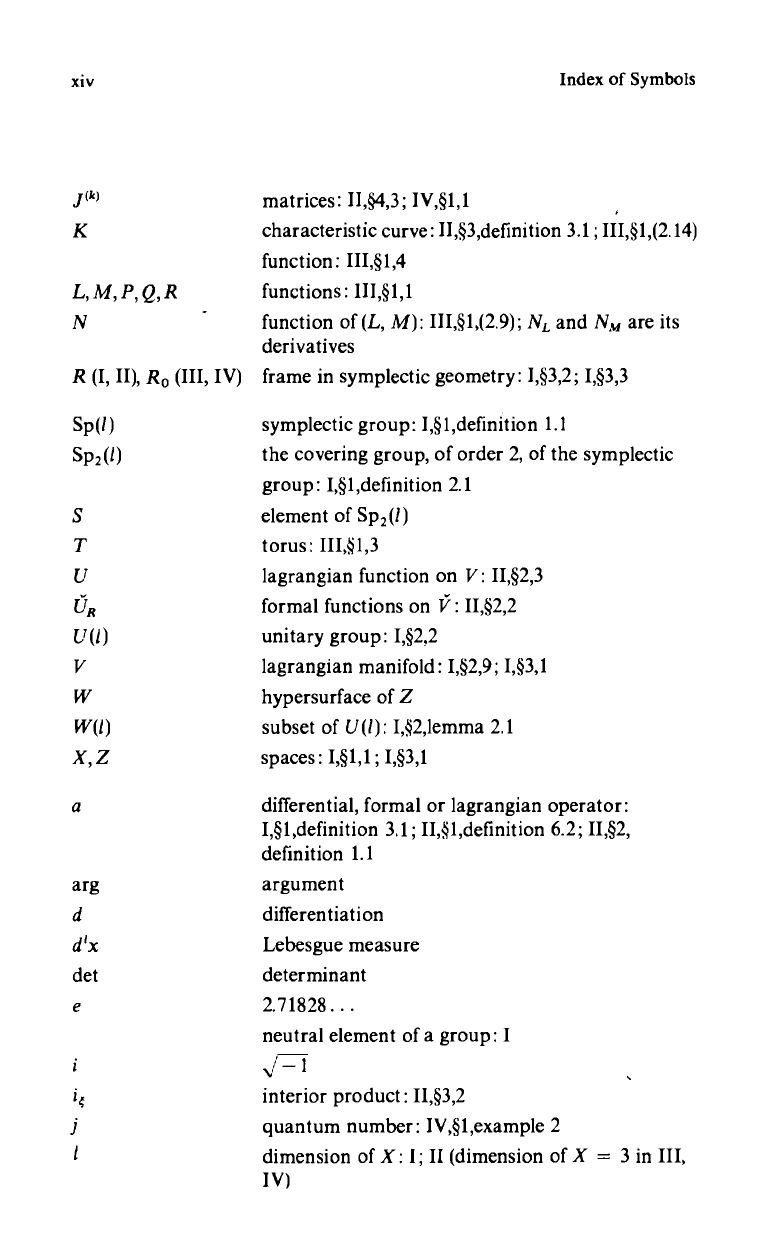
xiv

Index of Symbols

matrices: II,§4,3; IV,§1,1

characteristic curve: II,§3,definition 3.1; III,§1,(2.14)

function: III,§1,4

L, M, P, Q, R

functions: 1II,§ 1,1

function of (L, M): III,§1,(2.9); NL and N,M are its

derivatives

R (I, II), Ro (III, IV)

frame in symplectic geometry: I,§3,2; I,§3,3

SP(1)

SPz(1)

U(1)

W(1)

X' Z

arg

d'x

det

symplectic group: I,§1,definition 1.1

the covering group, of order 2, of the symplectic

group: I,§l,definition 2.1

element of Sp2(1)

torus: III,§ 1,3

lagrangian function on V: II,§2,3

formal functions on V: II,§2,2

unitary group: I,§2,2

lagrangian manifold: I,§2,9; I,§3,1

hypersurface of Z

subset of U(1): I,§2,lemma 2.1

spaces: I,§1,1; I,§3,1

differential, formal or lagrangian operator:

I,§1,definition 3.1; II,§l,definition 6.2; II,§2,

definition 1.1

argument

differentiation

Lebesgue measure

determinant

2.71828.. .

neutral element of a group: I

dimension of X : 1; II (dimension of X = 3 in III,

J-1

interior product: I1,§3,2

quantum number: IV,§1,example 2

IV)

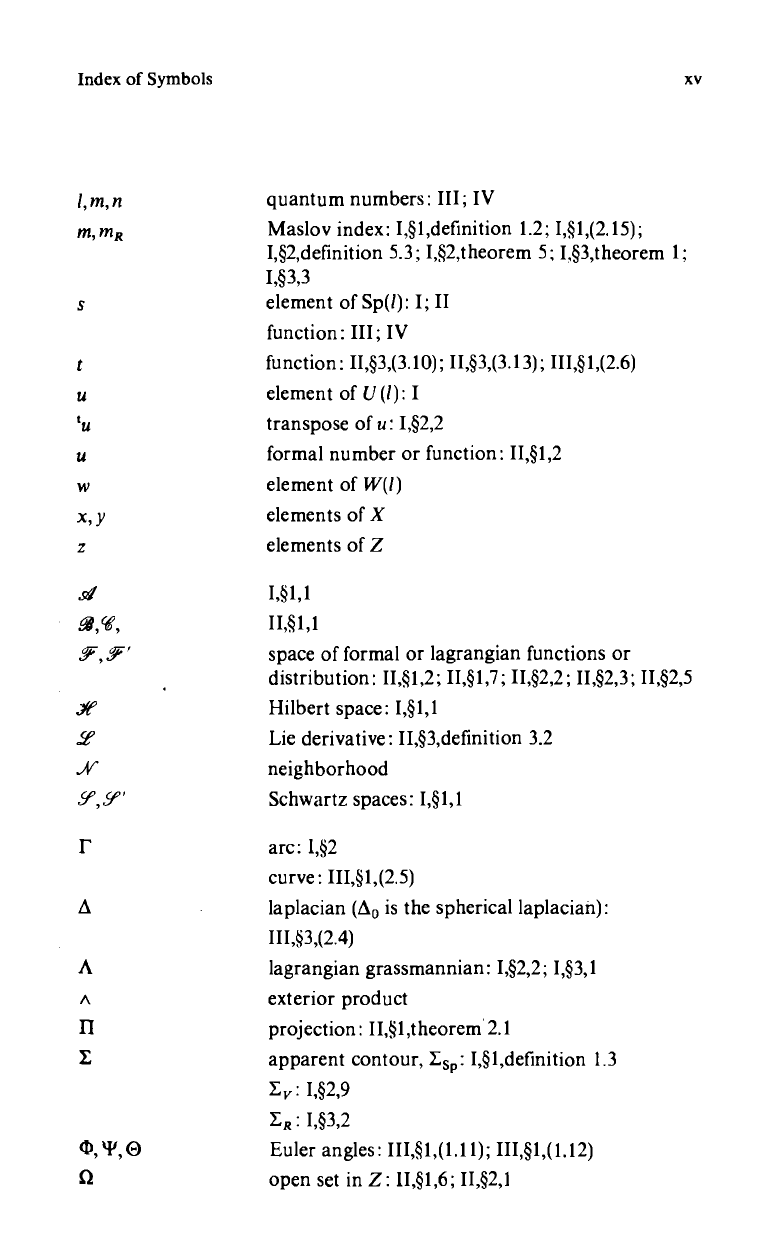
Index of Symbols

1, m, n

m, MR

quantum numbers: III; IV

Maslov index: I,§1,definition 1.2; I,§1,(2.15);

I,§2,definition 5.3; I,§2,theorem 5; I,§3,theorem 1;

Q3,3

element of Sp(1): I; II

function: III; IV

function: II,§3,(3.10) ; 11,§3,(3.13); 111,§ 1,(2.6)

element of U (1) : I

transpose of u: I,§2,2

formal number or function: II,§1,2

element of W(1)

elements of X

elements of Z

I I,§ 1,1

space of formal or lagrangian functions or

distribution: II,§1,2; II,§1,7; II,§2,2; II,§2,3; II,§2,5

Hilbert space: I,§1,1

Lie derivative: II,§3,definition 3.2

neighborhood

Schwartz spaces: I,§1,1

arc: 1,§2

curve: III,§1,(2.5)

laplacian (A0 is the spherical laplacian):

III,§3,(2.4)

lagrangian grassmannian: I,§2,2; I,§3,1

exterior product

projection : II,§1,theorem' 2.1

apparent contour, EsP: I,§1,definition 1.3

Y,,: I,§2,9

ER : I,§3,2

b,'I',0

Euler angles:

III,§1,(1.12)

open set in Z: II,§1,6; II,§2,1

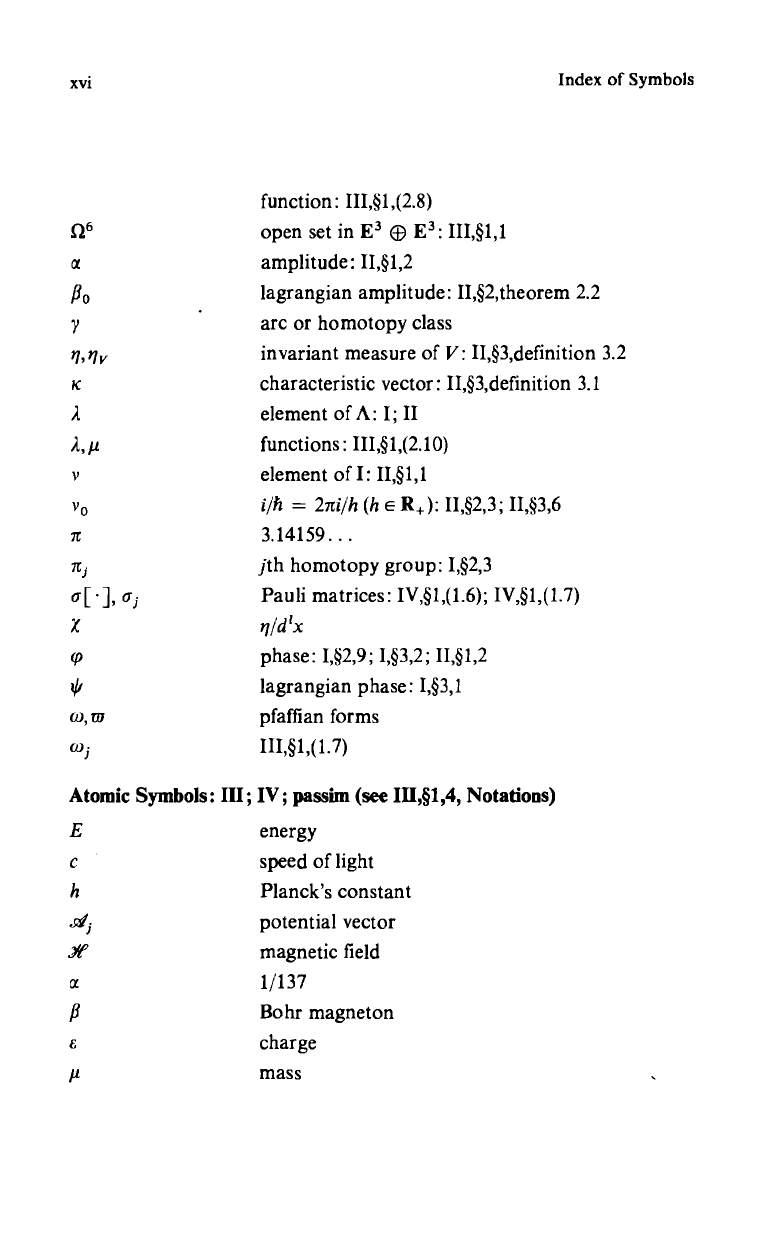
xvi

11, 11 v

A,p

a[ ],Ori

m,m

toi

Index of Symbols

function : III,§1,(2.8)

open set in E3 Q E3: III,§1,1

amplitude: II,§1,2

lagrangian amplitude: II,§2,theorem 2.2

arc or homotopy class

invariant measure of V: II,§3,definition 3.2

characteristic vector: II,§3,definition 3.1

element of A: I; II

functions : III,§1,(2.10)

element of I: II,§1,1

i/h = 2ni/h (h e R+): II,§2,3; II,§3,6

3.14159...

jth homotopy group: I,§2,3

Pauli matrices: IV,§1,(1.6); IV,§1,(1.7)

ri/d `x

phase: I,§2,9; I,§3,2; II,§1,2

lagrangian phase: I,§3,1

pfaffian forms

III,§1,(1.7)

Atomic Symbols: III; IV; passim (see III,§1,4, Notations)

energy

speed of light

Planck's constant

potential vector

magnetic field

1/137

Bohr magneton

charge

mass

Index of Concepts

amplitude

asymptotic class

characteristic curve K

characteristic vector

energy

Euler angles

(1),`I',0

formal number, functions

u, UR

frames

R;(1,,12,13);(JI;J2;J3)

groups

Sp(1); U(1)

hamiltonian

hessian

Hess

homotopy

index of inertia

Inert

interior product

lagrangian amplitude

lagrangian function

lagrangian manifold

lagrangian operator

lagrangian phase

Lie derivative

Maslov index

matrix

J(k)

operator

Planck's constant

VO = i/h

quantum numbers

1, m,n,j

spaces

X, Z,

', A r, ,9", ,9,

symplectic space

The Fourier Transform and Symplectic Group

Introduction

Chapter I explains the connection between two very classical notions: the

Fourier transform and the symplectic group.

It will make possible the study of asymptotic solutions of partial differen-

tial equations in chapter II.

§1. Differential Operators, the Metaplectic and Symplectic Groups

0. Introduction

Historical account.

The metaplectic group was defined by I. Segal [14];

his study was taken up by D. Shale [15]. V C. Buslaev [3, 11] showed

that it made Maslov's theory independent of the choice of coordinates.

A. Well [18] studied it on an arbitrary field in order to extend C. Siegel's

work in number theory.

Summary. We take up the study of the metaplectic group in order to

specify its action on '(R'), .*'(R'), and 9'(R') (see theorem 2) and its

action on differential operators (see theorem 3.1).

1. The Metaplectic Group Mp(1)

Let X be the vector space R' (1 > 1) provided with Lebesgue measure d'x.

Let X * be its dual, and let < p, x> be the value obtained by acting p e X

onxEX.

Spaces of functions and distributions on X.

The Hilbert space. °(X) con-

sists of functions f : X - C satisfying

If (x) I'

d'x1/2

< ao.

Ifi = (tf2dIx)

The Sch

wartz space 9'(X) [13] consists of infinitely differentiable,

rapidly decreasing functions f : X - C. That is, for all pairs of 1-indices

(q, r)

IfI9,r = Sup Ix°(c'xlrf(x)I < 00.

The topology

of\

Y(X) is defined by a countable fundamental system of

I,§1,1

neighborhoods of 0, each depending on a pair of 1-indices (q, r) and a

rational number E > 0 as follows:

4t(q, r, E) = {f I

IfI,,

< E}.

The bounded sets B of .9'(X) are thus all subsets of bounded sets of .'(X)

of the following form:

B({be,.}) = {fI If I,., < ba.rdq,r},

q,rEN',

bq1 re1 +.

The Schwartz space 59''(X) is the dual of Y (X) [13]; its elements are

the tempered distributions: such an element f' is a continuous linear

functional

.9'(X)-,C.

The value of f' on f will be denoted by f

x f'(x) f (x)

d'x, although the

value of f' at x is not in general defined. The bound of f' on a bounded

set B in ,9'(X) is denoted by

If'IB = Sup I

f'(x) f(x) d'xI.

The continuity of f' is equivalent to the condition that f' is bounded:

I f' I e < oc dB. The topology of 59''(X) is defined by a fundamental system

of neighborhoods of 0, each depending on a bounded set B of 99'(X) and

a number e > 0, as follows:

"(B, E) _{ f I

I f' B<_ E}.

Unlike the above, this topology cannot be given by a countable fundamen-

tal system of neighborhoods of zero.

Let us recall the following theorems.. °(X) can be identified with a sub-

space of .So'(X ):

5(X)ca/l'(X)c.9''(X).

The Fourier transform is a continuous automorphism of ,9''(X) whose

restrictions to ,Y(X) and 5o(X) are, respectively, a unitary automorphism

and a continuous automorphism.

Y (X) is dense in Y'(X ).

For the proof of the last theorem, see L. Schwartz [13] : chapter VII, §4,

the commentary on theorem IV, and chapter III, §3, theorem XV; alter-

natively, see chapter VI, §4, theorem IV, theorem XI and its commentary.

Differential operators associated with elements of Z(l) = X (D X*. Let

v be an imaginary number with argument n/2: v/i > 0.

Let a° be a linear function, a°: Z(1) --). R. Let a°(z) = a°(x, p) be its

value at z = x + p [z e Z(1), x e X, p c X*]. The operator

a = a°

(x, l

(

v ox

is a self-adjoint endomorphism of .9"(X ): the adjoint of a, which is an

endomorphism of So(X ), is the restriction of a to So(X ). The operators a

and the functions a° are, respectively, elements of two vector spaces sy and

.sad°. These spaces are both of dimension 21 and are naturally isomorphic:

We say that a is the differential operator associated to a° E .d°. By (1.2),

sl°, which is the dual of Z(1), will be identified with Z(1).

The commutator of a and b e sl is

[a, b] = ab - ba E C;

c c- C denotes the endomorphism of 9"(X):

c : f H c f

df E Y' (X ).

In order to study this commutator, we give Z(l) the symplectic structure

] defined by

[z, z'] = (P, x'> - < P x>,

where z = x + p, z' = x' +p',xand x'cX,and pand p'eX*.

Each function a° e sl° is defined by a unique element a' in Z(l) such that

a°(z) = [a', z].

(1.1)

This gives a natural isomorphism

Z(1) E) a' H a° e

°.

(1.2)

The commutator of a and b c- d is clearly