Leray J. Lagrangian Analysis and Quantum Mechanics: A Mathematical Structure Related to Asymptotic Expansions and the Maslov Index

Подождите немного. Документ загружается.

I,§1,2

Remark 2.2.

we have

Writing A + A' + A" for A(x, x') + A'(x', x") + A"(x", x),

Inertx(A + A' + A") =

A' + A")

= Inert,-.(A + A' + A"). (2.11)

.(A + A' + A") =

A2(A)A2(A')

Hess

(2.12)

x A2(A'*)

Proof of I°).

By (1.11), the relations

(x, P) = SA(x', P'),

(x', p') = p")

may be written

p = Ax(x, x'),

p' = -As (x, x') = A'x,(x', x"),

p" -A'x.,(x' x").

It results from the elimination of p' and x' in these relations that

(x, P) = SASA'(x", p")

The condition (2.7) that SASA' 0 Esp is then equivalent to each of the

following conditions:

The elimination of p' and x' in the preceding step leaves x and x"

independent.

The relation

A, (x, x') + A'x-(x', x") = 0

leaves x and x" independent.

For any x and x", there exists an x' satisfying this relation.

Now in (1.9), det L A 0. Therefore (2.7) is equivalent to (2.8).

Proof of 2°). Assumption (2.9) means that any two of the following

three relations implies the third:

(x, P) = SA(x', P'),

(x', P) = SA,(X", p"),

(x", P") =

Then by (1.11), each of the next three relations implies the other two:

(A+A'+A")x=0, (A+A'+A")x.,=0,

(2.13)

I,§1,2

where

A + A' + A" = A(x, x') + A'(x', x") + A"(x", x).

Now by Euler's

formula, these three relations imply

A+A'+A"=0.

Therefore

(A + A' + A")s, = 0, that is, (A + A')x. = 0, implies A + A' + A", = 0.

Proof of 30).

We have

Hessx.(A + A' + A") = Hess(P' + Q),

which gives the first statement. For the other, the three pairwise equivalent

relations (2.13) can be written

(P+Q")x-`Lx' -L'x"=0,

-Lx + (P' + Q)x' -`L'x" = 0,

`L"x-L'x'+(P"+Q')x"=0.

(210) clearly expresses the equivalence of these three relations.

Proof of Remark 2.2.

By (2.10), the symmctric matrices

P" + Q',

(P' + Q)-1,

P + Q"

can be transformed one into the other. They therefore have the same

inertia. This is (2.11).

By (2.10)3,

Hess(P' + Q)

= (det L) (det L')/(-1)' det L".

By definition 2.2, this is (2.12).

Definition 2.4.

Given

3A, SA SA' SA" = e,

we define

Inert(sA,

5A,, sA.-) = Inertx(A + A' + A")

[see (2.11)].

(2.14)

We define

I,§1,2

Inert(SA, SA,, SA,.) = Inert(sA, SA,, SA,.).

Moreover, we define the Maslov index of SA, m(SA) E Z4, by

m(SA) = m(A) mod 4.

(2.15)

§2,8 will connect this with the index that V. I. Maslov actually introduced.

Lemma 2.1 and (2.15) have these obvious consequences:

Inert(sA,l, sA.', sA 1) = I - Inert(sA, SA., SA..),

(2.16)

m(SA 1) = 1 - m(SA),

m(-SA) = m(SA) + 2 mod 4.

We can at last study compositions of the SA.

LEMMA 2.4.

Consider a triple A, A', A" of elements of A such that

SASA, SA,. = e.

(2.17)

Then

SASA'SA.. = ±E [E is the identity element of Mp(1)].

(2.18)

We have

(2.19)

if and only if

Inert(SA, SA,, SA,.) = m(SA) -

m(SA,.) mod4.

(2.20)

Remark.

Condition (2.17), which is equivalent to (2.18), implies (2.20)

mod 2.

Proof. Let Y E X. Formula (1.10) holds if f' is replaced by the Dirac

measure with support y, given by

6'(x) = 6(x - y).

We obtain

(SA.(>)(x)

(21ri

0(A )eA (s y)

from which follows, by lemmas 2.2 and 2.3,2°),

I,§1,2

(;)u2

(SASAb'x) =

iA(A)A(A'){Hessx.[A(x, x')

+ A'(x',

y)]}-1f2e-,A"(y.x)

Multiplying this by f'(y)d'y, where f' c- Y (X), and integrating, we get

SASA' f' =

A(A(AO(j)

[Hessx,(A + A' +

which gives, by lemma 2.1 and formula (2.12),

SASA.SA = ±E.

Now specify the sign. By definition 2.4,

arg[Hessx.(A + A' + A")] 1j2 = Z Inert(SA, SA., SA..) mod 2n.

By definition 1.2, (2.16), and lemma 2.1,

arg A(A) = 2m(SA),

arg A(A') = 2m(SA,) = 2 [l -

n 1 nargA(A"*)

[l - m(SA..)] mod 2n.

Therefore

arg(± 1) =

[Inert(SA, SA., SA..) - m(SA) + m(SA.1) - M(SA")] mod 271,

which proves the lemma.

Recall that Sp2 (1) denotes the group generated by the SA.

LEMMA 2.5.

Every element of Sp2(1) is a product of two of the SA.

Proof.

By lemma 2.1, every element of Sp2(1) is a product of the SA.

It then suffices to prove that given U, V, W c A, there exist B and C in A

such that

SUSvSW = SBSS.

(2.21)

Now, by lemmas 2.3,1°) and 2.4, for every W e A and every T

a generic

element of A, SWST belongs to {SA} and is generic. Therefore, for T

generic,

SVST E {SA},

SUSVST E {SA},

ST'SW E {SA},

which gives (2.21) with

Sg = SUSVST E {SA}, SC = ST1Sk E {SA}.

The restriction to Sp2(0 of the natural morphism Mp(l) - Sp(1) is

clearly a natural morphism:

SP2(1) - SP(1)-

LEMMA 2.6.

The kernel of this morphism is the subgroup

S° = {E, -E}.

Therefore

Sp2(1)/S° = Sp(1).

Proof. By the preceding lemma, the kernel of this morphism is the

collection of the SASA.(A, A' c- A) such that sASA. = e. From this, by lemma

2.1,

Therefore, by (1.11),

A'(x, x') = A*(x, x')

Vx, x' c- X.

Consequently by definition 1.2.

A(A') = ±1 (A*),

and

SA, _ ±SA.; therefore SASH- = ±E.

LEMMA 2.7.

The group Sp2(1) is connected.

Proof. Given k e Z4 (additive group of integers mod 4), let Dk be the

collection of SA such that

m(A) = k, or equivalently, i-kA(A) > 0.

The collection of quadratic forms A satisfying A2(A) > 0 [or A2(A) < 0]

is connected. Each Dk is thus a connected set in Sp2(1).

Given k e Z4, let SA and SA, be such that

1,§1,2 19

m(SA) - -k mod 4;

p' + Q has one eigenvalue equal to zero and I - 1 eigenvalues > 0.

Let B and B'

be elements of A near A and A' and such that

Hess,,,(B + B')

Inerts,(B + B') takes the values 0 and 1. Since m is locally constant,

M(SB) = m(SA),

m(Sa.l) = m(SA1).

We define B" E A by

E. By (2.20),

takes the values k and

k + 1 in any neighborhood of the element

(SASA.)-1

of Sp2(1). This

element thus belongs to Dk n Dk+1:

pk n Dk+ 1

which gives the lemma.

The above lemmas prove the following theorem. Part 1 of the theorem

reduces the study of Mp(l) to that of Spz(I). Its equivalent can be found in

the work of D. Shale and A. Weil, but the proof we have given has es-

tablished various other results that will be indispensible to us. One of

these is part 3 of the theorem. This will be used in §2,8.

THEOREM 2.

1°) The elements SA of Mp(l) that are defined by (1.10) generate

a subgroup Sp2(l) of Mp(l). Sp2(l) is a covering group (see Steenrod [17],

1.6, 14.1) of the group Sp(l) of order 2. It is a group of automorphisms of

E/(X) that extend to unitary automorphisms of,Y(X) and to automorphisms

Of 99'(X).

2°) The formulas (2.11) and (2.14) define the inertia of every triple s, s', s"

of elements of Sp(l)\Esp such that

ss's" = e [identity element of Sp(l)].

The inertia is

a locally constant function (discontinuous on Esp) with values

in {0, 1,

... ,

1} satisfying

Inert(s, s', s")

= Inert(s", s, s') _

= I -

Inert(s"-1

s'-1, s-1).

Let Esp2 be the hypersurface of Sp2(l) that

is mapped onto Esp in Sp(l)

under the natural projection. The elements SA defined

by (1.10) are the

elements of Sp2(l)\Esp=

. Let S, S', S" be a triple of such elements satisfying

I,§1,2-I,§1,3

SS'S" = E [identity element of Sp2(1)].

Let s, s', s" be the images of these elements under the natural projection onto

Sp(l). We define

Inert(S, S', S") = Inert(s, s', s").

3°) Formula (2.15) and definition 1.2 define the Maslov index m on

Sp2(1)\Y-SD,. It is a locally constant function (discontinuous on 1sP2) with

values in Z4. It satisfies

m(S-') = 1 - m(S),

m(-S) = m(S) + 2 mod4,

Inert(S, S', S") = m(S) - m(S'-1) + m(S") mod 4.

Remark 2.3.

We shall see later that m is characterized by the last formula

and the property of being locally constant.

Remark 2.4.

Sp2(1) contains the three subgroups of G(l) defined in section

1 by (i) Fourier transformation, (ii) quadratic forms, and (iii) automor-

phisms of X.

Proof. Let S be an element of one of the three subgroups. It is easy to

find A E A such that

SSA = S,,.,

where A' E A.

Remark 2.5.

It can be shown that every S E Sp2(!) is of the form

S = S1S2S3S4,

where S3 E (i),

that

is,

is a Fourier transformation in at most

I coordinates; S, and S4 E (ii), that is, they are of the form f' i-+ e"4f',

where Q is a real quadratic form; and S2 E (iii), that is, S2 has the form

f' i--

det T f' o T, where T is an automorphism of X.

3. Differential Operators with Polynomial Coefficients

By definition 1.1, the elements of Sp2(1) transform differential operators

with polynomial coefficients into operators of the same type. Section 3

describes this transformation more explicitly.

Let a+ and a- be two polynomials in 1/v, x, and p:

a+(v,

x, p) _

a. (v, x)pa,

(v, p, x) _ >paaa (v, x)

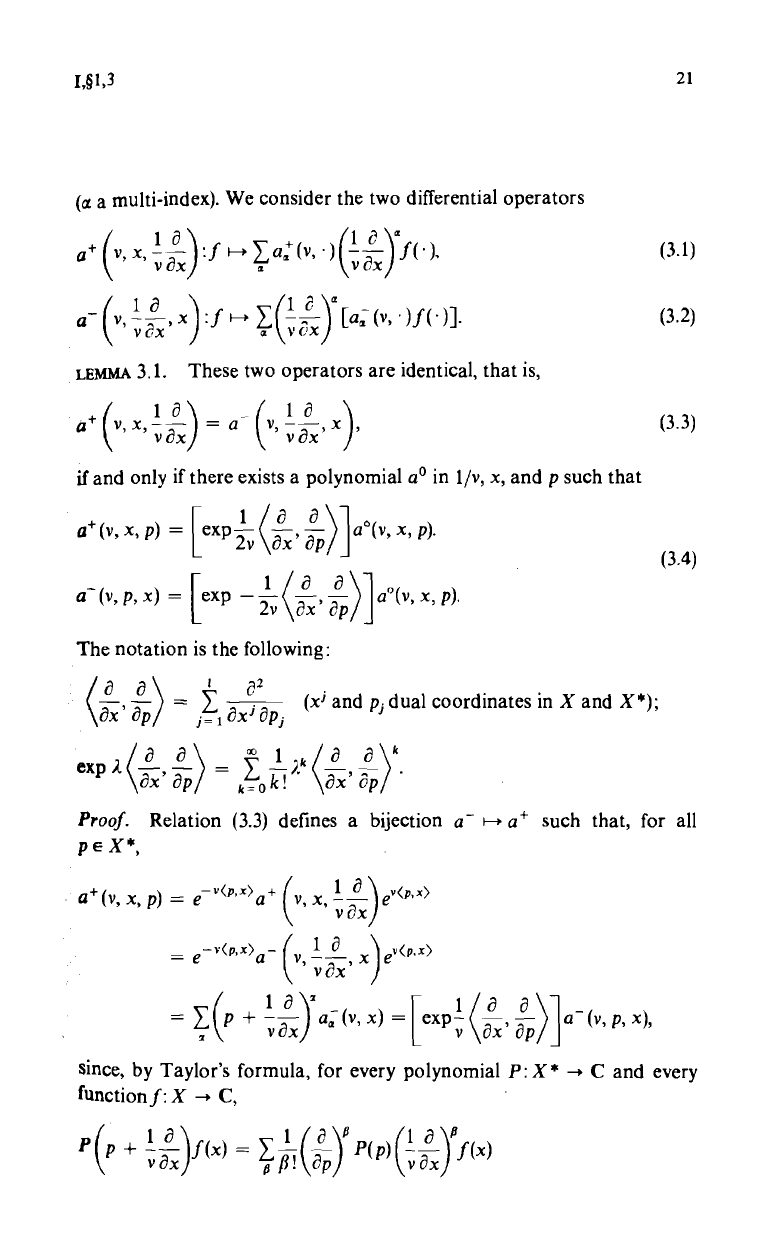
I,§1,3

(a a multi-index). We consider the two differential operators

(v, x,

v a x

) :f a, (v'

v e xx)af( )'

(v,

, x

: f

vex

[aa (v, .)f( )]-

LEMMA 3.1.

These two operators are identical, that is,

)

= a

a 1

,vax

'vax,

if and only if there exists a polynomial a° in 1/v, x, and p such that

a (v, x, P) _

[exp 1

aax,

/] a '(v, x, p)-

_ 1 a a

a (v, p, x)

exp - - -, -

a°(v, x, p).

[ 2v

ax ap

The notation is the following:

(xi and pi dual coordinates in X and X*);

ax api

°° 1

k/ a

a k

exp

ax, ap/ =

k=°k!ax,

op/

Proof.

Relation (3.3)

defines

a bijection a- i-- a+ such that, for all

pEX*,

a+(v, x, p) = e

v<P,x>a+

l a )Y<P,x>

V ax

v(P,x>a-

(v'

1 a

x) ev<P,x>

vex

- 1a1

\Ox, a

p )]a (v, p, x),

P + vaxJ aQ (v, x)

-[eXp

since, by Taylor's formula, for every polynomial P:

C and every

function f : X

- C,

P (p

+ v

ax)f (x)

Y P.

P(P) (vex) f (x)

I,§1,3

[exp!(.

ap)] [P(P)f (x)]

The bijection a- r-+ a+ can then be defined by the relation

_ 1

a a\

(v, x, p)

exp-

)]a-(v, p, x)

[ v

ax' ap

This is what the lemma asserts.

Definition 3.1. Let a be a differential operator that can be expressed as

in (3.1) and (3.2). It is defined by the polynomial a° in (1/v, x, p) that

satisfies (3.4). We say that a is the differential operator associated to the

polynomial a°.

Theorem 3.1 will describe the transform SaS-1 of a by Sc Sp2(1);

Lemma 1.1 has already dealt with the case in which a° is linear in (x, p).

The proof of this theorem will use the following properties.

LEMMA 3.2.

If a and b are the operators associated to the polynomials

a° and b°, then the operator

c=ab

is associated to the polynomial co, where

c°(v, x, p) _ }[exp 2v \ay' ap)

2v Cax' aqM

[a°(v, x, P)b°(v, y, q)]

Y='-

(3.5)

e-n

Proof.

If b°(v, x, p) only depends on p, then the polynomial co associated

to c = ab is

c°(v, x, P) _

[exp

- 2v

ax' ap

)][a4

(v, x, P)b°(P)]

{[exp

(ax

aq)]

[a+(v, x,

P)b°(q)]}

9-P

_ 1

{[exp

2v ax

ap)]

[a°(v, x,

P)b°(q)}q=r

Similarly, if h°(v, x, p) only depends on x, then the polynomial associated

to c = ab is

I,§1,3

c°(v, x, P) =

{[ex-(-

, a

)]

[a°(v, x,

P)b°(Y)]Lx

Thus if b+(x,p) =

b'(x)b"(p), then the polynomial associated to c = ab is

c°(v, x, P) =

[exp

- 2v

aq)]

{Lexp

(P' a-)]

[a°(v, x, p)b'(y)]

1)y=x

{[exp

ax' aq)

2v (ay aq)

+ 2v

(ay' P)]

[a°(v,

}y_}-

4=p

This is (3.5) since, by (3.4),

[exp

2v (ax' 4)] [b

b°(Y, q)

This implies lemma 3.2, which has the following obvious consequence:

LEMMA 3.3. The operator

c = 2(ab + ba)

is associated to the polynomial

1 o a

x, p) _ cosh

[2V(8Y'2

aq)]

y=z

[a°(v, x, P)b°(v, y,

q)]lq=p

If b is linear in (y, q), then

Oy, ap -

(,)]2[ao(v,x,p)bo(v,y,q)]

= 0,

from which follows

cosh[

] a°b° = a°b°

therefore we have the following lemma.

LEMMA 3.4.

If b is linear in (x, p), then the operator associated to a° b° is

(ab + ba).