Leray J. Lagrangian Analysis and Quantum Mechanics: A Mathematical Structure Related to Asymptotic Expansions and the Maslov Index

Подождите немного. Документ загружается.

174

III,§1,2-III,§1,3

_ dR

_ d'Y

_ d) _ _ dQ

dL = dM = 0

Hp(x, p) RHQ[L, M, Q, R] HL HM

RHR'

and thus (2.14), by (2.6) and (2.12).

Proof of 4°). Formula (1.17) can be written

d 3x n d3 p= dL n dM n dH n

n d(l) n d'Y,

RHQ

where, for H = 0,

= dt mod(dL, dM)

RHQ

by (2.14); hence (2.15) holds, the following meaning being given to its

left member:

d3x A dap

denotes the restriction ww to W of any form w of degree 5 such that

dH A m = d 3 x A d 3 p ; ww is clearly independent of the choice of w.

3. The Quantized Tori T(1, m, n) Characterizing Solutions, Defined

mod(1/v) on Compact Manifolds, of the Lagrangian System

1 .1)

aU=(aL=-LoU=(aM-Mo)U=O mod

(3.1)

a, aL2, and ay denote the lagrangian operators associated, respectively, to

the hamiltonians

H, L2, M,

which are in involution; Lo and MO are two real constants such that

IM0I < Lo.

From theorem 7.1 of II,§3, solutions of this system are lagrangianfunc-

tions U with constant lagrangian amplitude, defined mod(1%v) on those

manifolds V[L0, M0] defined by (2.4), whether compact or not, that

satisfy Maslov's quantum condition (II,§3, definition 6.2). V[L0, M0] is

chosen to be connected. The measure nv on V, which is invariant under

the characteristic vectors of H, L2 - Lo, and M - M0, and which serves

to define the lagrangian amplitude, is

III,§1,3

175

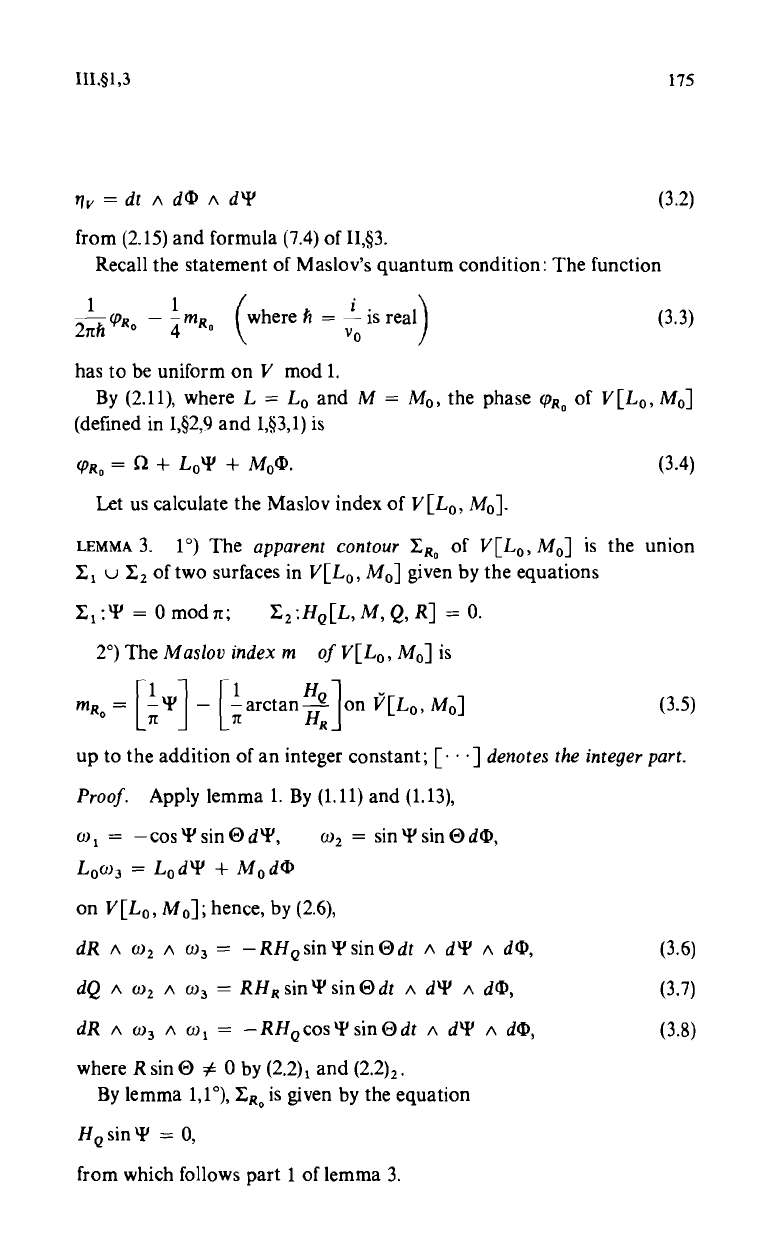
nv = dt A d' A dW (3.2)

from (2.15) and formula (7.4) of II,§3.

Recall the statement of Maslov's quantum condition: The function

Z h

cOR° - 1

MRo

(whereh =

is real

has to be uniform on V mod 1.

By (2.11), where L = Lo and M = M0, the phase cpR, of V [L0, M0]

(defined in I,§2,9 and I,§3,1) is

(pR0 = 0 + LOT + MO(D.

(3.4)

Let us calculate the Maslov index of V [L0, M0].

LEMMA 3.

1°) The apparent contour ER0 of V[L0, M0] is the union

E, u E2 of two surfaces in V[L0, M0] given by the equations

E, :'Y = 0 mod 7r;

E2 :HQ[L, M, Q, R] = 0.

2°) The Maslov index m of V[L0, M0] is

[i-p]

mR° _

- [V [L0, M0]

(3.5)

up to the addition of an integer constant; [ ] denotes the integer part.

Proof.

Apply lemma 1. By (1.11) and (1.13),

0)1 = - cos'I' sin O dW, 0w2 = sin W sin O dcb,

L00)3 = L0 d'I' + M0 da)

on V[L0i M0]; hence, by (2.6),

dR A w2 A 0)3 = -RHQsin'I'sin0dt A d'I' A dD, (3.6)

dQ A 032 A W3 = RHR sin W sin O dt A d'I' A d(D,

(3.7)

dR A w3 A 0), = -RHQcos'I'sinOdt A d'! A dcb,

(3.8)

where R sin O : 0 by (2.2), and (2.2)2 .

By lemma 1,1°), ER° is given by the equation

HQ sinW = 0,

from which follows part 1 of lemma 3.

176

III,§1,3

In a neighborhood of a point of E1\E1 U E2, HQ cos `P # 0; in lemma

1,2°), we can choose

w = dR n w3 n w1;

dR n W2 n w3/w = tan `P,

whence by this lemma

mRo =

[i: `Pl

+ const.

By (2.2)3, in a neighborhood of a point of E2\E1 U E2' HR sin `P

in lemma 1,2°), we can choose

w = dQ A wz A 0)3;

dR A 0)2 A m3/m = - HQ/HR,

where

MR,, = const. - C1 arctan

]. (3.10)

The global expression for mRo follows from the two local expressions (3.9)

and (3.10).

By (3.4) and (3.5), Maslov's quantum condition may be formulated as

follows: The function

1 arctan

Lo _ 1

Mo do

h 2n 4n

2 27c

it 2n

is defined mod 1 on V[L0, Mo].

If V[L0, Mo] is compact, that is, if the curve F[L0, Mo] is a closed

curve, by (2.9) this condition is that

IN[Lo, M]

+ 1,

LO -

2 2 h°

be three integers. Denote them by

n-1, 1, m

in order to recover the classical notation of quantum physics. Since N >

and IMOI < Lo, we have

Iml<1<n.

If V[L0, Mo] is not compact, Maslov's quantum condition is that

III,§1,3

177

Lo-2=1,

1MO=m

be two integers such that Iml _< 1.

Thus the conclusion of this section is the following theorem.

THEOREM 3.

The connected manifolds on which the solutions of the lagran-

gian system (3.1) are defined are

1°) The compact manifolds V [L,, M0] defined by (2.4) such that there

exist three integers

1, in,

satisfying the conditions

Imll<n,

(3.11)

Lo = h(1 + z),

Mo = hm,

Lo + N[LO, M0] = hn;

(3.12)

thus V[L0, M0] is a torus, which will be denoted by T(1, m, n); the co-

ordinates at a point of this torus are

t mod c [L 0, M0] defined by (2.6)-(2.7),

`P mod 27t,

b mod 2n.

(3.13)

2°) The noncompact manifolds Y [L,, M0] defined by (2.4) such that

there exist two integers

1, m

satisfying the conditions

Imi < 1,

Lo=h(l+z), Mo=hm;

(3.14)

(3.15)

thus V[L0, M0] is a product of

a 2-dimensional torus with the coordinates `P mod 2n, D mod 2n,

a line, half-line, or segment with coordinate t.

We provide V [Lo, M0], whether compact or noncompact, with the follow-

ing measure, which is invariant under the characteristic vectors of H,

L2 - L0,and M - Mo:

tlv = dt n d(D A d`Y;

(3.16)

178

111,§1,3

then the solutions of (3.1) defined on V[L°, M°] are lagrangian functions

with constant lagrangian amplitude.

Remark 3.1. From now on, we shall limit ourselves to the study of com-

pact lagrangian manifolds (for example, that of the electron belonging to

an atom).

Remark 3.2.

The characteristic vectors of LZ - Lo and M - M° are

KL2_L0:dL=dM=dt=0,

d'h=2L°,

d(D = 0,

(3.17)

1M_MU:dL = dM = dt = 0, d'P = 0, d(D = 1, (3.18)

respectively. It is immediately verified that these vectors leave q, invariant.

Proof of (3.17).

It follows from (1.2),, then (1.5), and (1.6), and finally

(1.5), and (1.12) that KL2_LQ is the vector tangent to V[L°, M°] such that

dx = 2LLP = 2(R2p - Qx) = 2LRJZ =

2LOx(L,

M, t, T, (D)

This proves (3.17).

Proof of (3.18).

It follows from (1.1), then (1.5), and (1.12), that Km-m, is

the vector tangent to V[L°, M°] such that

dx =

(-x2, x1, 0) =

8x(L, M, t,'P, (D)

O(D

Remark 3.3.

In agreement with theorem 7.1,2°) of II,§3, when the mani-

fold V[L°i M°] is compact, hence a torus, the characteristic vector of H

[see (2.14)],

x:dL=dM=0,

dt = 1, dP=HL,

d'P=HM,

(3.19)

and those of L2 - Lo and M - M° generate

V[L°, M°], namely, the translations in

(2.7) and 111,§2,(1.5) and 111,§2,(2.3)]:

t modc[L°, M°],

'P + A[L0, M0, t] -

NL[L°,

M t

c[L0, M0]

a group of translations of

the following

mod 2n,

coordinates [see

:p + p - NM t mod 2ir.

111,§ 1,4

179

4. Examples: The Schrodinger

Let us choose

- K[R') = - M

And Klein-Gordon Operators

[

(x, p

where K: R+ Q+ R --+ R is a given function. In other words,

- K[R

M M]]

L R

[L2 +

[

, Q, , .

, ]

Theorem 3 can be applied immediately.

The condition that (2.4) define at least one compact hypersurface

V [LO, M0], which is a torus, is that the function

L2eR

be positive between two consecutive zeros R1 and R2 (0 < R1 < R2).

Then (2.9) defines

N[L,, M0] =

/K[R M0] - Lo

R R

Remark 4.1.

if K is an affine function of M, then, by (4.1),

7x'

P(._)H(xP)

= 0,

and consequently [II,§1,definition 6.2 and II,§1,(6.3)], the expression in

Ro of the operator associated to H is

2vzA 2R2K[R'v(xlax

x2ax )J,

where

A = 1:3

J=' a2iax;,

the multiplication by any function of R commutes with the operator

1(XI a

Cxz

- Xz

ax,

Example 4.

We call the operator associated to the hamiltonian

180

III,§1,4

H(x

p)=2 [P2 +A(M)-2B M-+C M)1

where A, B, and C:R -+ R are some given functions, the Schrodinger-

Klein-Gordon operator. Let

AO = A(Mo),

Bo = B(Mo), Co = C(Mo).

The condition that there exist at least one compact manifold V[LO, Mo]

defined by (2.4) may be expressed as follows:

Ao>0, L2O+Co>0, Bo>-,/A0V/L+C (4.7)

When it is satisfied, V [Lo, Mo] is unique, and by (4.3),

N [Lo, Mo] =

- AOR,

+ 2Bo R - Lo - Co R ,

where the integral is calculated along a cycle of C enclosing the cut

JR1 i R21; taking residues at R = oo and R = 0 gives

N[Lo, Mo] =

BO - v[L:o + Co > 0.

v' Ao

The statement of theorem 3 becomes the following.

THEOREM 4.1. Let a be the Schrodinger-Klein-Gordon operator, that is,

the operator associated to the hamiltonian (4.6). Let aLl and am be the

operators associated to the hamiltonians L2 and M. Then the lagrangian

system

aU = (aL: - Lo) U = (am - MO)U = 0 mod

has solutions defined on a compact manifold if and only if there exists a

triple of integers (l, m, n) such that

Lo=h(l+Z), Mo=hm,

(4.10)

Iml

l < n,

(I + )z +

Coh-z

> 0,

11)

AO =Boh-z[n-l-I+

(l+1)2+Coh-z] z

If this condition is satisfied, this compact manifold is unique: it is the

torus T(l, m, n) given by the equations

III,§1,4

181

T(l, m, n): H(x, p) = L(x, p) - Lo = M(x, p) - Mo = 0.

(4.12)

We provide this torus with the invariant measure (3.16); then the solutions of

(4.9) defined mod(l/v) on T(l, m, n) are the lagrangianfunctions with constant

lagrangian amplitude.

Notation.

(Related to physics and used only at the end of this section)

In E3, we choose an electric potential sago : E3 -+ R and a magnetic

potential vector ('41, d2, a3): E3 - E3 satisfying the physical law

= 0; (4.13)

i=i

axi

they are time independent.

The trajectories in E3 of the relativistic or nonrelativistic electron of

mass µ and electric charge -F < 0 are the solutions of Hamilton's

system, defined by the hamiltonian given by

H(x,p) = 1 CPi

sii(z)12-E

- Fsdo(x) (4.14)

2µi=1

in the nonrelativistic case, and by

2 + iµc2

(4.15)

H(x, p) =

2µ iY-i

[pj

sii(z)12

- 2µc2 [E

in the relativistic case. Here

c is the speed of light,

E is a constant, which is the energy of the electron whose position x and

momentum p satisfies H(x, p) = 0 (energy including the rest mass µc2 in

the relativistic case).

a'x,

By (4.113)

ap) H(x, p) = 0.

Then

by//

I1,§1,(6.3) and II,§l,definition 6.2, in the nonrelativistic case the

operator associated to H is the Schrodinger operator

a =

2µ

v2 0

+ ce

Ed'(x)

ax j

(x)1

-E

- Fdo(x),

(4.16)

182

III,§1,4

and in the relativistic case it is the Klein-Gordon operator

a = 2Lyz + 2z

I.i

E2 I d2]

2-t- [E + Ed0]2 +

µ2z

(4.17)

Let us choose these hamiltonians and operators as follows: d0 is the

electric potential of a nucleus with atomic number Z, placed at the origin;

the magnetic field is constant, parallel to the third coordinate axis, and

of intensity .*

. In other words,

SIo(W ) = R ,

-Zrxz,

z(x) = Z.

x1,

S13 = 0.

(4.18)

Let us neglect the

Z terms, as is done in physics; the hamiltonians

(4.14) and (4.15) of the electron become hamiltonians of the form (4.6)

H(x, p) = Z

[P2

+ c .M - 2µE -

2µR2

(4.19)

in the nonrelativistic case, and

' I

P2+E M+ µz

Ez_2e2ZE-e4Zz

(

' P)

2µ

c2R c2R2

(4.20)

in the relativistic case.

The Schrodinger operator becomes

µz

a=2µ[v A+E (xla3Z-xzOX

-2µE-2RZ(4.21)

the Klein-Gordon operator becomes

1 e

Ez 2e2ZE

e4Zz

a =

2µ vz

+ c xl

aX2

8x1

+ µzcz -

- czR - czRzl.

(4.22)

Relation (4.11)3 defines E as a function of (l, m, n); in the course of this

calculation, two constants, which are classical in physics, appear.

137,

the dimensionless fine-structure constant,

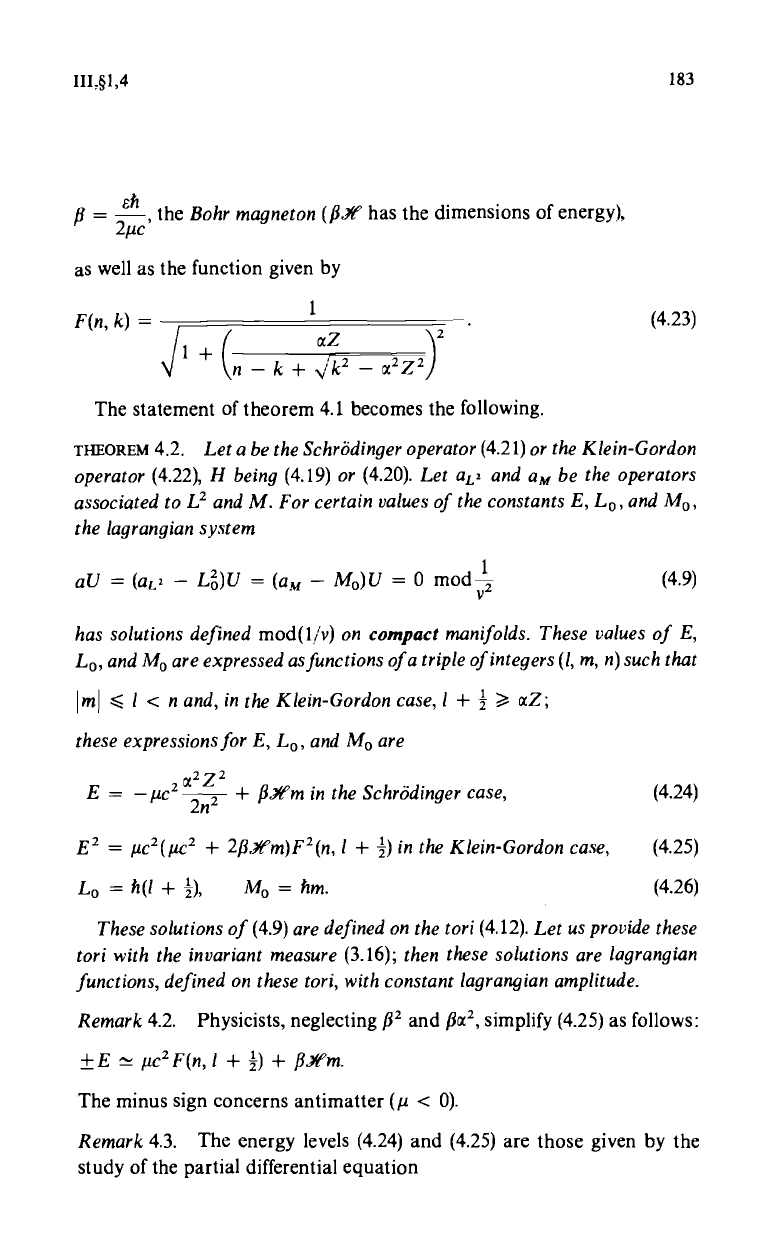
III7§1,4

183

p =

the Bohr magneton (j3.)(° has the dimensions of energy),

as well as the function given by

(4.23)

F(n, k) =

1 +

The statement of theorem 4.1 becomes the following.

THEOREM 4.2.

Let a be the Schrodinger operator (4.21) or the Klein-Gordon

operator (4.22), H being (4.19) or (4.20). Let aL= and aM be the operators

associated to L2 and M. For certain values of the constants E, Lo, and M0,

the lagrangian system

aU = (aL2 - Lo )U = (am - Mo)U = 0 mod z

(4.9)

has solutions defined mod(1/v) on compact manifolds. These values of E,

Lo, and MO are expressed as functions of a triple of integers (1, m, n) such that

ml < I < n and, in the Klein-Gordon case, l + z 3 aZ;

these expressions for E, Lo, and MO are

E _

-ucZ azZz

2n2 +

j3.*'m in the Schrodinger case, (4.24)

E2 = uc2(uc2 + 2f3.°m)F2(n, l + Z) in the Klein-Gordon case, (4.25)

Lo = h(l + Z),

Ma = hm.

(4.26)

These solutions of (4.9) are defined on the tori (4.12). Let us provide these

tori with the invariant measure (3.16); then these solutions are lagrangian

functions, defined on these tori, with constant lagrangian amplitude.

Remark 4.2.

Physicists, neglecting j32 and j3a2, simplify (4.25) as follows:

±E

uc2F(n, I + Z) + f.#°m.

The minus sign concerns antimatter (u < 0).

Remark 4.3.

The energy levels (4.24) and (4.25) are those given by the

study of the partial differential equation