Higgins R.A. Engineering Metallurgy: Applied Physical Metallurgy

Подождите немного. Документ загружается.

electrons. A light wave striking the surface of a metal causes a free electron

to vibrate and absorb all the energy of the wave, thus stopping trans-

mission. The vibrating electron then re-emits the wave from the metal

surface giving rise to what we term 'reflection'.

The very important property of most metals in being able to undergo

considerable plastic deformation is also due to the existence of the metallic

bond. Under the action of shearing forces, layers of positive ions can be

made to slide over each other without drastically altering their relationship

with the shared electron cloud.

Secondary Bonding Forces

1.80 Stable atoms—and molecules—always contain equal numbers of

protons and electrons. Consequently they will be electrically neutral and

carry no resultant charge. Yet they must attract each other for how else

can we explain the fact that all gases condense to form liquids and that all

liquids either crystallise to form solids or else become so viscous that the

strong forces of

attraction

between molecules make them behave almost

like solids?

In short, whilst we have related the cohesion between metallic particles

to the metallic bond we have not yet attempted to explain the forces which

hold together covalently bonded molecules, or, for that matter, the single

atoms in noble gases which, although they contain no valence electrons,

must attract each other in some way since they ultimately liquify and

solidify at very low temperatures. These weak secondary bonding forces

are often referred to as van der Waals' forces since it was this Dutch

physicist who first explained the deviations in the Gas Law (PV = RT) as

being partly due to forces of attraction between molecules (or atoms in the

case of noble gases) within the gas.

Consider two atoms of a noble gas such as argon. If these two atoms are

in close proximity and their electrons happen to be concentrated as in Fig.

1.9, it is reasonable to suppose that mutual attraction will occur between

the positively charged nucleus of atom X and the negatively charged elec-

trons of atom Y at the moment when the nucleus of X is 'unshielded'

by its own electrons. This situation will be continually changing as the

distribution of electrons alters, but a resultant weak force of attraction

exists.

Engineers will be familiar with the idea of 'centre of mass' in a solid

body. In a similar way we can imagine electrical charges 'resolved' to give

'centres' of positive and negative charges respectively in a molecule. If

Fig.

1.9.

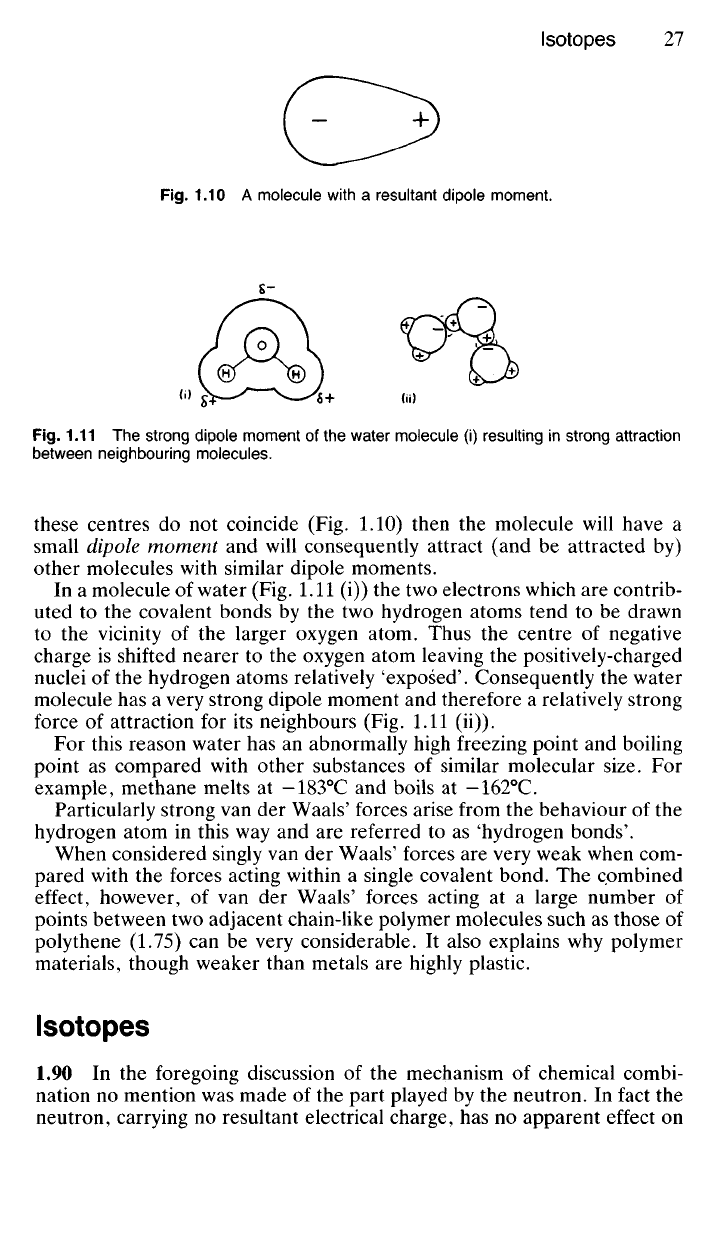
Fig.

1.11 The strong dipole moment of the water molecule (i) resulting in strong attraction

between neighbouring molecules.

these centres do not coincide (Fig. 1.10) then the molecule will have a

small dipole moment and will consequently attract (and be attracted by)

other molecules with similar dipole moments.

In a molecule of water (Fig. 1.11 (i)) the two electrons which are contrib-

uted to the covalent bonds by the two hydrogen atoms tend to be drawn

to the vicinity of the larger oxygen atom. Thus the centre of negative

charge is shifted nearer to the oxygen atom leaving the positively-charged

nuclei of the hydrogen atoms relatively 'exposed'. Consequently the water

molecule has a very strong dipole moment and therefore a relatively strong

force of attraction for its neighbours (Fig. 1.11 (ii)).

For this reason water has an abnormally high freezing point and boiling

point as compared with other substances of similar molecular size. For

example, methane melts at

—

183°C and boils at

—

162°C.

Particularly strong van der Waals' forces arise from the behaviour of the

hydrogen atom in this way and are referred to as 'hydrogen bonds'.

When considered singly van der Waals' forces are very weak when com-

pared with the forces acting within a single covalent bond. The combined

effect, however, of van der Waals' forces acting at a large number of

points between two adjacent chain-like polymer molecules such as those of

polythene (1.75) can be very considerable. It also explains why polymer

materials, though weaker than metals are highly plastic.

Isotopes

1.90 In the foregoing discussion of the mechanism of chemical combi-

nation no mention was made of the part played by the neutron. In fact the

neutron, carrying no resultant electrical charge, has no apparent effect on

Fig.

1.10 A molecule with a resultant dipole moment.

(i)

(ii)

ordinary chemical properties which are mainly a function of the electron

structure of the atom. The principal role of the neutron is to increase the

actual mass of an atom. Thus, the sodium atom with 12 neutrons in the

nucleus, in addition to the 11 protons already mentioned, has a total

nuclear mass of 23. The 11 electrons present are negligible in mass when

compared with the massive protons and neutrons, so that the mass of the

total atom is approximately 23 units, and it is from this value that the

relative atomic mass is derived.

There are many instances, however, in which two atoms contain the

same number of protons but unequal numbers of neutrons. Clearly, since

they have equal numbers of protons, they will also have equal numbers of

electrons and, chemically, such atoms will be identical. Differing numbers

of neutrons, however, in respective atoms will cause these atoms to have

unequal masses. An element possessing atoms which are chemically identi-

cal but which are of different mass is said to be isotopic and the different

groups are known as isotopes.

1.91 Two such isotopes occur in the element chlorine. The chemical

properties of these isotopes are identical because in each case an atom will

contain 17 protons and 17 electrons. Only the relative masses of each atom

will be different, since the nucleus of isotope II contains two more neutrons

than that of isotope I (Fig.

1.12).

Since there are about three times as

many atoms of isotope I (usually written

Cl) as of isotope II (written

Cl) the relative atomic mass of chlorine 'averages out' at 35.45.

Fig.

1.12 The

particle 'make-up'

of the two

isotopes

chlorine.

1.92

There are three isotopes of hydrogen of atomic masses one, two

and three respectively (Fig.

1.13).

The 'ordinary' hydrogen atom (now

called 'protium') contains a single proton in its nucleus—and no neutrons.

Its atomic mass is therefore one. However, approximately one hydrogen

atom in every 6900 also contains a neutron in its nucleus and since the

proton and neutron are roughly equal in mass then the atomic mass of the

ISOTOPE

17 PROTONS

18 NEUTRONS

17 PROTONS

2O NEUTRONS

NUCLEUS

17 PROTONS

M^NEUTRONS

35_

..TOTAL WEICHT

ELECTRONS

NUCLEUS

17 PROTONS

2Q NEUTRONS

TOTAL WEIGHT

Fig.

1.13 The three isotopes of hydrogen.

Each isotope has the same electron/proton make-up so that the simple chemical properties

of all three will be similar.

atom will be two. This atom, often known as 'heavy hydrogen', fH, is also

given the special name deuterium and is the basis of the 'hydrogen bon b

reaction'. A third, radioactive, isotope of hydrogen, called tritium, contai s

two neutrons along with the proton in the nucleus and so has an atomic

mass of three, ie iH. It must be emphasised that deuterium and tritium

are not different elements from hydrogen but only isotopes of that element.

It is perhaps fortunate that separate isotopes of other elements are not

given special names in this manner or confusion would be rife. The term

'isotope' tends to be associated in modern technology with the release of

nuclear energy by suitable elements. The properties of the isotopes of

uranium in this connection are dealt with later (18.75).

Exercises

If the valence of aluminium is three, write down the chemical formula of its

oxide (1.31)

What mass of metallic copper would be deposited by the electrolysis of 1Og of

copper sulphate (CUSO4) in water solution? (1.33)

Calculate the mass of iron obtained by reducing 10 tonnes of the ore hematite,

assuming that hematite as mined contains 70% of the oxide Fe2O3. (1.33)

Why must aluminium be obtained from its ore by electrolysis instead of by the

more usual process of reduction by carbon? (1.44)

In the chemical reaction: Fe3O

+ C = 3FeO + CO, has the Fe3O4 been

oxidised or reduced? (1.44)

6. Complete (and balance) the chemical equation:

HNO

+ MgO = ? (1.51)

Nitric Magnesium

acid oxide

The melting points of Li, Na, K and Rb are 179, 97, 62 and 39°C respectively.

Estimate graphically the melting point of Cs. (1.69 and Fig. 1.2)

8. Without reference to any tables sketch (i) the electron structure of the element

which has an atomic number of 14 (1.67); (ii) the electron structure of the

magnesium ion. (1.71)

9. Show how far modern theory is successful in explaining not only many of the

electron

proton

neutron

TritiumDeuterium

Ordinary

Hydrogen

mechanical properties of a metal but also that it is a conductor of electricity.

(1.76).

10.

Explain why water has abnormally high freezing point and boiling point as

compared with substances of similar molecular mass such as ammonia. (1.80)

11.

The metal copper (relative atomic mass—63.55) exists as two isotopes. 69.2%

by mass of the metal consists of the isotope with a mass number 63. What is

the likely mass number of the other isotope? (1.91)

Bibliography

Brown, G. L, A New Guide to Modern Valency Theory, Longman, 1971.

Cooper, D. G., Chemical Periodicity, John Murray, 1974.

Companion, A. L., Chemical Bonding, McGraw-Hill, 1979.

Cox, P. A., The Elements, their

origin,

abundance and

distribution,

Oxford Science

Publications, 1989.

Hume-Rothery, W. and Coles, B. R., Atomic Theory for Students of Metallurgy,

Institute of Metals, 1969.

Underwood, D. M. and Webster, D. E., Chemistry, Edward Arnold, 1985.

Wilson, J. G. and Newall, A. B., General and Inorganic Chemistry, Cambridge

University Press, 1971.

The Physical

and

Mechanical Properties

Metals

and

Alloys

2.10 Of well over one hundred elements—if we include the increasing

number of man-made ones—only eighteen have definite non-metallic

properties. Six are usually classed as 'metalloids'—elements like silicon,

germanium and arsenic—in which physical and chemical properties are

generally intermediate between those of metals and non-metals, but the

remainder have clearly defined metallic properties. Metals are generally

characterised by their lustrous, opaque appearance and, in respect of other

physical properties, metals and non-metals contrast strongly.

As we have seen (1.76) a metal consists of an orderly array of ions

surrounded by and held together by a cloud of electrons. This is reflected

in many of the physical properties of metals.

2.11 Melting point All metals (except mercury) are solids at ambient

temperatures and have relatively high melting points (see Table 1.1) which

vary between 234K (-39

C) for mercury and 3683K (3410

C) for tungsten.

Non-metals include gases, a liquid (bromine) and solids. Their melting

points vary much more widely: between IK (-272

C) for helium and

approximately 5300K (5000

C) for carbon.

2.12 Density The relative density (formerly specific gravity) of a material

is defined as

the weight of a given volume of the material

the weight of an equal volume of water.

Metals generally have higher relative densities (Table 1.1) than non-

metals. Values vary between lithium (0.534) which will float in water and

osmium (22.5) which is almost twice the density of lead, which suggests

that the simile 'as heavy as lead' needs revision.

2.13 Electrical conductivity Non-metals are generally very poor con-

ductors of electricity, indeed those where the bonding is entirely covalent

will be insulators since all valance electrons are held captive in individual

bonds and can move only in restricted orbits. By comparison in metals

electrical conductivity arises from the presence of a sea or cloud of mobile

electrons permeating the static array of ions. The electrons are able to flow

through the ion framework when a potential difference is applied across

the ends of the metal—which may be many miles apart as in the electric

grid system. As indicated in Table 2.1 the electrical and thermal conduc-

tivities of metals follow roughly the same order. This is to be expected

since both the flow of electricity and heat depend upon the ability of

electrons to move freely within the metallic structure. For purposes of

simple comparison Table 2.1 relates electrical and thermal conductivities

of some important metals to those of silver (100). Although silver is mar-

ginally superior in terms of electrical conductivity to copper, the latter is

used industrially because of relative costs. In fact for power transmission

through the national grid aluminium lines are generally used for reasons

given later (17.13). Electrical conductivity is reduced by alloying and the

presence of impurities (16.21) as well as by mechanical straining.

Table 2.1 Relative electrical and thermal conductivities of some metals

Metal Relative electrical conductivity Relative thermal conductivity

Silver 100 100

Copper 96 94

Gold 69.5 70

Aluminium 59 57

Magnesium 41 40

Beryllium 40 40

Tungsten 29 39

Zinc 27 26.5

Cadmium 22 22

Nickel (23) 21

Iron 16 17

Platinum 15 17

Tin 12.5 15.5

Lead 7.7 8.2

Titanium 2.9 4.1

Mercury 1.6 2.2

Electrical conductivity is measured in units Sm"

, where the unit of

conductance the Siemen (S), is equivalent to Q "

. Generally it is more

convenient to consider the electrical resistivity (Q) of a material which is

the inverse of its conductance and is of course measured in flm. Resistivity

varies with temperature and over a limited temperature range a linear

relationship of the form:

= R(I + Qt)

holds good. Here R

is the resistance at the upper temperature, R the

initial resistance and t the increase in temperature. Q is the temperature

coefficient of resistance of the material.

2.14 Thermal conductivity This arises

in a

similar way

electrical

con-

ductivity. Electrons pick

kinetic energy from

the

increased vibrations

the

ions where

the

metal

is hot.

They pass rapidly through

the ion

framework where they collide with distant ions, causing them

turn

vibrate more rapidly.

this manner electrons behave

transporters

energy.

Metals

are

very good conductors

heat whereas most non-metals

are

not.

The

flow

heat

in a

conductor

governed

by:

Q=-X

where

Q is the

heat flow across unit area,

X is the

coefficient

thermal

ri T

conductivity

and T

the temperature.

—

will

be the

'temperature gradient'

at that unit area. Thermal conductivity

measured

units, Wm

-1

2.15 Specific heat capacity

The

specific heat capacity

, C

) of a

substance

is the

quantity

heat required

raise

the

temperature

of lkg

the

substance

IK. The

units

are

JlCg

-1

The

specific heat capacities

of metals

are low

compared with those

non-metals

that

it is

less

expensive

raise their temperatures.

Dulong

and

Petit's

Law

states that

for all

elements

the

product

of the

specific heat capacity

and the

atomic weight

approximately constant

and

this product

called

the

Atomic heat. This

law was

used more than

century

ago to

assess

the

atomic weights

many (then)

new

elements.

involves

the

relationship between heat capacity

and the

vibrational energy

of atoms.

Table

2.2

Thermal properties

some important metals

Metal Coefficient

thermal expansion

(^)

Specific heat capacity

(K"

x 10"

) (J

kg"

)

Aluminium

23 913

Copper

17 385

Gold

14 132

Iron

12 480

Lead

29 126

Magnesium

25 1034

Nickel

13 460

Silver

19 235

Tin

23 226

Titanium

9 523

Zinc

31 385

2.16 Thermal expansion

materials

are

heated

the

amplitude

atomic vibrations increases

and

this

evident

as an

increase

volume.

The coefficient

cubic expansion

(y) is the

increase

volume

per

unit

This is basically similar to Ohm's Law governing the flow of electricity (electrons) through a con-

ductor.

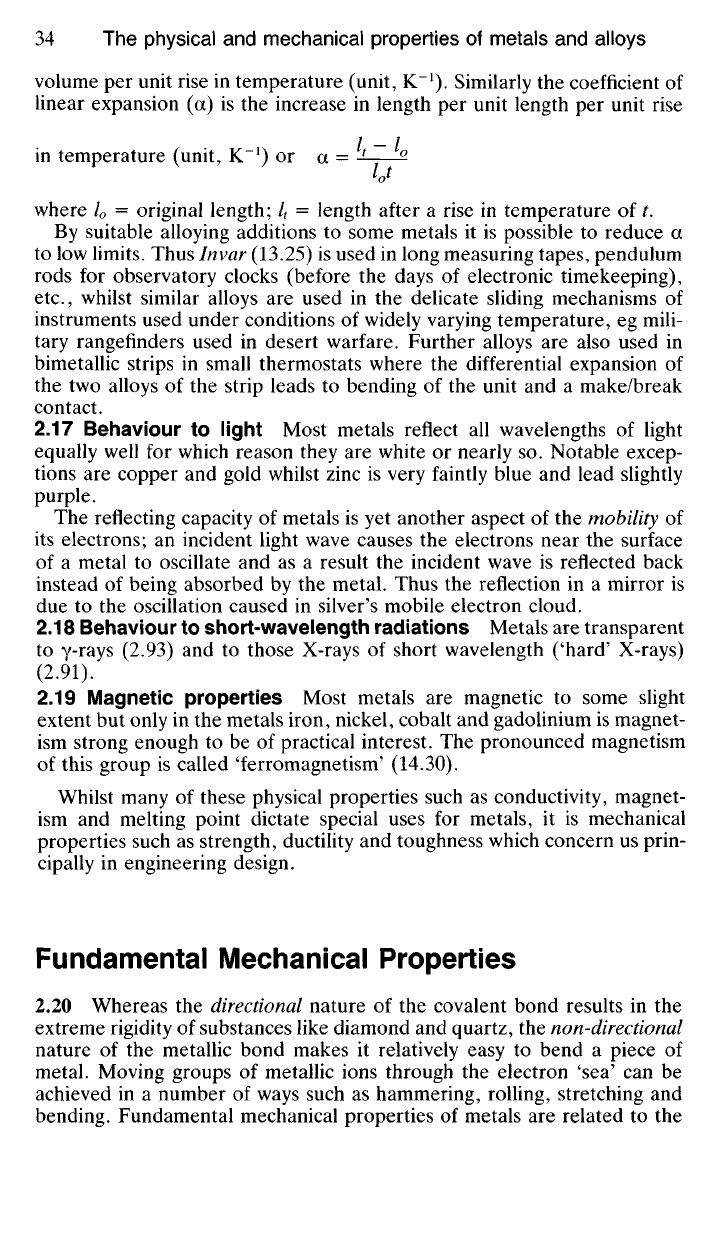
volume per unit rise in temperature (unit, K

). Similarly the coefficient of

linear expansion (a) is the increase in length per unit length per unit rise

in temperature (unit, K"

) or a = - -

where l

= original length; l

= length after a rise in temperature of t.

By suitable alloying additions to some metals it is possible to reduce a

to low limits. Thus Invar (13.25) is used in long measuring tapes, pendulum

rods for observatory clocks (before the days of electronic timekeeping),

etc.,

whilst similar alloys are used in the delicate sliding mechanisms of

instruments used under conditions of widely varying temperature, eg mili-

tary rangefinders used in desert warfare. Further alloys are also used in

bimetallic strips in small thermostats where the differential expansion of

the two alloys of the strip leads to bending of the unit and a make/break

contact.

2.17 Behaviour to light Most metals reflect all wavelengths of light

equally well for which reason they are white or nearly so. Notable excep-

tions are copper and gold whilst zinc is very faintly blue and lead slightly

purple.

The reflecting capacity of metals is yet another aspect of the mobility of

its electrons; an incident light wave causes the electrons near the surface

of a metal to oscillate and as a result the incident wave is reflected back

instead of being absorbed by the metal. Thus the reflection in a mirror is

due to the oscillation caused in silver's mobile electron cloud.

2.18 Behaviour to short-wavelength radiations Metals are transparent

to y-rays (2.93) and to those X-rays of short wavelength ('hard' X-rays)

(2.91).

2.19 Magnetic properties Most metals are magnetic to some slight

extent but only in the metals iron, nickel, cobalt and gadolinium is magnet-

ism strong enough to be of practical interest. The pronounced magnetism

of this group is called 'ferromagnetism' (14.30).

Whilst many of these physical properties such as conductivity, magnet-

ism and melting point dictate special uses for metals, it is mechanical

properties such as strength, ductility and toughness which concern us prin-

cipally in engineering design.

Fundamental Mechanical Properties

2.20 Whereas the directional nature of the covalent bond results in the

extreme rigidity of substances like diamond and quartz, the non-directional

nature of the metallic bond makes it relatively easy to bend a piece of

metal. Moving groups of metallic ions through the electron 'sea' can be

achieved in a number of ways such as hammering, rolling, stretching and

bending. Fundamental mechanical properties of metals are related to the

amounts of deformation which metals can withstand under different cir-

cumstances of force application. Ductility refers to the capacity of a sub-

stance to undergo deformation under tension without rupture, as in wire-

or tube-drawing operations. Malleability, on the other hand, is the capacity

of a substance to withstand deformation under compression without rup-

ture,

as in forging or rolling. Substances which are highly ductile are also

highly malleable but the reverse may not be true since some extremely

malleable substances are weak in tension and therefore liable to tear.

Moreover, whilst malleability is usually increased by raising the tempera-

ture (for which reason metals and alloys are often hot-forged or hot-rolled),

ductility is generally reduced by heating, since strength is also reduced.

2.21 Toughness refers to a metal's ability to withstand bending or the

application of shear stresses without fracture. Hence, copper is extremely

tough, whilst cast iron is not. Toughness should not, therefore, be confused

with either strength or hardness, properties which will be discussed later.

2.22 Since these fundamental mechanical properties of ductility, malle-

ability and toughness cannot be expressed in simple quantitative terms, it

has become necessary to introduce certain mechanical tests which are

related to these properties and which will allow of comparative numerical

interpretation. Moreover, the engineer is more concerned with the forces

which cause deformation in metals rather than with the deformation

itself.

Consequently tensile tests and hardness tests correlate the amounts of

deformation produced with given forces in tension and compression

respectively, whilst impact tests are an almost direct measurement of

toughness. Such precise measurements of force-deformation values make

it possible to draw up sets of specifications upon which the mechanical

engineer can base his design.

Tenacity or Tensile Strength

2.30 The tensile strength of a material is defined as the maximum force

required to fracture in tension a bar of unit cross-sectional area. In practice

a test-piece of known cross-sectional area is gripped in the jaws of a testing

machine and subjected to a tensile force which is increased by suitable

increments. For each increment of force the amount by which the length

of a pre-determined 'gauge length' on the test piece increases is measured

by some device. The test piece is extended in this way to destruction.

A force-extension diagram can then be plotted (Fig. 2.1). At first the rate

of extension is very small and such extension as there is is directly pro-

portional to the applied force; that is, OQ is a straight line. If the applied

force is removed at any point before Q is reached the gauge-length will return

to its original dimensions. Thus the extension between O and Q is elastic

and the material obeys Hooke's Law, which states that, for an elastic

body, the strain produced is proportional to the stress applied. The value

Stress

—

is constant and is equivalent to the slope of OQ. This constant value

Strain