Hawkes P.W., Spence J.C.H. (Eds.) Science of Microscopy. V.1 and 2

Подождите немного. Документ загружается.

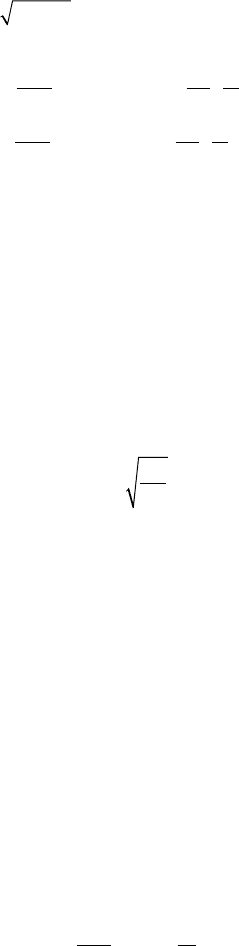
848 M. Howells et al.

(⬁ and f) and the parabolic zone plate we are analyzing is not corrected

for spherical aberration. Treating the case of a microscope (x ≈ f) and

using the notation

rwl=+

, we now substitute from (15) into (14) to

obtain the ray aberrations

∆

′

()













′

()

wlw

wll













. (16)

Thus we see that the image-plane ﬁ gure produced by a point object via

the rays passing through the rim of the lens (r = r

), is a circle of diam-

eter D

where

= M(NA)

(17)

This is produced irrespective of the position of the object point. The

presence of uncorrected spherical aberration means there will be an

optimum value of the NA, that is the largest NA for which the resolu-

tion is still diffraction-limited. This can be estimated (Michette, 1986)

by requiring that the path error be less than the Rayleigh quarter-wave

limit

opt

(18)

In practice the spherical aberration of a parabolic zone plate is often

not negligible in the soft X-ray region (see Figure 13–9 for example)

and therefore soft X-ray zone plates are usually made according to Eq.

(5), which means that the spherical aberration is corrected for the

chosen wavelength. In these cases we may ask what happens when

such a zone plate is used with a wavelength other than the correction

wavelength. We therefore consider a zone plate corrected for a given

wavelength in ﬁ rst positive order with M very small as shown in

Figure 13–6 and we assume that the correction is also small (NA << 1).

Now using a subscript zero to represent the properties of the corrected

zone plate and subscript one to represent the properties of the same

zone plate operating at another wavelength, we can apply Eq. (5) for

the nth and (n − 1)th zone to show that ∆r

≅ (λ

+ nλ

/2)/2r

. From

that we can use the grating equation to get the ray deviation angle (ε

)

at the zone plate and thus the ray displacement (ε

) at the detection

plane when the zone plate images a distant axial point (M small):

=−













=−()

, (19)

where Eq. (4) and Eq. (7) have been used. For imaging an axial point

with M large the ray displacement would be approximately Mε

. The

ﬁ rst term of these expressions, r

or Mr

, is the ray displacement needed

for correct imaging of an axial point and the second term is an aberra-

tion equal to minus the radius of the spherical aberration disk of a

parabolic zone plate (compare Eq. (19) with Eq. (17)). This results in

perfect cancellation of the spherical aberration as intended.

Chapter 13 Principles and Applications of Zone Plate X-Ray Microscopes 849

When the same zone plate is used at another wavelength λ

with

allowance for the change of focal length, ∆r

remains the same so

Eq. (19) becomes

=−













=−()

(20)

In other words the amount of correction has not changed, whereas for

exact correction it should now be r

(NA

)

/2. Thus the residual error is

an aberration disk of diameter |r

[(NA

)

− (NA

)

]| or a new disk of

diameter |(λ

− λ

)/λ

| times the diameter of the original uncorrected

disk. As an example, if the new wavelength differs by 10% from the

wavelength of correction, then the aberration disk will be reduced to

about 20% of its uncorrected size. This would be enough to make the

aberration negligible in the soft X-ray example shown in Figure 13–9.

Evidently this general conclusion applies equally to M very small or

very large.

2.2.4 Astigmatism and Field Curvature

Taking the astigmatism and ﬁ eld curvature terms together and again

calculating the ray aberrations by substituting the path function terms

into Eq.(14), we obtain

100

1000

0.1 1

Object size in units of the zone plate radius

Aberration blur size (nm)

Coma

Astig/Field curv

Diffraction

Spherical ab

0.01

0.1

Diffraction

Astig/Field curv

Coma

Spherical ab

5000 eV

500 eV

Object size in units of the zone plate radius

0.1 1

100

1000

Aberration blur size (nm)

Figure 13–9. Size of the aberrations of a soft X-ray (500 eV; left) and a hard X-ray (5000 eV; right) zone

plate as a function of object size. The soft X-ray zone plate has an outer zone width of 30 nm, diameter

of 62 µm, and a focal length of 0.75 mm at 500 eV. The hard X-ray one has an outer zone width of 60 nm,

diameter 124 µm and focal length 30 mm at 5000 eV. The graphs illustrate the general trend that hard

X-ray zone plates have lower aberrations on account of their lower NA. In the example the hard X-ray

zone plate has only negligible aberrations up to a sample diameter of about ten radii, which is far

beyond the sample size allowed by penetration requirements. On the other hand the resolution of the

soft X-ray zone plate is degraded by the ﬁ eld-angle-dependent aberrations for objects of diameter more

than about half a radius. This is still good for many experiments but, as the diagram shows, spherical

aberration is not negligible for the parabolic soft-X-ray zone plate. Therefore, as explained in Section

2.2.3, zone plates designed for soft-X-ray applications are normally made with built-in spherical-

aberration correction according to Eq. (5).

850 M. Howells et al.

∆

′

=−

′

=−

′

yxw

zxl

. (21)

Squaring and adding we ﬁ nd that the marginal rays trace out an ellipse

of major axis 3k and minor axis k

∆

′

(

)

′

(

)

kMNArz

232

1where ( )

. (22)

The parameter z¯ = z/r

expresses the position of the object point in units

of the zone plate radius. Therefore, unlike spherical aberration, the size

of this aberration does depend on the position of the object point, and

therefore will limit the ﬁ eld of view of the microscope.

2.2.5 Coma

In this case, the above procedure leads to

∆∆

′

=−

′

=− +

()

−

ywlQzwlQ Q

,where

(23)

By expressing w and l in polar coordinates one can show that each

circle of radius r in the lens produces an aberration ﬁ gure

(∆z′ − 2K)

+ (∆y′)

= K

where K = r

Q. (24)

This is a series of circles of radius K, each shifted by 2K from the origin

which is the usual “comet-shaped” ﬁ gure associated with coma. The

outer boundary of the ﬁ gure has a length 3K

max

and the largest circle

has a diameter 2K

max

where (assuming M is large so that M − 1 ≈ M)

max

= M(NA)

. (25)

2.2.6 Relative Size of the Aberrations

The relative size of these aberration ﬁ gures can be obtained from Eq.

(17), Eq. (22) and Eq. (25) respectively. The diameter of the spherical

aberration circle, the diameter of the largest coma circle and the major

axis of the astigmatism ellipse are in the ratio 1 : z

: 3z

(Michette, 1986).

For points close to the axis (z

<< 1) the ﬁ eld-angle-dependent aberra-

tions coma and astigmatism/ﬁ eld curvature will be negligible and the

resolution will be determined by diffraction if the numerical aperture

is below NA

opt

or by spherical aberration if it is above. On the other

hand for points further from the axis, coma and astigmatism/ﬁ eld

curvature become more signiﬁ cant and for points more than about a

zone plate radius away from the axis (z

≥ 1) astigmatism/ﬁ eld curva-

ture dominates. The general behavior of these aberrations is shown in

Table 13–1, while Figure 13–9 shows examples of parabolic zone plates

working at 0.5 keV and 5 keV. However, note that the plots in the ﬁ gure

show the size of the outer boundary of the aberration ﬁ gures which is

a conservative estimate of their contribution to the resolution because

the light is somewhat concentrated near the center of the ﬁ gure. For

example, we can deduce from Eq. (17) that half of the light is concen-

trated in a circle of diameter about one third of D

Chapter 13 Principles and Applications of Zone Plate X-Ray Microscopes 851

Table 13 –1. Seidel aberrations of a planar zone plate.

Spherical Astigmatism/ﬁ eld

Name aberration curvature Distortion Coma

w, l, z/x term (w

+ l

)

+ 3l

)(z/x)

l{(z/x)

+ (z′/x′)

} (w

+ l

)l(z/x)

Aberration ﬁ gure circle ellipse vanishes two lines through

boundary identically O at ±30º to Oz

touching a family

of circles

y parameter circle diameter major axis na diameter of the

largest circle

Value y parameter M(NA)

3M(NA)

na M(NA)

z parameter circle diameter minor axis na distance from the

origin to the far

side of the largest

circle

Value z parameter same M(NA)

()

MNA rz

2.3 Zone Plate Efﬁ ciency

2.3.1 Idealized Structures

One can see from Eq. (4) that an ideal Fresnel zone plate is periodic in

space with period λf, which means that its amplitude transparency

function T

(shown in Figure 13–10) can be written as the Fourier

series

im r

aq mq

zp m m













=−

()

(

)

−∞

+∞

∑

exp

1where sinc

(26)

The sinc function is deﬁ ned by sinc(x) = sin(πx)/(πx) and q is the frac-

tion of each period that is opaque. For a classical zone plate, q = 0.5, so

in that case (26) gives the power in the ±mth harmonic as |a

= 1/(mπ)

for m odd, zero for m even and 0.25 for m = 0. These are therefore the

intensity efﬁ ciencies of the classical zone plate in those orders. The

Figure 13–10. The transparency function of one period of a zone plate plotted

in r

space. The fraction that has transparency unity is q = c/L.

q=c/L

Period=L

852 M. Howells et al.

main point here is that this type of zone plate has a maximum efﬁ -

ciency in the ﬁ rst order focus of about 10%. By taking the derivative of

we can show that the classical zone plate (q = 0.5) is, in fact, the

optimum choice of q for ﬁ rst-order efﬁ ciency. Similarly, the optimum

choice for second order efﬁ ciency is q = 0.25 or 0.75 which may have

some practical signiﬁ cance, as discussed by Simpson and Michette

(1984). By applying Parseval’s theorem to the series in (26) with q = 0.5,

one can determine the disposition of the energy for the classical zone

plate as shown in Table 13–2.

Another idealized type of zone plate, ﬁ rst proposed by Rayleigh

(1888) and implemented by Wood (1898), is the phase plate. Here, the

opaque rings are replaced by transparent rings, that impart a phase

change of φ = π. The phase plate transparency function is T

= 1 + (e

iφ

− 1)T

, so the Fourier series becomes

im r

bqm

pp m













=+−

()

−

()

−∞

+∞

∑

exp

11where e sinc

(

)

(27)

This shows that when φ = π and q = 0.5, which are the optimum values,

the power in the ±mth harmonic is |b

= 4/(mπ)

for m odd, zero for m

even and |b

= |1 + q(e

iφ

− 1)|

= 0 for m = 0. The efﬁ ciency in the ﬁ rst-

order focus is now about 40% and the disposition of energy is again

shown in Table 13–2.

A third idealized type of zone plate is the Gabor plate (the hologram

of a point) in which the rectangular proﬁ le of the last two devices is

replaced by a sinusoid in r

space or a chirp function in r space. An

absorption Gabor plate has only three orders, m = ±1 and 0 of which

the +1 order has efﬁ ciency 1/16. A phase Gabor plate, with a maximum

phase change of 1.84 radians, has all the odd orders and the +1 order

receives 34% of the light (Table 13–2).

Table 13 –2. Efﬁ ciency of various types of zone plate.

Type of zone plate Fresnel Rayleigh-Wood Gabor amplitude Gabor phase

Type of zones Opaque, Phase-change p, Sine-wave Sine-wave

transparent transparent transparency phase change

max = 1.84 rad

Efﬁ ciency ±1 order 1/4 4/p

1/16 0.34

Efﬁ ciency in general 1/(m

) m odd 4/(m

) m odd 0 π 0

0 m even 0 m even

Total positive orders 1/8 1/2 1/16 0.45

(m π 0)

Total negative orders 1/8 1/2 1/16 0.45

(m π 0)

Efﬁ ciency zero order 1/4 0 1/4 0.10

Absorbed 1/2 0 5/8 0

Overall total of last 1 1 1 1

four rows

Chapter 13 Principles and Applications of Zone Plate X-Ray Microscopes 853

2.3.2 Real Structures

To make practically useful X-ray lenses, we must extend the treatment

given so far to include the optical properties of real materials, suited

to manufacturing zone plates. Such an extension was ﬁ rst provided in

1974 in an important paper by Kirz (1974) in which it was demonstrated

that

1. Phase zone plates with primary efﬁ ciencies of 20–40% can be made

from realistic materials.

2. Such zone pates can be designed to reduce or eliminate the zero-

order beam and to reduce the absorbed fraction compared to a clas-

sical Fresnel zone plate.

3. These improvements can be effected essentially throughout the

wavelength range 0.1–80 nm.

4. Realistic fabrication errors lead to only moderate deterioration in the

optical performance.

For a phase-reversal zone plate made of a material with complex refrac-

tive index (Henke et al., 1993) ñ = 1 − δ − iβ, the efﬁ ciency |b

can be

found by making the replacement φ = φ

+ iφ

in (27) where φ

= ktδ, φ

= ktβ, k = 2π/λ, t is the thickness and we use the shorthand r

= e

−φ

for

the amplitude attenuation factor. For m ≠ 0, this gives

maa

12=

()

+−

()

φcos

(28)

and for m = 0

brr

aa0

12=++

()

cosφ

(29)

These equations (Kirz’s (7) and (10) (Kirz, 1974)) give the efﬁ ciency of

a planar zone plate of known thickness and refractive index. As dis-

cussed by Kirz and later by Michette (Michette, 1986), the optimum

phase change is no longer π but is about 10–20% less. However, one

can certainly choose a thickness to optimize the efﬁ ciency of a planar

zone plate based on the use of Eq. (28). Plots of the theoretical efﬁ -

ciency, calculated using Eq. (28), for zone plates made of nickel and

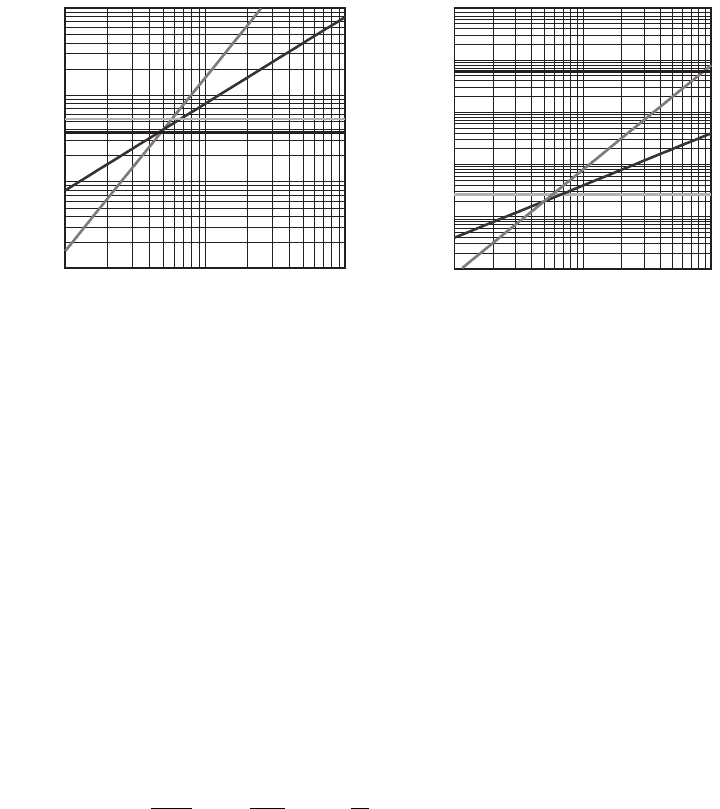
gold are shown in Figure 13–11.

2.4 Zone Plates: Fabrication and Examples

2.4.1 Fabrication Technique

The fabrication of X-ray zone plates involves several challenges. For

high resolution imaging, one wishes to obtain the smallest possible

value for the outermost zone width ∆r

or about 15–80 nm in high reso-

lution modern examples. At the same time, the thickness t of the zone

plate should ideally be that required to deliver a phase shift near π so

as to maximize efﬁ ciency; this generally implies a thickness of greater

than 100 nm for soft X-rays of 100–1000 eV energy, and a thickness near

1 µm for zone plates designed to operate at multi keV energies (see

Figure 13–11). These two requirements are difﬁ cult to meet at the same

time, for they lead to a demand for the fabrication of nanostructures

854 M. Howells et al.

with very high aspect ratios (structure height over width). In addition,

to obtain usable focal lengths of order 1 mm or larger, most zone plates

used as high resolution objectives have diameters of 50–200 µm yet

to minimize loss of efﬁ ciency all zones must be placed accurately to

roughly one-third of their width (Simpson and Michette, 1983). This

implies an absolute accuracy of zone placement of about 0.01% which

is quite challenging but which can be achieved in modern 100 keV

electron beam lithography systems that incorporate laser interferome-

ter positioning control (Anderson et al., 2000; Tennant et al., 2000). Such

zone plates typically have 300–1000 zones, and require corresponding

quasimonochromatic illumination with E/∆E > 300–1000 or better

(Thieme, 1988).

Early demonstrations of X-ray zone plate fabrication used optical

lithography to create free-standing zone plates with ∆r

= 20 µm (Baez,

1960). An important early advance was the use of holographic methods

to create zone plates with submicron zone widths (Schmahl and

Rudolph, 1969; Niemann et al., 1974), eventually leading to outermost

zone widths of ∆r

= 56 nm (Schmahl et al., 1984b). The method used

by nearly all laboratories today involves electron beam lithography,

which was ﬁ rst suggested by Sayre (Sayre, 1972) and subsequently

demonstrated in several laboratories (Shaver et al., 1980; Kern et al.,

1984; Buckley et al., 1985). In order to obtain high aspect ratio nano-

structures, most laboratories now use some variation of tri-layer resist

schemes (Tennant et al., 1981) with electroplating (Schneider et al.,

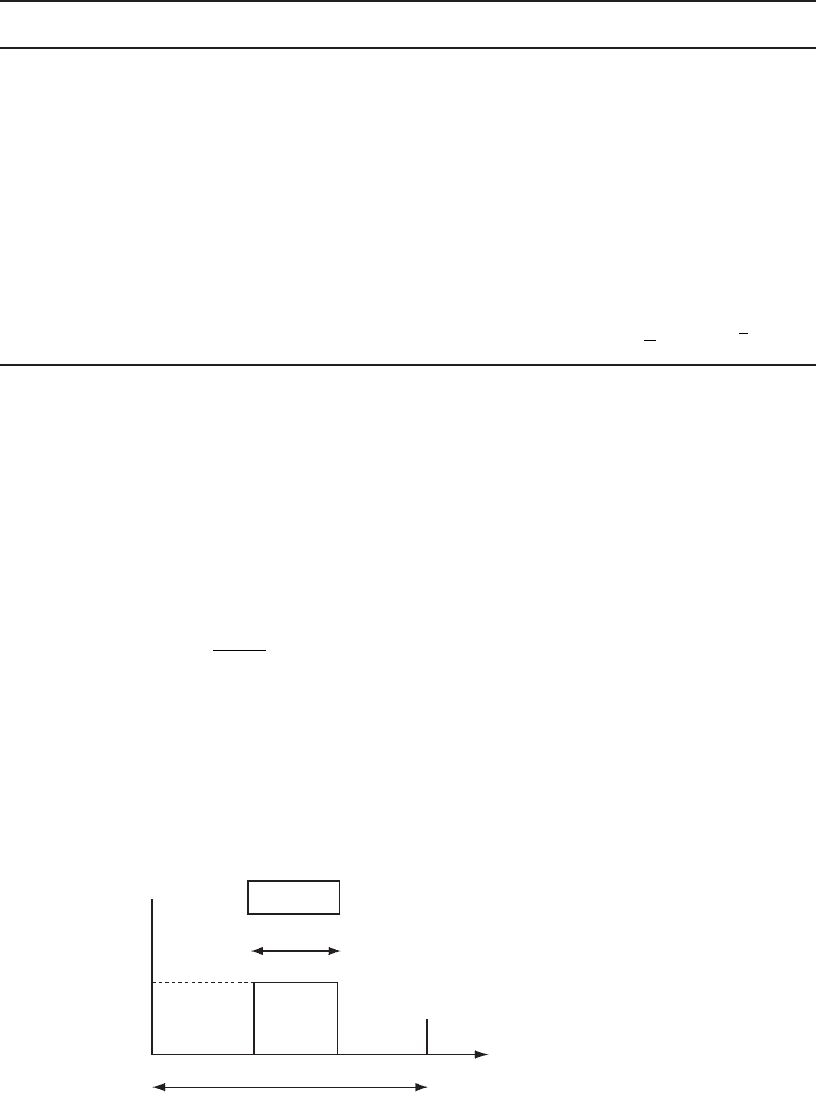
1995) as illustrated in Figure 13–12. This approach allows for the writing

of ﬁ ne, dense features in an electron beam resist which is sufﬁ ciently

thin that electron side scattering is minimized. A series of reactive ion

etches are used to ﬁ rst transfer the e-beam pattern into a hard mask,

and to use that hard mask to transfer the pattern into a second polymer

Thickness (nm)

100

200

500

1000

2000

5000

Energy (eV)

200 500 1000 2000 5000 10000 20000

Energy (eV)

200 500 1000 2000 5000 10000 20000

100

200

500

1000

2000

5000

Thickness (nm)

100

200

500

1000

2000

5000

Nickel

(ρ=8.87)

Gold

(ρ=18.9)

10%

15%

10%

15%

20%

25%

10%

15%

10%

15%

20%

25%

30%

10%

15%

20%

25%

30%

35%

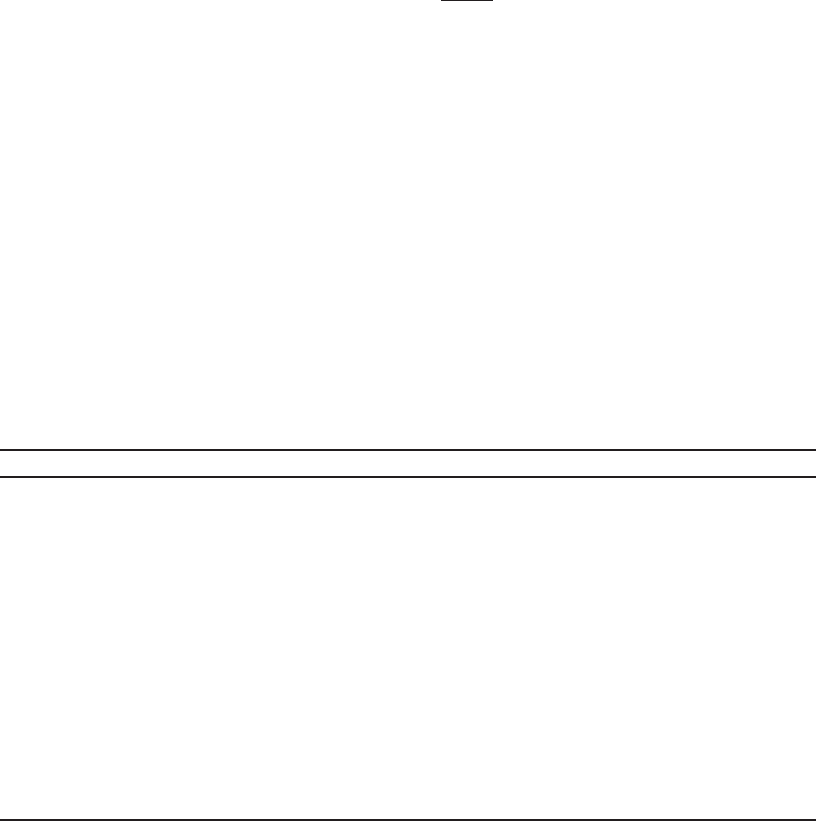
Figure 13–11. Contour plot showing the efﬁ ciency of planar zone plates made of Ni (left) and Au

(right) according to Eq. (24) as a function of thickness and X-ray energy.

Chapter 13 Principles and Applications of Zone Plate X-Ray Microscopes 855

used either to deﬁ ne an etch mask for the underlying zone material

(Tennant et al., 1991; David et al., 1995, 2000) or, more commonly, as a

mold for electroplating of the zone structures (Schneider et al., 1995).

Using these approaches, several groups have fabricated zone plates

with outermost zone widths of 20 nm or below (Bögli et al., 1988;

Schneider et al., 1995; Spector et al., 1997; Anderson et al., 2000; Peuker,

2001; Chao et al., 2003).

A recent promising approach (Chao et al., 2005) has been to write

every other zone in one pass, and then to write the other half of the

zones in a subsequent processing step (of course extremely high overlay

accuracy is required). By increasing the distance from the next zone

written in one pass, the proximity effect of electron beam lithography

is reduced, leading to higher contrast and thus higher aspect ratio

in the developed resist. In addition, the width of the resist used as a

plating mold is tripled, thus reducing collapse during processing. This

approach has been used to fabricate zone plates with an outermost

zone width of 15 nm (Chao et al., 2005) and a theoretical zone efﬁ ciency

of 6% (see next section). A key challenge lies in maintaining high

efﬁ ciency as the outermost zone width is decreased. For applications

requiring higher efﬁ ciency for greater ﬂ ux in STXM, or reduced radia-

tion dose in TXM, efﬁ ciencies of 10–18% have been obtained at 30 nm

outermost zone width (Spector et al., 1997; Peuker, 2001). These efﬁ cien-

cies are for zone plates operating at 400–550 eV; zone plates for use at

higher X-ray energies are discussed in Section 2.4.5.

2.4.2 Resolution-Determining Zone Plates

While a variety of groups are fabricating high resolution zone plates

as described above, we consider here one example which is the effort

of the Center for X-ray Optics at Lawrence Berkeley National Labora-

tory. By using a 100 keV electron beam lithography system with inter-

ferometric positioning control and customized circular pattern

generation (Anderson et al., 1995), this group has made a series of zone

plates with steadily improving resolution (Anderson et al., 2000; Chao

et al., 2003) and have developed a sophisticated technique for deter-

mining the resolution (Jochum and Meyer-Ilse, 1995; Heck, 1998; Chao

Si frame

Window

Hard mask

Plating mold

E-beam resist

1. E-beam expose, develop 2. Etch hard mask

3. Etch plating mold; strip hard mask

4. Metal plating

Plating base

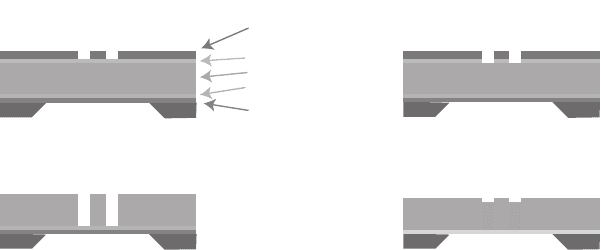
Figure 13–12. Schematic of zone plate fabrication technique as discussed in

the text.

856 M. Howells et al.

et al., 2003). The tests have been done using the XM-1 microscope at

beam-line 6.1 at the Advanced Light Source at Berkeley USA (see

Microscopes Layouts and Illumination Schemes (Sectin 3.1)). The latest

results (Chao et al., 2005), which represent the best zone-plate-micro-

scope resolution that we know of, show a resolution of <15 nm at a

photon energy of 815 eV (see Figure 13–13). This measured value

depends on the degree of partial coherence of the illumination and we

discuss it in that context in a later section. The zone plate had an outer

zone width of 15 nm, which, with the XM-1 illumination system, gave

a theoretically expected resolution of 12 nm. The zone plate was made

by the above-mentioned new process in which the n = 2, 6, 10, . . . and

the n = 4, 8, 12, . . . opaque zones are made in two separate groups. Chao

and colleagues suggest that the new process is not yet at its limit and

that 10 nm zone plates should be within reach.

2.4.3 Condenser Zone Plates

Condenser zone plates serve the dual function of imaging the source

on to the sample (in critical illumination) and, in combination with a

pinhole close to the sample, of acting as a moderate-resolution mono-

chromator. Ideally they should deliver a beam which (1) has the same

NA as the objective zone plate, (2) exactly ﬁ lls the sample with light

and (3) has a spectral bandwidth equal to the reciprocal of the number

of zones of the objective. In practice, due to the fact that bending-

magnet synchrotron-radiation sources usually have smaller phase

space area than the microscope and due to the fabrication difﬁ culties

described below, conditions (1) and (2) cannot be fully met. Moreover,

condition (3) implies that the condenser zone plate must be about 5–

10 mm in diameter. We discuss the trade-offs involved here in more

detail in Section 3.1.1.

The ﬁ rst condenser zone plates were fabricated by the Göttingen

group (Schmahl and Rudolph, 1984b; Hettwer, 1998) using roughly the

same holographic process then used to make objective zone plates. As

a result, the ﬁ nest line widths (and thus the NAs) of the condenser and

d/2=19.5 nm

∆r

=25 nm

d/2=19.5 nm

∆r

=15 nm

d/2=15.1 nm

∆r

=25 nm

d/2=15.1 nm

∆r

=15 nm

100 nm

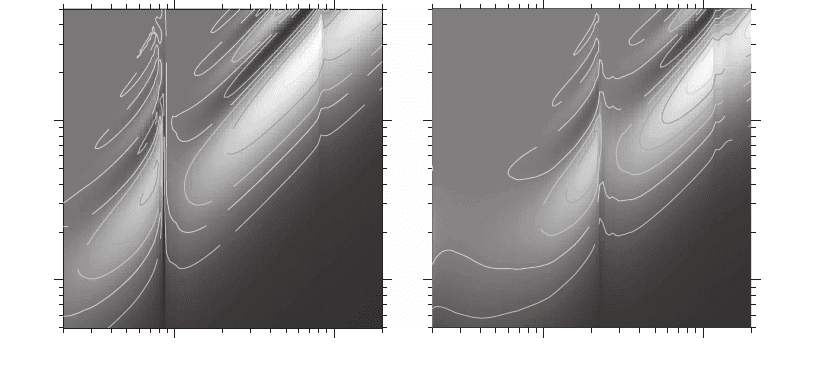
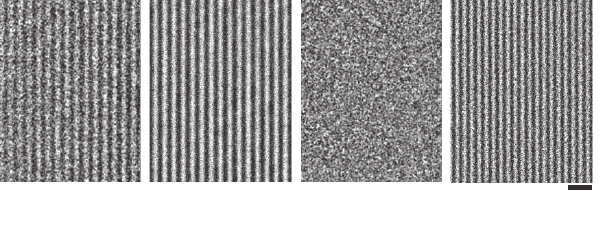
Figure 13–13. The soft X-ray images of square-wave test objects used by Chao

and coworkers (2005) to demonstrate a microscope resolution of <15 nm using

a 15-nm- and a 25-nm-outer-zone-width zone plate as explained in the text.

The half-period of the test object and the outer zone width of the zone plate

mission from Nature.)

Chapter 13 Principles and Applications of Zone Plate X-Ray Microscopes 857

objective tended to match, which is broadly what is required for best

resolution (see for example Fig.10.13 of (Born and Wolf, 1999)). With

electron beam lithography, new challenges have arisen. To achieve the

same ﬁ nest zone width and efﬁ ciency in the condenser as in the objec-

tive, would require the use of the same high-resolution but necessarily

slow electron beam resists. Since the area of a 5 mm diameter con-

denser is 10

times larger than a 50 µm objective zone plate, it would

take 10

times longer to fabricate the condenser with the same process.

(Because aberrations on condensers do not degrade image quality, the

requirements for zone placement accuracy do not scale up in the same

fashion). For these reasons, as the resolution of the objectives has been

pushed down below 20 nm, the condenser zone plates have not kept

up. Instead, typical condenser zone plates fabricated by electron beam

lithography have outer zone widths of 50–60 nm. As one example, the

fabrication of a TXM condenser zone plate with 9 mm diameter and

55 nm outer-zone width required a 48-hour writing time (Anderson

et al., 2000). Although process improvements have since reduced that

time by about a factor of two, such large zone plates are still not widely

available. The mismatch of numerical aperture between condenser and

objective zone plates limits the modulation transfer at high spatial fre-

quency (Born and Wolf, 1999) and thus limits the ability to detect small

structures such as immunogold labels in bright ﬁ eld or dark ﬁ eld

modes (Vogt et al., 2001a).

Besides the challenges of matching the NA of the best objectives and

their limited availability, condenser zone plates are usually required

to operate in an unfriendly environment, relatively near the source

in a synchrotron beam line. Even with the protection of an energy-

ﬁ ltering mirror, they still often take signiﬁ cant heat load and the heat

removal pathways are poor. This is an undesirable circumstance for

optics that take so much effort to build. The problem of power deposi-

tion in condenser zone plates can be understood by solving the bound-

ary value problem of a uniformly heated membrane (Howells et al.,

2002). Assuming a square membrane of side a, thickness t and conduc-

tivity k, the solution for the temperature is

Txy

kt mn

odd

(

)

∞

∑∑

16 1

sin sin

(30)

where Q is the absorbed power density. The double sum is a simple

function that has a peak of height 0.448 in the center and is zero at the

edges. The center temperature is then given by the useful relation

max

.= 717

. (31)

Since the maximum temperature depends on Q/t and, for a simple thin

membrane, Q is proportional to t, the temperature is not reduced by

making the membrane thicker. However, if the absorption is princi-

pally in the zone plate rings then a thicker membrane may help. A

smaller or better-conducting membrane always helps.