Giga Y. Surface Evolution Equations: A Level Set Approach

Подождите немного. Документ загружается.

78 Chapter 2. Viscosity solutions

under positive multiplication, i.e., af ∈Fif f ∈F and a>0 for (2.1.14) with

q>1. This important property also holds for geometric F .

Deﬁnition 2.1.5. Assume that F is continuous on W

= Ω × [0,T] × R ×

\{0})×S

with values in R. Assume that F is nonempty. An F-subsolution of

(2.1.5) with F is deﬁned as in Deﬁnition 2.1.1 by replacing (i)(b) by the condition:



(ˆz)+F (ˆz, u

∗

(ˆz), ∇ϕ(ˆz), ∇

ϕ(ˆz)) ≤ 0if∇ϕ(ˆz) =0,

(ˆz) ≤ 0otherwise

for all (ϕ, ˆz) ∈ C

(O) ×O satisfying (2.1.6). An F-supersolution of (2.1.5) with

F is deﬁned as in Deﬁnition 2.1.1 by replacing (ii)(b) by the condition:



(ˆz)+F (ˆz, u

∗

(ˆz), ∇ϕ(ˆz), ∇

ϕ(ˆz)) ≥ 0if∇ϕ(ˆz) =0,

(ˆz) ≥ 0otherwise

for all (ϕ, ˆz) ∈ C

(O) ×O satisfying (2.1.8). One may deﬁne F-subsolution is an

open set O in Ω × (0,T] as for usual subsolutions.

Remark 2.1.6. If

lim

p→0

X→O

sup

z∈O

sup

r∈R

|F (z, r,p, X)| =0, (2.1.15)

(which in particular implies (2.1.10)), then clearly

F = {f ∈ C

(0, ∞); f(0) = f



(0) = f



(0) = 0 and f



(r) > 0forr>0}.

It turns out that C

(O)equals

= {ϕ ∈ C

(O); ∇ϕ(ˆz) = 0 implies ∇

ϕ(ˆz)=O}.

We postpone its proof. By this observation a subsolution is an F-subsolution since

∗

(z,r,0,O) = 0 by (2.1.15). It is curious whether or not an F-subsolution agrees

with a subsolution in Deﬁnition 2.1.4. It turns out that the converse is true if

(2.1.15) is fulﬁlled and F is degenerate elliptic as proved in Proposition 2.2.8.

Here we just give a simple equivalent deﬁnition of F- subsolutions.

Proposition 2.1.7. Assume that (2.1.15) holds for F .Afunctionu : O→R ∪

{−∞} is an F-subsolution of (2.1.5) if and only if u is a solution of

+ F

(z,u,∇u, ∇

u) ≤ 0 in O

with

(z,r,p,X)=

⎧

⎨

⎩

F (z, r,p, X) if p =0,

0 if (p, X)=(0,O),

−∞ otherwise.

A similar assertion holds for an F-supersolution by replacing the above inequality

+ F

(z,u,∇u, ∇

u) ≥ 0 in O

2.1. Deﬁnitions and main expected properties 79

with

(z,r,p,X)=

⎧

⎨

⎩

F (z, r,p, X)ifp =0,

0if(p, X)=(0,O),

+∞ otherwise.

This follows from the deﬁnition of an F-solution if we admit A

= C

(O),

which is proved in Proposition 2.1.8. Note that F

(≤ F

∗

)andF

(≥ F

∗

)are,

respectively, still lower and upper semicontinuous functions on

Ω × [0,T] × R ×

× S

by (2.1.15). Here is a trivial remark. One may weaken (2.1.15) by

lim

p→0

X→O

sup

z∈O

sup

r∈R

|F (z, r,p, X) − a

| =0

for a ﬁxed constant a

so that F

∗

(z,r,0,O)=a

= F

∗

(z,r,0,O). To deﬁne an

F-subsolution in this situation we should replace (2.1.11) by

lim

p→0

sup

z∈O

sup

r∈R

|F (z, r,∇(f(|p|)), ±∇

(f(|p|))) − a

| =0

and replace ϕ

(ˆz) ≤ 0byϕ

(ˆz)+a

≤ 0andϕ

(ˆz) ≥ 0byϕ

(ˆz)+a

≥ 0in

Deﬁnition 2.1.5. The statement of Proposition 2.1.7 is still valid by replacing 0

by a

in the deﬁnition of F

and F

. One may even replace a

by a continuous

function a

(z,r) to handle the equation having an external force term.

For the level set equation (1.6.12) of the Gaussian curvature ﬂow equation it is

not diﬃcult to see that F

∗

(0,X)=−∞ and F

∗

(0,X)=+∞ (see §1.6.5) so a sub-

solution in Deﬁnition 2.1.4 may not be an F-subsolution, while an F-subsolution

is always a subsolution for (1.6.12) diﬀerent from the case when (2.1.15) is ful-

ﬁlled. In this case Deﬁnition 2.1.5 is more restrictive than Deﬁnition 2.1.4 which

is indeed important to get the comparison principle.

Proposition 2.1.8. Assume that (2.1.15) holds for F .ThenC

(O)=A

Proof. It is easy to see that A

includes C

(O). Indeed, the condition (2.1.12)

implies that ∇

ϕ(ˆz)=O for ˆz with ∇ϕ(ˆz)=0.

It remains to prove that C

(O) includes A

.If∇ϕ(ˆz)=0and∇

ϕ(ˆz)=O,

then

|ϕ(z) − ϕ(ˆz) − ϕ

(ˆz)(t −

t)|≤ω

(|t −

t|)+ω

(|x − ˆx|)

for all (x, t)with|x − ˆx|≤δ, |t −

t|≤δ for some δ>0andω

,ω

≥ 0with

lim

σ→0

(σ)/σ

=0 (k =1, 2). (2.1.16)

We would like to ﬁnd f

∈ C

[0, ∞)withf

(0) = f



(0) = f



(0) = 0 and f



≥ 0

that satisﬁes

(σ) ≤ f

(σ)for0≤ σ ≤ δ. (2.1.17)

If such an f

exists, f (σ)=f

(σ)+σ

belongs to F since (2.1.11) is always fulﬁlled

for f ∈ C

[0, ∞)withf



(0) = f



(0) = 0 under the condition (2.1.15).

80 Chapter 2. Viscosity solutions

We shall prove the existence of f

.Ifweset

(σ)=sup{ω

(η)/η

;0≤ η ≤ σ, η ≤ δ},

then ω

is a nondecreasing function on [0, ∞)withω

(0) = 0 which is continuous

at zero by (2.1.16). Since

(σ) ≤ ω

(σ)σ

for 0 ≤ σ ≤ δ,

the existence of f

that satisﬁes (2.1.17) follows from the next elementary lemma

by taking f

= θ with k =2. 

Lemma 2.1.9.

(i) Let ω

be a nondecreasing function on [0, ∞) with ω

(0) = 0. Assume that

is continuous at zero. Then there is a modulus ω (i.e., ω is a nondecreas-

ing continuous function on [0, ∞) with ω(0) = 0)withω ∈ C

∞

(0, ∞) that

satisﬁes ω

≤ ω on [0, ∞).

(ii) Let ω be a modulus and let k be a positive integer. Then there is θ ∈ C

[0, ∞)

such that θ

(j)

(0) = 0 and θ

(j)

(σ) ≥ 0 for σ ≥ 0 with 0 ≤ j ≤ k and

ω(σ)σ

≤ θ(σ) for all σ ≥ 0.Hereθ

(j)

denotes the j-th derivative of θ.

Proof of Lemma 2.1.9. (i) We set ω(m)=ω

(m +1), m =1, 2,... and ω(1/2

(1/2

j−1

), j =1, 2,.... Interpolating the value of ω(σ)form ≤ σ ≤ m +1and

1/2

≤ σ ≤ 1/2

j−1

by an aﬃne function, we get a continuous function ω on (0, ∞).

It is possible to mollify ω without changing values at 1/2

, j =1, 2,...,sothat

ω ∈ C

∞

(0, ∞)andω

≤ ω.Sinceω

is nondecreasing so is ω and ω

≤ ω on

(0, ∞). Since ω

is continuous at zero, we have

lim

σ→0

ω(σ) = lim

j→∞

(1/2

j−1

)=0.

Thus ω extends a continuous function on [0, ∞) (still denoted ω) by setting ω(0) =

(ii) We set

(σ)=



2σ

j−1

(s)ds, j ≥ 1,θ

= ω for σ ≥ 0,

so that θ

∈ C

[0, ∞)withθ

(i)

(0) = 0 for 0 ≤ i ≤ j.Sinceθ

is nondecreasing, we

have

(σ) ≥ σθ

j−1

(σ)forσ ≥ 0

so that θ

(σ) ≥ σ

ω(σ). We thus observe that θ = θ

has all desired properties. 

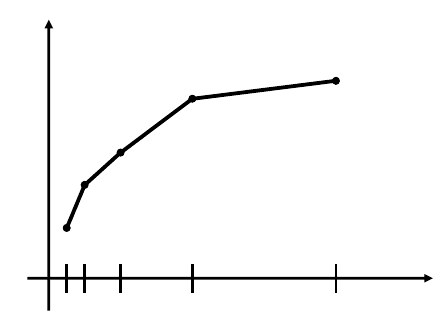
2.1. Deﬁnitions and main expected properties 81



Figure 2.4: Construction of ω

Remark 2.1.10. For F-subsolutions the statement of Proposition 2.1.2 should

be altered. Assume that u ∈ C

(O)satisﬁes

(ˆz)+F (ˆz, u(ˆz), ∇u(ˆz), ∇

u(ˆz)) ≤ 0(resp.≥ 0.)

for ˆz ∈Owith ∇u(ˆz) =0andthat

(ˆz) ≤ 0(resp.≥ 0) (2.1.18)

for ˆz ∈Owith ∇u(ˆz)=0.Thenu is an F-subsolution (resp. F-supersolution) of

(2.1.5) in O provided that F is degenerate elliptic. For ˆz with ∇u(ˆz) = 0, (2.1.18)

is unnecessary if there are no f ∈F and ω

(with ω

(σ)/σ → 0asσ → 0) that

satisﬁes

u(x, t) − u(ˆz) − u

(ˆz)(t −

t) ≤ (resp. ≥)f (|x − ˆx|)+ω

(t −

for all z =(x, t) suﬃciently close to ˆz =(ˆx,

t). These statements immediately

follow from the deﬁnition. We also note that the localization property is still

valid for F-solutions. Although the proof for (i) (in the localization property) is

immediate, the proof for (ii) needs an extension property as follows. Suppose that

max



∗

− ϕ)=(u

∗

− ϕ)(ˆz)

in a neighborhood O



of ˆz in O with ϕ ∈ C



). If ∇ϕ(ˆz) = 0, then there is

ψ ∈ C

(O)thatsatisﬁesϕ = ψ in some neighborhood of ˆz in O



, ∇ψ(z) =0for

all z ∈Oand

max

∗

− ψ)=(u

∗

− ψ)(ˆz).

If ∇ϕ(ˆz) = 0, then there is ψ ∈ C

(O) that satisﬁes ϕ ≤ ψ in some neighborhood

of ˆz in O



and ϕ(ˆz)=ψ(ˆz). The proof is easy and left to the reader.

82 Chapter 2. Viscosity solutions

2.2 Stability results

We shall give typical results on the stability principle in its strong form.

Theorem 2.2.1. Let Ω be a domain in R

and T>0.LetO be an open set in

Ω × (0,T).

(i) Assume that F

and F are lower (resp. upper) semicontinuous in W = Ω ×

[0,T] × R × R

× S

with values in R ∪{−∞} (resp. R ∪{+∞})forε>0.

Assume that

F ≤ lim inf

ε→0

∗

in W (resp. F ≥ lim sup

ε→0

∗

in W ). (2.2.1)

Assume that u

is a subsolution (resp. supersolution) of

+ F

(z,u,∇u, ∇

u)=0 (2.2.2)

in O.Then

u = lim sup

ε→0

∗

(resp. lim inf

ε→0

∗

)isasubsolutionof

+ F (z,u,∇u, ∇

u)=0 (2.2.3)

in O provided that

u(z) < ∞ (resp. u(z) > −∞)foreachz in O.

(ii) Assume that F

and F are continuous in W

= Ω×[0,T]×R×(R

\{0})×S

with values in R. Assume that F(F ) is included in F

= F(F

) for all

(suﬃciently small) ε and that F

converges to F locally uniformly in W

as ε → 0. Assume that F is invariant under positive multiplication, i.e.,

f ∈F= F(F ) implies af ∈F for all a>0. Assume that for any f ∈F(F ),

lim inf F

(ζ,ρ,∇(f(|p|)), ∇

(f(|p|))) ≥ 0,

(resp. lim sup F

(ζ,ρ,∇(f(|p|)), ∇

(f(|p|))) ≤ 0)

(2.2.4)

as ε → 0, ζ → z, ρ → r, p → 0 for all z ∈O,r ∈ R. Assume that u

is an F

-subsolution (resp. supersolution) of (2.2.2) in O.Thenu is an F-

subsolution of (2.2.3) in O provided that

u(z) < ∞ (resp. u(z) > −∞)for

each z ∈O.

Clearly, the condition (2.2.1) is fulﬁlled if F

converges to F locally uniformly

in W as ε → 0. However, the uniform convergence in W is not expected if F has

singularities. This is a reason why we assume (2.2.1) instead of uniform conver-

gence in W .IfF and F

(ε>0) satisfy (2.1.15), then F = F

= A

by Proposition

2.1.8. In this case if F

→ F

locally uniform in W

and

≤ lim inf

ε→0

∗

in W (2.2.5)

(which is a special form of the left inequality in (2.2.1)), then it is easy to see that

the ﬁrst inequality of (2.2.4) holds, since F

(z,r,0,O) = 0 by Proposition 2.1.8.

2.2. Stability results 83

However, the ﬁrst inequality of (2.2.4) does not seem to imply (2.2.5) under the

uniform convergence assumption in W

even if F = F (z,r,p, X) does not depend

on z and r, although both conditions are closely related.

The proof of Theorem 2.2.1 is not diﬃcult if we are familiar with various

equivalent deﬁnitions of viscosity solutions and convergence of maximum points

explained in the next few subsections.

2.2.1 Remarks on a class of test functions

We give several observations on test functions which are practically important to

prove expected properties for viscosity solutions.

Proposition 2.2.2. In (2.1.6) of Deﬁnitions 2.1.1, 2.1.4,and2.1.5 one may replace

O by some neighborhood of ˆz. The maximum may be replaced by a strict maximum

in the sense that

∗

− ϕ)(z) < (u

∗

− ϕ)(ˆz),z=ˆz, z ∈O (2.2.6)

or even by a local strict maximum in the sense that (2.2.6) holds with O replaced by

some neighborhood of ˆz provided that F is invariant under positive multiplication.

Similarly, in (2.1.8) the minimum may be replaced by a strict minimum or by a

local strict minimum.

Proof. It is easy to see that a global maximum may be replaced by a local max-

imum in (2.1.6) and (2.2.6) in deﬁnitions similar to the proof of the localization

property in §2.1.1. For F-subsolution see also Remark 2.1.10.

If ϕ ∈ C

(O) satisﬁes (2.1.6), then ψ(z)=ϕ(z)+|z − ˆz|

satisﬁes (2.2.6).

If (2.1.7) holds for ψ,sodoesϕ. This shows that global maximum in (2.1.6)

may be replaced by global strict maximum in Deﬁnitions 2.1.1 and 2.1.5. For F-

subsolutions one should be a little bit careful. If ϕ ∈ C

(O) satisﬁes (2.1.6), then

ψ(z)=ϕ(z)+f(|x − ˆx|)+(t −

with f ∈F satisfying (2.2.6). However, ψ may

not be in C

(O). So we choose ψ in another way. We may assume that ∇ϕ(ˆz)=0

and recall (2.1.12). Since ϕ is C

, we may assume that

(σ)=ϕ

(

t)σ

/2+ω(σ)σ

where ω is a modulus. By Lemma 2.1.9 (ii) there is θ

∈ C

[0, ∞)withθ

(0) =



(0) = 0 and θ



(0) = ϕ

(

t) that satisﬁes

(|t −

t|) ≤ θ

(|t −

t|).

If we set

ψ(x, t)= ϕ(ˆz)+ϕ

(ˆz)(t −

t)+f(|x − ˆx|)+θ

(|t −

t|)

+f(|x − ˆx|)+(t −

(2.2.7)

84 Chapter 2. Viscosity solutions

then ϕ(ˆz)=ψ(ˆz)and

ϕ(z) <ψ(z), for z =ˆz, z =(x, t) ∈O



with



= {(x, t); |x − ˆx| <δ, |t −

t| <δ}.

The last two terms f(|x − ˆx|)and|t −

are added to get a strict inequality.

Since 2f ∈F,ψ ∈ C



) and (2.2.6) holds with O replaced by O



.Herethe

property that F is invariant under positive multiplication is invoked. If the second

diﬀerential inequality in Deﬁnition 2.1.5 holds for ψ, i.e., ψ

(ˆz) ≤ 0, so does ϕ

(ˆz) ≤

0. This explain the reason why we may replace maximum by strict maximum even

for F-subsolutions. The proof for supersolutions parallels that for subsolutions. 

Proposition 2.2.3.

(i) In Deﬁnitions 2.1.1 and 2.1.4 the class of test functions may be replaced by

(O),C

∞

(O) or

(O)={ϕ(x, t)=b(x)+g(t) ∈ C

(O)}

where ∞≥k ≥ 2. In Deﬁnition 2.1.5 C

(O) may be replaced by

(O)={ϕ(x, t)=b(x)+g(t) ∈ C

(O),g ∈ C

(R)}

where ∞≥k ≥ 1 provided that F is invariant under positive multiplication.

(ii) The classes of test functions C

(O) and C

(O) may be replaced also by

2,1

(O) and

2,1

(O)=the set of ϕ ∈ C

2,1

(O) that satisﬁes (2.1.12),

where C

2,1

(O) is the space of ϕ whose derivatives ϕ

, ∇

ϕ, ∇ϕ are continu-

ous in O.OfcourseforC

2,1

the class F should be invariant under positive

multiplication.

Remark 2.2.4. It is sometimes convenient to extend a class of test functions

other than C

for continuous F in Deﬁnition 2.1.1. For example one would like

to consider Sobolev spaces W

2,1

(O) as a class of test functions. Here W

2,1

(O)

denotes the space of ϕ ∈ L

(O) that satisﬁes ∇

ϕ ∈ L

(O)andϕ

∈ L

(O). By

the Sobolev embedding for large p,sayp>N+1, W

2,1

(O) ⊂ C(O) so that the

value of ϕ at each point of O is meaningful. However, ∇

ϕ may not be continuous,

it is merely a p-th integrable measurable function and the value of ∇

ϕ at each

point of O is only determined up to a measure zero set in O. The condition (i)(b) in

Deﬁnition 2.1.1 should be interpreted as follows. If (ϕ, ˆz) ∈ W

2,1

(O) ×O satisﬁes

(z)+F (z, u

∗

(z), ∇ϕ(z), ∇

ϕ(z)) ≥ ε>0

2.2. Stability results 85

for some ε>0 in some neighborhood of ˆz,thenu

∗

−ϕ does not attain its maximum

over O at ˆz. It is immediate that for ϕ ∈ C

(O) this is equivalent to (i)(b). Such

a type of deﬁnition is important to study regularity theory for fully nonlinear

equations when z-dependence of F is just measurable.

To prove (i) we approximate a test function ϕ by a smoother function ϕ

However, the maximum point z

of u

∗

− ϕ

may be diﬀerent from the maximum

point ˆz of u

∗

− ϕ so we need to study the behavior of z

as ε → 0. The next

general lemma is useful not only to prove Proposition 2.2.3 but also to prove

stability results.

For a sequence {S

} of subsets of a metric space the upper relaxed limit

Limsup

j→∞

is deﬁned by Limsup

j→∞



x ∈ X; lim sup

∗

j→∞

(x)=1



where χ

is the characteristic function of a set A.

2.2.2 Convergence of maximum points

Lemma 2.2.5. Let X be a metric space. For ε>0 let U

be an upper semicontin-

uous function on X with values in R∪{−∞}.Let

U be a function on X deﬁned by

U = lim sup

∗

ε→0

.LetB and S be compact sets in X. Assume that S is included

in the interior of B, i.e., S ⊂ int B in the topology of X. Assume that

U equals

a constant M on S and that

U(z) <M for z ∈ B\S. (In other words, U takes

a ‘strict’ maximum over B modulo points of S.) Let S

be the set of maximum

points of U

on B, i.e.,

= {z ∈ B; U

(z)=max

(The set S

is nonempty and U

) < ∞ for z

∈ S

since U

is upper semicon-

tinuous and B is compact.) Then there exists subsequence ε(j) →∞(as j →∞)

such that

Limsup

j→∞

ε(j)

⊂ S.

This is equivalent to saying that for each r>0 there exists j

satisfying

ε(j)

⊂ B

(S)={z ∈ B; d(z, S) ≤ r} for all j ≥ j

where d(z, S) denotes the distance from a point z to the set S.(Inparticular,

ε(j)

⊂ intB for suﬃciently large j since S ⊂ intB.) Moreover, for any z

ε(j)

∈

ε(j)

lim

j→∞

ε(j)

)=M.

Proof. 1. We may assume that U

≡−∞on B by taking a subsequence if neces-

sary. By deﬁnition of

U for each ζ ∈ S there exist a subsequence {U

ε(j)

}

∞

j=1

and a

sequence ζ

∈ X converging to ζ as j →∞that satisﬁes

M =

U(ζ) = lim

j→∞

ε(j)

(ζ

86 Chapter 2. Viscosity solutions

2. For a sequence {z

ε(j)

}(z

ε(j)

∈ S

ε(j)

)letA denote the set of its accumulation

points, i.e.,

A = {z ∈ B; there is a subsequence {z

ε(j(k))

}

of {z

ε(j)

} that satisﬁes z

ε(j(k))

→ z as k →∞}.

The set A is a nonempty compact set since B is compact.

If z

ε(j(k))

→ z, then by deﬁnition of U, lim sup

k→∞

ε(j(k))

) ≤

U(z) ≤ sup

U; the last inequality is trivial since z ∈ A by deﬁnition of A.Since

any subsequence limit of {z

ε(j)

} belongs to A,wehave

lim sup

j→∞

ε(j)

) ≤ sup

3. Since ζ

converges to a maximum point ζ ∈ S of U over B and S is contained

in int B,weobserveζ

∈ B for suﬃciently large j,sayj ≥ j

.Since

ε(j)

(ζ

) ≤ U

ε(j)

)

for j ≥ j

, Steps 1 and 2 yield

M = lim

j→∞

ε(j)

(ζ

) ≤ lim inf

j→∞

ε(j)

)

≤ lim sup

j→∞

ε(j)

) ≤ sup

Since M ≥ sup

U is trivial, we get

M =sup

U = lim

j→∞

ε(j)

Since S is the set of all maximum points of

U in B, S includes A. This inclusion

implies that

Limsup

j→∞

ε(j)

⊂ S. 

Remark 2.2.6. (i) If U

converges to U locally uniformly in a neighborhood of

S, then we need not take a subsequence ε(j). If, moreover, S consists of only one

point

z,sothatU attains a strict local maximum at z,thenz

converges to z and

) → U (z) without taking subsequences.

Indeed, if U

converges to U locally uniformly in B

(z), for any sequence

→ z the formula

M =

U(z) = lim

ε→0

(ζ

)

is valid without taking any subsequence of U

. This is stronger than Step 1. We ar-

gueasinStep2,3butz

replaces z

ε(j)

respectively. Since A is contained

in S = {

z}, z

converges to z without taking a subsequence.

This simple version is also important to develop the theory of viscosity solu-

tions, for example, in proving Proposition 2.2.3.

2.2. Stability results 87

(ii) Lemma 2.2.5 is a trivial modiﬁcation of [G.Barles (1994), lemma 4.2] where S is

assumed to be a singleton. An essentially same proof is presented there. However,

it seems that [p.90, line 6] needs a further explanation, which is included in our

proof. The inequality in [p.90, line 6]

v(y) ≤ lim sup v



)fory ∈ Ω ∩ B

(x)

may not be true even for y = x unless one ﬁrst chooses a subsequence of v

such

that

v(x) = lim

j→∞

ε(j)

)forsomez

→ x and then takes further subsequences



and x



. In our terminology, we should ﬁrst take subsequence U

ε(j)

as in Step

1 of our proof.

(iii) Similar statements for lower semicontinuous U

(with values in R ∪{+∞})

are obtained by replacing

U by U = lim inf

∗

, U(z) <M by U(z) >M and a

maximum point by a minimum point. This assertion follows by Lemma 2.2.5 by

replacing U

by −U

. Also, we may replace a maximum point by a minimum point

in Remark 2.2.6 (i).

2.2.3 Applications

Proof of Proposition 2.2.3. (i) As in Proposition 2.2.2 we may interpret the maxi-

mum in (2.1.6) as a local maximum for each class of test functions. To show the ﬁrst

part it suﬃces to prove (2.1.7) (with lower semicontinuous F )forϕ ∈ C

(O)sat-

isfying (2.1.6) by assuming that (2.1.7) holds for all z ∈Oand all ψ ∈∩

∞

k=2

(O)

such that u

∗

− ψ takes a local maximum at z.Sinceϕ is C

there is a modulus ω

that satisﬁes

Φ=ϕ(x, t) − ϕ(ˆx,

t) − ϕ

(ˆx,

t)(t −

t) −∇ϕ(ˆx,

t),x− ˆx

−

∇

ϕ(ˆx,

t)(x − ˆx),x− ˆx

≤ ω

(|x − ˆx|

+ |t −

t|)(|x − ˆx|

+ |t −

t|)

for (x, t) suﬃciently close to (ˆx,

t). The right-hand side is dominated from above

2ω

(2|x − ˆx|

)|x − ˆx|

for |x − ˆx|

≥|t −

t|,

2ω

(2|t −

t|)|t −

t| for |x − ˆx|

≤|t −

t|.

The left-hand side Φ is now dominated by

Φ ≤

(|x − x|

)|x − ˆx|

+ ω

(|t −

t|)|t −

with

(σ)=2ω

(2σ). Applying Lemma 2.1.9 (ii) yields the existence of θ

∈

[0, ∞)andθ

∈ C

[0, ∞)withθ

(j)

(0) = 0 for 0 ≤ j ≤ k that satisﬁes

Φ ≤ θ

(|x − ˆx|)+θ

(|t −

t|)