Durst F. Fluid Mechanics: An Introduction to the Theory of Fluid Flows
Подождите немного. Документ загружается.

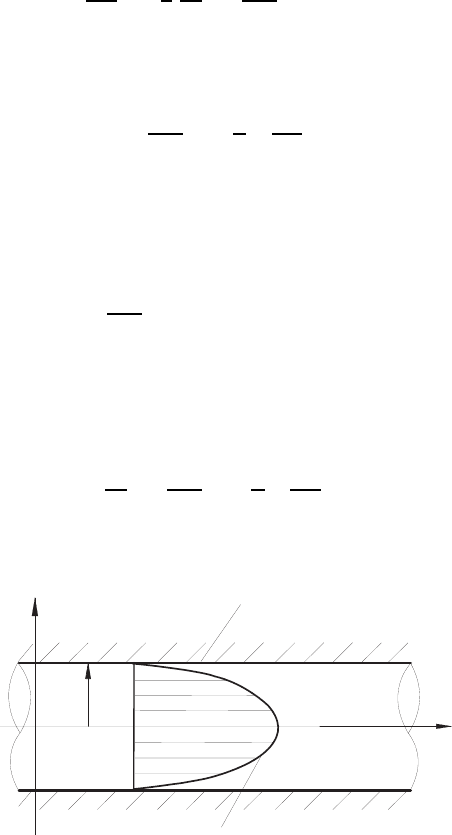
13.7 Pipe Flow (Hagen–Poiseuille Flow) 379
13.7 Pipe Flow (Hagen–Poiseuille Flow)
The laminar fully developed pipe flow is another important fluid flow which
can be treated as stationary, one-dimensional flow, i.e. by solving the following
differential equation:
−
dΠ
dz
+ µ
1
r
d
dr
r
dU
z
dr
+ ρg
z
=0. (13.94)
When considering the horizontal pipe flow as indicated in Fig. 13.9, the
following simplified differential equation holds, as g
z
=0:
r
dU
z
dr
=
1
µ
dΠ
dz
r. (13.95)
This equation expresses the fact that the external pressure gradient im-
posed on the fluid is maintained in equilibrium by viscous forces acting also
on the fluid, so that a non-accelerated flow results.
The boundary conditions for this flow are:
r =0;
dU
z
r
= 0 and for r = R; U
z
=0.
The flow occurring in the cylindrical pipe indicated in Fig. 13.9 requires a
pressure gradient to be maintained in the developed state ( dΠ/z), i.e. this
quantity has to be applied externally for a pipe flow to be established. For
the resultant flow velocity one obtains the following differential equation:
d
dr
r
dU
z
dr
=
1
µ
dΠ
dz
r. (13.96)
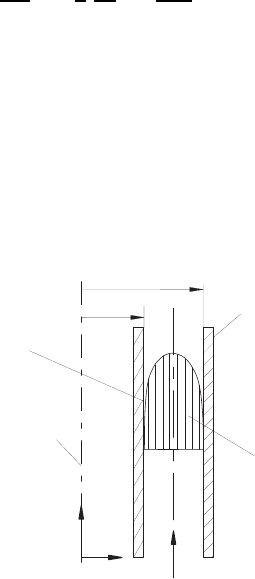
r
z
Pipe wall
Parabolic velocit
y
profile
R
Fig. 13.9 Laminar flow in a pipe
380 13 Incompressible Fluid Flows
By a first integration of (13.96), the following results:
dU
z
dr
=
1
2µ
dΠ
dz
r +
C
1
r
. (13.97)
By a second integration one obtains:
U
z
=
1
4µ
dΠ
dz
r
2
+ C
1
ln r + C
2
. (13.98)
Applying the boundary conditions:
r → 0;
dU
z
dr
→ 0andr = R : U
2
=0. (13.99)
C
1
and C
2
can be determined:
C
1
=0 and C
2
= −
1
4µ
dπ
dz
R
2
. (13.100)
Thus the equation for the velocity distribution U
z
(r) for the laminar pipe
flow reads:
U
z
= −
R
2
4µ
dΠ
dz
1 −
r
R
2
. (13.101)
The velocity profile is parabolic and U
z
is positive; the minus sign takes into
account the presence of a negative pressure gradient in the z direction, i.e.
the pressure decreases in the +z direction and the fluid thus flows in that
direction.
The volume flow through the pipe (volume per unit time) can be
computed as follows:
˙
Q =
R
#
0
2πrU
z
dr = −
πR
4
8µ
dΠ
dz
(13.102)
or rewritten:
dΠ
dz
=
∆p
∆z
= −
8µ
˙
Q
πR
4
. (13.103)
In the case of a laminar pipe flow, the pressure drop per unit pipe length is
proportional to the dynamic viscosity of the flowing fluid and the volume flow
rate, as well as inversely proportional to the fourth power of the pipe radius.
The mean velocity results as:
˜
U =
˙
Q
πR
2
= −
R
2
8µ
dΠ
dz
. (13.104)
The above connection between the volume flow, the inner radius R of the pipe,
the viscosity of the flow medium and the resultant pressure gradient is known
13.7 Pipe Flow (Hagen–Poiseuille Flow) 381
as the Hagen–Poiseuille law. It was found by Hagen in 1839 and by Poiseuille
in 1840/41 independently of one another in experimental investigations. The
experimental confirmation of the above-derived relations stresses the validity
of the assumptions made for the pipe flow and beyond that the fact that the
validity of the Navier–Stokes equations for the description of fluid flows of
Newtonian media hold.
The momentum loss to the wall of the pipe, due to the laminar, fully
developed pipe flow, can be computed as
τ
w
= −µ
dU
z
dr
w
=
1
2
dΠ
dz
R. (13.105)
The friction coefficient can thus be calculated as follows:
c
f
=
τ
w
ρ
2
˜
U
2
=
2τ
w
(2R)
˜
Uµ
*
˜
U2R
ν
+
=
dΠ
dz
R(2R)
R
2
8
dΠ
dz
Re
=
16
Re
, (13.106)
i.e. we obtain the following functional relationship:
c
f
=
16
Re
with Re =
˜
U2R
ν
. (13.107)
The representation of the friction coefficient as function of the Reynolds num-
ber yields, in a diagram with double-logarithmic axes, a straight line with the
gradient (−1).
Further insight into the fluid flow and the molecule interactions, taking
place in viscous mediums, can be gained by computing the energy dissipation
in the pipe flow by the action of the fluid viscosity. Based on the general
relationship for the energy dissipation per unit volume in a Newtonian fluid,
one obtains:
dE
diss
dV
=2µ
3
∂U
r
∂r
2
+
1
r
∂U
∂ϕ
+
U
r
r
2
+
∂U
z
∂z
2
4
+µ
r
∂
∂r
U
ϕ
r
+
1
r
dU
r
dϕ
2
+ µ
1
r
∂U
z
∂ϕ
+
∂U
ϕ
∂z
2
+µ
∂U
r
∂z
+
∂U
z
∂r
2
. (13.108)
When considering all the simplifications which were introduced for the
derivation of (13.94), the above general relationship for the energy dissipation
of a viscous pipe flow can be described as follows:
dE
diss
dV
= µ
∂U
z
∂r
2
= µ
dU
z
dr
2
. (13.109)
382 13 Incompressible Fluid Flows
By introducing dV =2πr dz dr, one obtains
dE
diss
= µ
dU
z
dr
2
2πr dz dr. (13.110)
dU
z
/dr can be written as:
dU
z
dr
= −
1
2µ
dΠ
dz
r. (13.111)
Thus the dissipated energy per unit length of a pipe flow can be calculated
as:
dE
diss
dz
=
π
2µ
dΠ
dz
2
r
3
dr. (13.112)
On integrating this equation, one obtains the energy dissipated per unit pipe
length dz:
dE
diss
dz
=
π
2µ
dΠ
dz
2
R
#
0
r
3
dr =
π
8µ
dΠ
dz
2
R
4
, (13.113)
i.e. the pressure gradient that has to be applied per unit length of the pipe
serves for supplying the mechanical energy dissipated into heat, per unit
length of the fluid motion. Considering:
˙
Q =
πR
4
8µ
dΠ
dz
, (13.114)
(13.113) can be written as:
dE
diss
dz
=
˙
Q
dΠ
dz
or ∆E
diss
=
˙
Q∆P
diss
. (13.115)
This relationship expresses that the pressure gradient to be applied per
unit length of the pipe corresponds to the energy dissipated per unit length
of the pipe and per unit volume flow:
dΠ
dz
=
1
˙
Q
dE
diss
dz
. (13.116)
The validity of the above-derived relationships for the pipe flow is, however,
limited to laminar flows, i.e. to Reynolds numbers which are smaller than
Re
crit
. This critical Reynolds number is for pipe flows in the range
Re
crit
=
˜
U2R
ν
2.3to2.5 × 10
3
. (13.117)
When the Reynolds number of a pipe flow is larger than this critical value,
and when no special precautions are taken to keep flow perturbations away
13.8 Axial Flow Between Two Cylinders 383
from the pipe flow, then the flow in the range of the critical Reynolds number
changes abruptly from laminar to turbulent. In this case there is no longer
a directed flow present as described by the above relationships. The flow
in the pipe shows, superimposed on a mean flow field, stochastic velocity
fluctuations which lead to an additional momentum transport transverse to
the flow direction. This momentum transport is not covered by the above
basic equations.
The most important properties of turbulent pipe-channel flows are in-
dicated in Chap. 18 and some references are made to deviations from the
laminar pipe flow as discussed here.
13.8 Axial Flow Between Two Cylinders
In chemical engineering, there are a large number of axially symmetric appa-
ratus in which flows can be treated as stationary, fully developed flows. They
are described by the following partial differential equation:
−
dΠ
dz
+ µ
1
r
∂
∂r
r
∂U
z
∂r
+ ρg
z
=0. (13.118)
Annular axial flows are among them, of the kind sketched in Fig. 13.10;
the boundary conditions for this flow can be given as:
for r = R
1
: U
z
= 0 and for r = R
2
: U
z
=0.
As an interesting example, the flow in a cylindrical annular channel, as
shown in Fig. 13.10, will be discussed here. The annular channel is formed by
two axially positioned pipes having radii R
1
and R
2
.
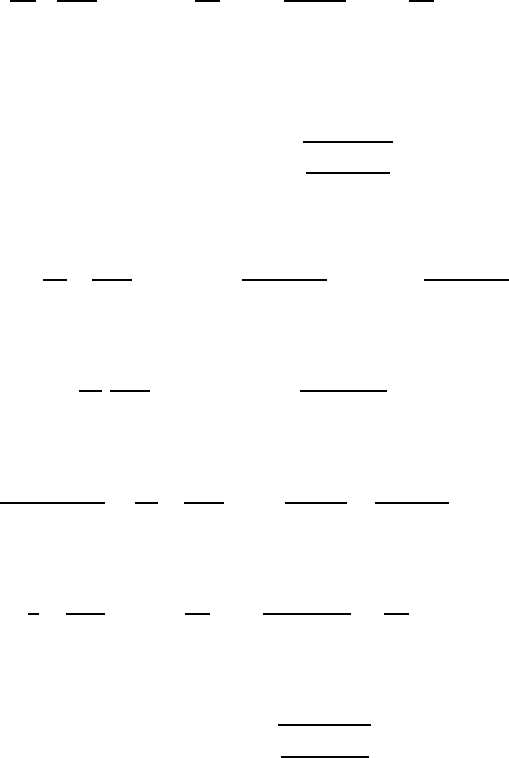
z
r
R
R
1
2
Inside wall
Axis
r = 0
Outside wall
Velocity
profile
m
.
Fig. 13.10 Upwards flow for a cylindrical annular clearance

384 13 Incompressible Fluid Flows
For further simplification of the derivation,
K = R
1
/R
2
,Π
∗
= Π + ρgz (13.119)
are introduced. Considering the coordinate system indicated in Fig. 13.10,
g
z
= −g holds and thus one obtains the following form of the differential
equation describing the annular channel flow of Fig. 13.10
µ
1
r
∂
∂r
r
∂U
z
∂r
=
∂
∂z
(Π + ρgz)=
∂Π
∗
∂z
. (13.120)
Taking into account the assumptions
∂
∂z
(···) = 0 for the flow field, i.e. as-
suming a fully developed flow in the z direction, the above-mentioned partial
differentials can be written as total differentials, as follows:
d
dr
r
dU
z
dr
=
1
µ
dΠ
∗
dz
r. (13.121)
In Sect. 13.6, it was shown that this equation has the following general
solution:
U
z
=
1
4µ
dΠ
∗
dz
r
2
+ C
1
ln r + C
2
. (13.122)
Based on the boundary conditions stated in Fig. 13.10, the integration con-
stants C
1
and C
2
for the flow in a cylindrical annular clearance can be
determined by (13.123) and (13.124).
From the boundary condition r = R
1
; U
z
= 0 results the first equation for
the computation of the integration constants C
1
and C
2
:
0=R
2
1
+ C
1
ln R
1
+ C
2
. (13.123)
On considering r = R
2
; U
z
=0,oneobtains:
0=R
2
2
+ C
1
ln R
2
+ C
2
, (13.124)
the second relationship for the computation of the integration constants C
1
and C
2
. In this way, one arrives at:
C
1
= R
2
2
3
1 −
R
1
R
2
2
4
1
ln (R
1
/R
2
)
(13.125)
or, considering K = R
1
/R
2
C
1
= R
2
2
(1 − K
2
)
1
ln K
. (13.126)
C
2
results as
C
2
= R
2
2
(K
2
− 1)
ln K
ln R
2
− 1. (13.127)
13.8 Axial Flow Between Two Cylinders 385
For the velocity distribution, the following equation results:
U
z
= −
R
2
2
4µ
dΠ
∗
dz
3
1 −
r
R
2
2
4
+
K
2
− 1
ln K
× ln
r
R
2
5
. (13.128)
The above equation shows that for K → 0 the velocity distribution for the
fully developed pipe flow is not obtained. The position of the maximum
velocity is computed as
r =(u
z
= u
max
)=R
z
$
1 − K
2
2ln(1/K)
. (13.129)
The maximum velocity is thus computed from the equation for U
z
(U
z
)
max
= −
1
4µ
dΠ
∗
dz
R
2
2
1 −
1 − K
2
2ln(1/K)
1 − ln
1 − K
2
2ln(1/K)
.
(13.130)
Thevolumeflowresultsas
˙
Q = −
π
∗
8µ
dΠ
∗
dz
R
4
2
!
1 − K
4
"
−
(1 − K
2
)
2
ln (1/K)
(13.131)
and for the mean velocity one obtains
˜
U
z
=
˙
Q
π (R
2
2
− R
2
1
)
=
1
8µ
dΠ
∗
dt
R
2
2
1 − K
4
1 − K
2
−
1 − K
2
ln (
1
/K)
. (13.132)
The molecular momentum transport can be computed as
τ
r,z
=
1
2
dΠ
∗
dz
R
2
r
R
2
−
1 − K
2
2ln(1/K)
R
2
r
. (13.133)
The quantity τ
r,z
is naturally at the position du
z
/dr = 0, i.e. at U
z
= U
max
,
equal to zero, so that one obtains from (13.133):
r (τ
r,z
=0)=R
2
$
1 − K
2
2ln(1/K)
. (13.134)
For the annular clearance it also holds that the above relationships can only
be employed for laminar flows. The additional momentum transports, occur-
ring in turbulent flows due to the turbulent velocity fluctuations, were not
taken into consideration in the above equations. Therefore, the derived equa-
tions in this section can be employed only when it has been confirmed that
the flow in the considered annular channel flows in a laminar way.
386 13 Incompressible Fluid Flows
13.9 Film Flows with Two Layers
The problems of steady, two-dimensional and fully developed flows of incom-
pressible fluids, discussed in the previous chapters, can be extended to fluid
flows that comprise of several non-mixable fluids. The derived basic equations
for fully developed flows have to be solved, in the presence of several fluids,
for each fluid flow and the boundary conditions existing in the inter-layers of
the fluids have to be considered in the solutions. This is shown below for a
film flow made up of two layers.
In coating technology, it is customary to insert superimposed film flows
of non-mixable fluids in order to coat several films, in one process step, on
to a substrate. In practice, up to 20 layers can be simultaneously applied
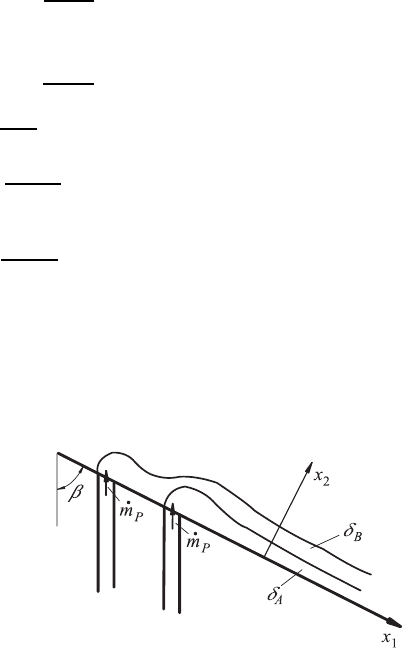
with high accuracy. If one limits oneself to two layers, flow configurations as
shown in Fig. 13.11 develop. The figure shows two superimposed film flows
which are moved by gravitation on top of a plane inclined wall. Flows of this
kind are described by the following differential equations:
0=µ
A
d
2
U
A
1
dx
2
2
+ ρ
A
g
1
(13.135)
and
0=µ
B
d
2
U
B
1
dx
2
2
+ ρ
B
g
1
(13.136)
with g
1
= g cos β and ν
A,B
=
µ
A,B
ρ
A,B
, so that one obtains by integration:
U
A
1
= −
g cos β
2ν
A
x
2
2
+ C
A
1
x
2
+ C
A
2
(13.137)
and
U
B
1
= −
g cos β
2ν
B
x
2
2
+ C
B
1
x
2
+ C
B
2
. (13.138)
By this integration, four integration constants were introduced in the above
relationships which have to be determined by appropriate boundary con-
ditions:
x
2
=0 ; U
A
1
= 0 (no-slip wall condition) ; C
A
2
=0, (13.139)
Fig. 13.11 Flow between fluid films
on top of a plane, inclined wall

13.9 Film Flows with Two Layers 387
x
2
= δ
A
+ δ
B
;
dU
B
1
dx
2
= 0 (free surface), (13.140)
x
2
= δ
A
; U
A
1
= U
B
1
and also µ
A
dU
A
1
dx
2
= µ
B
dU
B
1
dx
2
. (13.141)
From the boundary condition for the free surface, C
B
1
results as:
C
B
1
=
g cos β
ν
B
(δ
A
+ δ
B
) . (13.142)
The equality of the local film velocities in the common interface between the
films yields:
−
g cos β
2ν
A
δ
2
A
+ C
A
1
δ
A
= −
g cos β
2ν
B
δ
2
A
+
g cos β
ν
B
(δ
A
+ δ
B
) δ
A
+ C
B
2
. (13.143)
The equality of the local momentum transport terms in the common interface
between the films further yields:
−δ
A
g cos βδ
A
+ C
A
1
= −ρ
B
g cos βδ
A
+ ρ
B
g cos β(δ
A
+ δ
B
). (13.144)
From the above equation, one can deduce:
C
A
1
=(ρ
A
δ
A
+ ρ
B
δ
B
)g cos β (13.145)
and for C
B
2
,oneobtains:
C
B
2
= −g cos β
ν
A
+ ν
B
ν
A
ν
B
δ
2
A
+(ρ
A
δ
A
+ ρ
B
δ
B
) g cos β −
g cos β
ν
B
(δ
A
+ δ
B
) δ
A
.
(13.146)
Thus, one obtains for the velocity distributions U
A
1
and U
B
1
:
U
A
1
= −
g cos β
2ν
A
x
2
2
+[(ρ
A
δ
A
+ ρ
B
δ
B
) β] x
2
for 0 ≤ x
2
≤ δ
A
(13.147)
and
U
B
1
= −
g cos β
2ν
B
x
2
2
+
g cos β
2ν
B
(δ
A
+ δ
B
) x
2
−g cos β
ν
A
+ ν
B
2ν
A
ν
B
δ
2
A
+(ρ
A
δ
A
+ ρ
B
δ
B
) g cos β
−
g cos β
ν
B
(δ
A
+ δ
B
) δ
A
for δ
K
≤ x
2
≤ δ
B
. (13.148)
For ˙m
A
and ˙m
B
,wecanwrite:
˙m
A
= ρ
A
B
δ
A
#
0
U
A
1
(x
2
)dx
2
and ˙m
B
= ρ
B
B
(δ
A
+δ
B
)
#
δ
A
U
B
1
(x
2
)dx
2
. (13.149)
388 13 Incompressible Fluid Flows
By integration one obtains
˙m
A
= ρ
A
B
−
g cos β
2ν
A
δ
3
A
3
+ C
A
1
δ
2
A
2
. (13.150)
˙m
B
= ρ
B
B
3
−
g cos β
2ν
B
(δ
A
+ δ
A
)
3
− δ
3
A
3
+ C
B
1
δ
A
δ
B
+ δ
2
B
2
+ C
B
2
δ
B
4
.
(13.151)
In this way, the layer mass flows ˙m
A
and ˙m
B
can be determined, when δ
A
and δ
B
are given and the properties of the fluids of the coating fluids are
known.
13.10 Two-Phase Plane Channel Flow
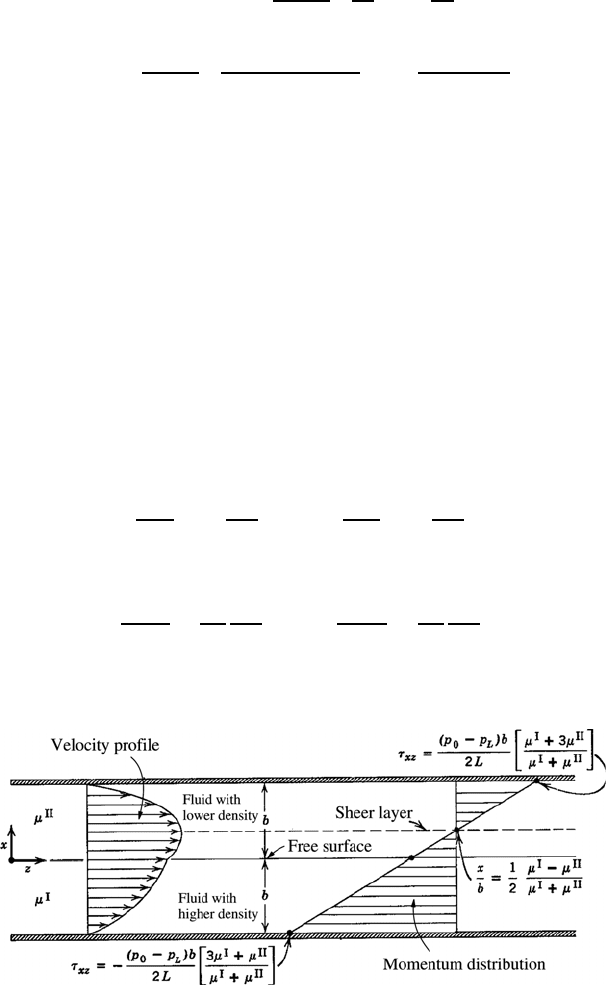
In Fig. 13.12, a plane channel flow is sketched which is composed of the flow
of two superimposed non-mixable fluids, i.e. fluids A and B that flow simul-
taneously through a channel formed by two parallel plates. Fluid A forms a
layer of thickness δ
A
and has density ρ
A
,viscosityµ
A
and mass flow ˙m
A
.
The fluid that is on top of it has the density ρ
B
,viscosityµ
B
and mass flow
˙m
B
. For both fluids, the following differential equations for the molecular
momentum transport τ
21
hold:
dτ
A
21
dx
2
= −
dΠ
dx
1
and
dτ
B
21
dx
2
= −
dΠ
dx
1
. (13.152)
With τ
21
= −µ dU
1
/x
2
the velocity field results
d
2
U
A
1
dx
2
2
=
1
µ
A
dΠ
dx
1
and
d
2
U
B
1
dx
2
2
=
1
µ
B
dΠ
dx
1
. (13.153)
Integration of (13.152) yields for both fluids
Fig. 13.12 Plane channel flow with two-layered flows; a solution is stated for δ =0
