Durst F. Fluid Mechanics: An Introduction to the Theory of Fluid Flows

Подождите немного. Документ загружается.

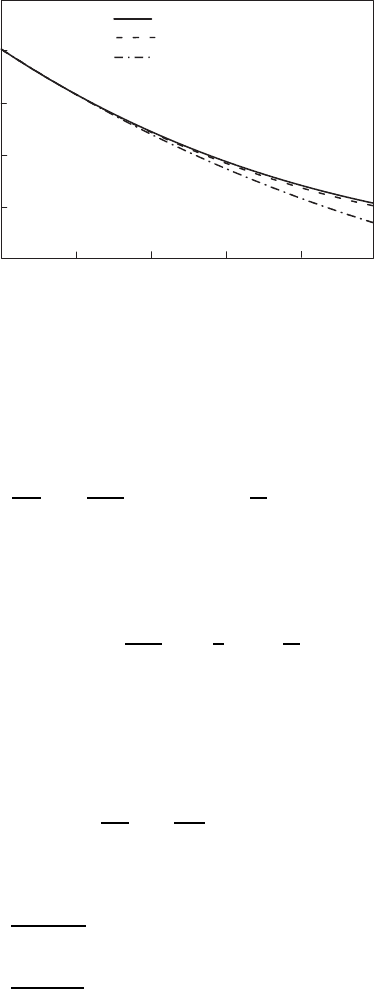
186 6 Hydrostatics and Aerostatics

200

400

600

800 1000

125

100

Standard pressure distribution

Pressure by equation (6.98)

Pressure by equation (6.

104)

Hei

/10 [m]

Pressure

/10 [mbar]

Fig. 6.28 Standard pressure distribution in the atmosphere and distributions

computed on the basis of approximate equation (6.98)

or the following approximate equation by neglecting the terms of higher order:

= −





α −





(6.97)

By solving this equation, the following ﬁnal relationship results for the

pressure distribution in the lower atmosphere:

= P

exp



−





α −





(6.98)

As Fig. 6.28 shows, this pressure relationship describes, with good preci-

sion, the standard pressure distribution existing in the atmosphere.

The approximate equations stated by Babinet for the pressure distribution

in the atmosphere can be derived from the general diﬀerential equation

= −

ˆg

(6.99)

by introducing the following hypotheses:

P =

+ P

= constant ˆg = g = constant

T =

+ T

= constant

(6.100)

When one introduces these simpliﬁcations, the following solution for the

diﬀerential equation (6.99) results:

6.4 Aerostatics 187

+ P

− P

)=−

(6.101)

or resolved:

− P

+ P

= −

R (T

+ T

)

(6.102)

H = −





− P

+ P



(6.103)

When one rearranges this equation to obtain P

relative to P

= P





− H





+ H

(6.104)

Figure 6.28 shows the pressure distribution by the two relationships (6.98)

and (6.104) in comparison with the standard pressure distribution in the

atmosphere.

6.4.2 Rotating Containers

As a further example of the employment of the basic equation of aerostat-

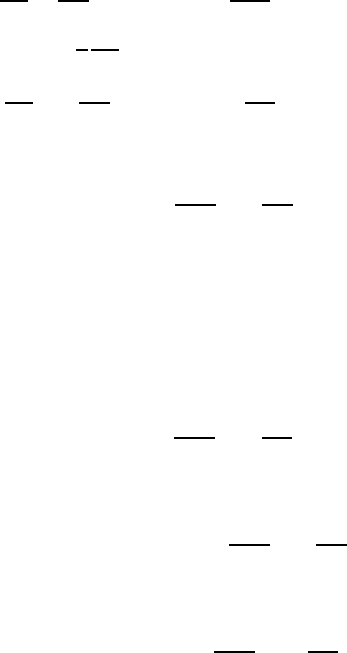
ics, the problem indicated in Fig. 6.29 will be considered. By a single pressure

measurement in the upper center point of the cylinder top surface and by em-

ployment of the partial diﬀerential equation of aerostatics, the entire pressure

The pressure distribution

n rotating containers can

become rather complex

Fig. 6.29 Rotating cylinder with a compressible medium

188 6 Hydrostatics and Aerostatics

load on the circumferential surface of a rotating cylinder, ﬁlled with a com-

pressible ﬂuid, can be deﬁned. In contrast to the example treated in Sect. 6.1,

here the cylinder will experience a pure rotational motion only, so that the

partial diﬀerential equations of aerostatics can be written for T = constant

as follows:

∂P

∂r

rω

−→ ln P =

2RT

+ F (ϕ, z) (6.105)

∂P

∂ϕ

=0−→ P = F (r, z) (6.106)

∂P

∂z

= −

g −→ ln P = −

z + F (r, ϕ) (6.107)

By comparing the solutions one obtains for the logarithm of the pressure:

ln P = C +

2RT

−

z (6.108)

The pressure P

, measured at the coordinate origin, permits the deﬁnition

of the integration constant C as:

C =ln P

(6.109)

Introducing C into the (6.108), one obtains the pressure distribution. The

pressure P is given by:

P = P

exp



2RT

−



(6.110)

Along the ﬂoor space z = −L the pressure distribution is computed as:

P (r, z = − L)=P

exp



2RT



(6.111)

Along the vertical circumferential surface r = R:

P (r = R, z)=P

exp



2RT

−



(6.112)

Pressure measurements show a very good agreement of P (R, z)ofequation

(6.112) with the experiments.

6.4.3 Aerostatic Buoyancy

When employing aerostatic laws, mistakes are often made by using relations

that are valid strictly only in hydrostatics. An example is the buoyancy of

bodies, which is computed according to Fig. 6.30 as the diﬀerence in the

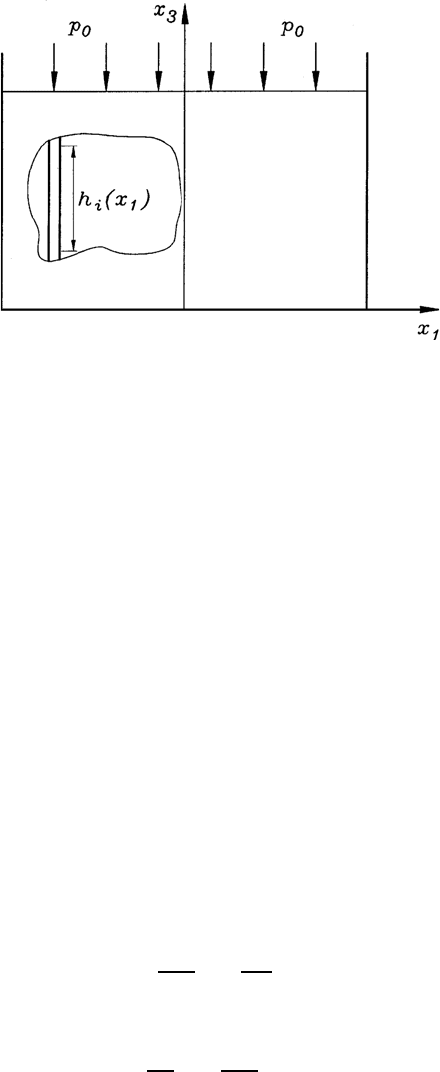
6.4 Aerostatics 189

Fig. 6.30 Illustration of the hydrostatic buoyancy of immersed bodies

pressure forces on the lower and upper sides of a immersed body. The basic

equations for these computations are stated in Fig. 6.30. They lead to the

generally known relation which expresses that the buoyancy force experienced

by a body immersed in a ﬂuid is equal to the weight of the ﬂuid displaced

by the body. The derivations on the basis of Fig. 6.30 make it clear that the

simple relationship holds only for ﬂuids with ρ = constant.

For the buoyancy force on a body element:

∆A

= ∆P

∆F

∆P

= ρ

)

Thus for the buoyancy force:

A =



) ∆F

= ρ

When one wants to compute the buoyancy forces in gases, the laws of

aerostatics have to be employed, i.e. the derivations shown in Fig. 6.30 are to

be modiﬁed as follows.

When one applies the basic equations (6.87) to an “isothermal atmo-

sphere”, in which the axis x

of a rectangular Cartesian coordinate system

points in the upward direction, one obtains

= −

g (6.113)

When one sets g = constant and T = T

= constant, one obtains

= −

(6.114)

190 6 Hydrostatics and Aerostatics

or when integrated:

= −

(6.115)

In this relation, the pressure P

prevails at the level x

=0.Forthe

pressure path in an isothermal atmosphere, one can therefore write:

P = P

exp



−



(6.116)

Employing now again the relation for the buoyancy occurring on a body

element:

∆A

= ∆P

∆F

(6.117)

and rewriting using (6.116), we obtain:

∆P

= P

exp



−



− P

exp



−

+ h

)



(6.118)

i.e.

∆P

= P

exp



−



1 − exp



−



(6.119)

and it quickly becomes evident that, for the total buoyancy in the present

case, a simple relation stated for ρ = constant for the total buoyancy force

does not result. The Taylor series expansion for P

can be written as:

∆P

∂P

∂x

∂

∂x

∂

∂x

+ ... (6.120)

= −

exp



−



exp



−



− (6.121)

∆P

≈ ρ (x

) gh

1 −

2RT





− + ...

(6.122)

Thus one obtains for the buoyancy force:

A  ρ (x

) g

V −



i=1

∆F

2RT

+ −...

(6.123)

The ﬁrst term in (6.123) corresponds to the buoyancy force of hydrostatics.

The second terms takes the compressibility of the considered gas into account.

6.4 Aerostatics 191

6.4.4 Conditions for Aerostatics: Stability of Layers

A ﬂuid can ﬁnd itself in mechanical equilibrium (i.e. show no macroscopic

motion) without being in thermal equilibrium. An equation similar to (6.7),

for the condition of mechanical equilibrium, can also be given when the tem-

perature in the ﬂuid is not constant. Here the question arises, however, of

whether an established mechanical equilibrium is stable. It appears from

simple considerations that the equilibrium shows the stability only under

certain conditions. When this condition is not met, the static state of the

ﬂuid is unstable and in the ﬂuid ﬂows of random motions occur, which

tend to mix the ﬂuid such that the condition of constant temperature is

achieved. This motion is deﬁned as free convection. The stability condi-

tion for mechanical equilibrium is, in other words, the condition for the

absence of natural convection for a stratiﬁed ﬂuid. It can be derived as

follows.

We consider a ﬂuid at a level z andwithaspeciﬁcvolumev(P, s), where

P and s are the equilibrium pressure and the equilibrium entropy of the

ﬂuid at this level. We assume that the considered ﬂuid element is displaced

upwards by a small stretch ξ adiabatically. Its speciﬁc volume thus becomes

v(P



,s), where P



is the pressure at the level z + ξ. For the stability of the

chosen equilibrium state it is necessary (although in general not suﬃcient)

that the force occurring here drives the element back to the starting position.

The considered volume element therefore has to be heavier than the ﬂuid in

which it was displaced into the new position. The speciﬁc volume of the ﬂuid

is v(P



), where s



is the equilibrium entropy of the ﬂuid at the level z + ξ.

Thus we have as a stability condition

v(P



) − v(P



,s) > 0

This diﬀerence is expanded in powers of s



− s =

ξ and we obtain



∂v

∂s



∂s

∂z

> 0 (6.124)

In accordance with thermodynamic relations, because T ds =dh −v dP ,the

following holds, namely:



∂v

∂s





∂v

∂T



being the speciﬁc heat at constant pressure. The speciﬁc heat c

is always

positive like the temperature T and therefore we can transform (6.124) into



∂v

∂T



> 0 (6.125)

192 6 Hydrostatics and Aerostatics

Most materials expand with warming, i.e.

∂v

∂T

> 0. The condition for the

absence of the convection is then reduced to the inequality:

> 0 (6.126)

i.e. the entropy has to increase with the level.

From this, one can easily ﬁnd a condition for the temperature gradient

dT/dz. From the derivation of ds/dz we can write



∂s

∂T





∂s

∂p



−



∂V

∂T



> 0

Finally, we insert dP/dz = −ρg according to (6.7) and obtain:

> −

gTρ



∂V

∂T



(6.127)

Hence convection will occur when the temperature in the direction from top

to bottom decreases and the temperature gradient is larger in value than

∂v

∂T

When one investigates the equilibrium of a gas column, one can assume the

gas stratiﬁcation for (6.127) to be fulﬁlled. The limiting value

∂V

∂T

exists. Hence the stability condition for the equilibrium simply reads

> −

(6.128)

When this stability requirement in the atmosphere is not met, the established

temperature stratiﬁcation is unstable and it will give way to a convective

temperature-driven ﬂow as soon as the smallest disturbances occur. The

motion will eliminate the unstable stratiﬁcation.

References

6.1. Schade, H., Kunz, E., Flow Mechanics, Walter de Gruyter, Berlin, 1989.

6.2. Potter, M.C., Foss, J.F., Fluid Mechanics, Wiley, New York, 1975.

6.3. Hutter, K., Fluid- and Thermodynamics, Springer, Berlin Heidelberg Newyork,

1995.

6.4. Pnueli, D., Gutﬁnger, C., Fluid Mechanics, Cambridge University Press,

Cambridge, 1992.

6.5. Spurk, J.H., Stroemungslehre, Springer, Berlin, Heidelberg Newyork, 1996.

6.6. H¨oﬂing, O., Physik: Lehrbuch f¨ur Unterrichtund Selbststudium, D¨ummler-

Verlag, Bonn, 1994.

6.7. Yuan, S.W., Foundations of Fluid Mechanics, Prentice-Hall, Taiwan, 1971.

6.8. Bergmann, L., Schaefer, C.-L., Lehrbuch der Experimentalphysik I: Mechanik-,

Akustik-, Waermelehre, Walter de Gruyter, Berlin, New York, 1961.

Chapter 7

Similarity Theory

7.1 Introduction

The knowledge gained from similarity theory is applied in many ﬁelds of nat-

ural and engineering science, among others in ﬂuid mechanics. In this ﬁeld,

similarity considerations are often used for providing insight into the ﬂow

phenomenon and for generalization of results. The importance of similarity

theory rests on the recognition that it is possible to gain important new

insights into ﬂows from the similarity of conditions and processes without

having to seek direct solutions for posed problems. This will be known to

most readers of this book from geometry, where geometrically similar ﬁgures

and bodies, e.g. similar triangles, are introduced and employed extend con-

siderations. In this way, the height of a tower or the width of a river can,

for example, be found by means of similarity considerations, without directly

determining the height or the width. From similarity considerations in the

ﬁeld of geometry, it is known that a suﬃcient condition for the presence

of geometrically similar triangles, quadrangles, etc., is the equality of corre-

sponding angles or equality of the ratios of the corresponding sides, i.e. it

holds for similar triangles:

= constant. (7.1)

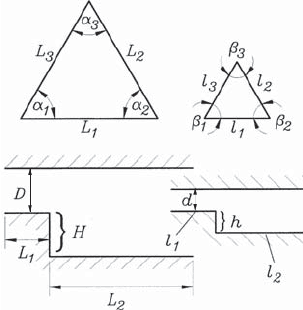
For a channel with a step:

= constant. (7.2)

ensures geometrical similarity (see Fig. 7.1). The geometric similarity of ﬂuid

ﬂow boundaries is always an important assumption for extended similarity

considerations in ﬂuid mechanics, i.e. the geometric similarity, is an inherent

part of the subject of this chapter.

The similarity relationships indicated above for the ﬁeld of geometry can

be transferred to other domains, e.g. to mathematics and physics, and can

193

194 7 Similarity Theory

Fig. 7.1 Explanation of geometric

similarity

Similar triangles

Similarity of backward

flowing steps

be introduced in engineering science with the objective of achieving indirect

solutions for problems. A similar methodology can be applied to obtain the

kinematic similarity of ﬂows, which is considered as given when, e.g. two

ﬂuid ﬂows exhibit similar spatial motions. This requires, in general, simi-

larity of the forces acting on individual ﬂuid elements, i.e. the existence of

dynamic similarity is a precondition for kinematic similarity of ﬂuid motions.

More detailed considerations show, in addition, that a further prerequisite for

kinematic similarity of ﬂuid motions is geometric similarity and the presence

of similar boundary conditions.

When one extends the similarity considerations also to heat transport

problems, it makes sense to introduce the term thermal similarity. Here again,

similar temperature ﬁelds can be expected only when similar heat ﬂows exist

in the considered temperature ﬁelds, i.e. when the caloric similarity of the

considered heat-transport problems is given. However, it is important here

to separate the thermal and caloric similarity from one another.

In similarity considerations, strictly, only quantities with the same physical

units can be included. The “dimensionless proportionality factors” of the dif-

ferent terms of a physical relationship computed from it by dividing all terms

by one term in the equation are designated similarity numbers or dimen-

sionless characteristic numbers of the physical problem. Physical processes of

all kinds can thus be categorized as similar only when the corresponding di-

mensionless characteristic numbers, deﬁning the physical problem, are equal.

This requires, in addition, that geometric similarity exists and the boundary

conditions for the considered problems are similar. The concept of similarity

can therefore only be applied to physical processes of the same kind, i.e. to

ﬂuid ﬂows or heat transport processes separately. When certain relationships

apply both to ﬂow processes and to heat transfer process, one talks of an

analogy between the two processes.

7.1 Introduction 195

The above general considerations on theoretical similarity deliberations in

ﬂuid mechanics make it clear that two fundamentally diﬀerent process exist

concerning the solution of ﬂow problems:

• Solutions of concrete ﬂow problems using dimensional physical quantities

permit ﬂow systems with all parameters to be considered providing a so-

lution of a speciﬁc ﬂow problem. Hence a solution with all dimensions of

the problem is provided.

• Generalization of solutions of ﬂow problems, with the help of dimensionless

“characteristic numbers”, in order to present the solution obtained for a

speciﬁc ﬂow problem as a generally valid solution for similar ﬂows.

In Chap. 2, physical quantities were introduced that consisted of a sign, a

numerical value and a unit. It was made clear that according to the chosen

system of basic units, the magnitude of a considered physical quantity can

vary. When one chooses the SI system as a basis of units (m, kg, s) for

some mechanical quantities, other numerical values result for the value of a

considered quantity then when the basic units (cm, g, s) are introduced, as is

sometimes still the case. The international system of basic units, comprising

the following units, is gaining more and more acceptance, however:

• Length L (m = metre)

• Time t (s = second)

• Amount of substance N (mol = mole)

• Light intensity S (cd = candela)

• Mass M (kg = kilogram)

• Temperature θ (K = kelvin)

• Current intensity I (A = ampere)

Thanks to the introduction of an international system of units, the commu-

nication of physical processes and, above all, the comparison of analytical,

numerical and experimental results have been simpliﬁed. Nevertheless, in

principle, there is a multitude of diﬀerent basic systems of units that can be

employed for the presentation of the same physical quantity. With each sys-

tem of units the considered physical quantity has another “absolute value”,

i.e. the physical quantity changes its numerical value according to the chosen

system of units. This indicates the signiﬁcance of the Table 7.1 of dimensions

and units of the most important physical quantities in ﬂuid mechanics. At

the same time the Table 7.1 points out the principle diﬀerences between the

dimensions and units of physical quantities.

In the presentation of ﬂuid mechanics, in this book, transport processes by

molecular motions (diﬀusive transport) or by ﬂows (convective transport) are

of particular interest. In order to guarantee the similarities between the trans-

port processes, diﬀerent dimensionless characteristic numbers are employed,

which are subdivided below into four groups: