Durst F. Fluid Mechanics: An Introduction to the Theory of Fluid Flows

Подождите немного. Документ загружается.

166 6 Hydrostatics and Aerostatics

6.2.2 Pressure-Measuring Instruments

The insights into the pressure distribution in containers gained in Sect. 6.2.1

were obtained through pressure relationships that were described for commu-

nicating systems. From the derived relationships for the pressure distribution

in the containers, information could be obtain for the location of the free

liquid surfaces. In return, it is possible, in the case that the established

ﬂuid levels are known, to employ the general pressure relationships, indi-

cated in Sect. 6.1, in order to obtain information on the pressures occurring

in containers.

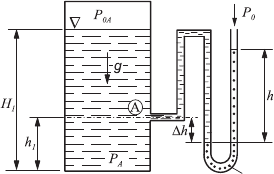
This is illustrated in Fig. 6.10, which shows a sketch for explaining the

basic principle according to which pressure measurements are carried out by

communicating systems.

To measure the pressure at point A in the container to which a “U-tube

manometer” is connected, the latter is ﬁlled with a measuring liquid (dotted

part of the U tube) and partly also with the liquid which enters into the U

tube from the container. The two liquids are assumed not to mix. For the

location of the separating plane between the two ﬂuids, the following pressure

equilibrium holds:

+ ρ

g∆h = p

+ ρ

gh (6.47)

For the pressure to be measured at point A, it follows that:

= p

+ ρ

gh − ρ

g∆h (6.48)

Equation (6.48) makes it clear that it is possible to determine the pressure

at point A in the container by measurements of h and ∆h when the ﬂuid

densities ρ

and ρ

are known. In Fig. 6.10, it was assumed that the pressure

in the container is high compared with the ambient pressure p

. When there

is a negative pressure in the container with respect to the outside pressure,

the conditions presented in Fig. 6.11 will exist for the ﬂuid level in the U-tube

manometer.

Thus, for the pressure equilibrium at the parting surface of the two ﬂuids,

the following relationship holds:

− ρ

g∆h = p

− ρ

gh (6.49)

Fig. 6.10 Diagram for explaining the ba-

sic principle of pressure measurements by

communicating systems

Measuring

Liquid

6.2 Connected Containers and Pressure-Measuring Instruments 167

Measuring

liquid

Fig. 6.11 Fluid columns in the U-tube manometer for “negative pressure” giving an

upward rise of the measuring liquid

Fig. 6.12 Basic principle of barometric pressure

measurements

Vacuum

Plain

For the pressure at point A one then obtains the following expression:

= p

− ρ

gh + ρ

g∆h (6.50)

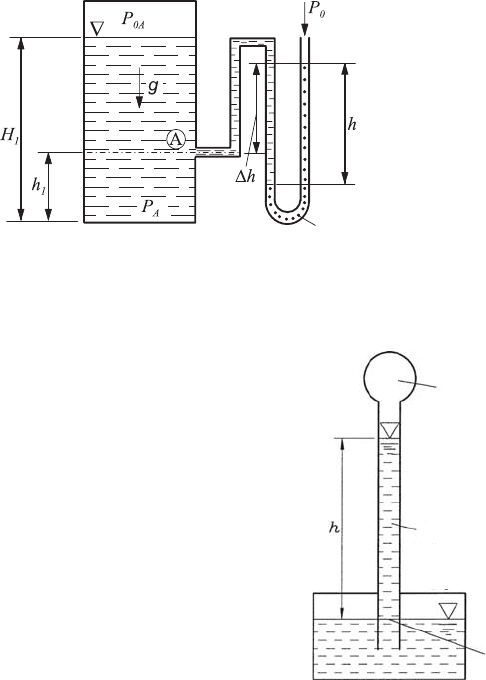
On the basis of communicating containers, measuring devices can also be

designed and employed to measure the atmospheric pressure, i.e. to carry out

barometric measurements. To explain this, reference is made to the pressure

measuring equipment shown in Fig. 6.12.

A system can in principle be produced as follows:

(a) A glass tube with a length of more than 1 m is ﬁlled to the top with

mercury; at the lowest end of the tube a spherical extension of the tube

section has been made.

(b) The glass tube, ﬁlled with mercury, is turned upside down and its lower

end is displaced into a container also ﬁlled with mercury. This needs to be

achieved without mercury running out of the tube, as shown in Fig. 6.12.

168 6 Hydrostatics and Aerostatics

the mercury in the external container is a measure of the barometric

pressure:

= ρ

gh (6.51)

A barometer, as shown in Fig. 6.12, can be employed to verify experimentally

the pressure distributions in the atmosphere stated in Sect. 6.4.1.

6.3 Free Fluid Surfaces

6.3.1 Surface Tension

In Chap. 1, it was emphasized that a special characteristic of ﬂuids is that, in

contrast to solids, they have no form of their own, but always adopt the form

of the container in which they are stored. While doing this, a free surface

forms and it was shown in Sect. 6.1 that this surface adopts a position which

is perpendicular to the vector of the gravitational acceleration.

In this way, the ﬂuid properties under gravitational inﬂuence were for-

mulated which are known from phenomena of every day life. It was always

assumed that the ﬂuid at disposal possesses a total volume having the same

order of magnitude as the larger container in which it is stored. The ﬂuid

properties hold only when these conditions are met. This is known from

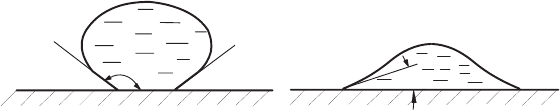
observations of small quantities of liquids which form drops when put on

surfaces, as sketched in Fig. 6.13. It is seen that diﬀerent shapes of drops

can form, depending on which surface and which ﬂuid for forming drops are

used. More detailed considerations show, moreover, that the gas surrounding

the ﬂuid and the solid surface all have an inﬂuence on the shape of a drop.

The latter is often neglected and one diﬀerentiates considerations of ﬂuid–

solid combinations with reference to their “wetting possibility”, depending

on whether the angle of contact established between the edge of the ﬂuid

surface and solid surface is smaller or larger than π/2.

The surface is classiﬁed as non-wetting by the ﬂuid when

>π/2 (6.52)

(a)

(b)

Fig. 6.13 (a) Shape of a drop in the case of non-wetting ﬂuid surfaces; (b)shapeof

a drop in the case of wetting ﬂuid surfaces

6.3 Free Fluid Surfaces 169

It holds furthermore that for

<π/2 (6.53)

the surface is classiﬁed as wetting for the ﬂuid.

Surfaces covered by a layer of fat are examples of surfaces that cannot be

wetted by water. Cleaned glass surfaces are to be classiﬁed as wetting for

many ﬂuids.

The above phenomena can be explained by the fact that diﬀerent “actions

of forces” are experienced by ﬂuid elements. Equivalent physical consider-

ations can be made also by referring to the surface energy that can be

attributed to free ﬂuid surfaces. The equivalence of forces and energy consid-

erations in mechanical systems is explained in Sect. 5.5. When a ﬂuid element

is located in a layer that is far away from a free ﬂuid surface, it is surrounded

from all sides by homogeneous ﬂuid molecules and one can assume that the

cohesion forces occurring between the molecules cancel each other. This is,

however, no longer the case when one considers ﬂuid elements in the prox-

imity of free surfaces. As the forces exerted by gas molecules on the water

particles are negligible in comparison with the interacting forces of the liq-

uid, a particle lying at the free surface experiences an action of forces in the

direction of the ﬂuid. “Lateral forces” also act on the ﬂuid element, which

thus ﬁnds itself, in the considered free interphase surface, in a state of ten-

sion that attributes special characteristics to the free surface. It is therefore

possible, for example, to deposit carefully applied ﬂat metal components on

free surfaces without the metal piece penetrating the liquid. The ﬂoating of

razor blades on water surfaces is an experiment that is often presented in

basic courses of physics to demonstrate this. In nature, animals like “pond

skaters” make use of this particular property of the water surface in order to

cross pools and ponds skillfully and quickly.

When a drop of liquid comes into contact with a ﬁrm support, attracting

forces also occur in addition to the internal interacting forces. When these

attracting forces are stronger than the internal forces that are typical for the

ﬂuid, we have the case of a wetting surface and water drops form as shown in

Fig. 6.13b. If, however, the forces attributed to a thin layer of the free surface

are stronger, we have the case of a non-wetting surface and the shapes of the

drops correspond to those in Fig. 6.13a.

More detailed considerations of the processes in the proximity of the free

surface of a liquid show that we have to deal with a complicated layer (with

ﬁnite extension vertical to the ﬂuid surface) from a liquid area to a gas area.

It suﬃces, for many considerations to be made in ﬂuid mechanics however,

to introduce the surface as a layer with a thickness δ → 0. To the same

are attributed the properties that comprise the complex layer between ﬂuid

and gas. The property that is of particular importance for the considerations

to be carried out here is the surface tension. This surface tension can be

demonstrated by immersing a strap, as shown in Fig. 6.14, in a ﬂuid. When

pulling the strap through the free surface upwards, one observes that this

170 6 Hydrostatics and Aerostatics

Bend wire frame

Liquid film

Free liquid surface

Fig. 6.14 Wire frame experiment to prove the action of forces as a consequence of

surface tension

requires an action of forces which is proportional to the distance between the

strap arms. The proportionality constant describing this fact is deﬁned as

the surface constant.

The surface tension thus represents a force of the free surface per unit linear

length. It can also be introduced as the energy that is required to build up

the tension in the liquid ﬁlm in Fig. 6.14. The two deﬁnitions are identical. In

both ways of looking at the energy equation, the length of the liquid ﬁlm in

the direction in which the wire frame is pulled can be understood as a process

to introduce energy to produce free surfaces. One can also look at the force

that is needed to pull the wire frame up to produce the free surface. This

makes it clear that both possibilities of introduction of the surface tension,

one as the action of forces per unit length and the other as the energy per

unit area, are identical.

In concluding these introductory considerations, the eﬀect of the surface

tension on the areas above and below a free surface will be looked at more

closely. From observations of free surfaces in the middle of large contain-

ers one can infer that the surface tension there has no inﬂuence on the

ﬂuid and the gas area lying above it, as the free surface forms vertically

to the ﬁeld of gravity of the earth, as stated in Fig. 6.1. From this it follows

that considerations of liquids with free surfaces can be carried out far away

from solid boundaries (container walls), without consideration of the wall

eﬀects.

When one considers a curved surface element, as shown in Fig. 6.15, one

understands that, as a consequence of the surface tensions that occur, actions

of forces are directed to the side of the surface on which the center points

of the curvatures are located. The forces acting on sides AD and BC of the

surface element are computed for each element ds

and the forces resulting

from them, in the direction of the center points of the circles of curvature,

are:

=2σ ds

sin β =2σ ds

(6.54)

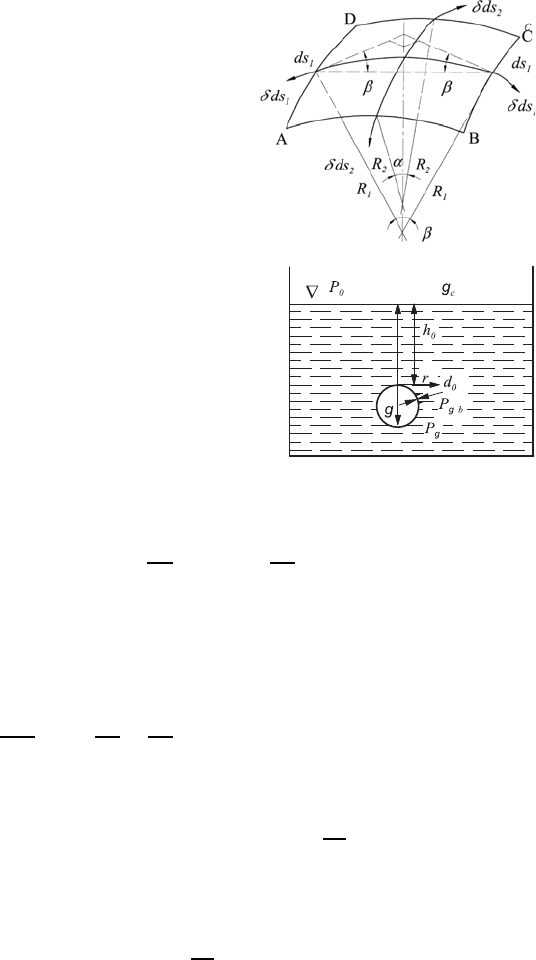
6.3 Free Fluid Surfaces 171

Fig. 6.15 Schematic representation of a

curved surface

Fig. 6.16 Diagram for the consideration of pres-

sure in bubbles

Similarly, the action of forces dK

is computed as

dO (6.55)

This shows that as a consequence of the surface tension, pressure eﬀects occur

that are directed towards the center points of the circles of curvature. This

pressure eﬀect is computed as force per unit area. As the considerations show,

a diﬀerential pressure results, which is caused by the surface tension:

∆p

= σ





, with dK =dK

+dK

(6.56)

If a spherical surface is considered, the following relationship holds:

= R

= R −→ ∆p

2σ

(6.57)

This relation means that the gas pressure in a spherical bubble is higher

than the liquid pressure imposed from outside:

2σ

= P

(6.58)

For very small bubbles this pressure diﬀerence can be very large.

When one considers the equilibrium state of a surface element of a bubble

(see Fig. 6.16), the following relationship can be written for the pressure in

the upper apex:

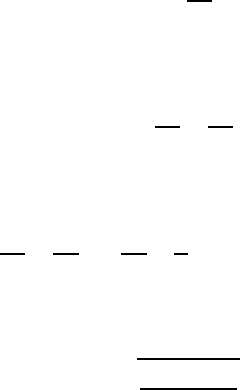
172 6 Hydrostatics and Aerostatics

+ ρ

+ σ





= p

g,0

(6.59)

For a surface element of any height, the following pressure equilibrium

holds:

+ ρ

g (h

+ y)+σ





= p

g,0

+ ρ

gy (6.60)

When one now forms the diﬀerence of these pressure relationships, one

obtains:





−

(ρ

− ρ

) gy = 0 (6.61)

Hence the characteristic quantity for the normalization of equation (6.61)

is to be introduced:

a =

2σ

g (ρ

− ρ

)

(6.62)

which is known as the Laplace constant or capillary constant. It has the

dimensions of length and indicates the order of magnitude when a perceptible

inﬂuence of the surface tension on the surface shape of a medium exists.

• When the Laplace constant of a free surface of a liquid is comparable to

the dimensions of the liquid body, an inﬂuence of the surface tension on

the free surface shape is to be expected.

• In the proximity of liquid rims (container walls), an inﬂuence of the surface

tension on the shape of the ﬂuid surface is to be expected with linear

dimensions that are of the order of magnitude of the Laplace constant.

6.3.2 Water Columns in Tubes and Between Plates

From the ﬁnal statements in Sect. 6.3.1, consequences result for considera-

tions of heights of liquid columns in pipes and channels of small dimensions.

Considerations, have been carried out already in Sect. 6.2, but inﬂuences of

the interactions between liquid, solid and gaseous phases remained uncon-

sidered there, i.e. the inﬂuence of the surface tension was not taken into

account. Considering the insights into the properties of connected containers

ﬁlled with liquid gained in Sect. 6.3.1, one sees that the considerations stated

for “communicating systems” in Sect. 6.2 hold only when the dimensions of

the systems are larger than the Laplace constant of the ﬂuid interface. More-

over, the considerations only hold far away from ﬂuid rims. In the immediate

proximity of the rim there exists an inﬂuence of the surface tension which

was neglected in the considerations in Sect. 6.2.

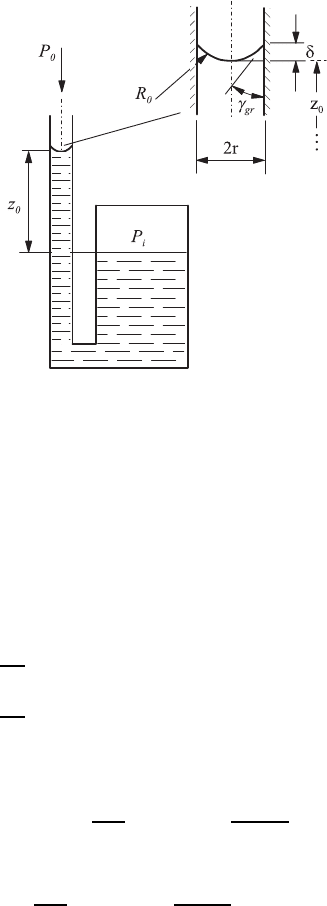
6.3 Free Fluid Surfaces 173

Fig. 6.17 Diagram for consideration of

heights of liquid column in tubes and

between plates

The processes taking place in ﬂuid containers of small dimensions can be

treated easily if one carries out a division of the properties of container walls

into “wetting” ones and “non-wetting” ones. When making the considerations

ﬁrst for wetting walls, experiments show that for such surfaces, in small tubes

and between plates with small distances forming small gaps, the ﬂuid in the

tube or between the plates assumes a height which is above the height of the

surface of a connected larger container, as indicated in Fig. 6.17.

From pressure equilibrium considerations it follows that

Pressure between plates: p

−

= p

− ρ

(6.63a)

Pressure in tubes: p

−

2σ

= p

− ρ

(6.63b)

or, written in another form,

Height of water surface between plates: z

− p

(6.64a)

Height of water surface in tubes: z

− p

2σ

(6.64b)

In these relationships, the radius of curvature R

has to be considered as

an unknown before its determination, for which two possibilities exist. To

simplify the corresponding derivations one can assume, with a precision that

is suﬃcient in practice, that the surface in the rising pipe adopts the form

of a partial sphere for the tube and that of a partial cylinder for the gap

174 6 Hydrostatics and Aerostatics

of the plate. The angle of contact between the ﬂuid surface and the tube

wall or plate wall has to be known from information regarding the wetting

properties of the wall materials. When one deﬁnes this angle as γ

,one

obtains the following relation:

r = R

cos γ

(6.65)

For the ﬁnal relationships of the height z

of the water surface for plates

and tubes, the following expressions hold:

Plates: z

− p

cos γ

(6.66a)

Tub e: z

− p

2σ

cos γ

(6.66b)

These ﬁnal relationships now show that even in the case of pressure equal-

ity, i.e. p

= p

, the height z

of the free liquid surface assumes ﬁnite values

if γ

exists. This has to be considered when employing communicating

systems for measurements of the height of the water level in containers and

when measuring pressures.

The second possibility for computing the pressure diﬀerence below and

above interphases is given by the fact that it is experimentally possible, al-

though with greater inaccuracy, to determine the quantity δ in Fig. 6.17 by

means of the following considerations:

+(R

− δ)

= R

+ δ

2δ

(6.67)

The height z

of the liquid surface is calculated from this as follows:

ρg

− p

4σδ

g (r

+ δ

)

(6.68)

This proves that for σ = 0 no height diﬀerence exists and a liquid level

increase due to surface eﬀects is not to be expected in tubes or between

plates. Under such conditions, for considerations of wetting of a surface, the

relations derived in Sect. 6.2 hold also for small tube diameters and small

gaps between plates.

In the case of non-wetting surfaces, it is observed that the ﬂuid in the

interior of a rising tube or the gap between plates does not reach the height

which the ﬂuid outside the tube (or the gap of the plate) assumes, as indicated

in Fig. 6.18. Analogous to the preceding considerations for wetting ﬂuids, it

can be stated that the following relationship holds:

= −

2σ

ρg

(6.69)

where R

can be introduced again as indicated above.

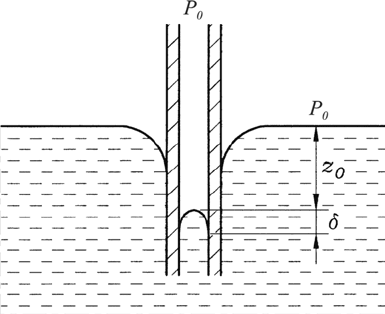
6.3 Free Fluid Surfaces 175

Fig. 6.18 Considerations of the height of the meniscus in tubes and between plates

for non-wetting surfaces

The equation thus obtained indicates that the ﬁnal relationships derived

for the wetting surfaces can often be applied also to non-wetting media,

if one considers the sign of γ

and δ,Thusδ needs, for example, to be

introduced positively in the above relationships for wetting ﬂuids, whereas

for non-wetting surfaces δ has to be inserted negatively.

6.3.3 Bubble Formation on Nozzles

The injection of gases into ﬂuids for chemical reactions or for an exchange of

gases represents a process which is employed in many ﬁelds of process engi-

neering. Thus bubble formation on nozzles as an introduction process for the

injected gases is of interest for these applications. Moreover, the simulation

of boiling processes, where steam bubbles are replaced by gas bubbles, repre-

sents another ﬁeld where precise knowledge of bubble formation is required.

When gas bubbles form on nozzles during the gassing of liquids, the pres-

sure in the interior of bubbles changes. For the theoretically conceivable static

bubble formation, this is attributed to diﬀerent curvatures of the bubble in-

terface during the formation of bubbles and thus to changes of the pressure

diﬀerence caused by capillary eﬀects . Superimposed upon these are changes

in pressure which have their origin in the upward movement of the bubble

vertex taking place during the formation. With the dynamic formation of

bubbles, additional changing pressure eﬀects are to be expected which are

essentially caused by accelerative and frictional forces.

By the term “static bubble formation”, one understands the formation

of bubbles under pressure conditions, which allow one to neglect the pres-

sure eﬀects on an element of the interface due to accelerative and frictional