Drake G.W.F. (editor) Handbook of Atomic, Molecular, and Optical Physics
Подождите немного. Документ загружается.

38 Part A Mathematical Methods
reducible tensor operator T
J
of rank J. An irreducible
tensor operator T
J
of rank J is a set of 2J +1 operators
T
J
=
(
T
J
M
&
&
M = J, J −1,... ,−J
)
with the following properties with respect to SU(2):
1. Commutation relations with respect to the angular
momentum J:
#
J
+
, T
J
M
$
=
[
(J −M)(J +M+1)
]
1
2
T
J
M+1
,
#
J
−
, T
J
M
$
=
[
(J +M)(J −M+1)
]
1
2
T
J
M−1
,
#
J
3
, T
J
M
$
= MT
J
M
,
i
#
J
i
,
#
J
i
, T
J
M
$$
= J( J +1)T
J
M
. (2.52)
2. Generation from highest “weight”:
T
J
M
=
(J +M)!
(2J)!(J − M)!
1
2
!
J
−
, T
J
J
"
(J−M)
,
where [A, B]
(k)
=[A, [A, B]
(k−1)
], k = 1, 2,... ,
with [A, B]
(0)
= B, denotes the k-fold commutator
of A with B.
3. Unitary transformation with respect to SU(2) rota-
tions:
e
−iψ
ˆ
n·J
T
J
M
e
iψ
ˆ
n·J
=
M
D
J
M
M
(U)T
J
M
, U =U(ψ,
ˆ
n). (2.53)
Angular momentum operators act in Hilbert spaces
by acting linearly on the vectors in such spaces. The con-
cept of a tensor operator generalizes this by replacing
the irreducible space H
J
by the irreducible tensor T
J
,
and angular momentum operator action on H
J
by com-
mutator action on T
J
, as symbolized, respectively, by
J :{states }→{states },
{ commutator action of J }:{tensor operators }
→{tensor operators } .
Just as exponentiation of the standard generator ac-
tion (2.13)and(2.14) gives relation (2.16), so does the
exponentiation of the commutator action (2.52)give
relation (2.53), when one uses the Baker–Campell–
Hausdorff identity:
e
tA
Be
−tA
=
k
t
k
k !
[A, B]
(k)
.
Thus, the linear vector space of states is replaced by
the linear vector space of operators. Abstractly, rela-
tions (2.13)and(2.52) are identical: only the rule of
action and the object of that action has changed.
An example of an irreducible tensor of rank 1 is
the angular momentum J itself, which has the special
property J :H
j
→H
j
. Thus, relations (2.52)and(2.53)
are realized as:
T
1
1
= J
+1
=−(J
1
+iJ
2
)/
√
2 ,
T
1
0
= J
0
= J
3
,
T
1
−1
= J
−1
= (J
1
−iJ
2
)/
√
2 ;
#
J
+
, T
1
µ
$
=
[
(1 −µ)(2 +µ)
]
1
2
T
1
µ+1
,
#
J
−
, T
1
µ
$
=
[
(1 +µ)(2 −µ)
]
1
2
T
1
µ−1
,
#
J
3
, T
1
µ
$
= µT
1
µ
,µ= 1, 0, −1 ;
e
−iψ
ˆ
n·J
Je
iψ
ˆ
n·J
= Jcos ψ +
ˆ
n(
ˆ
n· J)(1 −cos ψ)
−(
ˆ
n× J) sin ψ,
e
−iψ
ˆ
n·J
T
1
µ
e
iψ
ˆ
n·J
=
ν
D
1
νµ
(ψ,
ˆ
n)T
1
ν
.
2.8.2 Universal Enveloping Algebra of J
The universal enveloping algebra A( J) of J is the set of
all complex polynomial operators in the components J
i
of J, or equivalently in ( J
+
, J
3
, J
−
). The irreducible
tensor operators spanning this algebra are the analogues
of the solid harmonics Y
lm
(x) and are characterized by
the following properties: Basis set:
T
k
k
= a
k
J
k
+
, a
k
arbitrary constant ,
T
k
µ
= a
k
(k+µ)!
(2k)!(k−µ)!
#
J
−
, J
k
+
$
(k−µ)
,
µ = k, k−1,... ,−k ; k =0, 1, 2,... .
Standard action with respect to J:
#
J
±
, T
k
µ
$
=
[
(k∓µ)(k±µ +1)
]
1
2
T
k
µ±1
,
!
J
3
, T
k
µ
"
= µT
k
µ
,
3
i=1
J
i
,
#
J
i
, T
k
µ
$
= k(k+1)T
k
µ
.
Unitary transformation:
e
−iψ
ˆ
n·J
T
k
µ
e
iψ
ˆ
n·J
=
ν
D
k
νµ
(ψ,
ˆ
n)T
k
ν
.
Part A 2.8
Angular Momentum Theory 2.8 Tensor Operator Algebra 39
2.8.3 Algebra
of Irreducible Tensor Operators
Irreducible tensor operators possess, as linear operators
acting in the same space, properties 1., 2., and 3. below,
and an additional multiplication property 4., which con-
structs new irreducible tensor operators out of two given
ones and is called coupling of irreducible tensor opera-
tors. Property 4. extends also to tensor operators acting in
the tensor product space associated with kinematically
independent systems. It is important that associativity
extends to the product (2.54), as well as to the prod-
uct (2.55). Commutativity in these products is generally
invalid. The coupling properties given in 4. and 5. are
analogous to the coupling of basis state vectors. The op-
eration of Hermitian conjugation of operators, which is
the analogue of complex conjugation of states, is also
important, and has the properties presented under 5.
1. Multiplication of an irreducible tensor operator of
rank k by a complex number or an invariant with
respect to angular momentum J gives an irreducible
tensor operator of the same rank.
2. Addition of two irreducible tensor operator of the
same rank gives an irreducible tensor of that rank.
3. Ordinary multiplication (juxtaposition) of three ir-
reducible tensor operators is associative, but the
multiplication of two is noncommutative, in general.
4. Two irreducible tensor operators S
k
1
and T
k
2
of dif-
ferent or the same ranks acting in the same space
may be multiplied to obtain new irreducible tensor
operators of ranks given by the angular momentum
addition rule (Clebsch–Gordan series):
!
S
k
1
× T
k
2
"
k
µ
=
µ
1
,µ
2
C
k
1
k
2
k
µ
1
µ
2
µ
S
k
1
µ
1
T
k
2
µ
2
, (2.54)
µ = k, k−1,... ,−k ;
rank =
k ∈
{
k
1
+k
2
, k
1
+k
2
−1,... ,|k
1
−k
2
|
}
.
The following symbol denotes the irreducible tensor
operator with the µ-components (2.54):
!
S
k
1
× T
k
2
"
k
.
5. Two irreducible tensor operators S
k
1
and T
k
2
of dif-
ferent or the same ranks acting in different Hilbert
spaces, say H and K, may first be multiplied by the
tensor product rule so as to act in the tensor product
space H ⊗K,thatis,
S
k
1
µ
1
⊗T
k
2
µ
2
: H ⊗K → H ⊗K ,
and then coupled to obtain new irreducible tensor
operators, acting in the same tensor product space
H ⊗K:
#
S
k
1
⊗T
k
2
$
k
µ
=
µ,µ
2
C
k
1
k
2
k
µ
1
µ
2
µ
S
k
1
µ
1
⊗T
k
2
µ
2
,
µ = k, k−1,... ,−k .
(2.55)
The following symbol denotes the tensor operator
with the µ-components (2.55):
#
S
k
1
⊗T
k
2
$
k
,
k ∈
{
k
1
+k
2
, k
1
+k
2
−1,... ,|k
1
−k
2
|
}
.
6. The conjugate tensor operator to T
J
, denoted
by T
J†
, is the set of operators with components T
J†
M
defined by
4
j
m
&
&
T
J†
M
&
&
jm
-
=
4
jm
&
&
T
J
M
&
&
j
m
-
∗
.
These components satisfy the following relations:
#
J
±
, T
J†
M
$
=−[(J ±M)(J ∓M+1)]
1
2
T
J†
M∓1
#
J
3
, T
J†
M
$
=−MT
J†
M
,
i
#
J
i
,
#
J
i
, T
J†
M
$$
= J( J +1)T
J†
M
;
e
−iψ
ˆ
n·J
T
J†
M
e
iψ
ˆ
n·J
=
M
D
J∗
M
M
(ψ,
ˆ
n)T
J†
M
;
I
J
=
M
T
J
M
T
J†
M
=
invariant operator to
SU(2) rotations
,
e
−iψ
ˆ
n·J
I
J
e
iψ
ˆ
n·J
= I
J
.
An important invariant operator is
I
k
1
k
2
k
=
µ
1
µ
2
µ
C
k
1
k
2
k
µ
1
µ
2
µ
T
k
1
µ
1
T
k
2
µ
2
T
k†
µ
.
7. Other definitions of conjugation:
T
T
M
→(−1)
J−M
T
J
−M
, T
J
M
→(−1)
J+M
T
J
−M
.
2.8.4 Wigner–Eckart Theorem
The Wigner–Eckart theorem establishes the form of
the matrix elements of an arbitrary irreducible tensor
operator:
4
j
m
&
&
T
J
M
&
&
jm
-
=
4
j
5
5
T
J
5
5
j
-
C
jJ j
mMm
=
j
5
5
T
J
5
5
j
(−1)
j+J+m
jJ j
mM−m
.
Part A 2.8
40 Part A Mathematical Methods
Reduced matrix elements with respect to WCG-
coefficients:
4
j
5
5
T
J
5
5
j
-
=
µM
C
jJ j
µMµ
4
j
µ
&
&
T
J
M
&
&
jµ
-
,
each µ
= j
, j
−1,... ,−j
(the reduced matrix elem-
ent is independent of µ
).
Reduced matrix elements with respect to 3– j coeffi-
cients:
j
5
5
T
J
5
5
j
= (−1)
2J
3
2 j
+1
4
j
5
5
T
J
5
5
j
-
.
Examples of irreducible tensor operators include:
1. The solid harmonics with respect to the orbital an-
gular momentum L:
Y
k
(x) ={Y
kµ
(x) : µ = k,... ,−k} ,
Y
kµ
|lm=
l
4
l
5
5
Y
k
5
5
l
-
C
lkl
m,µ,m+µ
&
&
l
, m +µ
-
,
where
x |lm
= Y
lm
(x),
4
l
5
5
Y
k
5
5
l
-
=r
l+k−l
(2l+1)(2k+1)
4π(2l
+1)
1
2
C
lkl
000
,
Y
kµ
(x)Y
lm
(x)
=
l
4
l
5
5
Y
k
5
5
l
-
C
lkl
m,µ,m+µ
Y
l
,m+µ
(x),
!
Y
k
1
(x) ⊗Y
k
2
(x)
"
k
µ
=
µ
1
µ
2
C
k
1
k
2
k
µ
1
µ
2
µ
Y
k
1
µ
1
(x)Y
k
2
µ
2
(x),
!
Y
k
1
(x) ⊗Y
k
2
(x)
"
k
µ
=
4
k
5
5
Y
k
1
5
5
k
2
-
Y
kµ
(x).
2. The polynomial operator T
k
in the components of J
(Sect. 2.8.2):
4
j
m
&
&
T
k
µ
&
&
jm
-
= δ
j
j
4
j
5
5
T
k
5
5
j
-
C
jk j
mµm
,
4
j
5
5
T
k
5
5
j
-
= a
k
(−1)
k
(2 j +k+1)!k!k!
(2 j +1)(2 j −k)!(2k )!
1
2
.
3. Polynomials in the components of an arbitrary vector
operator V, which has the defining relations:
!
J
i
, V
j
"
= ie
ijk
V
k
,
!
J
±
, V
µ
"
=[(1 ∓µ)(2 ±µ)]
1
2
V
µ±1
,
!
J
3
, V
µ
"
= µV
µ
,
V
+1
=−(V
1
+iV
2
)/
√
2, V
0
= V
3
,
V
−1
= (V
1
−iV
2
)/
√
2 .
This construction parallels exactly that given
in Sect. 2.8.2 upon replacing J by V. The explicit form
of the resulting polynomials may be quite different since
no assumptions are made concerning commutation re-
lations between the components V
i
of V. The solid
harmonics in the gradient operator ∇ constitute an irre-
ducible tensor operator with respect to the orbital angular
momentum L.
2.8.5 Unit Tensor Operators
or Wigner Operators
A unit tensor operator is an irreducible tensor opera-
tor
ˆ
T
J,∆
, indexed not only by the angular momentum
quantum number J, but also by an additional label ∆,
which specifies that this irreducible tensor operator has
reduced matrix elements given by
4
j
5
5
ˆ
T
J,∆
5
5
j
-
= δ
j
, j+∆
.
This condition is to be true for all j = 0, 1/2, 1,....
There is a unit tensor operator defined for each
∆ = J, J −1,... ,−J .
The special symbol
*
J +∆
2J 0
•
+
denotes a unit tensor operator, replacing the boldface
symbol
ˆ
T
J,∆
, while the symbol
*
J +∆
2J 0
J +M
+
, M = J, J −1,... ,−J
denotes the components. In the same way that abstract
angular momentum J and state vectors {|jm} extract
the intrinsic structure of all realizations of angular mo-
mentum theory, as given in Sect. 2.2, so does the notion
of a unit tensor operator extract the intrinsic structure of
the concept of irreducible tensor operator by disregard-
ing the physical content of the theory, which is carried
in the structure of the reduced matrix elements. Physical
theory is regained from the fact that the unit tensor oper-
ators are the basis for arbitrary tensor operators, which
is the structural content of the Wigner–Eckart theorem.
The concept of a unit tensor operator was introduced by
Racah, but it was Biedenharn who recognized the full
significance of this concept not only for SU(2),butfor
all the unitary groups.
All of the content of physical tensor operator the-
ory can be regained from the properties of unit tensor
operators or Wigner operators as summarized below:
Part A 2.8

Angular Momentum Theory 2.8 Tensor Operator Algebra 41
Notation (double Gel’fand patterns):
*
J +∆
2J 0
J +M
+
,
M,∆= J, J −1,... ,−J
2J = 0, 1, 2,... .
Definition (shift action):
*
J +∆
2J 0
J +M
+
|jm=C
jJ j+∆
m,M,m+M
| j +∆, m +M
(2.56)
for all j = 0,
1
2
,... ; m = j, j −1,... ,−j.
Conjugation:
*
J +∆
2J 0
J +M
+
†
|jm
= C
j−∆ Jj
m−M,M,m
| j −∆, m −M .
(2.57)
Orthogonality:
M
*
J +∆
2J 0
J +M
+*
J +∆
2J 0
J +M
+
†
= δ
∆
∆
I
J
∆
,
(2.58)
∆
*
J +∆
2J 0
J +M
+
†
*
J +∆
2J 0
J +M
+
= δ
M
M
,
(2.59)
m
jm|
*
J
+∆
2J
0
J
+M
+*
J +∆
2J 0
J +M
+
†
|jm
=
2 j +1
2J +1
δ
J
J
δ
M
M
δ
∆
∆
. (2.60)
The invariant operator I
J
∆
is defined by its action on an
arbitrary vector ψ
j
∈ H
j
:
I
J
∆
ψ
j
=
j−∆, J, j
ψ
j
.
Tensor operator property:
e
−iψ
ˆ
n·J
*
J +∆
2J 0
J +M
+
e
iψ
ˆ
n·J
=
M
D
J
M
M
(ψ,
ˆ
n)
*
J +∆
2J 0
J +M
+
.
(2.61)
Basis property (Wigner–Eckart theorem):
T
J
M
| jm
=
∆
4
j +∆
5
5
T
J
5
5
j
-
*
J +∆
2J 0
J +M
+
| jm .
(2.62)
Characteristic null space:
The characteristic null space of the Wigner opera-
tor defined by (2.56) is the set of irreducible subspaces
H
j
⊂ H given be
{H
j
: 2 j = 0, 1,... ,J −∆ −1} .
Coupling law:
αβ
C
abc
αβγ
*
b+σ
2b 0
b+β
+*
a+ρ
2a 0
a+α
+
= W
abc
ρ,σ,ρ+σ
*
c+ρ +σ
2c 0
c+γ
+
,
(2.63)
where W
abc
ρστ
is an invariant operator (commutes with J)
and is called a Racah invariant. Its relationship to Racah
coefficients and 6– j coefficients is given in Sect. 2.9.
Product law:
*
b+σ
2b 0
b+β
+*
a+ρ
2a 0
a+α
+
=
c
W
abc
ρ,σ,ρ+σ
C
abc
α,β,α+β
*
c+ρ +σ
2c 0
c+α +β
+
.
(2.64)
Racah invariant:
W
abc
ρστ
=
αβγ
C
abc
αβγ
×
*
b+σ
2b 0
b+β
+*
a+ρ
2a 0
a+α
+*
c+τ
2c 0
c+γ
+
†
.
(2.65)
The notation W
abc
ρστ
for a Racah invariant is designed
to “match” that of the WCG-coefficient on the left, the
latter being associated with the lower group theoretical
labels, for example,
2a 0
a+α
→| aα ,
Part A 2.8

42 Part A Mathematical Methods
the state vector having a group transformation law under
the action of SU(2), and the former with the shift labels
of a unit tensor operator,
α +ρ
2α 0
,
and having no associated group transformation law. The
invariant operator defined by (2.65) has real eigenvalues,
hence, is a Hermitian operator,
W
abc†
ρστ
= W
abc
ρστ
, (2.66)
which is diagonal on an arbitrary state vector in H
j
(Sect. 2.9).
The Racah invariant operator does not commute with
a unit tensor operator, and it makes a difference whether
it is written to the left or to the right of such a unit
tensor operator. The convention here writes it to the
left.
Relation (2.65) is taken as the definition of W
abc
ρστ
and
the following properties all follow from this expression:
Domain of definition:
W
abc
ρστ
: a, b, c ∈{0, 1/2, 1, 3/2,...};
ρ =a, a−1,... ,−a
σ =b, b−1,... ,−b
τ =c, c−1,... ,−c ;
W
abc
ρστ
= 0, if ρ +σ = τ; if
abc
= 0 .
Orthogonality relations:
ρσ
W
abc
ρστ
W
abd
ρστ
= δ
cd
δ
ττ
abc
I
c
τ
, (2.67)
cτ
W
abc
ρστ
W
abc
ρ
σ
τ
= δ
ρρ
δ
σσ
I
ab
ρσ
, (2.68)
where the I invariant operators in these expressions
have the following eigenvalues on an arbitrary vector
ψ
j
∈ H
j
:
I
c
τ
ψ
j
=
j−τ,c, j
ψ
j
,
I
ab
ρσ
ψ
j
=
j−σ −ρ,a, j−σ
j−σ,b, j
ψ
j
.
The orthogonality relations for Racah invariants parallel
exactly those of WCG-coefficients.
Using the orthogonality relations (2.67) for Racah
invariants, the following two relations now follow
from (2.63)and(2.64), respectively:
WCG and Racah operator coupling:
ρσ
αβ
W
abd
ρ,σ,ρ+σ
C
abc
α,β,α+β
×
*
b+σ
2b 0
b+β
+*
a+ρ
2a 0
a+α
+
= δ
cd
abc
I
d
τ
*
c+τ
2c 0
c+γ
+
.
(2.69)
Racah operator coupling of shift patterns:
ρσ
W
abc
ρστ
*
b+σ
2b 0
b+β
+*
a+ρ
2a 0
a+α
+
= C
abc
α,β,α+β
*
c+τ
2c 0
c+α +β
+
.
(2.70)
Relations (2.56–2.70) capture the full content of
irreducible tensor operator algebra through the con-
cept of unit tensor operators that have only 0 or 1
for their reduced matrix elements. Using the Wigner–
Eckart theorem (2.62), the relations between general
tensor operators can be reconstructed. Unit tensor op-
erators were invented to exhibit in the most elementary
way possible the abstract and intrinsic structure of the
irreducible tensor operator algebra, stripping away the
details of particular physical applications, thus giving the
theory universal application. It accomplishes the same
goal for tensor operator theory that the abstract multi-
plet theory in Sect. 2.2 accomplishes for representation
theory.
Physical theory is regained through the concept of re-
duced matrix element. The coupling rule (2.54)isnow
transformedtoaruleemptyofWCG-coefficient con-
tent and becomes a rule for coupling of reduced matrix
elements using the invariant Racah operators:
4
α
j
5
5
!
S
k
1
× T
k
2
"
k
5
5
(α) j
-
= (−1)
k
1
+k
2
−k
(α
) j
W
k
2
k
1
k
j
−j, j
−j
, j
−j
( j
)
×
4
α
j
5
5
S
k
1
5
5
(a
) j
-4
α
j
5
5
T
k
2
5
5
(α) j
-
.
(2.71)
This coupling rule is invariant to all SU(2) rota-
tions, and reveals the true role of the Racah coefficients
and reduced matrix elements in physical theory as in-
variant objects under SU(2) rotations. It now becomes
imperative to understand Racah coefficients as objects
free of their original definition in terms of WCG-
coefficients.
Part A 2.8
Angular Momentum Theory 2.9 Racah Coefficients 43
2.9 Racah Coefficients
Relation (2.65) is taken, initially, as the definition of the
Racah coefficient with appropriate adjustments of nota-
tions to conform to Racah’s W-notation and to Wigner’s
6– j notation. Corresponding to each of (2.63–2.65),
(2.69, 2.70), there is a corresponding numerical relation-
ship between WCG-coefficients and Racah coefficients.
Despite the present day popularity of expressing all such
relations in terms of the 3– j and 6– j notation, this temp-
tation is resisted here for this particular set of relations
because of their fundamental origins. The relation be-
tween the Racah invariant notation and Racah’s original
W-notation is
W
abc
ρστ
| jm=W
abc
ρστ
( j) | jm ,
W
abc
ρστ
( j) = 0ifτ = ρ +σ, or
abc
= 0 ,
W
abc
ρστ
( j) =[(2c+1)(2 j −2σ +1)]
1/2
× W( j −τ, a, j, b; j −σ, c),
[(2e+1)(2 f +1)]
1/2
W(abcd;ef)
= W
bdf
e−a,c−e,c−a
(c),
W(abcd;ef) = 0 unless the triples of nonnegative inte-
gers and half-integers (abe), (cde), (acf ), (bdf ) satisfy
the triangle conditions.
2.9.1 Basic Relations Between WCG
and Racah Coefficients
βδ
C
bdf
βδγ
C
edc
α+β,δ,α+γ
C
abe
α,β,α+β
=[(2e+1)(2 f +1)]
1/2
W(abcd;ef)C
afc
α,γ,α+γ
,
f
[(2e+1)(2 f +1)]
1/2
W(abcd;ef)
× C
bdf
β,δ,β+δ
C
afc
α,β+δ,α+β+δ
= C
edc
α+β,δ,α+β+δ
C
abe
α,β,α+β
,
δ
cc
[(2e+1)(2 f +1)]
1/2
W(abcd;ef)
=
βδ
C
bdf
β,δ,β+δ
C
edc
γ −δ,δ,γ
C
abe
γ −β−δ,β,γ −δ
× C
abc
γ −β−δ,β+δ,γ
,
βδe
[(2e+1)(2 f +1)]
1/2
W(abcd;ef)
× C
bdf
βδγ
C
edc
α+β,δ,α+γ
C
abe
α,β,α+β
= δ
ff
C
afc
α,γ,α+γ
,
e
[(2e+1)(2 f +1)]
1/2
W(abcd;ef)
× C
edc
α+β,δ,α+β+δ
C
abe
α,β,α+β
= C
bdf
β,δ,β+δ
C
afc
α,β+δ,α+β+δ
.
2.9.2 Orthogonality and Explicit Form
Orthogonality relations for Racah coefficients:
e
(2e+1)(2 f +1)W(abcd;ef )W(abcd;ef
)
= δ
ff
ac f
bdf
, (2.72)
f
(2e+1)(2 f +1)W(abcd;ef )W(abcd;e
f )
= δ
ee
abe
cde
. (2.73)
Definition of 6– j coefficients:
abe
dcf
= (−1)
a+b+c+d
W(abcd;ef). (2.74)
Orthogonality of 6– j coefficients:
e
(2e+1)(2 f +1)
abe
dcf
ab e
dcf
= δ
ff
acf
bdf
, (2.75)
f
[(2e+1)(2 f +1)]
abe
dcf
abe
dc f
= δ
ee
abe
cde
. (2.76)
Explicit form of Racah coefficients:
W(abcd;ef) = ∆(abe)∆(cde)∆(acf )∆(bdf )
×
k
(−1)
a+b+c+d+k
(k+1)!
(k−a−b−e)!(k−c−d−e)!
×
1
(k−a−c− f )!(k−b−d − f )!
×
1
(a+b+c+d −k)!
×
1
(a+d+e+ f −k)!(b+c+e+ f −k)!
,
(2.77)
where ∆(abc) denotes the triangle coefficient, defined
for every triple a, b, c of integers and half-odd integers
satisfying the triangle conditions by:
∆(abc)
=
(a+b−c)!(a−b+c)!(−a+b+c)!
(a+b+c+1)!
1
2
.
(2.78)
Part A 2.9

44 Part A Mathematical Methods
2.9.3 The Fundamental Identities
Between Racah Coefficients
Each of the three relations given in this section
is between Racah coefficients alone. Each expresses
a fundamental mathematical property. The Biedenharn–
Elliott identity is a consequence of the associativity rule
for the open product of three irreducible tensor op-
erators; the Racah sum rule is a consequence of the
commutativity of a mapping diagram associated with
the coupling of three angular momenta; and the triangle
coupling rule is a consequence of the associativity of the
open product of three symplection polynomials [2.1]. As
such, these three relations between Racah coefficients,
together with the orthogonality relations, are the build-
ing blocks on which is constructed a theory of these
coefficients that stands on its own, independent of the
WCG-coefficient origins. Indeed, the latter is recovered
through the limit relation (2.50).
Biedenharn–Elliott identity:
W(a
ab
b;c
e)W(a
ed
d;b
c)
=
f
(2 f +1)W(abcd;ef)W(c
bd
d;b
f )
× W(a
ad
f;c
c), (2.79a)
a
ac
bb
e
a
eb
dd
c
=
f
(−1)
φ
(2 f +1)
abe
dc f
c
bb
dd
f
×
a
ac
fd
c
,
φ = f −e+a
+a+b
+b+c
−c+d
−d .
(2.79b)
Racah sum rule:
f
(−1)
b+d− f
(2 f +1)W(abcd;ef )W(adcb;gf)
= (−1)
e+g−a−c
W(bacd;eg), (2.80a)
f
(−1)
e+g+ f
(2 f +1)
abe
dcf
adg
bc f
=
bae
dcg
.
(2.80b)
Triangle sum rule:
[∆(acf )∆(bdf )]
−1
= (2 f +1)
e
[∆(abe)∆(cde)]
−1
W(abcd;ef),
(2.81a)
(−1)
a+b+c+d
[∆(acf )∆(bdf )]
−1
= (2 f +1)
e
[∆(abe)∆(cde)]
−1
abe
dcf
.
(2.81b)
2.9.4 Schwinger–Bargmann Generating
Function and its Combinatorics
Triangles associated with the 6– j symbol
j
1
j
2
j
3
j
4
j
5
j
6
:
( j
1
j
2
j
3
), (j
3
j
4
j
5
), (j
1
j
5
j
6
), (j
2
j
4
j
6
).
Points in R
3
associated with the triangles:
( j
1
j
2
j
3
) → (x
1
, x
2
, x
3
), (j
3
j
4
j
5
) → (y
3
, x
4
, x
5
),
( j
1
j
5
j
6
) → (y
1
, y
5
, x
6
), (j
2
j
4
j
6
) → (y
2
, y
4
, y
6
).
Tetrahedron associated with the points:
The points define the vertices of a general tetrahe-
dron with lines joining each pair of points that share
a common subscript, and the lines are labeled by the
product of the common coordinates (Fig. 2.2).
Monomial term:
Define the triangle monomial associated with a tri-
angle ( j
a
j
b
j
c
) and its associated point (z
a
, z
b
, z
c
) in R
3
by
(z
a
, z
b
, z
c
)
( j
a
j
b
j
c
)
= z
j
b
+j
c
−j
a
a
z
j
c
+j
a
−j
b
b
z
j
a
+j
b
−j
c
c
.
(2.82)
Cubic graph (tetrahedral T
4
) functions:
Interchange the symbols x and yin the coordinates of
the vertices of the tetrahedron and define the following
polynomials on the vertices and edges of the tetrahedron
with this modified labeling.
Vertex function: multiply together the coordinates of
each vertex and sum over all such vertices to obtain
V
3
= y
1
y
2
y
3
+x
3
y
4
y
5
+x
1
x
5
y
6
+x
2
x
4
x
6
;
Edge function: multiply together the coordinates of
a given edge and the opposite edge and sum over all
such pairs to obtain
E
4
= x
1
y
1
x
4
y
4
+x
2
y
2
x
5
y
5
+x
3
y
3
x
6
y
6
.
Part A 2.9
Angular Momentum Theory 2.9 Racah Coefficients 45
(y
1
y
5
x
6
)
x
1
y
1
x
5
y
5
x
3
y
3
(x
1
x
2
x
3
)
(y
3
x
4
x
5
)
x
6
y
6
(y
2
y
4
y
6
)
x
2
y
2
x
4
y
4
Fig. 2.2 Labeled cubic graph (tetrahedron) associated with
6– j coefficients
Generating function:
(1 +V
3
+E
4
)
−2
=
∆
T(∆)Z
∆
, (2.83a)
Z
∆
= (x
1
, x
2
, x
3
)
( j
1
j
2
j
3
)
(y
3
, x
4
, x
5
)
( j
3
j
4
j
5
)
× (y
1
, y
5
, x
6
)
( j
1
j
5
j
6
)
(y
2
, y
4
, y
6
)
( j
2
j
4
j
6
)
, (2.83b)
∆ =
( j
1
j
2
j
3
)
( j
3
j
4
j
5
)
( j
1
j
5
j
6
)
( j
2
j
4
j
6
)
;
T(∆) =
k
(−1)
k
(k+1)
×
k
k
1
, k
2
, k
3
, k
4
, k
5
, k
6
, k
7
,
(2.83c)
k
i
= k−t
i
, i = 1, 2, 3, 4 ,
k
j
= e
j−4
−k , j = 5, 6, 7 ;
t
i
= triangle sum = vertex sum,
e
j
= opposite edge sum, in pairs,
t
1
= j
1
+ j
2
+ j
3
, t
2
= j
3
+ j
4
+ j
5
,
t
3
= j
1
+ j
5
+ j
6
, t
4
= j
2
+ j
4
+ j
6
,
e
1
= ( j
2
+ j
5
) +( j
3
+ j
6
),
e
2
= ( j
1
+ j
4
) +( j
3
+ j
6
),
e
3
= ( j
1
+ j
4
) +( j
2
+ j
5
).
Thesummationin(2.83b) is over the infinite set of all
tetrahedra; that is, over the infinite set of arrays ∆ having
nonnegative integral entries. The 6– j coefficients is then
given by
j
1
j
2
j
3
j
4
j
5
j
6
=
T(∆)
∆( j
1
j
2
j
3
)∆( j
1
j
5
j
6
)∆( j
2
j
4
j
6
)∆( j
3
j
4
j
5
)
.
Since the factor T(∆) is an integer in the expansion
(2.83a), this result shows that the 6– j coefficient is
an integer, up to the multiplicative triangle coefficient
factors.
2.9.5 Symmetries of 6– j Coefficients
There are 144 symmetry relations among the Racah
6– j coefficients. The 24 classical ones, given already
by Racah, and corresponding to the tetrahedral point
group T
d
of rotations-inversions (isomorphic to the sym-
metric group S
4
) mapping the regular tetrahedron onto
itself, are realized in the 6– j symbol
abe
dcf
as permutations of its columns and the exchange of any
pair of letters in the top row with the corresponding pair
in the bottom row. Regge discovered the 6-fold increase
in symmetry by noting that each term in the summation
in (2.77) is invariant not only to the classical 24 sym-
metries, but also under certain linear transformations of
the quantum labels. These symmetries are also implicit
in Schwinger’s generating function.
The full set, including the original 24 substitutions,
of linear transformations of the letters a, b, c, d, e, f
thus yields a group of linear transformation isomorphic
to S
4
× S
3
. The column permutations and row-pair in-
terchanges described above applied to each of the six
symbols in the equalities below yield the set of 144
relationships:
abe
dcf
=
a
b+c+e− f
2
b+e+ f −c
2
d
b+c+ f −e
2
c+e+ f −b
2
=
a+d +e− f
2
b
a+e+ f −d
2
a+d + f−e
2
c
d +e+ f −a
2
Part A 2.9
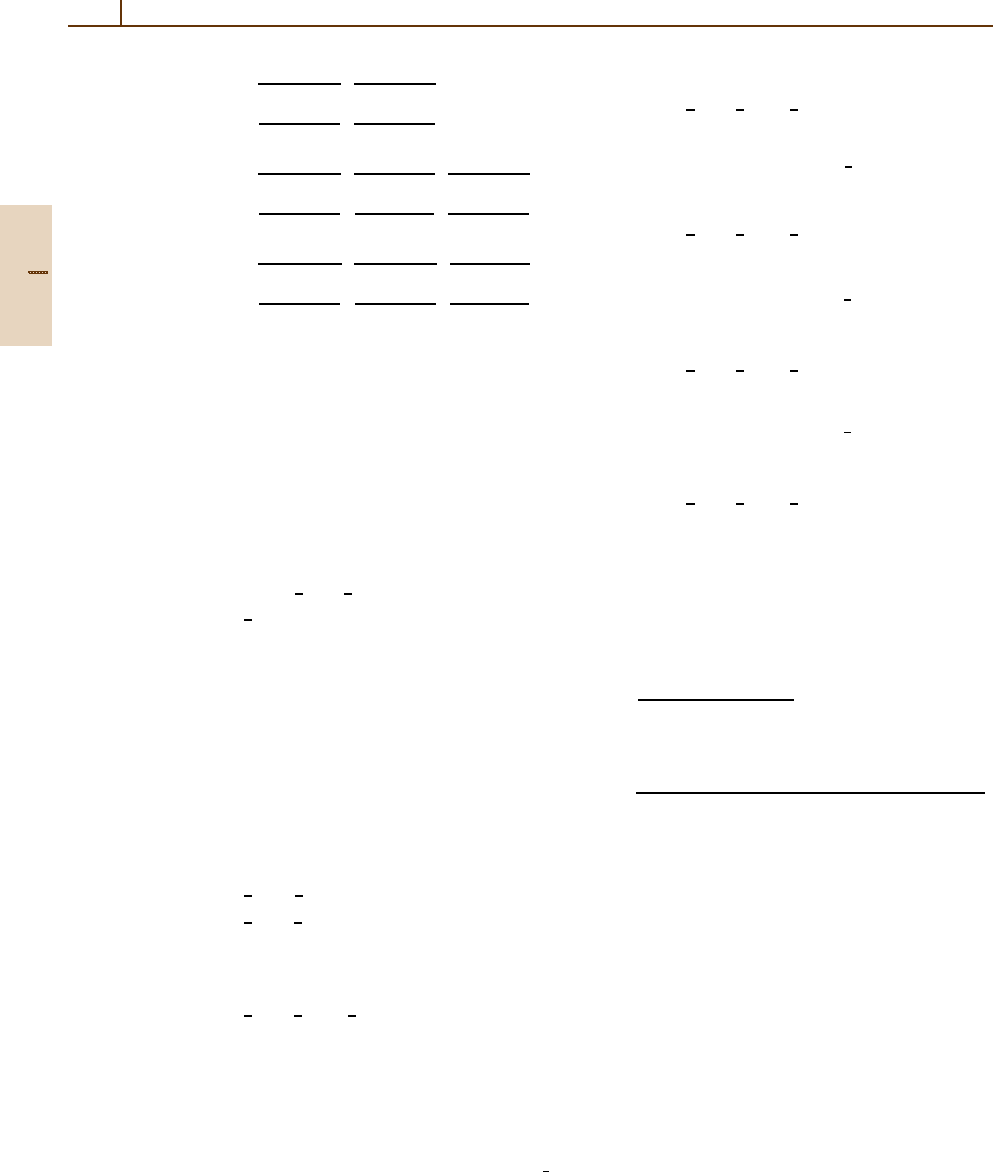
46 Part A Mathematical Methods
=
a+b+d−c
2
a+b+c−d
2
e
a+c+d−b
2
b+c+d−a
2
f
=
a+b+d−c
2
b+c+e− f
2
a+e+ f −d
2
a+c+d−b
2
b+c+ f −e
2
d +e+ f −a
2
=
a+d +e− f
2
a+b+c−d
2
b+e+ f −c
2
a+d + f−e
2
b+c+d−a
2
c+e+ f −b
2
.
2.9.6 Further Properties
Recurrence relations:
Three-term:
[(a+b+e+1)(b+e−a)
× (c+d +e+1)(d+e−c)]
1/2
abe
dcf
=−2e[(b+d + f +1)(b+d− f )]
1/2
×
ab−
1
2
e−
1
2
d −
1
2
cf
+[(a+b−e+1)(a+e−b)(c+d−e+1)
× (c+e−d)]
1/2
abe−1
dc f
,
(2.84a)
[(a+c+ f +1)(c+e−d)
× (d+e−c+1)(b+d − f +1)]
1/2
abe
dcf
=[(a+c− f )(a+e−b)
× (b+ f +d+2)(b+e−a+1)]
1/2
×
a+
1
2
b+
1
2
e
d +
1
2
c−
1
2
f
.
+[(c+ f −a)(c+e−d)(b−a−c+d+1)]
1/2
×
abe
d +
1
2
c−
1
2
f −
1
2
.
(2.84b)
Five-term:
(2c+1)(2d)(2 f +1)
abe
dcf
=[(b+d − f )(b+ f −d +1)(d +e−c)
× (c+e−d +1)(c+ f −a+1)(a+c+ f +2)]
1
2
×
abe
d −
1
2
c+
1
2
f +
1
2
+[(b+d− f )
× (b+ f −d+1)(c+d −e)(c+d +e+1)
× (a+c− f )(a+ f −c+1)]
1
2
×
abe
d −
1
2
c−
1
2
f +
1
2
−[(d + f −b)
× (b+d + f +1)(c+d−e)(c+d +e+1)
× (c+ f −a)(a+c+ f +1)]
1
2
×
abe
d −
1
2
c−
1
2
f −
1
2
+[(d + f −b)
× (b+d + f +1)(d +e−c)(c+e−d+1)
× (a+ f −c)(a+c− f +1)]
1
2
×
abe
d −
1
2
c+
1
2
f −
1
2
.
(2.84c)
Relation to hypergeometric series:
abe
dcf
= (−1)
a+b+c+d
W(abcd;ef)
= ∆(abe)∆(cde)∆(acf )∆(bdf )
×
(−1)
β
1
(β
1
+1)!
(β
2
−β
1
)!(β
3
−β
1
)!
×
4
F
3
α
1
−β
1
,α
2
−β
1
,α
3
−β
1
,α
4
−β
1
−β
1
−1,β
2
−β
1
+1,β
3
−β
1
+1,
;1
(β
1
−α
1
)!(β
1
−α
2
)!(β
1
−α
3
)!(β
1
−α
4
)!
,
β
1
= min(a+b+c+d, a+d+e+ f, b+c+e+ f ),
The parameters β
2
and β
3
are identified in either way
with the pair remaining in the 3-tuple
(a+b+c+d, a+d+e+ f, b+c+e+ f )
after deleting β
1
.The(α
1
,α
2
,α
3
,α
4
) may be identified
with any permutation of the 4-tuple
(a+b+e, c+d+e, a+c+ f, b+d+ f ).
The
4
F
3
series is Saalschützian:
1 +
(numerator parameters)
=
(denominator parameters) .
Part A 2.9

Angular Momentum Theory 2.10 The 9–j Coefficients 47
2.10 The 9–j Coefficients
2.10.1 Hilbert Space
and Tensor Operator Actions
Let T
a
(1) and T
b
(2) denote irreducible tensor operators
of ranks a and b with respect to kinematically indepen-
dent angular momentum operators J(1) and J(2) that
act, respectively, in separable Hilbert spaces H(1) and
H(2).LetH(1) and H (2) be reduced, respectively, into
a direct sum of spaces H
j
1
(1) and H
j
2
(2). The angular
momentum J(1) has the standard action on the or-
thonormal basis
(
| j
1
m
1
&
&
m
1
= j
1
, j
1
−1,... ,−j
1
)
of H
j
1
(1),andJ(2) has the standard action on the or-
thonormal basis
(
| j
2
m
2
&
&
m
2
= j
2
, j
2
−1,... ,−j
2
)
of H
j
2
(2). The irreducible tensor operators T
a
(1) and
T
b
(2) also have the standard actions in their respective
Hilbert spaces H (1) and H (2), as given by the Wigner–
Eckart theorem. The total angular momentum J has the
standard action on the coupled orthonormal basis of the
tensor product space H
j
1
⊗H
j
2
:
|( j
1
j
2
) jm=
m
1
m
2
C
j
1
j
2
j
m
1
m
2
m
|j
1
m
1
⊗|j
2
m
2
.
(2.85)
The tensor product operator T
a
(1) ⊗T
b
(2) acts in the
tensor product space H(1) ⊗H (2) according to the rule:
T
a
(1) ⊗T
b
(2)
(
|j
1
m
1
⊗|j
2
m
2
)
= T
a
(1)|j
1
m
1
⊗T
b
(2)|j
2
m
2
,
so that
T
a
(1) ⊗T
b
(2)
|
(
j
1
j
2
)
jm
=
m
1
m
2
C
j
1
j
2
j
m
1
m
2
m
T
a
(1)|j
1
m
1
⊗T
b
(2)|j
2
m
2
.
(2.86a)
The angular momentum quantities called 9– j coef-
ficients arise when the coupled tensor operators T
(ab)c
with components γ defined by
T
(ab)c
γ
=
αβ
C
abc
αβγ
T
a
α
(1) ⊗T
b
β
(2),
γ = c, c−1,... ,−c ,
(2.86b)
are considered. The quantity T
(ab)c
is an irreducible ten-
sor operator of rank c with respect to the total angular
momentum J for all a, b that yield c under the rule of
addition of angular momentum.
2.10.2 9– j Invariant Operators
The entire angular momentum content of rela-
tion (2.86b) is captured by taking the irreducible
tensor operators T
a
(1) and T
b
(2) to be unit ten-
sor operators acting in the respective spaces H(1)
and H(2):
T
(ab)c
(ρσ)γ
=
αβ
C
abc
αβγ
*
α +ρ
2a 0
a+α
+
1
⊗
*
b+σ
2b 0
b+β
+
2
.
(2.87)
The placement of the unit tensor operators shows in
which space they act, so that the additional iden-
tification by indices 1 and 2 could be eliminated.
For each given c ∈{0, 1/2, 1, 3/2, 2,...} and all a, b
such that the triangle relation (abc) is satisfied, and,
for each such pair a, b, all ρ, σ with ρ ∈{a, a−
1,... ,−a},σ ∈{b, b−1,... ,−b}, an irreducible ten-
sor operator of rank c with respect to the total angular
momentum J with components γ is defined by (2.87).
By the Wigner–Eckart theorem, it must be possible to
write
αβ
C
abc
αβγ
*
α +ρ
2a 0
a+α
+
1
⊗
*
b+σ
2b 0
b+β
+
2
=
τ
abc
ρστ
*
c+τ
2c 0
c+γ
+
.
(2.88)
where: (i) The unit tensor operator on the right-hand
side is a irreducible tensor operator with respect to J;
that is, has the action on the coupled states given
by
*
c+τ
2c 0
c+γ
+
|( j
1
j
2
) jm
= C
jc j+τ
m,γ,m+γ
|( j
1
j
2
) j +τ, m +γ ; (2.89)
and (ii) the symbol
#
abc
ρστ
$
denotes an invariant
operator with respect to the total angular mo-
mentum J. Using the orthogonality of unit tensor
operators, we can also write relation (2.88)inthe
Part A 2.10
