Czichos H., Saito T., Smith L.E. (Eds.) Handbook of Metrology and Testing
Подождите немного. Документ загружается.

228 Part B Chemical and Microstructural Analysis
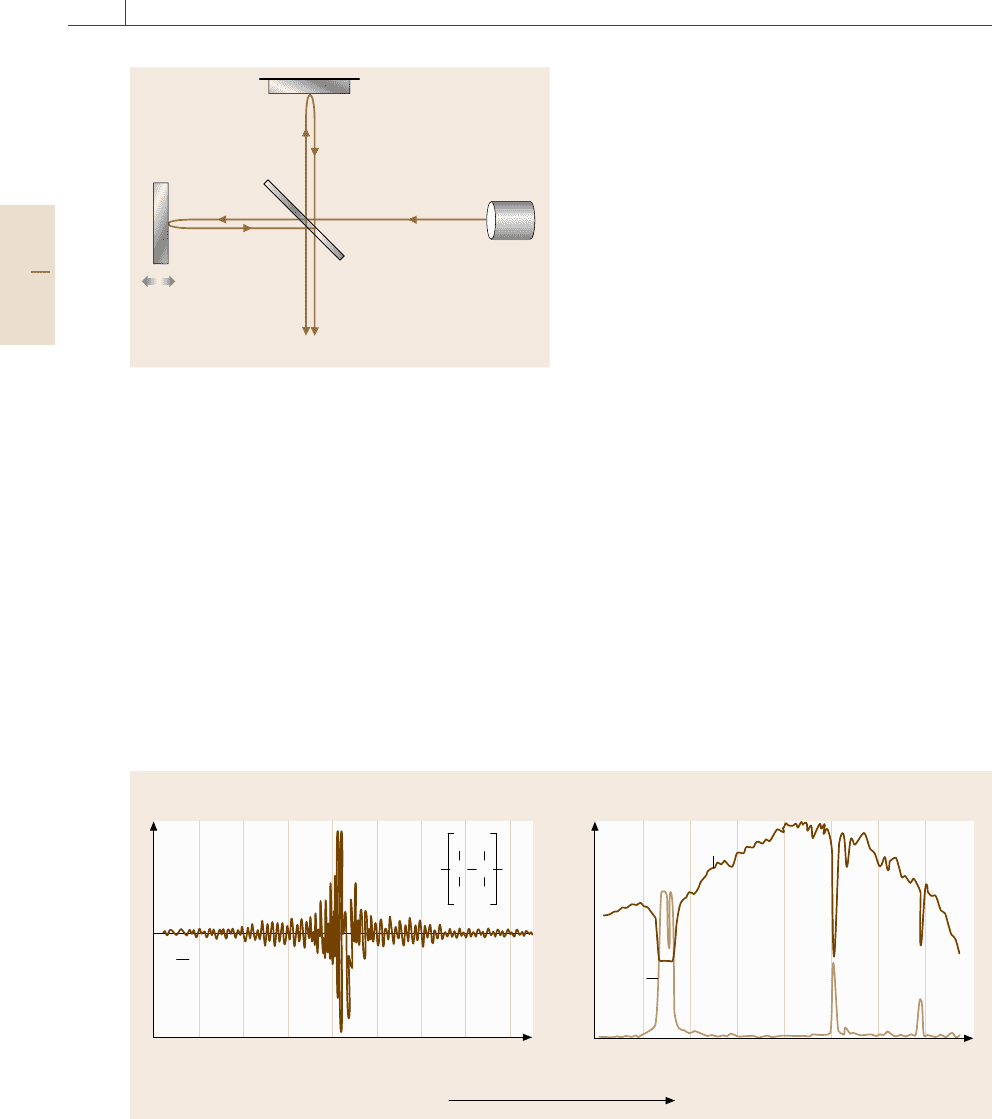
Fixed mirror
Light source
Movable
mirror
Half mirror
(beam splitter)
Δx
I
λ
(Δx)
Fig. 5.32 Michelson-type interferometer used in Fourier
transform infrared (FT-IR) spectroscopy
the wavelength λ. In other words, when the light in-
put to the interferometer contains different spectral
components, the interference fringes or interferogram
(Fig. 5.33a), the temporal superposition of the mod-
ulated signals, constitutes the Fourier transform of
the whole spectrum. Conversely, the inverse Fourier
transform of the experimentally measured interfero-
gram provides the spectrum (Fig. 5.33b) without any
spectral dispersive apparatus. The FT spectroscopy is
advantageous in terms of the signal-to-noise ratio when
the detector noise is large and we need high spectral
resolution. The infrared (IR) spectroscopy is a typi-
cal case to which the FT scheme is most efficiently
applied.
Intensity
100 900
Time (rel. units) Wave number (cm
–1
)
Interferogram FT-IR spectrum
Fourier transform
800 3500 300110019002700700600500400300200
Polyethylene
Absorbance, transmission (arb. units)
(abs)
(sb)
a) b)
I
0
2
H
C
H
H
C
H n
Fig. 5.33a,b An example of FT-IR absorption spectroscopy. (a) An interferogram of transmission through a polyethylene
sample, (b) (sb) Fourier transform of the interferogram and (abs) the absorbance spectrum corrected for the instrumental
background (after [5.27])
Raman Scattering
Raman scattering is a generic term for inelastic scat-
tering of light but in most cases it refers to inelastic
scattering brought about by optical phonons (quan-
tized vibrations) in molecules and solids. While infrared
absorption originates from the polarization of the sub-
stance, Raman scattering originates from a modulation
of optical polarizability of the substance by phonons.
Therefore, the selection rule is different for the two phe-
nomena. In molecules, infrared absorption occurs when
the molecules have an electric dipole transition moment
in the polarization direction of the incident light, as in
the cases shown in Fig. 5.34. Raman scattering occurs
when a molecular vibration in a certain mode alters
the electronic distribution of the molecule (Fig. 5.34)
and consequently the optical polarizability for the in-
cident light. In solids, although the selection rules are
not as intuitive as for molecules, the principles are the
same. Due to this difference in the selection rules, in-
frared spectroscopy and Raman scattering spectroscopy
are complementary in studies of atomic vibrations in
matter.
In the energy diagram, the sequential two-photon
process in Raman scattering is depicted in Fig. 5.35,
which shows only the case of the Stokes shift, in which
a phonon mode of energy hΩ is excited and as a re-
sult the photon energy is decreased on scattering by
this amount. The broken line in Fig. 5.35a indicates
an intermediate virtual electronic state via which the
system evolves in the sequence of photon absorption
and emission. In contrast to infrared absorption, Raman
Part B 5.1
Nanoscopic Architecture and Microstructure 5.1 Fundamentals 229
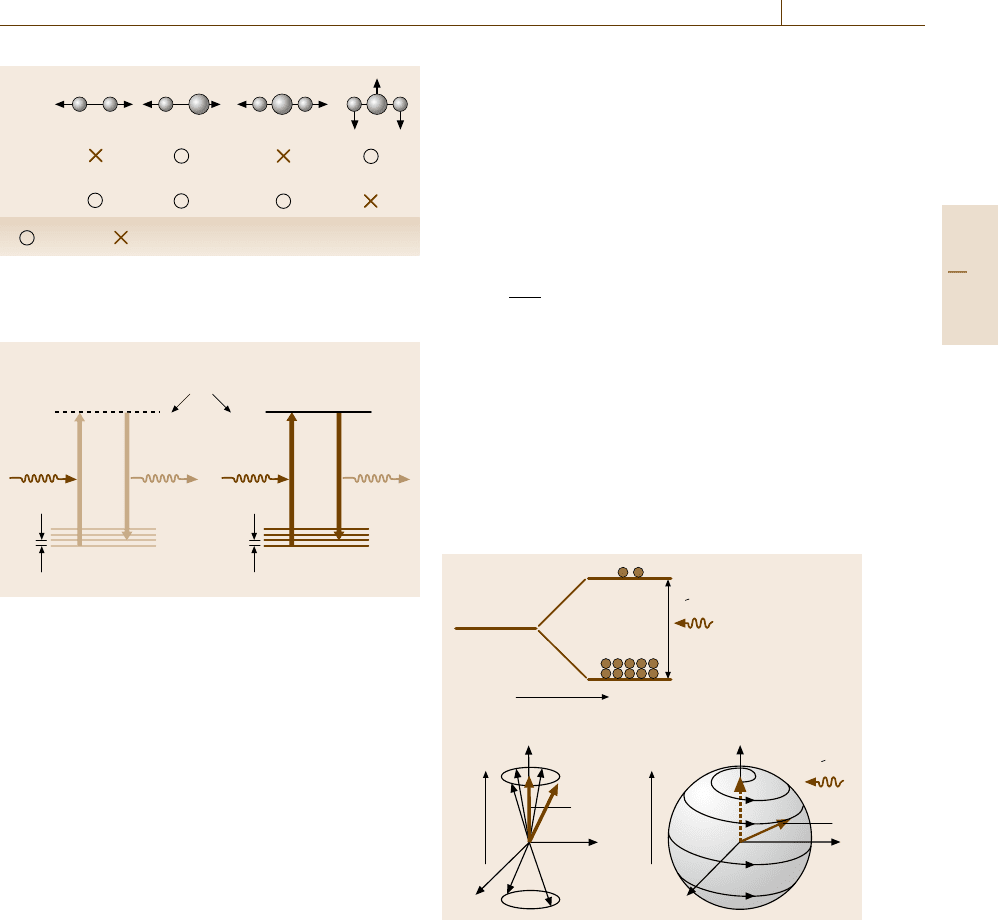
+–+
IR
Raman
: allowed : forbidden
Fig. 5.34 Selection rules of infrared absorption and Ra-
man scattering illustrated for molecules in various vibra-
tion modes
a) b)
Intermediate state
Vibronic ground state
hv–hΩ
hΩ
hv
hv–hΩ
hΩ
hv
Virtual Real
Fig. 5.35a,b Raman scattering process: (a) nonresonant,
(b) resonant, shown for the Stokes shift by exciting a single
phonon. hν and hΩ are the energy of the incident photon
and the phonon energy, respectively
scattering is a two-photon process and the scattering
signal is generally very weak. However, when the inter-
mediate electronic state is not virtual but really present
(Fig. 5.35b), Raman scattering occurs in a resonant
manner with the scattering intensity being substantially
enhanced (resonant Raman scattering). The minimum
content of Raman-active centers required is 0.01–1%
or > 10
19
cm
−3
in nonresonant cases. When the cen-
ters to be detected are present in the surface layer of
samples, the sensitivity is further enhanced (up to 10
6
times) by employing the scheme of surface-enhanced
Raman scattering [5.28, 29], which exploits a dramatic
increase of the optical electric field in the vicinity of
metallic nanoparticles such as Ag and Au.
Magnetic Resonance Spectroscopy
Experimental methods based on magnetic resonance of
nuclear or electronic spins are applied to the inves-
tigation of defects and impurities in solids, structural
analysis of molecules, and in some cases, microstruc-
ture analysis.
When electrons and nuclei have an angular mo-
mentum S (where is Planck’s constant), a magnetic
moment
μ =γ S (5.16)
is associated with it. S stands for s = 1/2 for electronic
spins, for j for electronic orbital angular momenta, and
for I, various multiples of 1/2, for nuclear spins. The
coefficient γ is called the magnetogyric ratio defined by
γ ≡
ge
2mc
, (5.17)
where g and m are the g-factor (a numeric constant in
the range 1–3 depending on the origin of the angular
momentum) and the mass of the particle, respectively,
and e is the electronic charge, and c the speed of light.
Quantum mechanically, a spin or a magnetic mo-
ment under a static magnetic field of intensity B
0
(magnetic flux density) has discrete eigenstates. For the
simplest case of S = 1/2, the spin has two states, as
shown by the energy level diagram in Fig. 5.36a, sep-
a)
b) c)
β
α
hω
L
hω
L
M
z
x
y
z
x
y
M
B
0
B
0
B
0
Fig. 5.36a–c Quantum-mechanical picture (a) and classi-
cal vector model (b,c of magnetic resonance illustrated for
an S = 1/2 system. In (a), since the Zeeman-split lower
spin state α, is more occupied than the higher state β,in
thermal equilibrium, the application of a resonant electro-
magnetic wave causes transitions between the two states,
resulting in a net energy absorption. Classically, (b) the
macroscopic magnetization M is the average of the spin
magnetizations precessing with random phase in thermal
equilibrium.
(c) On resonance the spin magnetizations are
bunched and the resultant magnetization starts to flips its
head down, tracing a spiral trajectory in real space
Part B 5.1

230 Part B Chemical and Microstructural Analysis
arated by an energy ω
L
where
ω
L
=γ B
0
(5.18)
is called the Larmor angular frequency. In magnetic res-
onance experiments, the sample is further subject to
a small magnetic field perpendicular to the static field
and alternating at the Larmor frequency, which reso-
nantly induces transitions between the two states. In
Fig. 5.36a, the number of filled circles indicates the
population of the respective states. Since, in thermal
equilibrium, the lower energy state (up-spin state) is
more occupied than the higher energy state (down-spin
state), the spin system absorbs a net energy from the
electromagnetic oscillations.
Classically, a magnetic moment under a static
magnetic field precesses around the axis of the
static magnetic field (normally taken as the z-axis)
at the Larmor frequency, as shown schematically
in Fig. 5.36b. In thermal equilibrium, however, the
precession of the spins cannot be detected because
each precession is random (incoherent) and hence, in
macroscopic samples containing many spins, the mag-
netization of the spin assembly is averaged out leaving
only the z-axis component, due to the excess population
of up spins. An intuitive picture of magnetic resonance
is such that the spins become bunched coherently under
a resonant microwave and the resultant magnetization,
represented by a vector shown in Fig. 5.36c, flips its
head down drawing a spiral trajectory. Although this
classical vector model is rarely used in electron spin
resonance (ESR) or electron paramagnetic resonance
(EPR), it is repeatedly applied to interpretation of nu-
clear magnetic resonance (NMR). (Although ESR and
EPR are often used interchangeably in textbooks, the
term ESR in this chapter refers to a generic methodol-
ogy including EPR.)
What makes magnetic resonance informative for
structural studies of materials is the interactions of var-
ious origins operating between spins. The magnetic
moments associated with spins exert an effective mag-
netic field on the dipole moment of other spins (classical
dipole–dipole interaction). The Pauli principle gives
rise to a quantum-mechanical interaction between two
electronic spins (exchange interaction). The behavior of
a general system consisting of electron spins and nu-
clear spins interacting with each other is described by
the effective spin Hamiltonian formally written as
H = B
0
γ
e
S−2SJ
e
S+SAI −B
0
γ
n
I
+IJ
n
I +IQI +··· . (5.19)
Here S and I are now quantum operators of to-
tal electronic spins and nuclear spins, respectively.
The tensorial magnetogyric ratios γ
e
[slightly modi-
fied from (5.17) by spin–orbit coupling, the detail of
which is given in Sect. 5.4.2]andγ
n
or the correspond-
ing tensorial g-factors describe the Zeeman splitting,
respectively, J
e
the direct exchange interaction between
spins (slightly modified by spin–orbit coupling), J
n
the
nuclear spin–spin indirect interaction, A the effect of the
environment of the spins (e.g., anisotropic crystal field),
Q the nuclear quadrupole interaction between the elec-
tric quadrupole moment associated with I > 1/2 nuclei
and an electric field gradient at the nuclear position. Due
to the large difference between the particle mass of elec-
trons and nuclei, γ
e
γ
n
. Therefore, electron spins feel
only small perturbations by nuclear spins, but the EPR
spectra exhibit quite informative fine structures arising
mainly from the third term of (5.19). On the contrary,
since the presence of electronic spins has enormous ef-
fects on the nuclear spin states, NMR spectra become
complicated in paramagnetic samples.
In the absence of electronic spins, the interaction
of nuclear spins with the environment is so weak that
the nuclei have a long spin lifetime, a quantity express-
ing how long the spin coherence once established is
maintained as long as the nuclei are stationary in space.
In other words, the decrease of nuclear spin lifetime
with dynamic motion of the nuclei gives ample infor-
mation of the dynamics. This unique feature of NMR
provides the basis of NMR studies of impurity diffu-
sion in solids (Sect.5.3.1) and of molecular architecture
(Sect. 5.4.2).
There are many other experimental methods
that may be classified as magnetic resonance spec-
troscopy. Optically detected magnetic resonance
(ODMR) (Sect. 5.3.1), magnetic circular-polarized
emission (MCPE) (Sect. 5.3.1), and magnetic circular
dichroic absorption (MCDA) (Sect. 5.3.1) are optical
spectroscopic versions of EPR. Muon spin resonance
(μSR) (Sect. 5.3.1) and perturbed angular correlation
(PAC) (Sect. 5.3.1) may be regarded as NMR of
exotic or radioactive particles. However, these tech-
niques will be described in a more relevant section
(Sect. 5.3.1).
Electron Spectroscopy
Electron Energy-Loss Spectroscopy (EELS). Nowadays,
transmission electron microscopes are often equipped
with an electron energy spectrometer for routine anal-
ysis of electron energy loss. Like photons, electrons
injected into solid samples lose their energy by ex-
Part B 5.1

Nanoscopic Architecture and Microstructure 5.1 Fundamentals 231
citing quanta of various forms, but the main loss
arises from excitation of other electrons. Among them,
the excitation of plasmons with a loss energy of
≈20 eV is the most efficient process, whereas this
is not the case in photoabsorption, determining the
limited penetration power of the electrons through
the sample. The plasmon energy is not sufficiently
sensitive to the material, so the plasmon loss does
not give significant information other than the thick-
ness of the thin samples used for TEM observations.
If the energy of incident electrons exceeds the en-
ergy necessary for a core electron excitation, another
energy-loss mechanism takes place. Since the electron
energy used in TEM is high enough, the correspond-
ing thresholds are observed in the EELS spectrum,
which is a common technique for element analysis by
TEM.
X-Ray Electron Spectroscopy. On core excitations by
x-rays, electrons may be emitted from of the solid via
two different mechanisms. Direct photoelectron emis-
sion occurs when the x-ray photon energy is high
enough to excite the core electrons to vacuum levels
(electronic energy levels just outside the solid surface).
The energy of the photoelectrons measured relative to
the Fermi level of the sample, therefore, directly reflects
the energy of the core electrons. Another mechanism is
Auger electron emission that occurs when a hole gen-
erated at the core level is annihilated with an electron
in an upper level. The energy released on the electron–
hole annihilation is spent either by creation of a photon
(x-ray fluorescence) or an excitation of another elec-
tron (Auger electron) to a vacuum level. The processes
are competitive but the Auger process dominates in
elements lighter than Z ≈ 30. Whether through direct
photoelectron emission or Auger emission, when the
energy of the electrons is of the order of 10 eV, the
electronic mean free path is so short due to the plas-
mon loss that they cannot escape from deep positions
in the sample. For this reason, x-ray photoemission
spectroscopy (XPS) and Auger electron spectroscopy
(AES) are surface-sensitive techniques, being power-
ful for studies of surfaces but not suitable for bulk
samples.
Mechanical Spectroscopy
Mechanical spectroscopy [5.30] is the generic term
for characterization methods of anelastic behavior such
as elastic aftereffect, internal friction and ultrasonic
attenuation. Anelastic spectroscopy is a synonym of me-
chanical spectroscopy. In the elastic range, the strain
response to applied stress is composed of the (instanta-
neous) elastic strain ε
e
and the (delayed) anelastic strain
ε
a
, where the ratio ε
a
/ε
e
is indicative of anelasticity.
The measurement time scale is quasistatic for elastic
after effect, below ≈ 10
4
Hz for internal friction and
above ≈10
4
Hz for ultrasonic attenuation. Interaction
of acoustic waves with phonons is out of the present
scope. Thermoelastic relaxation is associated with the
adiabatic temperature change induced by application
of a stress and can be commonly observed in solid
materials, but its magnitude is negligibly small except
in the vicinity of the resonant condition. Mechanical
spectroscopy can be applied to detect the recoverable
motions of/in atoms, lattice defects, interfaces, mag-
netic domain walls and so on when they modify the
strain response. The value of ε
a
/ε
e
is a function of
the anelastic strain for each process and the concentra-
tion of participating agents, which may be a function of
the strain amplitude ε, frequency f and temperature T .
For example, the value of ε
a
/ε
e
at room tempera-
ture is of the order of 10
−7
in silicon single crystals,
10
−1
in highly damping metals and more than unity in
polymers.
The anelastic relaxation is characterized by the re-
laxation time τ and ε
a
/ε
e
, where the relaxation peak
exhibits a maximum at ωτ =1(ω = 2π f ). Thermally
activated anelastic relaxation is studied as a function
of temperature or frequency. The elastic aftereffect
is isothermally measured as a function of elapsed
time, where τ and ε
a
/ε
e
at a given temperature are
found directly. In subresonance measurements, inter-
nal friction is isothermally measured as a function of
frequency, e.g., in the range between 10
−3
and 10 Hz.
These methods are applicable for ε
a
/ε
e
> 10
−5
be-
cause of limitations on experimental accuracy. It is
emphasized that these methods can also be applied
to materials with ε
a
/ε
e
> 1. The resonance meth-
ods are applicable to cases of ε
a
/ε
e
< 10
−1
, where
internal friction is measured as a function of tem-
perature. These methods are applicable to thin plates
or rod specimens. Ultrasonic attenuation is applica-
ble to bulky specimens, where the specimen length
should be longer than the pulse length. In Sects. 5.3.1
and 5.3.2, some more detail of internal friction is
described.
Part B 5.1

232 Part B Chemical and Microstructural Analysis
5.2 Crystalline and Amorphous Structure Analysis
The subject covered in this section will be structural
analysis of ordinary metallic and nonmetallic crystalline
solids, molecular crystal, liquid crystals, amorphous
solids, and quasicrystals. The subsections are divided
according to the extent of structural order in space.
Surface-specific problems are beyond the scope of this
chapter.
5.2.1 Long-Range Order Analysis
Single Crystal Analysis
As mentioned in Sect. 5.1.1, the structure factor F(K)
in wavenumber space is related to the electronic den-
sity ρ(r) in real space through a Fourier transformation.
Since the electrons are concentrated at the atomic
positions, knowing the electronic density ρ(r)isto
know the crystal structure. In principle, therefore, the
crystal structure can be deduced by the inverse Fourier
transformation of the structure factors if known exper-
imentally. In practice, however, the only quantities we
can measure in experiments are not F(g) = F
g
exp
iφ
g
but
|
F(g)
|
2
(hereafter, reciprocal lattice vectors g in
structure factors implicitly indicate the structure fac-
tors integrated over the broadening width), which means
that we lack the knowledge of the phase factors φ
g
to
calculate the electronic distribution immediately. This
phase problem is the main difficulty in structural anal-
ysis of x-ray diffraction, to which much effort has been
devoted. The standard analytical methods to tackle this
problem are the direct method, the heavy atom method
and the anomalous dispersion method.
The direct method is based on a general relation
(Sayre’s formula)
F(h) =a
g
F(g)F(h−g) a : a real coefficient
(5.20)
which holds for arbitrary structure factors. In many
practical cases, diffraction intensities are concentrated
in a limited number of diffraction spots. In the sim-
plest case, where a single term containing a specific g
predominates in the sum,
F(h) ≈aF(g)F(h −g) , (5.21)
we have a phase relationship of
φ
h
≈φ
g
+φ
h−g
(5.22)
approximately. Thus, having assumed two phase values,
one could evaluate the other and so on, successively. In
the actual procedure, starting with an initial set of phase
values for dominant diffractions, one repeats an iterative
procedure to recalculate the phase values until conver-
gence is achieved. Such procedures are attempted for
many initial sets of F(g) = F
g
exp
iφ
g
, or model struc-
tures that yield the sets, until the best fit with experiment
is achieved.
The heavy atom method is based on the fact that
the atomic scattering factor f (K) increases with the
number of electrons in the atom and hence the atomic
number. This method can be efficient in those crystals
containing metal complexes in which the structure fac-
tors are determined mainly by the arrangement of the
heavy metal atoms. Exploiting this situation, we could
estimate the approximate positions of the heavy atoms
in the unit cell. The experimentally measured inverse
Fourier transform of |F(g)|
2
is called the Patterson
function, the autocorrelation function of the electron
density ρ(r),
P(u) =
ρ(r)ρ(r +u)dv. (5.23)
Therefore, the Patterson function has strong peaks at
relative positional vectors connecting two heavy atoms
in real space. From these peaks one could deduce the
real positions of only the heavy atoms in the unit cell
(for more details, see the relevant textbooks [5.8]). The
heavy atoms may be intentionally introduced in the pro-
cess of crystal growth or in the synthesis of chemicals
for macromolecules that are to be grown into crystalline
samples. Chemical substitution is a method commonly
used in structural analysis of biomolecules (Sect. 5.4.1).
Instead of substituting atoms, in some cases the
anomalous dispersion effect can be used. Anomalous
dispersion is a phenomenon in which the complex
atomic structure factor changes considerably at wave-
lengths around the core excitation edge. Therefore, if
we use x-rays of an appropriate wavelength, we can
effectively substitute atoms with different atomic scat-
tering factors. Since the advent of synchrotron radiation
sources, it has become possible to use strong monochro-
matic x-rays tunable to a specific wavelength so that the
anomalous dispersion method now constitutes a power-
ful technique applicable to many materials.
In addition to the phase problem, measurements of
F
obs
(K) for only a limited number of K vectors could
lead to spurious results due to a cut-off effect in the
inverse Fourier transformation. These difficulties are
now being overcome by the application of the maxi-
Part B 5.2
Nanoscopic Architecture and Microstructure 5.2 Crystalline and Amorphous Structure Analysis 233
mum entropy method (MEM)[5.31], a state-of-the-art
technique that allows very precise analysis of diffrac-
tion data based on the method of least presumption and
structural models of the crystal.
Regarding the sample, the availability of a single
crystal is an essential prerequisite. As shown in (5.8),
the intensity of the diffracted x-rays depends linearly
on the number of atoms or the sample volume, in other
words, it decreases rapidly as the third power of the
sample dimension. If we use a conventional sealed x-ray
tube, the size of single crystals must be larger than
0.1 mm, but if a synchrotron radiation source is avail-
able, the sample size may be reduced by one order of
magnitude. The crystal quality must be high enough that
peak broadening due to imperfections is small enough
to allow peak separation. This is of particular impor-
tance in molecular crystals because the lattice constant
of molecular crystals is likely to be so large that the
diffraction peaks crowded one another.
The samples are mounted on a goniometer as shown
in Fig. 5.37 and rotated around three Eulerian axes. Usu-
ally the ω–2θ (θ–2θ) scan scheme is employed: the
angles φ and χ are adjusted so that the sample can
be rotated around a zone axis coincident with the ω-
axis by increasing the incidence angle θ step by step
while simultaneously rotating the detector by 2θ so as
to maintain specular reflection conditions.
Powder Diffraction
Single crystal diffraction experiments using a relatively
large crystal give a data set from which we could deduce
the crystal structure with high accuracy. However, since
most materials of technical interest cannot grow to large
single crystals, one has to resort to the use of powder
samples consisting of small crystallites. At this point,
one might suspect that, instead of using crystalline sam-
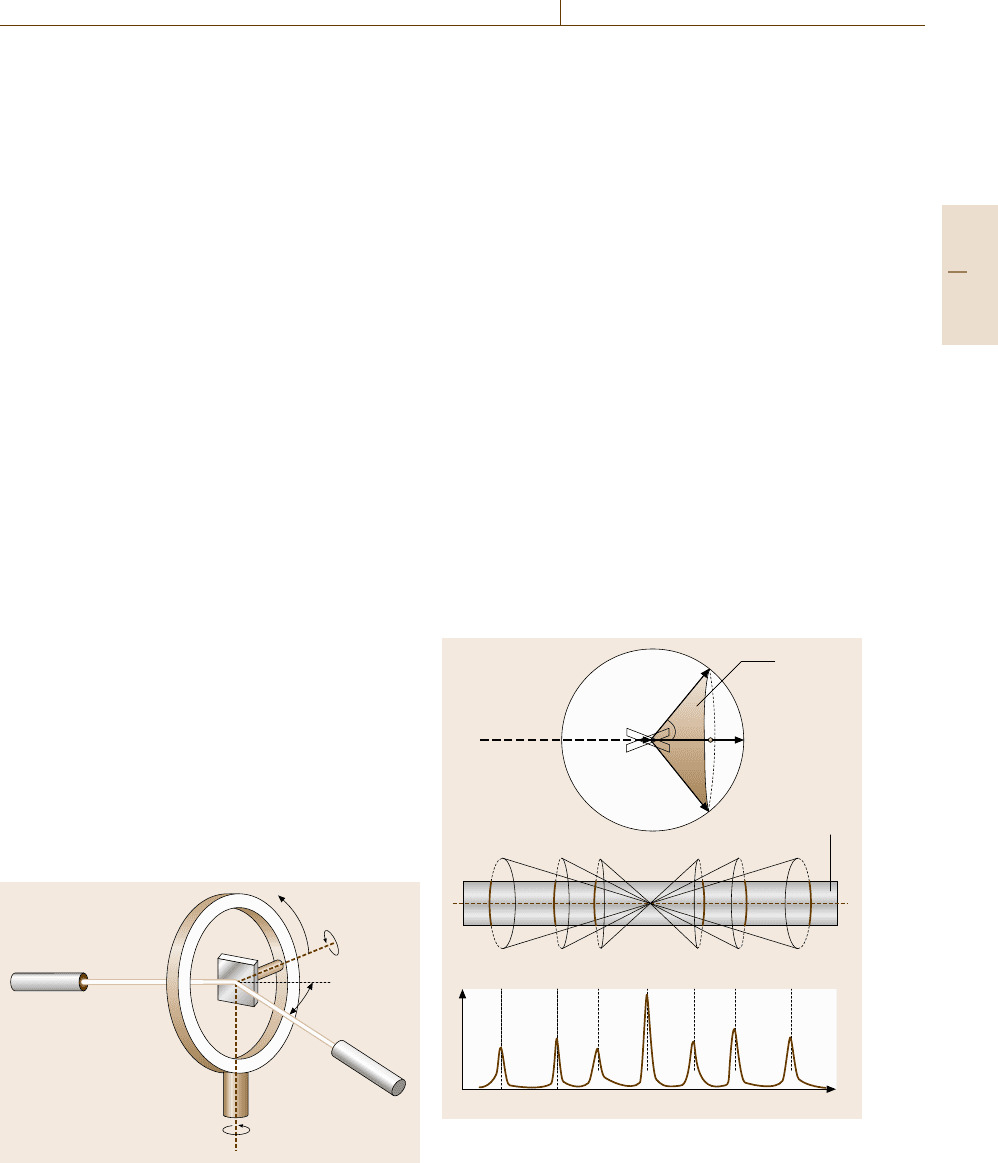
Beam source
Detector
χ
2θ
φ
ω
Fig. 5.37 Rotation angles in a four-circle goniometer
ples, one could use amorphous samples as the extreme
of small crystallites. This view is totally wrong because
for the Bragg diffraction, which is a consequence of
constructive interference, to occur, the crystal must have
a finite size. For crystal structure determination by pow-
der diffraction, the crystallite size should preferably be
larger than the micron scale. X-ray analysis of short-
range order in amorphous solids will be described in
Sect. 5.2.3.
In powder diffraction, the reciprocal lattice is ran-
domly oriented with respect to the incident x-ray beam
and the Ewald sphere. As a consequence, the x-ray
diffraction by crystallographically equivalent lattice
planes occurs in directions forming a cone with a com-
mon diffraction angle 2θ, as illustrated in Fig. 5.38a.
The diffraction pattern thus obtained consists of rings
(Debye rings) of different diameters the values of
which are related to the interplanar spacings in the
Bragg condition (5.1). The historical method of study-
ing the Debye rings is to use a Debye–Scherrer camera
(Fig. 5.38b) in which a slice of Debye rings is recorded
on a strip of x-ray film placed around the sample. In
modern experiments, the x-ray film is replaced with an
x-ray detector turning on a diffractometer (Fig. 5.38c).
If the Debye rings or diffraction peaks are properly in-
a)
b)
c)
Diffraction
cone
2υ
k
0
k
X-ray film
2υ
Counts
Fig. 5.38 (a) Ewald construction for powder diffraction,
(b) Debye–Scherrer camera and (c) diffraction profile
recorded by a diffractometer
Part B 5.2
234 Part B Chemical and Microstructural Analysis
dexed with knowledge of the crystal structure and the
extinction rule, the lattice parameter of the crystal can
be determined with accuracy higher than 10
−6
.
The sample requirements for powder diffraction
measurements are as follows.
1. The sample must be an assembly of crystallites each
smaller than ≈10 μm (ideally ≈3 μm).
2. Individual crystallites must be oriented randomly.
Condition 1 is important because, if exceptionally
large crystallites are present, they introduce bright spots
in the Debye rings (as exemplified in Fig. 5.99), which
introduces a spurious diffraction profile. Similarly, if the
orientational randomness is lost, it is impossible to mea-
sure correct profiles. In such cases, the sample must
be rotated to remove such artifacts. For assessment of
orientationally textured materials see Sect. 5.5.1.
The main drawback of the powder diffraction
method compared to methods using single crystals is
the overlap of diffraction peaks that prevents the ac-
curate determination of the integrated intensity of each
reflection and hence the structure factor. The Rietveld
method, which allows an effective separation of these
overlapping peaks, is a standard technique routinely
used nowadays in the analysis of powder diffraction
data. For the phase problem, the power of MEM
(Sect. 5.2.1) in the analysis of x-ray powder diffraction,
combined with the Rietveld method, has also been pro-
gressively discovered [5.33]. Using powder diffraction,
one can determine the structures of considerably large
molecules such as metmyoglobin, the single crystal unit
cell of which contains two molecules (≈ 5000 atoms)
and ≈800 waters [5.34].
Selected Area Diffraction (SAD)
The general merit of using TEM is that one can in-
vestigate solid structures at a medium-scale range, but
most of this matter is more relevantly addressed in
the section on microstructure analysis (Sect. 5.5). Se-
lected area diffraction in TEM corresponds to single
crystal diffraction of monochromatic x-rays, displaying
a two-dimensional projection of the reciprocal lattice
in the zeroth-order Laue zone (ZOLZ). In this re-
gard, there is no great difference in principle between
x-ray diffraction and SAD. Nevertheless, the real-time
graphic presentation of the reciprocal lattice in SAD
may give us more chance to notice subtle changes in
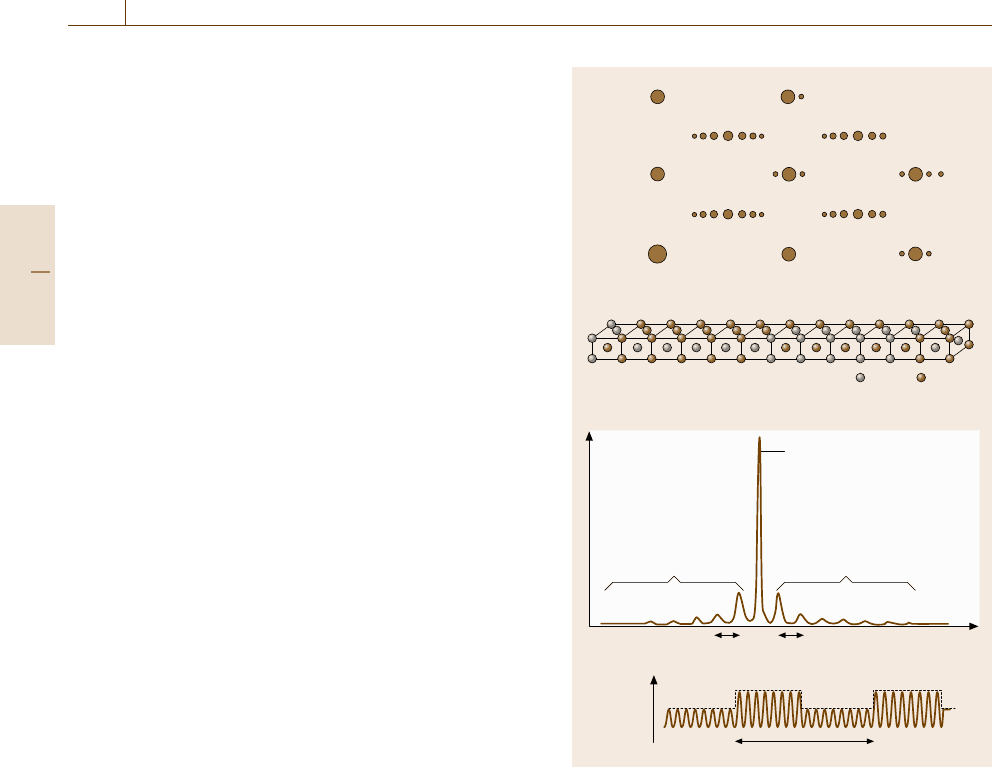
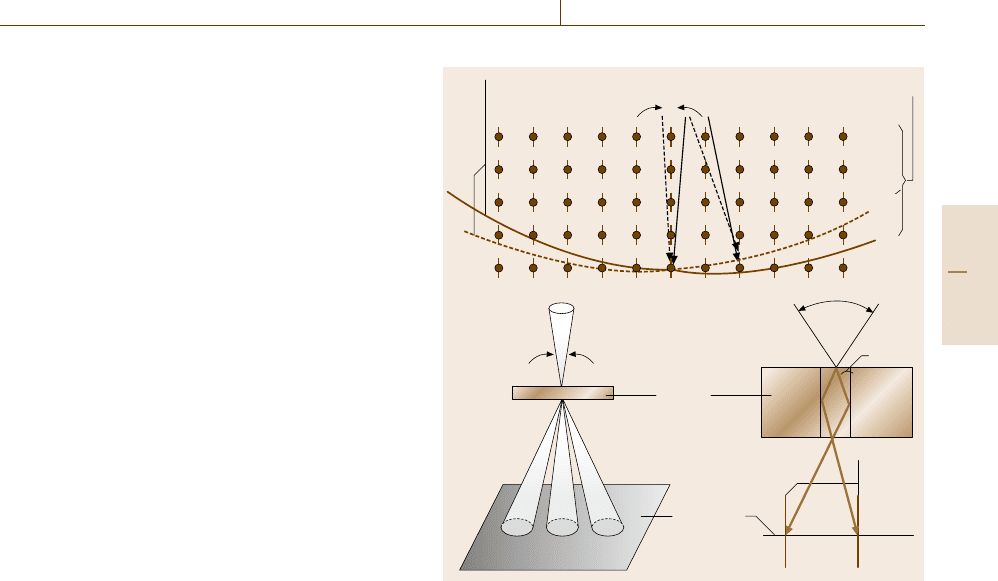
crystal ordering visually. Figure 5.39ashowsaSAD
pattern of an CuAu stoichiometric alloy ordered with
a superperiodicity (b). The SAD pattern is characterized
by satellite spots which are absent when the crystal is
Diffraction intensity
K
b)
c)
a)
d)
400
200
000
Satellites
1/Λ
Š (r)
1/Λ
Λ
Satellites
Bragg
reflection
420
310 330
110 130
220 240
040
AuCu
020
Fig. 5.39a–d Superlattice reflections (a) in the super-
periodic ordered phase of CuAu stoichiometric alloy.
(b) After [5.32] and Fourier transform (c) of a periodically
modulated sinusoidal wave (d)
ordered but not in the long range. The origin of the satel-
lites may be understood by examining Fig. 5.39c, which
illustrates the Fourier transform of a periodically modu-
lated sinusoidal wave (d) that mimics the superperiodic
structure.
Similar satellites can be observed by x-ray diffrac-
tion of artificial superlattice thin films grown by the
molecular beam epitaxial method. For the superlat-
tice spots to be observed, x-ray diffraction experiments
are conducted in a reflection configuration, where the
x-rays incident to the front side of the films are
Bragg reflected by lattice planes that are parallel to
the film surface, as shown schematically in Fig. 5.2
(Sect. 5.1.1).
Part B 5.2
Nanoscopic Architecture and Microstructure 5.2 Crystalline and Amorphous Structure Analysis 235
5.2.2 Medium-Range Order Analysis
Convergent Beam Electron Diffraction (CBED)
Although the selected area diffraction (SAD) (Sects.
5.1.2 and 5.2.1)inTEM gives us structural informa-
tion of the local parts (> 0.5 μm in size) of the sample
selected by the objective aperture, the crystallographic
information is limited compared with x-ray diffraction.
The convergent beam electron diffraction (CBED)is
even more informative than x-ray diffraction in some
cases. In contrast to the parallel electron beam used in
SAD, the electron beam used in CBED is focused onto
a sample with a convergence semi-angle α>10 mrad.
Figure 5.40 illustrates how CBED patterns are formed
in reciprocal space (a) and in real space (b). The
beam convergence not only improves the spatial res-
olution down to tens of nanometres but also changes
substantially the information contained in the diffrac-
tion patterns if the sample thickness t is larger than
the extinction distance ξ
g
, the case in which the dy-
namical effect (Sect. 5.1.2) must be taken into account.
Figure 5.41 shows a schematic CBED pattern: the zero-
order Laue zone (ZOLZ) diffraction spots in SAD are
observed as disks in CBED. If the convergence angle 2α
is large enough, diffraction is also excited from higher-
order Laue zones (HOLZ) outside of the ZOLZ disks
(HOLZ is the general term for the first-order Laue zone
(FOLZ), the second-order Laue zone (SOLZ), and so
on). An effect of multiple reflections in CBED is the
characteristic dynamical contrasts within the disks that
are absent when t <ξ
g
. In kinematical x-ray diffraction,
centrosymmetric crystals and noncentrosymmetric ones
cannot be distinguished because (h, k,)and(
¯
h,
¯
k,
¯
)
diffraction spots are, in any case, observed with the
same intensity. In CBED, however, the diffraction pat-
tern exhibits the same symmetry as the point group of
the crystal due to the dynamical effect, so that one may
fully determine the point group symmetry from a set of
CBED patterns obtained for different zone axes.
Measurements of lattice parameter by SAD is not
as accurate (≈ 2%) as in x-ray diffraction even if the
camera length, which directly affects the diameter of
the diffraction rings on the screen, is calibrated care-
fully by using a reference crystalline powder (e.g., Au).
However, the accuracy could be enhanced to ≈ 0.2%
by measuring the positions of sharp CBED Kikuchi
lines, another effect of dynamical diffraction in CBED.
When the convergence semi-angle α is larger than the
Bragg angle θ
B
(Fig. 5.40c), some electrons in the beam
may already satisfy the Bragg condition and hence be
reflected by the net planes to form Kikuchi lines sim-
b)
a)
c)
Ewald
sphere
HOLZ
TOLZ
SOLZ
FOLZ
ZOLZ
Sample
2α
2α
2α
θ
B
k
0
k
Back focal
plane
Kikuchi
lines
Fig. 5.40a–c Convergent beam electron diffraction (CBED)inre-
ciprocal space (a) and in real space (b).Ifα>θ
B
(Bragg angle),
CBED Kikuchi lines are formed (c)
ilar to those also observed in SAD.However,while
the Kikuchi lines in SAD originate from inelastic scat-
tering of incident electrons, the CBED Kikuchi lines
are formed by elastically scattered electrons. There are
twotypesofCBED Kikuchi lines, those bisecting each
ZOLZ disk (ZOLZ Kikuchi lines) and those arising
from HOLZ diffractions (Fig. 5.41). The Kikuchi lines
of particular importance here are the deficient (dark)
Kikuchi lines observed within the direct (0, 0, 0) disk,
which have their origins in the HOLZ Kikuchi lines.
The positions of the HOLZ Kikuchi lines are more sen-
sitive to lattice strain than ZOLZ Kikuchi lines because
the larger |g|=1/d (d: interplanar spacing), the larger
its shift Δ|g|=−(Δd/d)|g|, which means the higher
sensitivity to strain Δd/d. The sharpness of the CBED
Kikuchi lines due to the smallness of the sampled area
also serves to enhance the strain sensitivity.
Methods for Paracrystalline Solids
The term paracrystal is used as a model of disorder in
crystalline solids. In this chapter, we use this term in
a broader sense to mean materials that are intermediate
between three-dimensionally periodic crystals and com-
Part B 5.2
236 Part B Chemical and Microstructural Analysis
HOLZ
reflections
Deficient
HOLZ
Kikuchi lines
ZOLZ disks
ZOLZ
Kikuchi
lines
(0, 0, 0) Disk
Fig. 5.41 A schematic CBED pattern featured by zero-
order Laue zone (ZOLZ) disks, deficient HOLZ Kikuchi
lines in the direct (0, 0, 0) disk, and reflections from higher-
order Laue zones (HOLZ)
pletely amorphous structures. Most of the largest size
macromolecules are polymers which are formed by the
linear repetition of a group of atoms called a monomer.
A polymer has no fixed configuration but takes an al-
most infinite variety of forms. Due to the flexibility of
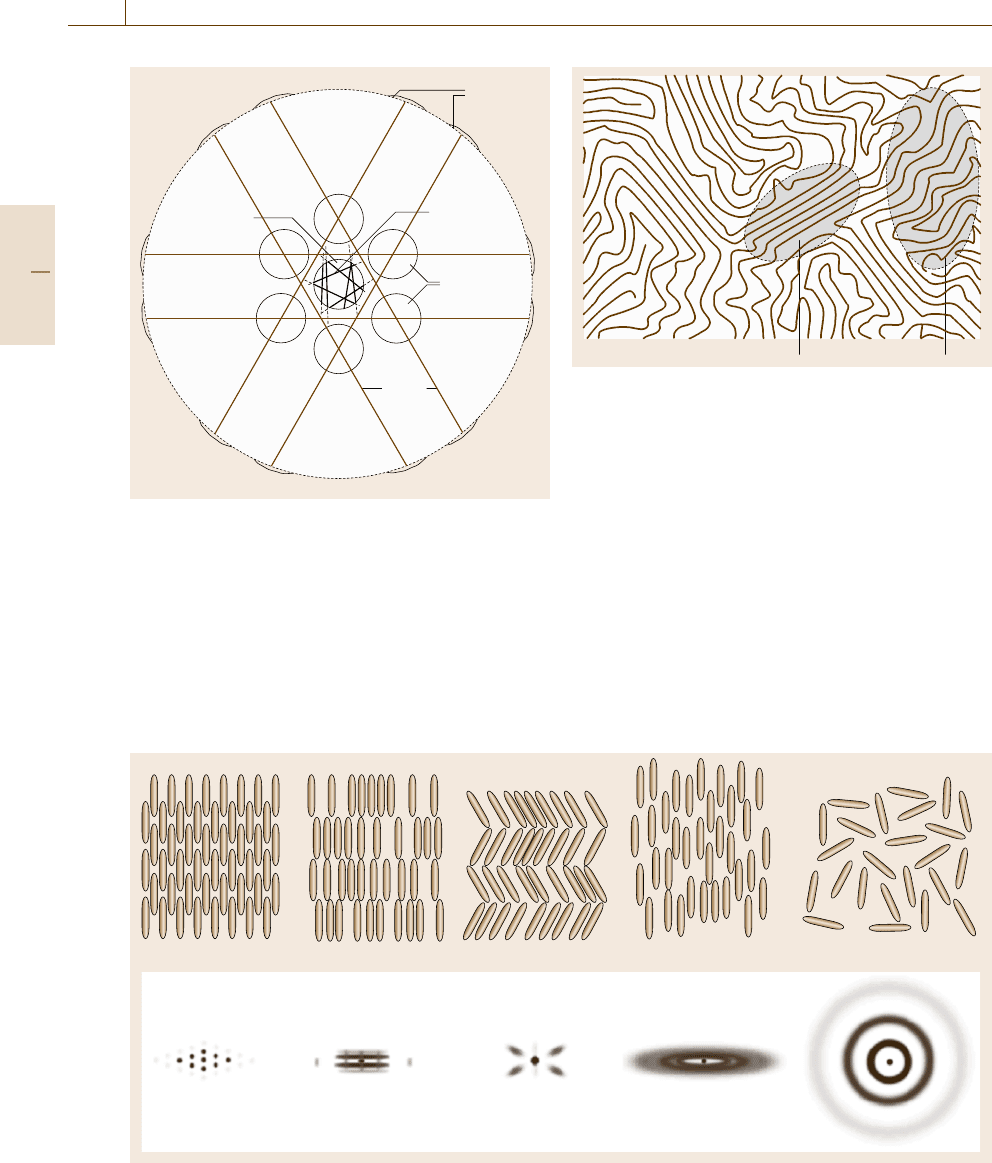
Crystal Smectic-A Smectic-C Nematic Amorphous
Fig. 5.43 Liquid crystal phases and their diffraction patterns (schematic)
Crystalline region Amorphous region
Fig. 5.42 Structure of polymer solid with coexisting crys-
talline and amorphous domains
polymeric chains and the tendency for mutual entangle-
ment, polymers are likely to solidify in an amorphous or
glassy form and are difficult to crystallize perfectly. So
it is common that the ordered structure, if present, ex-
tends only to a limited range, as illustrated in Fig. 5.42.
For the assessment of the medium-range order, one of
the most common methods is to use interference optical
microscopes, in which phases having different optical
properties in terms of refractive index, birefringency,
the rotary power, etc. are imaged as different contrasts
or colors.
Between completely three-dimensional crystals and
glasses, some molecular solids have structures in which
Part B 5.2
Nanoscopic Architecture and Microstructure 5.2 Crystalline and Amorphous Structure Analysis 237
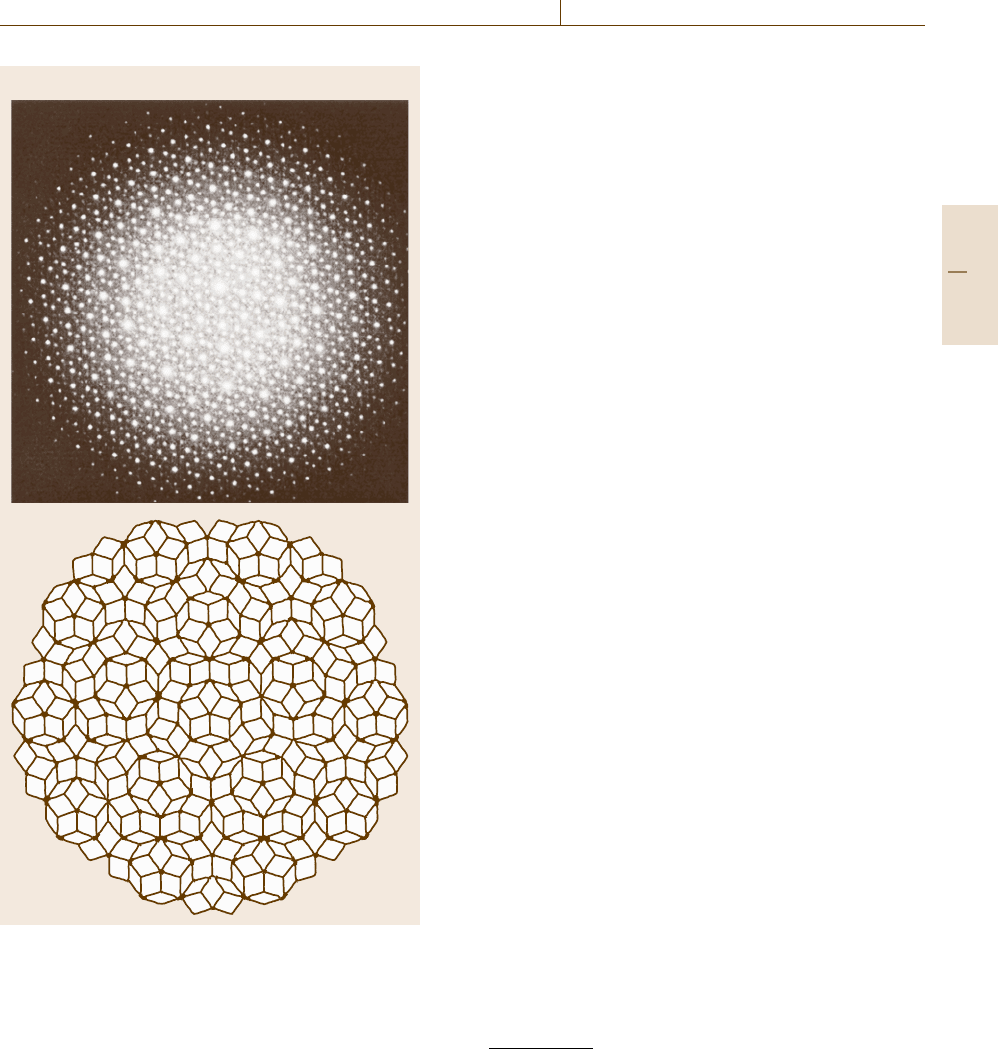
a)
b)
Fig. 5.44a,b SAD pattern of a quasicrystal (a) and the
model structure (b). Courtesy of Dr. K. Suzuki
the crystalline order is lost in some dimensions. Solids
belonging to this category are called liquid crystals.
The molecules in liquid crystals are commonly elon-
gated rigid rods with a typical length of ≈2.5nm and
a slightly flattened cross section of 0.6nm×0.4nm.
The upper drawings in Fig. 5.43 show various forms
of liquid crystals in different degrees of order. The
crystalline phase on the left and the amorphous phase
on the right are completely ordered and disordered,
respectively, in terms of both molecular position and
orientation. In the so-called nematic phase in between,
the molecules are orientationally ordered but position-
ally disordered, and in smectic phases, the molecules
are orientaitonally ordered forming layers but the mo-
lecular positions are random within each layer. The
lower drawings in Fig. 5.43 illustrate the schematic
x-ray diffraction patterns of the aforementioned phases.
Thus, we may judge the phase from its characteristic
diffraction pattern.
Quasicrystals are noncrystalline solids condensed
with no periodicity that are not amorphous but that have
regularity different from crystals. Quasicrystals consist
of unit cells with five-fold symmetry which is incom-
patible with any crystalline long-range order. In spite
of the absence of crystalline order, however, quasicrys-
tals exhibit clear diffraction, as demonstrated by the
SAD pattern in Fig. 5.44a. This is due to the fact that,
even if the crystalline periodicity is absent, there are
atomic net planes regularly spaced as one may find in
the model structure of a two-dimensional quasicrystal
showninFig.5.44b.
5.2.3 Short-Range Order Analysis
Diffraction Methods
Although the atomic arrangement in amorphous solids
is extremely disordered, it is not completely random as
in a gas phase but preserves a short-range order to some
extent. This is evidenced by halo diffraction rings from
amorphous solids, as shown in Fig. 5.45. For amorphous
solids, the intensity of the diffracted wave is simply
givenby(5.6) where the unit cell extends over the whole
solid. Hence
I(K) = I
e
j
f
j
(K)exp
iK ·r
j
2
= I
e
m,n
f
m
(K) f
n
(K)exp
[
iK ·(r
m
−r
n
)
]
.
(5.24)
For single component solids ( f
m
= f
n
≡ f ) consist-
ing of N atoms, some calculations show that
K
I(K)/N − f
2
f
2
=4π
∞
0
r [ρ(r) −ρ
0
] sin Kr dr ,
(5.25)
where ρ(r) is the radial distribution function, which is
defined as the mean density of atoms located at dis-
tance r from an atom, and ρ
0
denotes the average
density of atoms. The above equation means that the
Part B 5.2
