Czichos H., Saito T., Smith L.E. (Eds.) Handbook of Metrology and Testing

Подождите немного. Документ загружается.

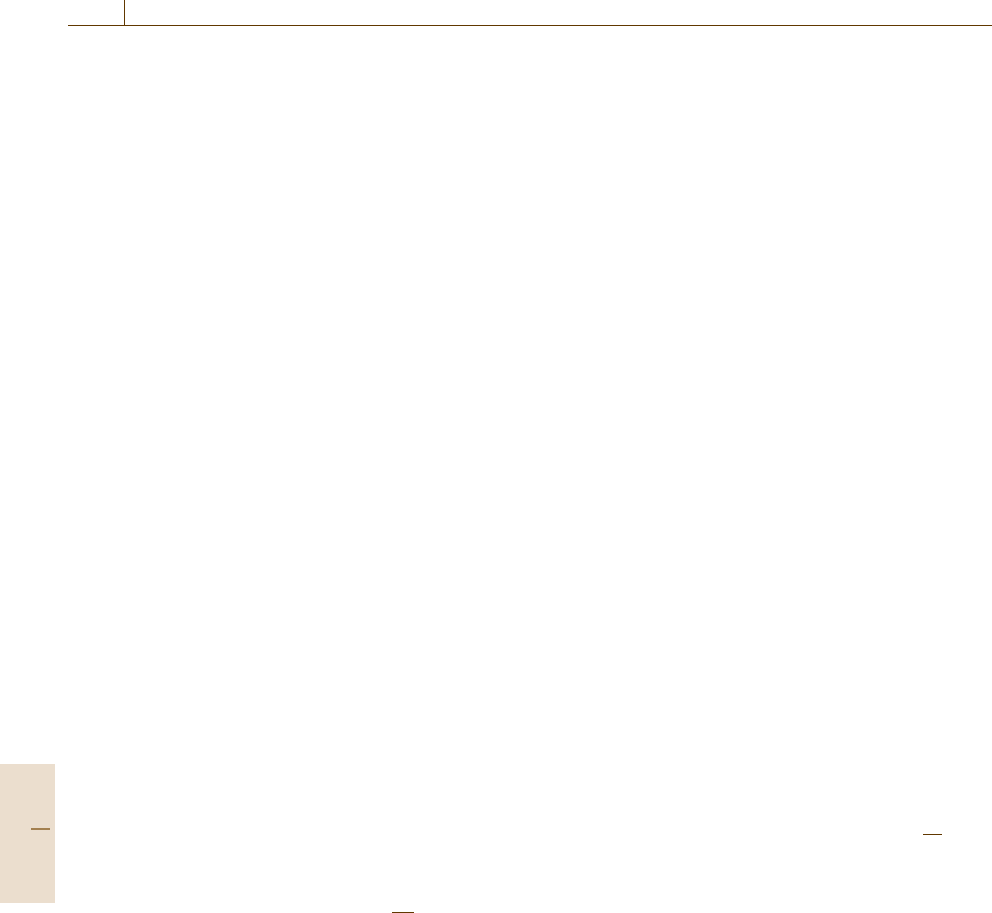
1138 Part E Modeling and Simulation Methods

easy. It is shown by the ε expansions of renormaliza-

tion group (RG) that the superconductivity to normal

phase transition should be ﬁrst order,uptothelow-

est order of ε [22.135]. This result is natural since the

melting of the ﬂux-line lattice is understood to be simi-

lar to most other melting phenomena in reality (d = 3).

However, the upper critical dimension for the supercon-

ductivity vortex state is d

=6, above which mean-ﬁeld

theory is correct, since thermal ﬂuctuations in the two

dimensions perpendicular to the magnetic ﬁeld are cut

off by the vortex spacing and thus cannot contribute

to the long-range order, noticing d

= 4 for supercon-

ductivity without magnetic ﬁeld. The treatment on RG

ﬂows is therefore quite hard using quantities only to the

lowest order of ε = d

−d = 3.Asamatteroffact,the

conclusion on the ﬁrst-order melting transition of vortex

lattice from RG was challenged by others.

22.5.1 Model Hamiltonian

This situation motivated many computer simulation

works. As the melting temperature is much lower than

(B), where the Meissner effect sets on, it is a rea-

sonable approximation to neglect ﬂuctuations in the

amplitude of the complex order parameter when the

melting transition is concerned. In addition, the high-

superconductors are extremely type-II, in which the

penetration length of magnetic ﬁeld is much larger than

the correlation length. This permits one to take the

magnetic induction uniform and equal to the applied

magnetic ﬁeld.

With these two approximations we can de-

rive the following three-dimensional frustrated XY

model [22.136, 137] from the Ginzburg–Landau (GL)

Lawrence–Doniach (LD) free-energy functional for lay-

ered superconductors [22.138,139]

H =−



i, j

cos

⎛

⎜

⎝

−ϕ

−

2π

A· d r

⎞

⎟

⎠

(22.82)

Namely, the main effects of thermal ﬂuctuations come

from the phase degrees of freedom of superconductivity

order parameter, which are deﬁned on the simple cu-

bic lattice in simulation. The couplings are limited to

nearest neighbors and J

= J with J = φ

d/16π

for links ij parallel to the superconducting layer while

= J/Γ

for the perpendicular. The lattice constant

along the c direction is the separation between neigh-

boring CuO

layers d, while the lattice constant in

the ab planes l

can be taken in a range satisfying

l

λ

. Then the anisotropy parameter should

be given by the relation Γ l

= γ d if one wants to

simulate a superconductor with anisotropy parameter

γ = λ

/λ

For magnetic ﬁelds parallel to the c axis, one can

set A

=0. The strength of the magnetic ﬁeld is given

in a dimensionless way f ≡ Bl

/φ

. Vortices are ﬁg-

ured out, including vorticity n, by counting the gauge

invariant phase differences around any plaquette



i, j

[ϕ

−ϕ

− A

]=2π(n − f ) . (22.83)

In the ground state, the areal density of vortices should

be equal to f .

In the above model, we assume a ﬁnite amplitude

of local superconductivity order parameter on each site,

which gives the coupling strength. At high tempera-

tures, the phases ﬂuctuate signiﬁcantly and thus a coarse

grain to any length scales larger than that given initially

in the model will result in a vanishing amplitude of su-

perconductivity order parameter. As for the long-range

order of superconductivity, one needs to investigate

the coherence of the phase degrees of freedom in the

present system. The helicity modulus proportional to

the rigidity of the system under an imposed phase twist

as in (22.75) should be taken as the true long-range

superconductivity order parameter.

22.5.2 First Order Melting

Here we present some of our results published

in [22.140–142], with simulation techniques devel-

oped partially in early studies [22.143]. Similar results

are reported by other groups [22.144–146]. For the

convenience of simulation, we take Γ =

√

10 when

modeling the moderately anisotropic high-T

supercon-

ductor YBa

7−δ

of γ = 8. The magnetic ﬁeld is

f = 1/25. The system size is L

× L

=50× 50×

40, where N

= 100 vortices induced by the external

magnetic ﬁeld are contained in each ab plane. Periodic

boundary conditions (PBCs) are set in all the directions.

The conventional Metropolis algorithm is adopted. The

number of MC sweeps at each temperature is 50 000

for equilibration and 100 000 for sampling. Around the

transition temperature, we have simulated up to several

million MC sweeps.

The Speciﬁc Heat

In Fig. 22.17 we display the temperature dependence of

the speciﬁc heat per vortex line per length d evaluated

Part E 22.5

Monte Carlo Simulation 22.5 Superconductivity Vortex State 1139

C (k

per vortex)

T (J/k

)

0 0.2 0.4 0.80.6 1.21 1.4 1.6

Fig. 22.17 Temperature dependence of the speciﬁc heat per

vortex line per length d

via the ﬂuctuation-dissipation theorem

C =



H

−H



. (22.84)

A sharp spike is observed in the speciﬁc heat around the

temperature T

 0.567J/k

. Quantitatively, the spe-

ciﬁc heat is C

 18.5k

just above T

, C

max

 23k

right on T

,andC

−

17.5k

just below T

in a narrow

regime of temperature.

Figure 22.18 displays the variation of the internal

energy around the transition temperature T

. A kink-

like anomaly takes place, and from the two straight

lines in Fig. 22.18 the latent heat is estimated as

Q  0.07k

per ﬂux per layer. The data on the spe-

ciﬁc heat and the latent heat indicate very clearly that

a ﬁrst-order phase transition occurs at T

According to the ﬁnite-size scaling theory for a ﬁrst-

order phase transition [22.1], one has

max

f +

−

, (22.85)

ΔT =

, (22.86)

providing that the system is already large enough such

that the estimate of the latent heat in the way de-

scribed in Fig. 22.18 is accurate enough. It has been

checked that our data satisfy these two relations very

e (J per vortex)

T (J/k

)

0.555 0.5650.56

0.5750.57 0.58 0.585

–38.9

–39

–39.1

–39.2

–39.3

–39.4

–39.5

Fig. 22.18 Temperature dependence of the internal energy

per vortex line per length d

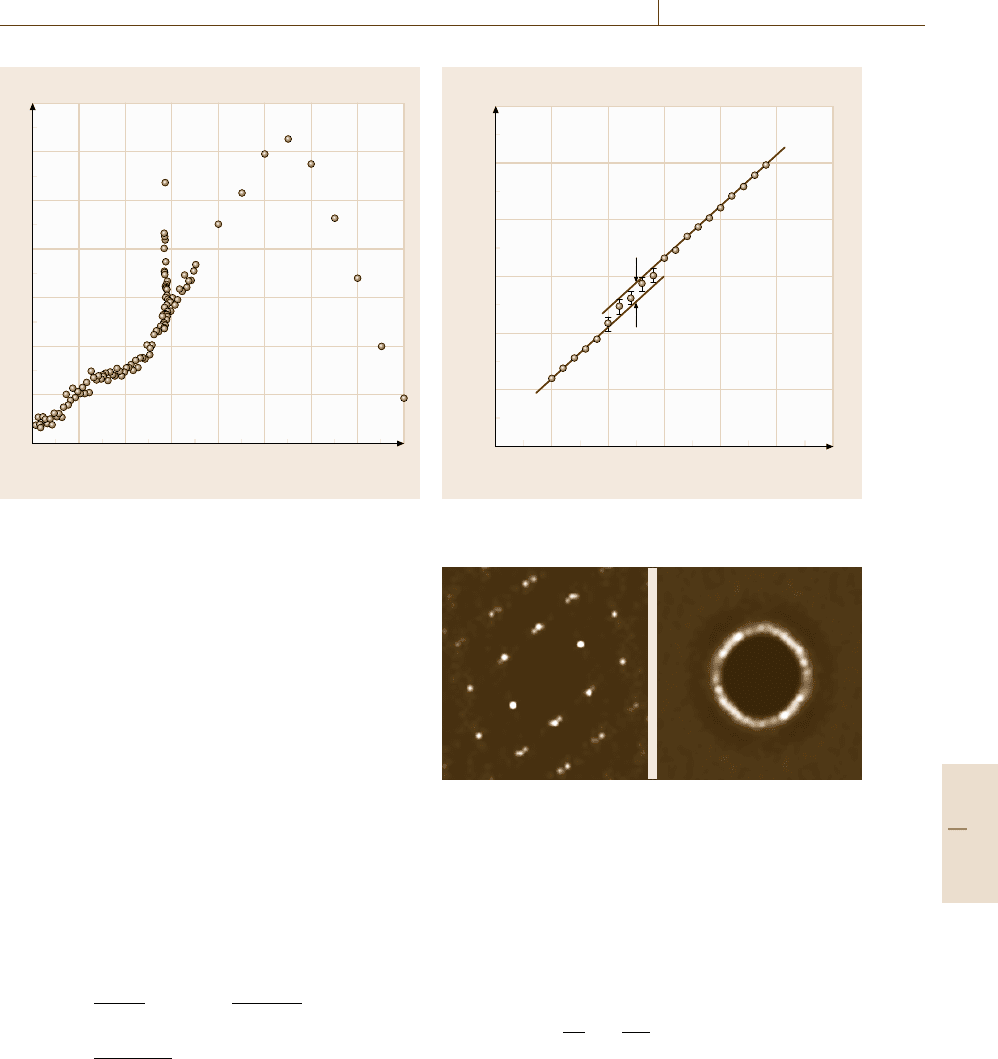
a) b)

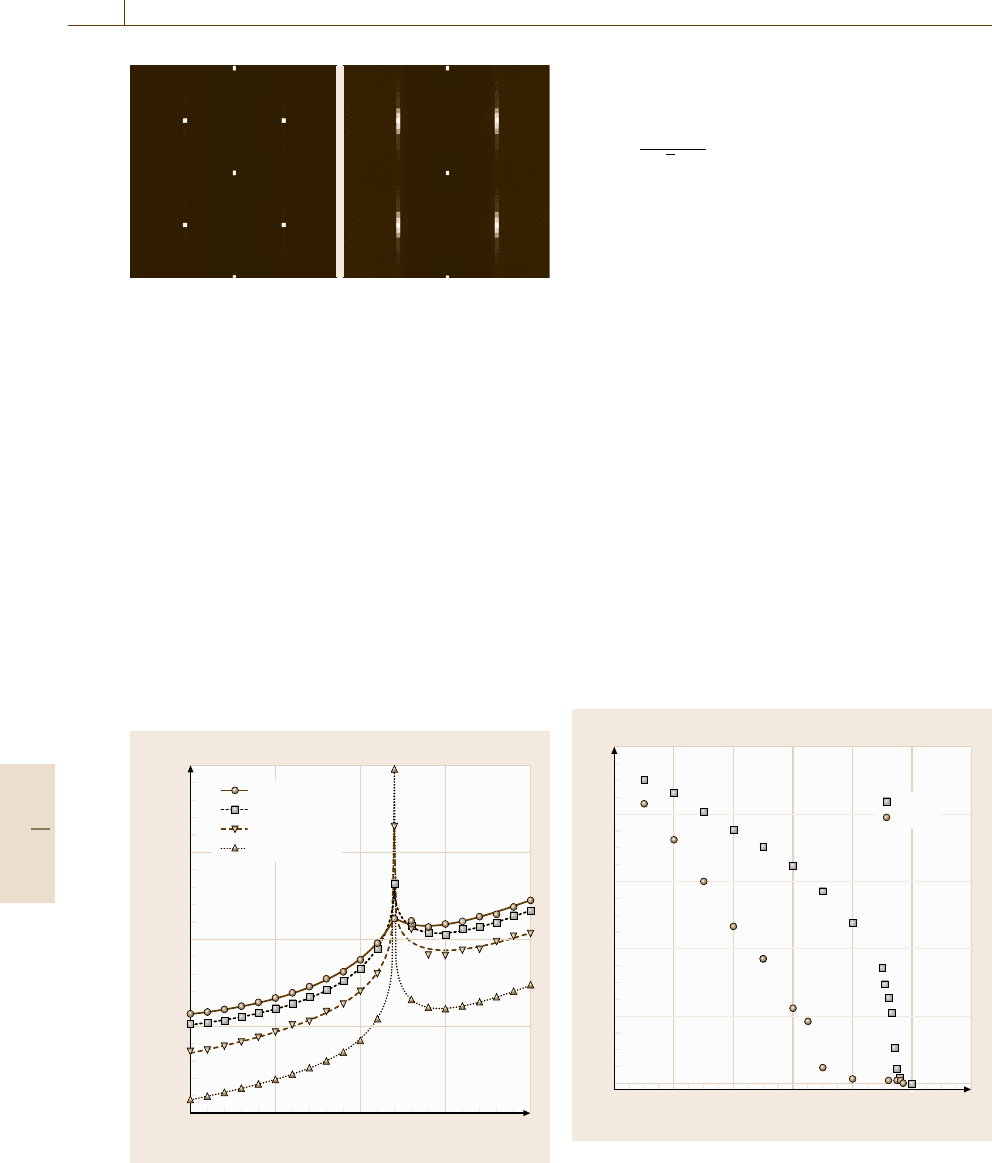
Fig. 22.19a,b Structure factors for vortex lattice at low

temperature (a) and vortex liquid at high temperatures (b)

well. Therefore, we can say that the present simulation

results are statistically and thermodynamically correct.

Structure Factor

The correlation functions of vortices can be described

by a structure factor deﬁned by

S(q

, z) =

∞

−∞

2π

n(q)n(−q), (22.87)

where n(q) =



n(r)exp(−iq ·r)andn(r)isthevor-

ticity in the c direction (i. e., along the magnetic ﬁeld)

at position r. Two typical structure factors S(q

, z = 0)

for the in-plane correlation functions of vortices are de-

picted in Fig. 22.19. It becomes clear that the ﬁrst-order

Part E 22.5

1140 Part E Modeling and Simulation Methods

phase transition is a melting transition of the vortex lat-

tice of hexagonal symmetry into the isotropic vortex

liquid.

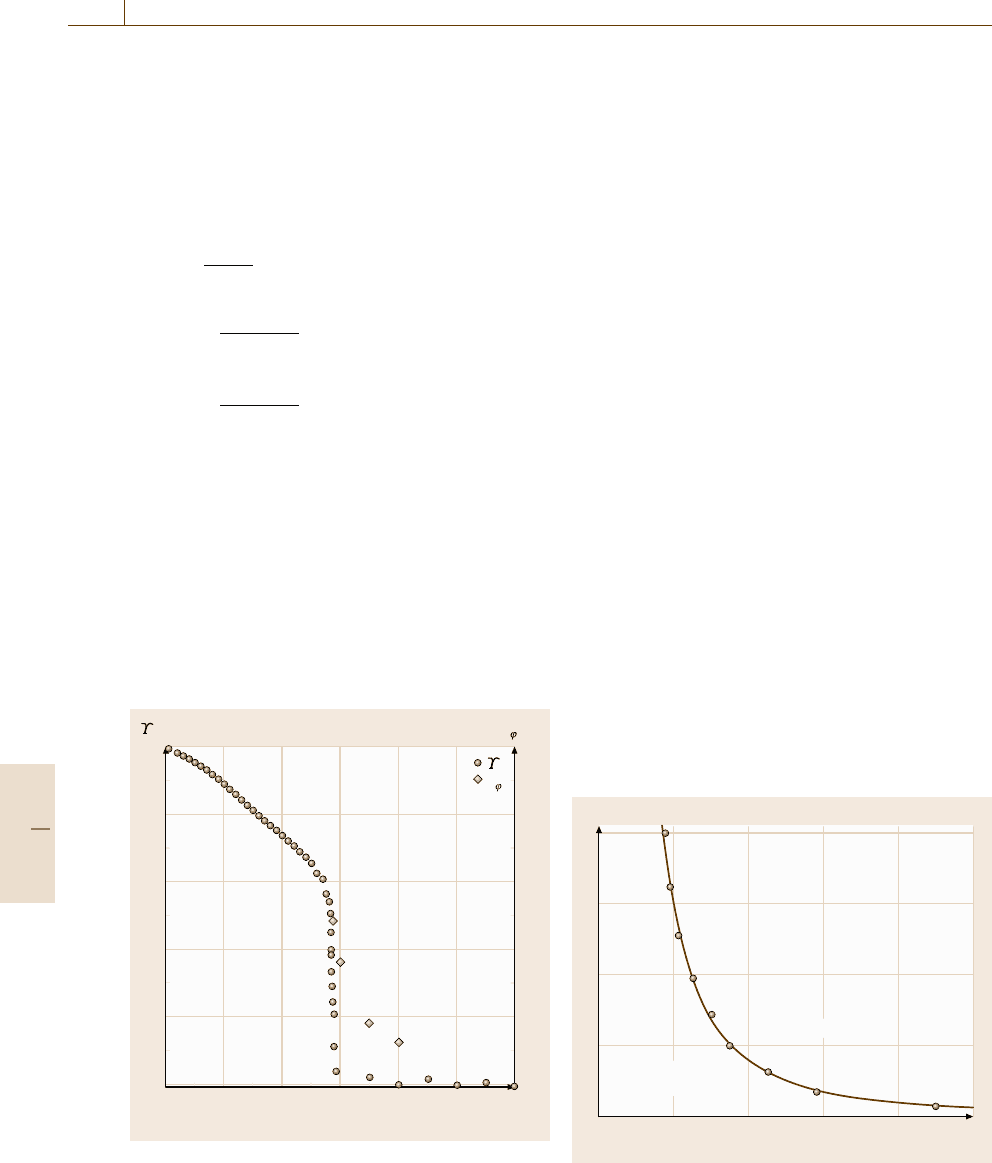
Helicity Modulus

The temperature dependence of the helicity modulus

along the c axis





i, j

cos(ϕ

−ϕ

)(



−





i, j

sin(ϕ

−ϕ

)(







i, j

sin(ϕ

−ϕ

)(



(22.88)

is presented in Fig. 22.20. It increases from zero to

a ﬁnite value Υ

) 0.6J/Γ

in a narrow tempera-

ture region around T

as the system is cooled down.

Therefore, the phase transition at T

is the normal to

superconducting phase transition. The sharp onset of Υ

is another indication of ﬁrst-order transition. The helic-

ity modulus in the ab plane remains zero even below

the transition temperature T

. Therefore, the system is

superconducting only along the c axis even below T

ξ (d)

T (J/k

)

0 0.2 0.4 0.80.6 1 1.2

0.8

0.6

0.4

0.2

(J/Γ

)

Fig. 22.20 Temperature dependence of the helicity modu-

lus and the correlation length

The correlation length of phase variables along

the c direction increases with cooling and reaches to

ϕ,c

 10d at the melting temperature. We notice that

our system size is L

=40 in units of d, and is therefore

sufﬁcient for simulating the true phase transition of the

vortex system of the given parameters. If a smaller sys-

tem of L

≤20 was taken, the system would behave as

if a long-range SC order had established at a tempera-

ture above the melting point, where the structure factor

evolves from that of the liquid into the lattice. Then one

would be led to two phase transitions, instead of the true

single one.

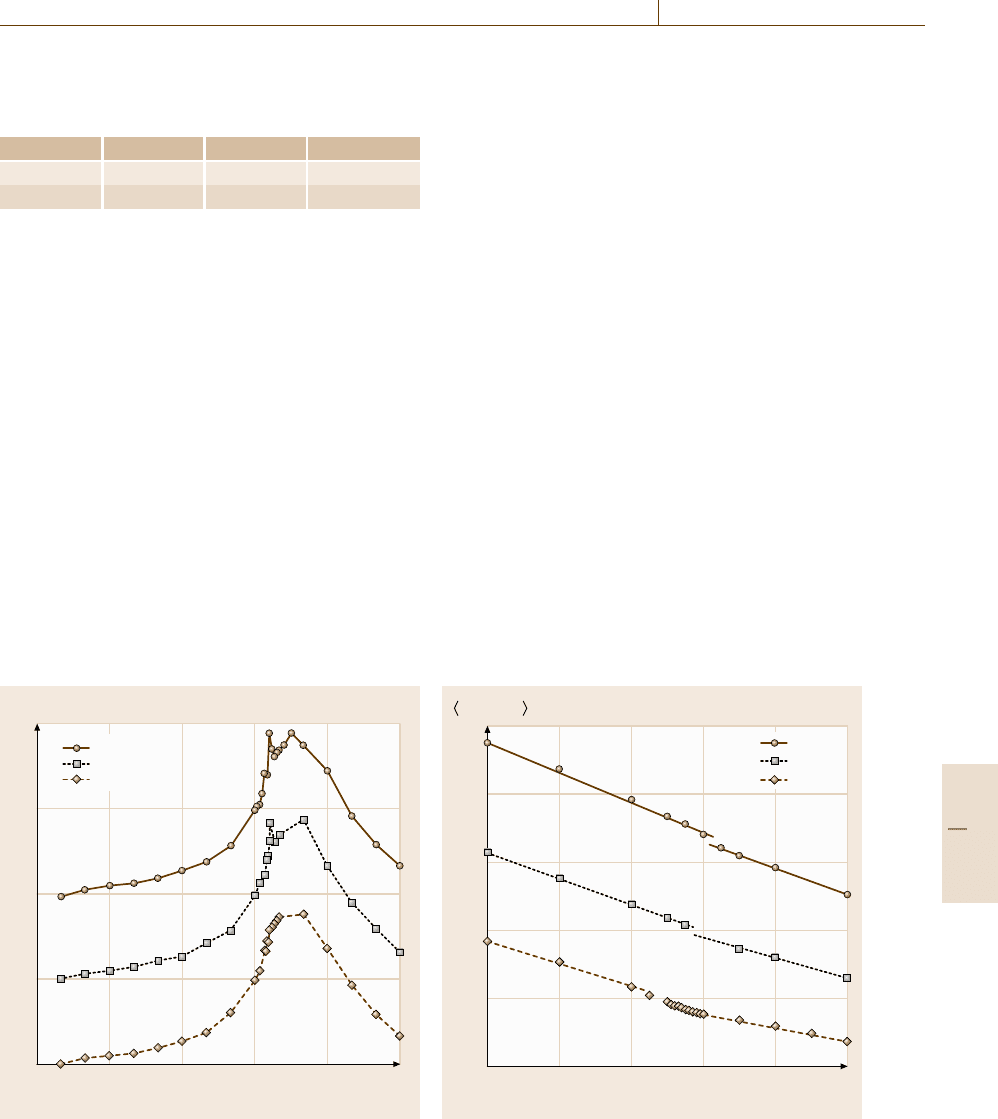
Phase Diagram

By tuning the magnetic ﬁeld, or equivalently the

anisotropy parameter, we have mapped out the B-T

phase diagram of the pancake vortices as shown in

Fig. 22.21. The decreasing melting temperature with in-

creasing magnetic ﬁeld is consistent with the Clausius–

Clapeyron relation

ΔB =−4πΔs/(dB

/dT ) , (22.89)

where Δs is the entropy jump of unit volume. Taking

into account the temperature dependence of the penetra-

tion depth, and thus that of the coupling strength in our

model, we can [22.142] compare our numerical results

with experiments. The agreement is quite satisfactory as

in Table 22.5.

The hump in the speciﬁc heat at T

 1.1J/k

is produced by a huge number of thermal excita-

tions of vortices in the form of closed loops. Even

local superconductivity order parameters cannot sur-

B (φ

/(γd)

)

T (J/k

)

0.2 0.4

Flux-line

lattice

Vortex liquid

0.80.6 10

Fig. 22.21 B-T phase diagram (after [22.142])

Part E 22.5

Monte Carlo Simulation 22.5 Superconductivity Vortex State 1141

Table 22.5 Comparison between our simulation results

and experimental observations for YBa

7−δ

. ΔS de-

notes the entropy jump per ﬂux line per length d

B =8Tesla T

[K] ΔS[k

] ΔB [G]

Simulation 81 0.55 0.19

Experiment 79 0.4 0.25

vive at any scale larger than the grid, which indicates

= T

. Therefore, treating thermal ﬂuctuations suc-

cessfully makes the mean-ﬁeld phase transition at H

a crossover.

22.5.3 Continuous Melting: B||ab Plane

When the external magnetic ﬁeld is applied parallel

to the CuO

layers of a high-T

superconductor, mag-

netic induction tends to penetrate the sample through

the positions where superconductivity concentration is

lower, namely the so-called block layers. Because of

the Josephson couplings, magnetic induction is still

quantized, the so-called interlayer Josephson vortices.

A Josephson vortex is of a large size along the ab

plane and squeezed in the c direction. The inter-vortex

repulsion in this geometry is highly anisotropic, and

meanwhile vortex lines feel strong layer pinning for mo-

tions along the c axis. In spite of much effort, which

C (k

)

T (J/k

)

0 0.3 0.6 1.20.9 1.5

2.5

1.5

0.5

γ = 8

γ = 9

γ = 10

Fig. 22.22 Temperature dependence of the speciﬁc heat for

several anisotropy parameters (after [22.147]). Data for

γ = 8 and 9 are shifted by constants

certainly makes the vortex physics very rich [22.148–

158], a uniﬁed picture for possible phases, phase tran-

sitions and phase diagrams is still not yet available.

We have performed extensive Monte Carlo simulations

of the frustrated XY model (22.82). The main pre-

diction from computer simulation [22.147, 159](see

also [22.160, 161]) is that there is a multicritical point

in the B-T phase. For magnetic ﬁelds above the criti-

cal value, there is an intermediate phase characterized

by intraplane (2-D) quasi-long-range crystalline order

of vortex lines in between the liquid and the 3-D lattice.

The model Hamiltonian is given in (22.82) with

a gauge A

= 0. We take Γ as the anisotropy constant

γ = 8 of the material YBa

7−δ

. The unit length of

our grid is then d for all the three directions. For the con-

venience of simulation, we choose f =1/32 and put the

system size as L

= 384, L

=200 and L

=20 with

=240 ﬂux lines in the system.

The Speciﬁc Heat

The speciﬁc heat is displayed in Fig. 22.22. There are

sharp spikes in the speciﬁc heat for γ =8and9atT



0.96J/k

, which indicates a ﬁrst-order melting. As the

total latent heats are very small, we plot in Fig. 22.23 the

expectation values for the cosine function of the gauge

invariant phase difference between neighboring layers,

which is proportional to the Josephson energy. There

T (J/k

)

0.9 0.92 0.94 0.980.96 1

0.18

0.16

0.14

0.12

0.1

0.08

γ = 8

γ = 9

γ = 10

cos φ

n,n+1

Fig. 22.23 Temperature dependence of the Josephson en-

ergy for several anisotropy parameters (after [22.147]).

Data for γ = 8and9areshiftedbyconstants

Part E 22.5

1142 Part E Modeling and Simulation Methods

a) b)

Fig. 22.24a,b Structure factors for Josephson vortices

S(k

, k

, y = 0) at T = 0.65J/k

(a) and T =0.70J/k

(b).Thepanels are for wave numbers within k

∈

[−25π/192d, 25π/192d] (horizontal)andk

∈[−2π/d,

2π/d] (vertical) (after [22.159])

are discontinuous jumps in this quantity for γ = 8and

9, corresponding to the spikes in the speciﬁc heat.

However, the ﬁrst-order melting transition is sup-

pressed when the anisotropy parameter is increased to

γ = 10, for which the spike in the speciﬁc heat and the

discontinuous jump in the Josephson energy disappear

as in Figs. 22.22 and 22.23. It is discussed in [22.147]

that there is a critical value of f γ above which the in-

tralayer repulsion force becomes signiﬁcantly stronger

than the interlayer one, such that the intralayer order

establishes ﬁrst upon cooling. For a more careful discus-

sion please refer to our original paper [22.159]. It is easy

to see that this critical magnetic ﬁeld should be above

S (k

, k

= π/d)/N

(π/192d)

0 5 10 15

γ=20

0.012

0.009

0.006

0.003

T = 0.96 J/k

T = 0.95 J/k

T = 0.92 J/k

T = 0.80 J/k

Fig. 22.25 k

proﬁles for the Bragg spots (after [22.159])

the following value when the anisotropy parameter γ is

given [22.153]

B =

√

3γ d

. (22.90)

To determine the value of the critical magnetic ﬁeld de-

mands a lot of simulation time, and has actually not yet

been done. Nevertheless, the above relation seems to

give a reasonable estimate for moderately anisotropic

superconductors such as YBa

7−δ

Structure Factor

The above scenario has been veriﬁed by checking the

vortex correlation function. In Fig. 22.24, we present

the structure factors for f = 1/32 and γ = 20. For

T = 0.65J/k

there is a 3-D long-range crystalline or-

der characterized by the δ-function Bragg peaks; for

T = 0.7J/k

the Bragg spots smear out signiﬁcantly in

the k

direction, corresponding to short-range correla-

tioninthec direction.

The k

proﬁle for the Bragg spot at (k

, k

) =

(±2 f π/d, ±π/d) is plotted in Fig. 22.25 for several

temperatures, from which we found that the real-space

correlation functions show power-law decay with ex-

ponents 1 ≤ η ≤ 2. The cusp singularity in the k

proﬁles is suppressed at T 0.96J/k

where η  1.97.

The suppression of the quasi long-range order upon

S (k

= π/d, k

= π/d)/N

T (J/k

)

0 0.6 0.80.40.2 1

γ=8

γ=20

1.2

0.8

0.6

0.4

0.2

Fig. 22.26 Temperature dependence of intensity of Bragg

peaks (after [22.159])

Part E 22.5

Monte Carlo Simulation 22.6 Effects of Randomness in Vortex States 1143

heating should be a Kosterlitz–Thouless (KT) transi-

tion [22.159].

The temperature dependence of the intensity of

Bragg peaks is displayed in Fig. 22.26 is such a fash-

ion that for quasi long-range orders the intensity should

be zero at the thermodynamics limit. As our system is

ﬁnite, small values of normalized intensity can be seen

for γ = 20 which increases very slowly with decreas-

ing temperature in the region 0.7J/k

≤ T ≤0.95J/k

Across T

0.65J/k

where the Bragg spots become

δ-function like, the intensity increases noticeably. The

onset of 3-D long-range order is revealed as a second-

order phase transition in the 3-D XY universality class.

In a sharp contrast, the intensity of Bragg peaks for

γ = 8 increases very sharply with decreasing tempera-

ture as soon as they become observable at the melting

point T

0.96J/k

asshowninFig.22.26.

Right now experimental veriﬁcations of the multi-

critical point and the KT phase are carried on by

several groups. For an estimate, we foresee the critical

ﬁeld at H ≈ 50 Tesla for YBa

7−δ

of γ = 8and

H ≈2 Tesla for Bi

CaCu

8+y

of γ = 150.

Since it is still not easy to simulate the vortex

states of low vortex density, namely small magnetic

ﬁeld, the total phase diagram is not available merely

by the computer simulation. Recently, using the density

functional theory, we [22.162] ﬁnd in certain magnetic

ﬁeld regimes below that given by (22.90) a smectic

phase [22.154, 155], characterized by long-range cor-

relation along the c axis and short-range correlations in

the ab plane, that may exist in between the lattice and

liquid phases. The melting is then two-step: ﬁrst-order

lattice-smectic and second-order smectic-liquid transi-

tions.

22.6 Effects of Randomness in Vortex States

It is highly nontrivial whether the vortex lattice can sur-

vive when defects are introduced into the system. The

answer depends on the dimensions of the space and the

type of defects. In the elastic theory, the Hamiltonian of

vortex states including point-like randomness is given

H =

[∇u(x)]

x −

V(x)ρ(x)d

x , (22.91)

where the ﬁrst term stands for the simpliﬁed elastic

energy associated with deviations of ﬂux lines u(x)

from their equilibrium positions, and V(x)andρ(x)de-

note the random potential and the density of ﬂux lines,

respectively. Larkin [22.163,164] proposed an approxi-

mation to replace the second term by

V(x)ρ(x)d

x ≈

f (x)u(x)d

x , (22.92)

where f (x) is the Gaussian random force.

Then, the situation becomes similar to Imry–Ma’s

argument [22.165] for the random-ﬁeld model. Let us

compare the energy between a polycrystal containing

domains of the linear size ∼ L with the perfect vortex

lattice. The elastic energy loss and the pinning energy

gain are proportional to L

d−2

and L

d/2

, respectively.

Since the second term is larger than the ﬁrst one for

large enough L in d < 4, the vortex lattice is unstable

for inﬁnitesimal randomness in d < 4. This simple con-

sideration turns out to be insufﬁcient and the picture

of a Bragg glass is now established where the vortex

correlations become quasi long ranged [22.166–170].

22.6.1 Point-Like Defects

Here our Monte Carlo simulations based on the frus-

trated XY model are reviewed. Modeling of point-like

randomness is not unique in the frustrated XY model.

One possible way is to introduce a Gaussian random

variable ε

with ε

=0andε

=1asJ

= J +

pε

[22.171]. In this deﬁnition, randomness is included

in every bond, and the strength of randomness p can

be treated as a continuous thermodynamic variable like

temperature. On the other hand, the pinning force of

these randomly-modulated interactions to vortices may

be very weak.

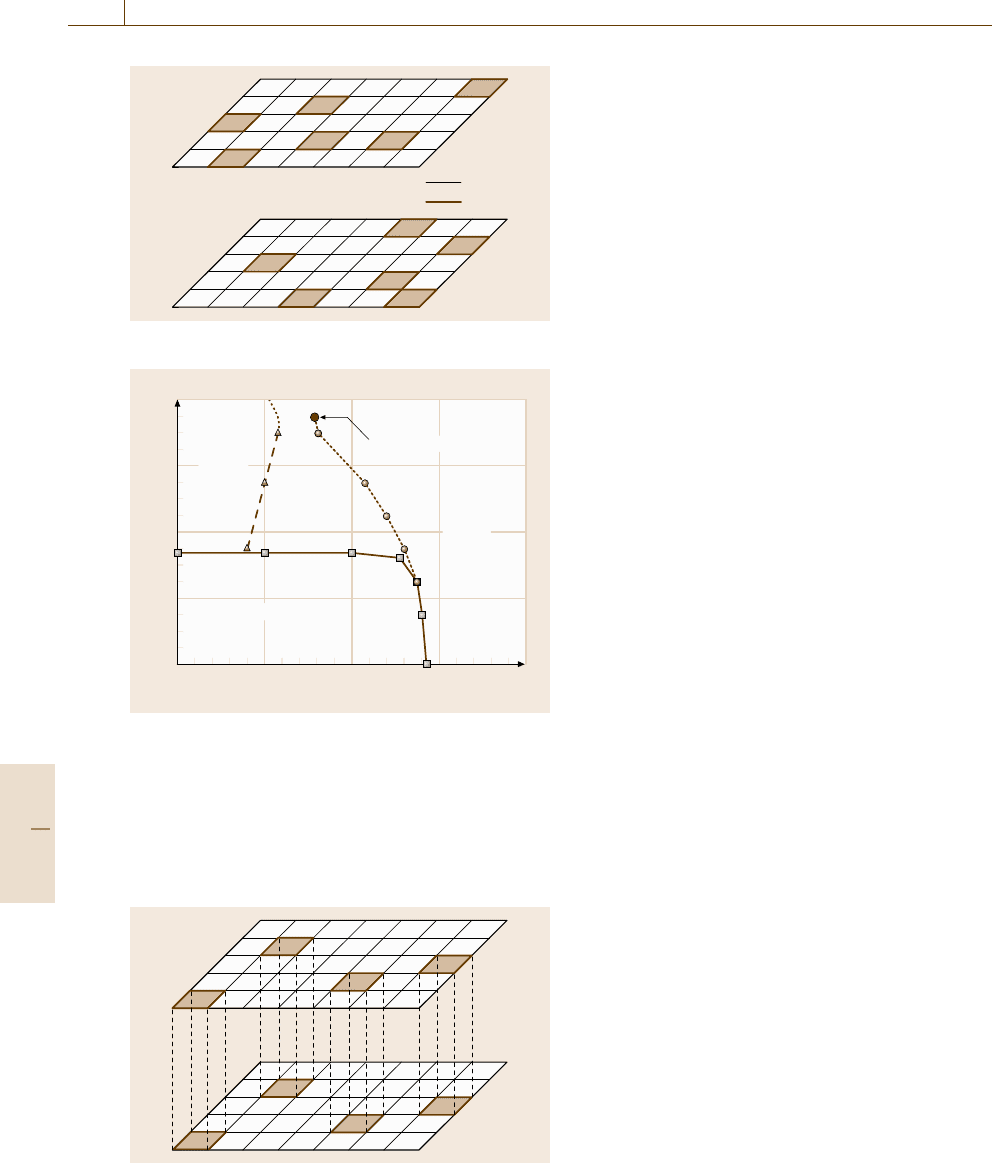
We introduced [22.172] point defects as the pla-

quettes which consist of four weaker couplings and are

randomly distributed in the xy plane with probability p.

Couplings are given by J

=(1−ε)J (0 <ε<1) on the

point defects, and J

= J elsewhere. This formulation

of point defects is schematically drawn in Fig. 22.27.As

will be seen later, most phases observed experimentally

in vortex states are reproduced by this modeling.

Bragg Glass

Monte Carlo simulations are performed for f = 1/25,

Γ = 20, p = 0.003, and various pinning strengths ε

with the system size L

= L

= 50 and L

= 40. The

same algorithm for the clean system discussed previ-

Part E 22.6

1144 Part E Modeling and Simulation Methods

(J–ε)J

Fig. 22.27 Modeling for point-like defects (after [22.172])

T (J/k

)

0.06 0.07 0.08 0.09

Vortex

glass

Vortex

slush

Vortex

liquid

Bragg glass

Critical point

0.1

0.16

0.12

0.08

0.04

Fig. 22.28 -T phase diagram of vortex states with the

point defects (after [22.172,173])

ously is adopted. The ε-T phase diagram is displayed in

Fig. 22.28.

The ﬁrst-order melting transition between the Bragg

glass (BrG) and vortex liquid (VL) phases is similar to

Fig. 22.29 Modeling for columnar defects (after [22.174])

that of the vortex lattice and VL phases in pure systems

as shown previously. As the strength of point-like de-

fects ε increases, the melting temperature T

decreases,

which shows a sharp contrast to the case with columnar

defects as will be discussed later.

The Bragg glass is destroyed when the pinning force

becomes large enough even though temperature is low,

and thus thermal ﬂuctuations are not signiﬁcant. This

randomness-induced melting was ﬁrst observed numer-

ically in the random-ﬁeld XY model [22.175]andthe

Lawrence–Doniach model [22.176]. The ﬁrst-order na-

ture of this phase transition is clariﬁed in more detail

by precise numerical studies on the phase boundary. We

showed [22.172] that the latent heat on the boundary

is one order smaller than that on the thermal melt-

ing line. It was also reported [22.171] that the latent

heat is vanishing within error bars while discontinuity

in other physical quantities remains observable on the

phase boundary.

Possible Vortex Glass

As a possible thermodynamic phase above the BrG, the

vortex glass (VG) phase [22.177, 178], where ﬂux lines

are tightly pinned by point-like defects, has been con-

sidered. In the early stage of numerical simulations,

the VG-VL boundary was detected by the jump of

the correlation time [22.179] or the deviation of ﬂux

lines [22.180]. In the frustrated XY model, the VG

phase is characterized by ﬁnite helicity modulus along

the c axis and vanishing Bragg peak [22.172]. Although

the absence of the VG was once argued [22.171], sta-

bility of the VG phase without the screening has now

been established [22.181–183]. It is noticed that the

stability of VG phase including the screening is still

unsettled [22.184,185].

Vortex Slush

There is a vortex slush phase [22.186–188] in the phase

diagram (Fig. 22.28). The vortex slush shares the same

symmetry of the liquid. The two phases are separated by

a ﬁrst-order transition line characterized by the jump of

the density of dislocations in the xy plane [22.189,190].

As in the gas–liquid case, this transition line is ter-

minated by a critical point as shown in Fig. 22.28.

It is interesting to notice that even above the critical

point, a step-like anomaly of the speciﬁc heat was re-

ported [22.173,191].

22.6.2 Columnar Defects

Columnar defects created by heavy-ion irradiation

along the c axis work as strong correlated pinning

Part E 22.6

Monte Carlo Simulation 22.6 Effects of Randomness in Vortex States 1145

Melting line

T (J/k

)

0.3 0.35 0.4

Vortex

liquid

Interstitial

liquid

Bose glass

Bragg–Bose glass

polycrystal

Vanishing

point of

Jump

of c

Matching field

0.45

0.04

0.3

0.02

0.01

Fig. 22.30 p-T phase diagram for vortex states with the

columnar defects (after [22.174])

centers to the vortex lines and produce new vortex

states such as the Bose glass (BG) [22.192–194]. We

introduced [22.174] columnar defects as the corre-

lated plaquettes surrounded by four weak couplings

=(1 −ε)J with probability p in the xy plane and

= J elsewhere, as shown in Fig. 22.29. Since the

density of defects p plays an essential role in vortex

states with columnar defects, we tune the parameter p

while ﬁxing ε. Parameters for simulation are Γ =5,

f =1/25, ε =0.1, and L

= L

=50 and L

=80.

Bose Glass

The p-T phase diagram obtained by Monte Carlo simu-

lations is displayed in Fig. 22.30. When the number

of columnar defects exceeds that of ﬂux lines, the

BG is stable at low temperatures. Here, we concen-

trate on small densities of columnar defects, ranging

from the pure system with p = 0 to the matching case

p = f =1/25.

In order to evaluate the BG phase boundary, the

tilt modulus c

is evaluated. The Hamiltonian un-

der the transverse ﬁeld H

⊥

is given by H



= H −

⊥

with the transverse magnetization m

⊥

,andc

is given [22.195]by

∼



∂m

⊥

/∂H

⊥



−1

∼ N

ﬂux



⊥



⊥

, (22.93)

aside from a coefﬁcient. The BG phase boundary is sig-

naled by a large jump of c

, which is expected to be

inﬁnite in the thermodynamic limit.

Interstitial Liquid

When the number of columnar defects is smaller than

that of ﬂux lines and the temperature is slightly higher

than the BG transition temperature, columnar defects

still trap some ﬂux lines, while other interstitial ﬂux

lines can move around. This state is known as the inter-

stitial liquid (IL) [22.196]. The helicity modulus along

the c axis Υ

takes a ﬁnite value as long as some of the

ﬂux lines are trapped by columnar defects. Therefore,

the vanishing of the helicity modulus Υ

corresponds to

the boundary of the IL region.

Possibility of Bragg–Bose Glass

Similar to the BrG phase of vortex state with point-

like defects, a Bragg–Bose glass (BBG) was proposed

in the presence of columnar defects [22.197], which

is characterized by quasi-long-range crystalline order

and diverging tilt modulus. However, stability of this

phase is not so trivial. At the ground state, ﬂux lines

are perfectly parallel to the magnetic ﬁeld and the

columnar defects. The system is then equivalent to the

two-dimensional vortex states with point defects, where

the absence of the BrG phase at T = 0 was shown nu-

merically [22.198]. Therefore, the BBG (if exists) may

be a kind of order caused by thermal ﬂuctuations.

For small enough p and low enough temperatures,

the structure factor shows sharp Bragg peaks, which is

consistent with the BBG. As the temperature increases,

the maximum value of the structure factor S

max

drops

just on the BG boundary with a narrow coexistence

region, and the δ-function peak of the speciﬁc heat is

observed. These facts indicate that the ﬁrst-order melt-

ing transition of the BBG phase occurs just on the BG

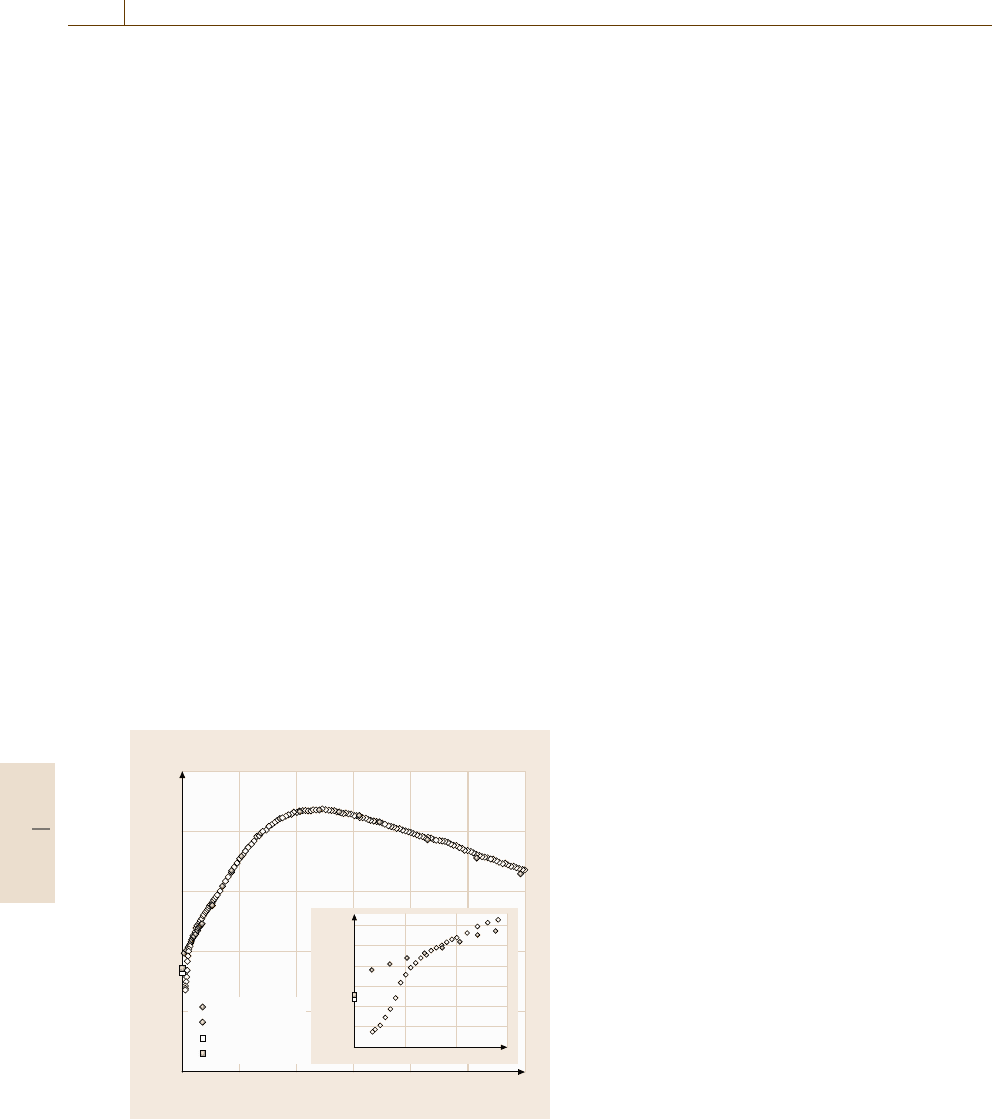
a) b)

Fig. 22.31a,b Density of vortices in the xy plane averaged

along the c axis in the (a) BBG and (b) IL phases with the

same conﬁguration of columnar defects (after [22.174]).

The defects are painted in yellow (no ﬂux lines)tored

(ﬁlled with ﬂux lines), and other regions are painted in vi-

olet (no ﬂux lines)tolight blue (largest probability of ﬂux

lines)

Part E 22.6

1146 Part E Modeling and Simulation Methods

boundary at low density of columnar defects. The BBG

at low temperature can be destroyed by introducing

more columnar defects, indicated by a sharp change

of S

max

. It is found that the BBG-BG phase bound-

ary is almost independent of temperature, as shown

in Fig. 22.30.

As can be seen in Fig. 22.31aforBBG phase, all

ﬂux lines are localized and form a triangular lattice.

There exist some unoccupied columnar defects (yellow

dots). The defects far from stable positions of vortex lat-

tice remain unoccupied in the BBG phase. On the other

hand, in Fig. 22.31bfortheIL phase, most defects are

occupied unless other occupied ones are very close by

chance. Owing to such selected pinning of ﬂux lines in

the BBG, introduction of more columnar defects to cer-

tain density enhances energy gain by trapping more ﬂux

lines using defects close to stable positions of vortex

lattice, without destroying the lattice structure. This re-

sults in increase of the melting temperature. When p

becomes large enough, the vortex lattice will be de-

stroyed by the overwhelming pinning energy and the

BG phase becomes stable.

The above description is consistent with the simu-

lations based on the boson model [22.199], while the

simulations based on the density-functional theory re-

sults in a different picture characterized by the two-step

melting transition with the “BrG phase” surrounded by

the BG phase [22.200,201].

22.7 Quantum Critical Phenomena

Here we show several examples investigated by quan-

tum Monte Carlo simulations, which is helpful for

understanding the methodology.

22.7.1 Quantum Spin Chain

It was naively expected that low-energy excitations

of spin systems are described by spin waves. In the

spin-wave approximation, low-energy excitations are

always gapless, regardless of the length of spins.

However, Haldane predicted that in one dimension

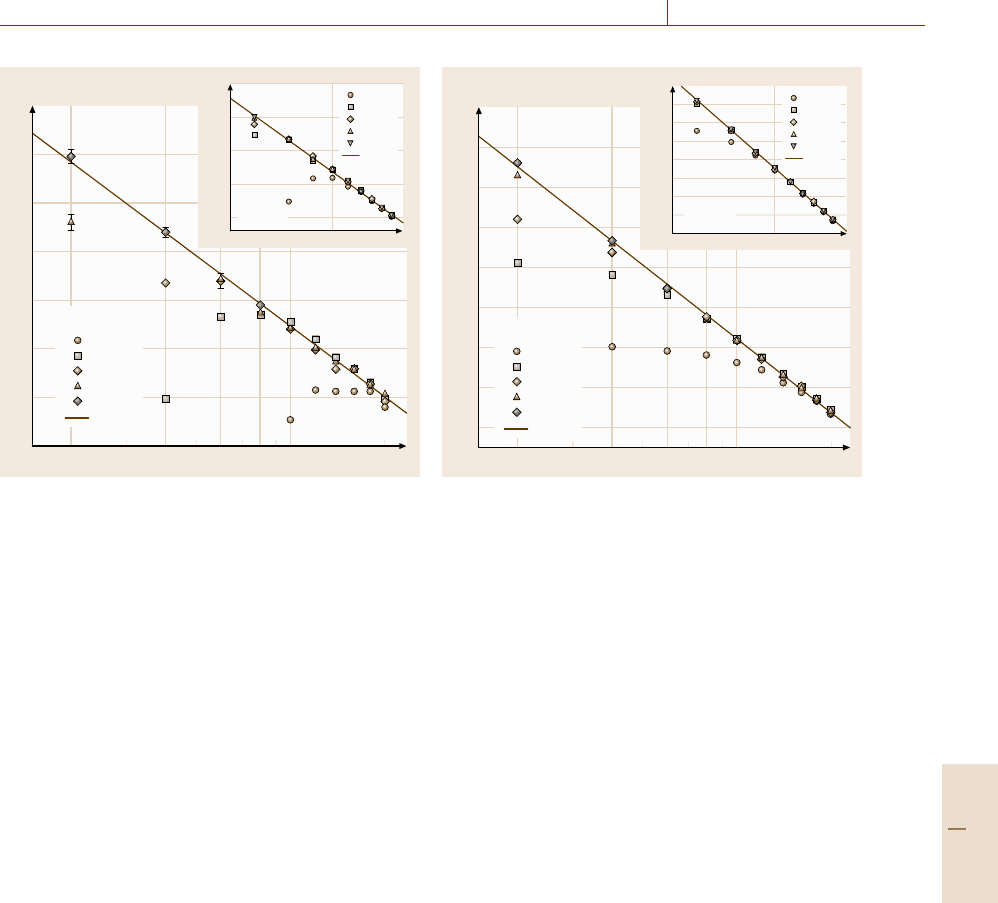
0.005

0.004

0.003

0.002

0.001

Experiment

Monte Carlo

v = 2.46 (T=0)

v = 2.39 (T=0)

0.0024

0.0022

0.0020

0.0018

0.0016

0.0014

0.0012

0 50 100 150 200 250 300

Temperature (K)

χ (q=0;T ) (emu/mol)

51015

Fig. 22.32 Uniform susceptibility χ(q =0;T) obtained by

the quantum Monte Carlo simulation and experiments

(after [22.202])

low-energy excitations have a ﬁnite gap for integer

spin systems [22.203, 204]. This prediction was later

conﬁrmed by numerical simulations [22.205–207]and

experiments [22.208–211]. Further, Afﬂeck extended

Haldane’s argument to bond-alternating systems and

predicted that integer spin chains can have a gap-

less excitation with special values of the alternating

strength [22.212–214].

Some compounds that can be regarded as spin

S = 1 bond-alternating Heisenberg chains have been

found experimentally [22.215–219]. Among them,

the susceptibility of [Ni(333-tet)(μ-N

)](ClO

)

(333-

tet denotes tetraamine N,N



-bis(3-aminopropyl)-1,3-

propanediamine) shows a gapless behavior [22.220].

In order to conﬁrm whether this compound can really

be described by an S = 1 bond-alternating Heisen-

berg chain, numerical simulations have been per-

formed [22.202]. Since the model is a nonfrustrated

Heisenberg model, quantum Monte Carlo simulations

can be performed without negative-sign problems as

explained in Sect. 22.3.8. Hence, the loop algorithm

(Sect. 22.3.3) is applied to this system. The comparison

between the result of the quantum Monte Carlo simula-

tion and the experimental result is shown in Fig. 22.32.

In the wide temperature range, numerical and experi-

mental data agree well. This indicates that the magnetic

properties of this compound are well described by the

S =1 bond-alternating Heisenberg chain at the gapless

point. Only in the very low temperature regime, data

show a little difference which may be due to single-ion

anisotropy of this compound.

It was also predicted that at the gapless point low-

energy properties are described by the level k = 1

Part E 22.7

Monte Carlo Simulation 22.7 Quantum Critical Phenomena 1147

0.55

0.5

0.45

0.4

0.35

0.02

S1/2AH

0.04 0.06 0.08 0.1 T

[χ (q=π;T ) T]

0.1 0.2

L =32

L =64

L =96

L =192

L =400

fit

S1BA

L =32

L =64

L =96

L =192

L =320

fit

Fig. 22.33 Staggered susceptibility χ(q = π;T) obtained

by the quantum Monte Carlo simulation (after [22.202]).

S1BA and S1/2AH denote the S = 1 bond-alternating

Heisenberg chain at the gapless point and the S = 1/2

Heisenberg chain, respectively

SU(2) Wess–Zumino–Witten (WZW) model [22.212–

214], which is the same universality class as the S =1/2

Heisenberg chain. The critical properties at the gapless

point are also investigated by the quantum Monte Carlo

simulation [22.202]. It is known that in the WZW uni-

versality class staggered susceptibility χ(q =π;T )and

staggered structure factor S(q = π;T) diverge toward

the critical point [22.221,222]as

χ(q = π;T) ∝ T

−1

[ln(T

/T)]

1/2

, (22.94)

S(q = π;T) ∝[ln(T

/T)]

3/2

. (22.95)

Figures 22.33 and 22.34 show the numerical results for

χ(q =π;T )andS(q =π;T ). The scaling properties of

(22.94)and(22.95) are clearly observed.

In this way, quantum Monte Carlo simulations

are powerful to investigate properties of spin systems.

There are many other examples to show the power of

quantum Monte Carlo simulations [22.66].

22.7.2 Mott Transition

It is widely believed that for the high-T

supercon-

ductivity strong correlations near the Mott transition

play an important role. In the insulating phase, there is

a magnetic order and the spin and charge excitations are

3.8

3.6

3.4

3.2

2.8

2.6

2.4

1.3

1.25

1.15

1.1

1.05

0.95

0.9

0.02

L =32

L =64

L =96

L =192

L =400

fit

S1BA

S1/2AH

0.04 0.06 0.08 0.1 T

[S (q=π;T ) T]

2/3

0.1 0.2

L=32

L=64

L=96

L=192

L=320

fit

Fig. 22.34 Staggered structure factor S(q =π;T) obtained

by the quantum Monte Carlo simulation (after [22.202])

separated. On the other hand, in the metallic phase, it

is expected that spin and charge degrees of freedom are

strongly coupled, and the excitations may be described

by fermionic quasi particles at least in the low electron-

density regime. The difﬁculty of approaching the Mott

transition comes from the complexity of the transit of

these two-types of states. Since the Hubbard and t-J

models are known to be insulators at half ﬁlling and be-

come metallic by doping, characteristic features of Mott

transitions would be described by these models.

Properties near the metal-insulator transition have

been numerically investigated by quantum Monte Carlo

simulations. For the two-dimensional Hubbard model,

Furukawa and Imada applied the auxiliary-ﬁeld quan-

tum Monte Carlo method (Sect. 22.3.6) and found

nontrivial power-law behaviors toward the Mott transi-

tion point [22.223]: The compressibility κ and the spin

structure factor S(Q) diverge as

κ ∝δ

−1

, (22.96)

S(Q) ∝δ

−1

, (22.97)

where δ denotes the doping concentration. Equation

(22.97) suggests that the antiferromagnetic correlation

length ξ

diverges as

∝δ

−1/2

, (22.98)

assuming that the spin–spin correlations behave as

S

∝e

iQr

· e

−r/ξ

. These features are observed by

Part E 22.7