Appel W. Mathematics for Physics and Physicists

Подождите немного. Документ загружается.

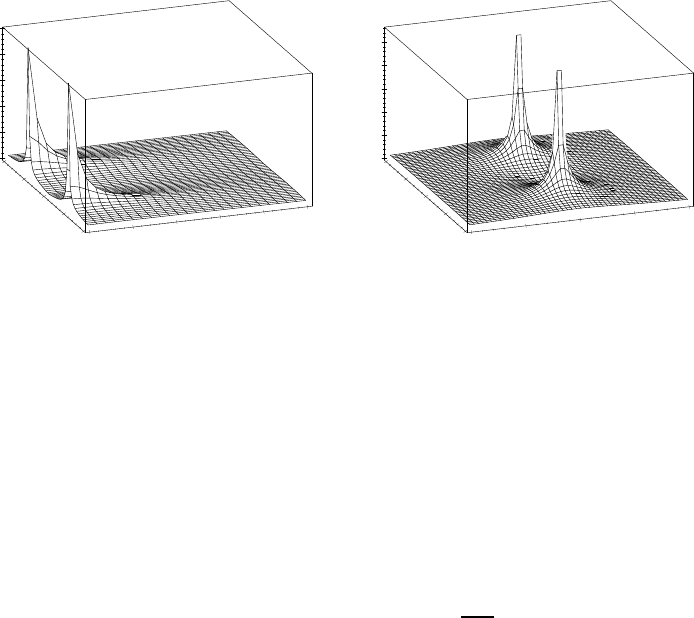
Inversion 337

−1

−2

−1

Fig. 12.2 — Representation of the Laplace transform of the function x 7→ cos x. The z-

axis is the modulus of the function p 7→ p/(p

+ 1). This function may be

analytically continued to the complex plane withou t i and −i.

which s hows that, for ﬁxed x, the function ν 7→ F (x + 2iπν) is the Fourier

transform of H (t) f (t) e

−x t

. The Fourier inversion formula leads, wh en t is a

point where H (t) f (t) is continuous, to

H (t) e

−x t

f (t) =

+∞

−∞

F (x + 2iπν) e

2iπνt

dν

H (t) f (t) =

+∞

−∞

F (x + 2iπν) e

x t

2iπνt

dν =

2iπ

F (p) e

dp,

where we have denoted p = x + 2iπν and D

is the ver tical line

def

= {x + iω ; ω ∈ R} (Bromwich contour).

Remark 12.15 Note that the resulting formula gives the original as a function of the image,

for an arbitrary x > α.

The result of the integral is indeed independent of the integration contour chosen since, F

being analytic on the convergence h al f-plane, Cauchy’s theorem guarantees that the difference

between the integral on D

and on D

′

vanishes (using the fact that lim

ω→±∞

F (p) = 0, as

given by Proposition 12.14).

The integration is performed by cutting the line D

and by closing it, on the left, by a

circle of arbitrarily large radius, cont aining all the singularitie s of F . (Sometimes it is necessary

to use a more subtle contour; see, for instance [31, § 7.5].)

338 The Laplace transform

THEOREM 12.16 (Inversion of the Laplace transform) Let f be an original

and F its Laplace transform. Let α be the convergence abcissa. For any point where f

is continuous, we have

f (t) =

2iπ

+i∞

−i∞

F (p) e

dp for any x

> α.

Remark 12.17 If we start with an arbitrary function, this formula leads us back to H (t) f (t).

Can you show this using the fact that F is analytic in the half-plane x > a and decays on

vertical lines?

12.3

Elementary properties and examples

of Laplace transforms

Just as in the case of the Fourier transform, there are relations linking

the Laplace transform and operations like translation, convolution, differenti-

ation, and integration.

12.3.a Translation

There is a translation theorem similar to what holds for the Fourier transform,

but which requires some care here: since we are looking at the unilateral

Laplace transform, we will write explicitly the function H (t) f (t) instead of

f (t). The, wr iting p = x + iω as usual and denoting by α the convergence

abcissa for f , we have

if H (t) f (t) ⊐ F (p) for α < x,

then H (t) f (t) e

−aτ

⊐ F (p + a) for α −Re(a) < x.

Similarly

if H(t) f (t) ⊐ F (p) for α < x,

then H (t −τ) f (t −τ) ⊐ F (p) e

−τ p

for α −τ < x.

Be careful to consider H (t −τ) f (t −τ), that is, the function which vanishes

for t < τ. T here is, a priori, no relation between the Laplace transform of the

function H (t) f (t −τ) and that of H (t) f (t).

Elementary properties and examples of Laplace transforms 339

12.3.b Convolution

For two causal functions f and g, we d eﬁne the convolution product, if it exists,

in t he same manner as was done in 7.64 on page 211, and the deﬁnition

simpliﬁes here to

f ∗ g(t) =

+∞

f (s) g(t − s) ds =

f (s) g(t − s) ds.

In particular, the quantity f ∗ g(t) depends only on the values of f and g

between the times 0 and t. This convolution, although formally identical to

what occurred with Fourier transforms, still has a causal aspect. To remember

this causality, we sometimes write explicitly H f ∗ H g instead of f ∗ g.

As for the Fourier transform, there is a convolution theorem, the proof of

which is left as an exercise:

THEOREM 12.18 Let f and g be originals with convergence abcissas respectively

equal to α and α

′

If f (t) ⊐ F (p) for α < x,

and g(t) ⊐ G(p) for α

′

< x,

then [H f ∗ H g](t) ⊐ F (p) G(p) for max(α, α

′

) < x.

Similarly, we have:

if f (t) ⊐ F (p) for α < x,

and g(t) ⊐ G(p) for α

′

< x,

then f (t) g(t) ⊐

2iπ

+i∞

−i∞

F (s) G(p − s) ds for



α < x

α + α

′

< x

12.3.c D ifferentiation and integration

Let us now try to ﬁnd the Laplace transform of th e derivative of a function

in the sense of functions. Take therefore an original function f and denote by F

its Laplace transform:

H (t) f (t) ⊐ F (p).

We ass ume that f is differentiable in the sense of functions; then we have

H (t) f

′

(t) ⊐

+∞

′

(t) e

−p t

dt =

+∞

p f (t) e

−p t

dt +

f (t) e

−p t

+∞

One shows (see Exercise 12.6) that lim

t→+∞

f (t) e

−p t

= 0 s o that the boundary

term is equal to −f (0) or, more precisely, to − lim

t→0

f (t), which is also denoted

−f (0

340 The Laplace transform

THEOREM 12.19 (Laplace transform and differentiation) Let f be an original

which is continuous, differentiable, and such that its derivative is also an original.

Then, if f (t) ⊐ F (p), the Laplac e transform of the derivative of f in the sense of

functions is given by

H (t)

f (t) ⊐ p F (p) − f (0

Similarly, if f can be differentiated n times and if its n-th derivative if an original,

we have

H (t)

f (t) ⊐ p

F (p) − p

n−1

f (0

) − p

n−2

′

) −···− f

(n−1)

Example 12.20 Let f (t) = 1 + e

−t

, which is an original with convergence abscissa α = 0, and

with Laplace transform equal to

1 + e

−t

⊐

p + 1

The function f is differentiable, with derivative g : t 7→ −e

−t

, which is an original with

convergence abscissa α

′

= −1. Then we have

p F (p) − f (0

) = 1 +

p + 1

−2 = −

p + 1

= G(p).

It is very important to remark that the derivative is considered in the sense

of functions and the derivative at 0 must not involve a Dirac distribution δ.

This is why the term H (t) was explicitly included outside the derivative sign.

We will see later what ha ppens if th e derivative is considered in the sense of

distributions.

There is also a converse result:

THEOREM 12.21 Let f (t) be an original with convergence abscissa α. Th en t

f (t)

is an original with the same convergence abscissa. If f (t) ⊐ F (p), then we have

(−t)

f (t) ⊐

F (p).

Similarly, there is the following integration result:

THEOREM 12.22 (Laplace transform and integration) Let f be an original,

with convergence abscissa equal to α.

If f (t) ⊐ F (p) for α < x,

then

f ( u) du ⊐

F (p)

for max(α, 0) < x.

Remark 12.23 This may be deduced f rom the convolution theorem since

f (u) du =



f ∗ H



(t).

Elementary properties and examples of Laplace transforms 341

Conversely, we have

THEOREM 12.24 Let f be an original.

If f (t) ⊐ F (p) for α < x,

then

f (t)

⊐

∞

F (z) dz for sup(α, 0) < x.

In this last relation, the path of integ rat ion joins the point p at inﬁnity, in

any direction in the half-plane of convergence. The result of the integration

is independent of the chosen path since F is holomorphic in th is half-plane.

It is still necessary that F (p) d ecay faster than 1/p at inﬁnity (this is true

for nice functions, but will not hold in general for the Laplace transform of

distributions).

12.3.d Examples

We have already shown that

H (t) ⊐

for 0 < Re(p).

Using the integration and translation theorems, we obtain

H (t) e

⊐

p − a

for Re(a) < Re(p),

H (t) e

n−1

(n −1)!

⊐

(p −a)

for Re(a) < Re(p).

Using a s imple fraction expansion, this provides a way to ﬁnd the original

for the Laplace transform of any rational function. Note (although we do not

prove it) that the formula remains valid if we take a value of n which is not

an integer; t hen the factor (n −1)! in the formula must b e replaced by Γ(n),

where Γ is the Euler function (see page 154).

In particular, we have:

H (t) e

iωt

⊐

p −iω

and H (t) e

−iωt

⊐

p + iω

, 0 < Re(p).

By linearity of the Laplace transform, we deduce that

H (t) cos(ωt) ⊐

+ ω

H (t) sin(ωt) ⊐

+ ω

, 0 < Re(p).

Similarly,

H (t) cosh(ωt) ⊐

−ω

H (t) sinh(ωt) ⊐

−ω

0 < Re(p).

342 The Laplace tr an sfor m

Remark 12.25 Be careful not to use the linearity to state someth ing like:

H (t) cosωt = Re



H (t) e

iωt



⊐ Re



p −iω



since taking the real part o f some expression is certainly not a C-linear operation.

12.4

Laplace transform of distributions

12.4.a Deﬁniti on

Since we are only discussing th e unilateral Laplace transform (or, what

amounts to the same thing, the Laplace transform of causal functions, zero

for t < 0), we will only generalize the Laplace transformation to distributions

with support bounded on the left, th at is, distributions in the space D

′

Recall that there is a convolution algebra structure deﬁned on this space —

namely, the product T ∗ S e xists for all T , S ∈ D

′

DEFINITION 12.26 Let T be a distribution with support bounded on the left.

If there exists an α ∈ R such th at, for all x > α, the distribution e

−x t

T (t) is

tempered, then the Laplace transform of T is deﬁned by the formula

T (t) ⊐

T (p)

def



T (t), e

−p t



T is a function. The lower bound of th e possible values of α is called the

convergence abscissa.

The fundamental example is the following:

PROPOSITION 12.27 (L a place transform of δ) The Dirac distribution being tem-

pered and with support bounded on the left, the Laplace transform of δ exists and is

equal to the constant function 1, with convergence abscissa α = −∞.

Proof. We have e

−αt

δ = δ for all α ∈ R, and δ is tempered. This proves that

α = −∞; furthermore, we have



δ(t),e

−pt



= e

−0

= 1.

12.4.b Pr operties

Let T be a distribution with support bounded on the left with Laplace trans-

form

T . Then the function

T is holomorphic at any point p ∈ C such that

Re(p) > α.

In addition, the following result may be shown:

Laplace transform of distributions 343

PROPOSITION 12.28 (Sufﬁcent condition for a Laplace transform) Any func-

tion F (p) which is holomorphic and bounded in modulus by a polynomial function

of p in a h alf-plane Re(p) > α is the Laplace transform of a distribution in D

′

The following important result then holds:

THEOREM 12.29 (Laplace transform and convolution) In the convolution a lge-

bra D

′

, the f undamental relation between convolution of distributions and product of

Laplace transforms holds:

T ∗ U ⊐

T (p) ·

U (p).

We may then state a differentiation th eorem for distributions:

THEOREM 12.30 (Laplace transform and differentiation) Let T ∈ D

′

be a

distribution with Lapla ce transform F (p), and let T

′

denote its derivative in the sense

of distributions. Then T

′

has a Lapla ce transform given by

′

(t) ⊐ p

T (p).

Remark 12.31 This result is compatible with Theorem 12.19: let f be a continuous function

on R (hence locally integ rable); the function t 7→ H (t) f (t) is a priori discontinuous at 0.

The derivative of H (t) f (t) is, in the sense of distributions, e qual to H (t)



′

(t)



+ f (0) δ(t).

Applying the differentiation theorem 12.19 for functions says that

H (t)



′

(t)



⊐ p F (p) − f (0).

Since in addition we have f (0 ) δ(t) ⊐ f (0), it follows that



H (t) f (t)



′

⊐ p F (p),

which is the statement in the differentiation theorem 12.30 in t h e sense of distributions.

Also, the following results remain valid for the La place transform of dis-

tributions:

THEOREM 12.32 Let T (t) ∈ D

′

have Laplace transform

T (p) with convergence

abscissa α. For τ, a ∈ R, we have

T (t −τ) ⊐

T (p) e

−pτ

for α < x,

T (t) e

−aτ

⊐

T (p + a) for α −Re(a) < x,

H ∗ T ⊐

T (p)

for max(α, 0) < x.

Remark 12.33 The last equation is the translation of the integration theorem, since H ∗ f is a

primitive of the distribution f .

344 The Laplace tr an sfor m

12.4.c Examples

Using the differentiation and translation theorems for the Laplace transform,

we ﬁnd:

THEOREM 12.34 The Dirac δ distribution δ and its derivatives have convergence

abscissas α = −∞ and

δ(t) ⊐ 1, δ

′

(t) ⊐ p,

(n)

(t) ⊐ p

, δ(t −τ) ⊐ e

−τ p

Putting

(t)

def

∞

n=0

δ(t −n),

it follows by linearity (and continuity) that

(t) ⊐

1 −e

−p

The convergence abscissa is α = 0.

It is possible to write symbolically X

(t) = H (t) X(t), although in

principle this is an ambiguous notation: indeed, H is not continuous at 0,

so H (t) δ(t) is not well deﬁned. By abuse of notation, we will consider that

H (t) δ(t) = δ(t).

 Exercise 12.2 Show that

cos(πt) X

(t) ⊐

1 −e

−p

and e

(t) ⊐

1 −e

1−p

12.4.d The z-transform

Let f b e a function of C

∞

class. We then have

H (t) f (t) X(t) =

∞

n=0

f (n) δ(t −n) ⊐

∞

n=0

f (n) e

−np

Putting z = e

−p

, the Laplace transform thus obtained may also be written

∞

n=0

f (n) z

DEFINITION 12.35 The z-transform of a function t 7→ f (t) is the power

series

z 7−→

∞

n=0

f (n) z

The z-transform of a function is used in signal theory, when it is necessary to

work with sampling (we do not know f (t) for all real t, but only for integer

values).

Laplace transform of distributions 345

12.4.e Relation between Laplace and Fourier trans forms

Consider a distribution f ∈ D

′

, with Laplace transform F (p) and conver-

gence abscissa α.

• If α > 0, th en f (t) is not a tempered distribution; it does not a priori

have a well-deﬁned Fourier transform.

• If α < 0, then f (t) has a Fourier transform; it is linked to the Laplace

transform by th e relation

f (ν) = F (2iπν).

• If α = 0, which is the most interesting case, it is possible to sh ow that

F (p) is a meromorphic function which has poles only on the imaginary axis

(remember that although F is not deﬁned on the left half-plane of the complex

plane, it may possibly have an analytic continuation to a larger domain). It

is not possible to consider directly the function F (2iπν), which is not locally

integrable and consequently does not correctly deﬁne a distribution — the

problems occur of course at the poles. Now expand F (p) as the sum of a

holomorphic function and rational functions with poles on the imaginary

axis:

F (p) = G(p) +

n∈I

(p −iω

)

with G h olomorphic for Re(p) ¾ 0. The integers m

are the orders of the

poles iω

. By means of the inverse La place transform, we d erive

f (t) = g(t) + H (t)

n∈I

iω

−1

−1)!

The Fourier transform of this function may be computed, as long as g has

a Fourier transform. Denoting ω

= 2iπν

, and using the “ﬁnite part”

distribution (see Exercises 8.6 on page 241 and 11.5 on page 324), we obtain:

f (ν) = eg(ν) +

n∈I

−1)

(ν −ν

)

2(−2iπ)

−1

−1)!

+ fp



2iπ(ν −ν

)



that is,

f (ν) = fp F (2iπν) +

n∈I

2(−2iπ)

−1

−1)!

−1)

(ν −ν

or also



2π



= fp F (iω) +

n∈I

(i)

−1

2(m

−1)!

−1)

(ω −ω

), (1 2.1 )

where fp is the ﬁnite part (for multiple poles), or the Cauchy principal value

(for simple poles).

346 The Laplace transform

 Exercise 12.3 Compute this way the Fourier transform of the Heaviside distribution and of

H (x) x

(see Exercise 11.5 on page 324).

12.5

Physical applications, the Cauchy problem

The La place transform may be used to solve problems linked to the evo-

lution of a system, when the initial condition is known. Suppose we have a

linear system of differential equations describing the evolution of a physical

system — for which the equations of motion are supposed to be known. We

are looking for the solutions t 7→ f (t), where the value of the function and

of a certain number of its derivatives at t = 0 are imposed. The Cauchy

problem is the data consisting of the differential equation (or the system of

differential equations) together with the initial conditions.

12.5.a Importance of the Cauchy problem

The physically essential notion — which is also philosophically fascinating —

of determinism is founded on the more mathematical concept of the Cauchy

problem. A system is deterministic if the knowledge, at a given time t = 0, of

the various parameters character izing th is system (typically, the posit ions and

velocities of the particles th at it is made of, in the case of a classical system),

determines theoretically the future (or past) evolution of the system without

ambiguity.

In other words, the differential equations that describe the dynamics of the

system must h ave a solution, for a given initial condition, and this s olution

must be unique.

A whole branch of mathematical physics deals with the proof of existence

and uniqueness theorems for s olutions of physical systems (often simpliﬁed).

But it should not be forgotten, taking as excuse that this kind of work is

highly technical (and t hus often a rid-looking), that this is the key to t he

precise notion of determinism.

Many quarrels between scientists have been based on this concept. It is to be seen already

in Newton’s equations and appears, in particular, later with Laplac e’s intuition of the deter-

ministic character of classical mechanics (the famous “I did not need this assumption”). These

disagreements continued into th e last century: Einstein, with h is “God does not play dice,” and

the ever-lively discussions between partisans of various interpretations of quantum mechanics.

For a theatrical approach to this subject, the reader is invited to go se e (or to read) the play

Arcadia by Tom Stoppard [88].