Appel W. Mathematics for Physics and Physicists
Подождите немного. Документ загружается.

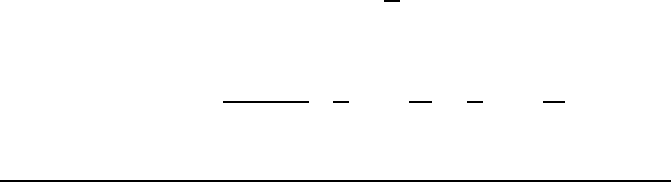
Justification of sinusoidal regime analysis 357
Indeed, a ssume that the input signal u and the output signal v are related
by a convolution equation v(t) = T (t) ∗u(t), where T is either a function or
a tempered distribution. If u is of th e form u(t) = e
2πiνt
, then v(t) = T (t) ∗
e
2πiνt
is infinitely differentiable (because the exponential is) and Theorem 8.24
yields
v(t) =
T (θ), e
2πiν(t−θ)
=
T (θ), e
−2πiνθ
e
2πiνt
,
which is indeed a complex exponential with the same frequency as the in-
put signal. The transfer function is, for the frequency ν, equal to Z(ν) =
T (θ), e
−2πiνθ
or, in other words, it is the value of the Fourier transform of
the impulse response T :
Z =
e
T .
The quantity Z(ν) may also be seen as the eigenvalue associated to the eigenvector
t 7→ e
2πiνt
of the convolution operator T .
The same reasoning applies for a real-valued exponential. If the input
signal is of t he type u(t) = e
pt
(where p is a real number), the output signal
can be wr itten
v(t) =
¬
T (θ), e
p(t−θ)
¶
=
T (θ), e
−pθ
e
pt
,
still according to Theorem 8.24. The “transfer function” p 7→
T (θ), e
−pθ
is then of course the Laplace transform of the impulse response T (see Chap-
ter 12).
Remark 13.2 (Case of a resonance) This discussi o n must be slightly nuanced: if Z(ν) is infi-
nite, which means the Fourier transform of the distribution T is not regular at ν, one cannot
conclude that the output signal w ill be sinusoidal.
A simple example is given by the equation of the driven harmonic oscillator:
v
′′
+ ω
2
v = e
2πiνt
= u(t).
This equation can be writ ten u = [δ
′′
+ ω
2
δ] ∗ v, the solution of which, in D
′
+
, is (see
Theorem 8.33 on p age 239)
v = T ∗ u with T (t) =
1
ω
H (t) sinωt.
The Fourier transform T (computed more easily by taking the Laplace transform evaluated at
2πiν, and applying the formula (12.1), page 345) is then
2
Z(ν) =
e
T (ν) = fp
1
ω
2
−4π
2
ν
2
+
1
4i
δ
ν −
ω
2π
−
1
4i
δ
ν +
ω
2π
.
For the values ν = ω/2π and ν = −ω/2π, there is divergence.
2
The transfer function Z(ν) is computed, ab initio, at the beginning of Chapter 15. This
will exp l ain why imaginary Dirac distributions appear, in relation with the c hoice of a solution
of the equation u = [δ
′′
+ ω
2
δ] ∗ v; for a solution in D
′
−
, the Dirac spikes would have the
opposite sign. A solut ion which does not involve any Dirac distribution exists also, but it does
not belong to any convolution algebra.
358 Physical applications of the Fourier transform
In fact, this reflects th e fact that the solutions of the differential equation are not sinu-
soidal; instead, they are o f the form
v(t) =
1
2iω
t e
i t
,
at the resonance frequency. More details are given in Chapter 15.
13.2
Fourier transform of ve c tor fields:
longitudinal and transverse fields
The Fourier transform can be extended to an n-dimensional space.
DEFINITION 13.3 The Fourier transform of a function x 7→ f ( x) with
variable x in R
n
is given by
e
f (νν
ν
ν
νν) =
ZZ
···
Z
R
n
f ( x) e
−2πiνν
ν
ν
νν·x
d
n
x.
All the properties of the Fourier transform extend easily to this case, mutatis
mutandis; for instance, some care is required for scaling:
F
f ( a x)
=
1
|a|
n
e
f
νν
ν
ν
νν
a
.
One may also define, coordinate-wise, the three-dimensional Fourier trans-
form of a vector field x 7→ A( x). Using this, it is possible to define transverse
fields and longitudinal fields.
Let x 7→ A( x) be a vector field, defined on R
3
and w ith values in R
3
.
This field may be decomposed into three components A = (A
x
, A
y
, A
z
), each
of which is a scalar field, that is, a function from R
3
to R. Denote by A
x
the
Fourier transform of A
x
, A
y
that of A
y
, and A
z
that of A
z
. Putting together
these three scalar fields into a vector field k 7→ AA
A
A
AA ( k), we obtain the Fourier
transform of the original vector field:
AA
A
A
AA ( k) =
ZZZ
R
3
A( x) e
−2πi k·x
d
3
x.
DEFINITION 13.4 Let A( x) be a vector field x ∈ R
n
with values in R
n
.
Denote by k 7→ AA
A
A
AA ( k) its Fourier transform. For any k ∈ R
n
, decompose the
vector AA
A
A
AA ( k) in two components:
• the first is parallel w ith k and is denoted AA
A
A
AA
//
(k); it is given by the
formula
AA
A
A
AA
//
(k)
def
=
AA
A
A
AA ( k) · k
kkk
2
k ;

Heisenberg uncertainty relations 359
• the second is orthogonal to k and is denoted AA
A
A
AA
⊥
(k); it is given by
AA
A
A
AA
⊥
(k)
def
= AA
A
A
AA ( k) −AA
A
A
AA
//
(k).
The longitudinal component of the field A is given by the inverse Fourier
transform of the field k 7→AA
A
A
AA
//
(k):
A
//
( x)
def
= F
−1
k
AA
A
A
AA ( k) · k
|k|
2
.
Its transverse component is then
A
⊥
( x)
def
= F
−1
AA
A
A
AA
⊥
(k)
= A( x) − A
//
( x).
DEFINITION 13.5 A vector field is transverse if its longitudinal component
is zero, and is longitudinal if its transverse component is zero.
From the differentiation theorem, we can der ive the well-known result:
PROPOSITION 13.6 A transverse field satisfies the relation
∇ · A( x) = 0 at any x ∈ R
3
,
that is, its divergence is identically zero.
It is important to notice that the property of “being transverse” or “being
longitudinal” is by no means a local property of the vector field (it is not suf-
ficient to know the value of the field at a given point, or in the neighborhood
of a point), but a property that d epends on the value of the field at all points
in space (see Exercise 13.1 on page 375).
Remark 13.7 A monochromatic field, with wave vector k, is transverse if it is perpendicular
to k at any k; it is easy to have an intuitive idea of this. A general transverse field i s then a
sum of monochromatic transverse fields.
13.3
Heisenberg unce rtainty relations
In nonrelativistic quantum mechanics, the state of a spinless particle is
represented by a complex-valued function ψ which is square integrable and
of C
2
class. More precisely, for any t ∈ R, the function ψ(·, t) : r 7→ ψ( r, t)
is in L
2
(R
3
). This function represents the probability amplitude of the particle
at the given point, that is, the probability density a ssociated wit h the presence

360 Physical applications of the Fourier transform
of the particle at point r in space and at time t is |ψ( r, t)|
2
. T he function
ψ(·, t) is normalized (in the sense of the L
2
norm) to have norm 1:
for any t ∈ R,
ψ(·, t)
2
2
=
ZZZ
R
3
ψ( r, t)
2
d
3
r = 1.
DEFINITION 13.8 The wave function in position representation is the
square-integrable function thus defined. The vector space H of the wave
functions, with the usual scalar product, is a Hilbert space.
3
DEFINITION 13.9 The position operator along the O x-axis is denoted X ,
and similarly Y and Z are the position operators along the O y- and O z-
axes, where R
def
= (X , Y , Z). The operator X is defined by its action on wave
functions given by
X ψ = ϕ with ϕ(·, t) : r 7−→ x ψ( r, t),
where we write generically r = (x, y, z).
Be careful not to make the (class ical!) mistake of stating tha t ψ and X ψ
are proportional, with proportionality constant equal to x!
It is customary to denote
ψ(t)
the function ψ(·, t), considered as a vector
in the vector space of wave functions.
4
The scalar product of two vectors is
then given by
ψ(t)
ϕ(t)
def
=
ZZZ
ψ( r, t) ϕ( r, t) d
3
r,
(recall the chosen convention for the hermitian product in quantum mechan-
ics: it is linear on the right and semilinear on the left), and the position operator
acts as follows:
X
ψ(t)
=
ϕ(t)
with ϕ( r, t) = x ψ( r, t).
With each vector |ψ〉 is associated a linear form on the space H by means
of the scalar product, which is denoted 〈ψ| ∈ H
∗
. Thus, the notation 〈ϕ|ψ〉
can be interpreted in two (equivalent) ways: as the scalar product of ϕ with ψ
(in the sense of the product in L
2
), or as the action of the linear form 〈ψ| on
the vector |ϕ〉 (see Chapter 14 for a thorough discussion of this notation).
3
Only “physical” wave functions belong to this space. Some useful fu nct ions, such as
monochromatic waves r 7→ e
i k·r
do not; they are the so-called “generalized kets,” whi ch are
discussed in Chapter 14, in particular, in Section 14.2.d.
4
In fact
ψ(t)
is a vector in an abstract Hilbert space H . However, there exists an isometry
between H and H = L
2
(R
3
), that is, the vector
ψ(t)
can be represented by the function
r 7→ ψ( r, t): this is the position representation. B u t, si nce the Fourier transform is itself
an isometry of L
2
(R
3
) into itself, the vector
ψ(t)
may also be represented by the f u nction
p 7→
e
ψ( p, t), which is the Fourier transform of the previous function. This is called the
momentum representation of the wave vector.

Heisenberg uncertainty relations 361
DEFINITION 13.10 The average position on the x-axis of a particle charac-
terized by the wave function ψ( r, t) is the quantity
〈x〉(t)
def
=
ψ(t)
X ψ(t)
=
ψ(t)
X
ψ(t)
=
ZZZ
x
ψ( r, t)
2
d
3
r.
In addition, to every wave function ψ( r, t) is associated its Fourier trans-
form with respect to the space variable r, namely,
Ψ( p, t)
def
=
ZZZ
ψ( r, t) e
−i p·r/ }h
d
3
r
p
(2π }h)
3
.
This function represents the probab ility amplitude for finding the particle
with momentum p. Of course, this function is also normalized to have norm
1, since for every t ∈ R we have
Ψ(t)
Ψ(t)
=
ZZZ
Ψ( p, t)
2
d
3
p =
ZZZ
ψ( r, t)
2
d
3
r = 1
by the Parseval-Plancherel identit y.
DEFINITION 13.11 The wave function in momentum representation is the
Fourier transform Ψ( p, t) of the wave function ψ( r, t) of a particle.
DEFINITION 13.12 The momentum operator P
def
= −i }h∇ = (P
x
, P
y
, P
z
) is
the linear operator a cting on differentiable functions by
P
x
ψ = ϕ
or P
x
|ψ〉 = |ϕ〉
with ϕ(·, t) : r 7−→ −i }h
∂ψ
∂ x
( r, t).
The average momentum along the x-direction of a particle character ized by
the wave function ψ( r, t) is
〈p
x
〉(t)
def
=
ψ(t)
P
x
ψ(t)
= −i }h
ZZZ
ψ( r, t)
∂ψ
∂ x
( r, t) d
3
r.
From the known properties of the Fourier transform, we s ee that it is
possible to extend the definition of the operator P
x
on wave f unctions “in
momentum representation” by
P
x
Ψ
def
= F
P
x
F
−1
[Ψ]
,
which gives explicitly, by the differentiation th eorem,
P
x
Ψ = Φ with Φ(·, t) : p 7−→ p
x
Ψ( p, t).

362 Physical applications of the Fourier transform
DEFINITION 13.13 The position uncertainty of a particle characterized by
the wave function ψ( r, t) is the quantity ∆x d efined by the following equiva-
lent e xpressions:
(∆x)
2
def
=
D
x −〈x〉
2
E
=
x
2
−〈x〉
2
= 〈X ψ|X ψ〉−〈ψ|X ψ〉
2
=
ZZZ
x −〈x〉
2
ψ( r, t)
2
d
3
r = kX ψ −〈x〉ψk
2
.
DEFINITION 13.14 The momentum u nce rtainty of a particle characterized
by the wave function ψ( r, t) is the quantity ∆ p
x
defined by the following
equivalent expressions:
(∆p
x
)
2
=
p
2
x
−〈p
x
〉
2
= 〈P ψ|P ψ〉−〈ψ|P ψ〉
2
=
ZZZ
}h
2
∂ψ
∂ x
( r, t)
2
d
3
r −〈p
x
〉
2
=
ZZZ
p
2
x
Ψ( p, t)
2
d
3
p −〈p
x
〉
2
= kP
x
ψ −〈p
x
〉ψk
2
Note that
p
2
x
may also be expressed as the average value of the operator
P
2
x
= −}h
2
∂
2
∂ x
2
in the state |ψ〉, since an easy integration by parts reveals that
P
2
x
def
=
ψ
P
2
x
ψ
= −}h
2
ZZZ
ψ( r, t)
∂
2
ψ
∂ x
2
( r, t) d
3
r
=
ZZZ
}h
2
∂ψ
∂ x
( r, t)
2
d
3
r.
To perform this integration by parts, it is necessary that the derivative of ψ
decay fast enough, which is the case when 〈∆p
x
〉 exists.
Remark 13.15 The average values and the uncertainties should be compared with the expecta-
tion value and standard deviation of a random variable (see Chapter 20).
We are going to show now t hat there exists a relation between ∆x and
∆p
x
. To star t with, we have the following lemma:
LEMMA 13.16 Let ψ be a wave function.
i) If the wave function is translated by the vector a ∈ R
3
by putting
ψ
a
( r, t)
def
= ψ( r − a, t),
then neither ∆x nor ∆ p
x
is changed.
ii) Similarly, if a translation by the quantity k ∈ R
3
is performed in momentum
space, or, what amounts to the same thing, if the wave function in position
representation is multiplied by a phase factor by defining
Heisenberg uncertainty relations 363
ψ
k
( r, t)
def
= ψ( r, t) e
i k·r / }h
,
then neither ∆x nor ∆p
x
is changed.
iii) Consequently, it is possible to define a wave function
ˆ
ψ( r, t) which is centered
in p osition and momentum (i.e., such that the average position and the average
momentum are zero) and has the same uncertainties as the original function
ψ( r, t) with respect to position a nd momentum.
Proof. The average position of the translated wave function ψ
a
is
〈x〉
a
=
ZZZ
x
ψ
a
( r, t)
2
d
3
r =
ZZZ
(x −a
x
)
ψ( r, t)
2
d
3
r = 〈x〉−a
x
.
The position uncertainty is then
(∆x)
2
a
=
x
2
a
−〈x〉
2
a
=
ZZZ
x
2
ψ( r − a, t)
2
d
3
r −
〈x〉− a
x
2
=
ZZZ
(x −a
x
)
2
ψ( r, t)
2
d
3
r −
〈x〉
2
−2a
x
〈x〉+ a
2
x
=
x
2
−2a
x
〈x〉+ a
2
x
−
〈x〉
2
−2a
x
〈x〉+ a
2
x
=
x
2
−〈x〉
2
= (∆x)
2
.
The momentum uncertainty of |ψ〉 is of course unchanged.
The proof is identical for the translates in momentum space.
Finally, the centered wave functio n is, for a given t, equal to
ˆ
ψ( r, t)
def
= ψ
r − 〈r 〉, t
e
i〈p〉·r/ }h
.
THEOREM 13.17 (Heisenberg uncertainty relations) Let ψ be a wave function
for which the average position and avera ge momentum, as well as the position and
momentum uncertainties, are defined. Then we have
∆x ·∆ p
x
¾
}h
2
(13.1)
(and similarly for y and z).
Moreover, equality holds only if ψ is a gaussian function.
Proof. Translating the wave function as in Lemma 13.16, we may assume that 〈x〉 = 0
and 〈p
x
〉 = 0. Moreover, to simplify notation, we omit the dependency on t. We have
(∆x)
2
=
ZZZ
x
2
ψ( r)
2
d
3
r and (∆p
x
)
2
= }h
2
ZZZ
∂ψ
∂ x
( r )
2
d
3
r.
The Cauchy-Schwarz inequality for functions in L
2
states that
ZZZ
f ( r ) g( r ) d
3
r
2
¶
ZZZ
f ( r )
2
d
3
r ·
ZZZ
g( r )
2
d
3
r.
Applying this inequality to f ( r) = x ψ( r) and g( r) =
∂ψ
∂ x
( r ), we obtain
ZZZ
x ψ( r)
∂ψ
∂ x
( r ) d
3
r
2
¶
ZZZ
x
2
ψ( r)
2
d
3
r ·
ZZZ
∂ψ
∂ x
( r)
2
d
3
r
¶
1
}h
2
(∆x)
2
(∆p
x
)
2
. (∗)
364 Physical applications of the Fourier transform
But, using an integration by parts, since x is real and the boundary terms cancel (see
Remark 13.19 concerning this point), we can write
ZZZ
x ψ( r)
∂ψ
∂ x
( r) d
3
r = −
ZZZ
ψ( r)
2
d
3
r −
ZZZ
x ψ( r)
∂ψ
∂ x
( r ) d
3
r,
or 2 Re
ZZZ
x ψ( r)
∂ψ
∂ x
( r) d
3
r
= −
ZZZ
ψ( r)
2
d
3
r = −1.
Since |z| ¾ |Re(z)| for all z ∈ C, t he left-hand side of (∗) satisfies
ZZZ
x ψ( r)
∂ψ
∂ x
( r ) d
3
r
2
¾
Re
ZZZ
x ψ( r)
∂ψ
∂ x
( r ) d
3
r
2
=
1
4
.
This immediately implies (13.1).
The Cauchy-Schwarz inequality is an equality only i f the two f u nctions considered
are proportional; but it is easy to see that X ψ and ∂ψ/∂ x can only be propor tional if
ψ is a gaussian as a function of x (this is a simple differential equation to solve).
If the usual definition of the Fourier transform is kept (with the factor 2π
and without }h), t he following equivalent theorem is obta ined:
THEOREM 13.18 (Uncertainty relation) Let f ∈ L
2
(R) be a function such that
the second moment
R
x
2
|f (x)|
2
dx and
R
|f
′
(x)|
2
dx both exist. Define
x
2
def
=
1
kf k
2
2
Z
x
2
f (x)
2
dx,
ν
2
def
=
1
kf k
2
2
Z
ν
2
e
f (ν)
2
dν =
4π
2
kf k
2
2
Z
f
′
(x)
2
dx.
Then we have
x
2
·
ν
2
¾
1
16π
2
with equality if and only if f is a gaussian.
Remark 13.19 A point that was left slightly fuzzy above requires explanation: the cancellation
of the boundary terms during the integration by parts. Denote by H the space of square
integrable functi ons ψ, such that X ψ and P
x
ψ are also square integrable. This space, with the
norm N defined by
N (ψ)
2
= kψk
2
2
+ kX ψk
2
2
+ kP ψk
2
2
=
ZZZ
ψ( r)
2
d
3
r +
ZZZ
x
2
ψ( r)
2
d
3
r +
ZZZ
p
2
x
Ψ( p)
2
d
3
p,
is a Hilbert space.
If ψ ∈ H is of C
∞
class with bounded support with respect to the variable x, the proof
above (and in particular the integration by parts w ith the boundary terms vanishing) is correct.
A cl assical result of analysis (proved using convolution with a Dirac sequence) is that the
space of C
∞
functions with bounded support is dense in H . By continuity, the preceding
result then holds for all ψ ∈ H .
Remark 13.20 Another approach of the uncertainty relations is p roposed in Exercise 14.6 on
page 404.
Analytic signals 365
13.4
Analytic signals
It is customary, in electricity or optics, for instance, to work with complex
sinusoidal signals of the type e
iωt
, for which only the real part has physical
meaning. This step from a real signal f
(R)
(t) = cos(ωt) to a complex signal
f (t) = e
iωt
may be generalized in the case of a nonmonochromatic signal.
First of all, notice that the real signal may be recovered in two different
ways: as the real part of th e associated complex signal, or as the average of the
signal and its complex conjugate.
We can generalize this. We consider a real signal f
(R)
(t) and search for
the associated complex signal. In the case f
(R)
(t) = cos(2πν
0
t) (with ν
0
> 0),
the spectrum of f
(R)
contains both frequencies ν
0
and −ν
0
. However, this
information is redundant, since the intensity for the frequency −ν
0
is the same
as that intensity for the frequency ν
0
, since f
(R)
is real-valued. The complex
signal f (t) = e
2πiνt
associated to the real signal f
(R)
is obtained as follows:
take the spectrum of f
(R)
, keep only the positive frequencies, multiply by two,
then reconstitute the signal.
In a general way, for a real-valued signal f
(R)
(t) for which the Fourier trans-
form
e
f
(R)
(ν) is defined, its spectrum is hermitian, that is , we h ave
e
f
(R)
(ν) =
e
f
(R)
(−ν) for a ll ν ∈ R;
thus, “half” the spectrum
e
f
(R)
suffices to recover f
(R)
.
If the spectrum
e
f
(R)
(ν) does not have a singularity at the origin, or at least
if is sufficiently regular, we can consider the truncated spectrum
2H (ν) ·
e
f
(R)
(ν).
The inverse Fourier transform of this truncated spectrum will give the analytic
signal associated to t he function f
(R)
. Since the inverse Fourier transform of
2H (ν) is
F
−1
2H (ν)
= F
−1
[1 + sgn ν] = δ(t) + pv
i
πt
(see Theorem 11.16 on page 304), we deduce tha t the inverse Fourier transform
of 2H (ν) ·
e
f
(R)
(ν) is
F
−1
2H (ν) ·
e
f
(R)
(ν)
=
δ + pv
i
πt
∗ f
(R)
= f
(R)
(t) −
i
π
pv
Z
f
(R)
(t
′
)
t
′
− t
dt
′
.
We make the following definition:
DEFINITION 13.21 An analytic signal is a function with caus al Fourier trans-
form, t hat is, a function f such that the Fourier transform vanishes for nega-
tive frequencies.

366 Physical applications of t he Fourier tran sform
DEFINITION 13.22 Let f
(R)
be a real signal with sufficiently regular Fourier
transform.
5
The imagina ry signal associated to f
(R)
is the opposite of its
Hilbert transform:
f
(I)
(t)
def
= −
1
π
pv
Z
f
(R)
(t
′
)
t
′
− t
dt
′
.
DEFINITION 13.23 The analytic signal associated to the real signal f
(R)
is the
function
t 7−→ f (t)
def
= f
(R)
(t) + i f
(I)
(t) = f
(R)
(t) −
i
π
pv
Z
f (t
′
)
t
′
− t
dt
′
,
the spectrum of which is
e
f (ν) = 2H (ν) ·
e
f
(R)
(ν).
The analytic signal associated with f
(R)
is analytic in the sense of Defini-
tion 13.21: it s spectrum is identically zero for frequencies ν < 0. Since
e
f (ν)
is causal, it has a Laplace transform F (p) defined at least on the right-hand
half-plane of the complex plane. Since the signal f is the inverse Fourier
transform of
e
f , it is given by
f (x) = F (−2πix).
Hence there is an analytic continuation of f to the upper half-plane,
6
which can
be obtained using the Laplace transform of t he spectrum:
f (z) = F (−2πiz).
This analytic continuation is holomorphic in this u pper half-plane, and there-
fore satisfies the Kramers-Kronig relations — which are neither more nor less
than the relation defining f
(I)
: we’ve made a complete loop!
The fact that f may be c ontinued to an analytic fu nction on the upper half-place
justifies also the terminology “analytic signal.”
All this may be summarized as follows:
THEOREM 13.24 Let f : R → C be a function which has a Fourier transform. If
the spectrum of f is causal, then f may be analytically continued to the complex upper
half-plane.
Conversely, if f may be analytically continued to this half-plane, and if the integral
of f along a half-circle in the upper half-plane tends to 0 as the radius tends to infinity,
then the spectrum of f is causal.
(See [79] for more details.)
5
In the sense that the product H (ν)
e
f
(R)
(ν) exists; this means that
e
f
(R)
cannot involve a
singular distribution at the origin 0.
6
The upper half-plane because of the similitude x 7→ −2πix: the i mage of the upper
half-plane is the right-hand half-plane.
