Appel W. Mathematics for Physics and Physicists

Подождите немного. Документ загружается.

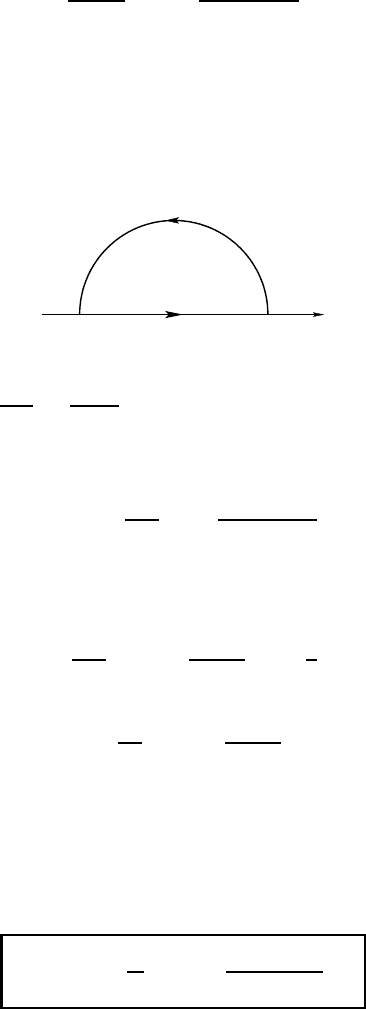
Cauchy principal value 227

Notice — and this will be convenient in certain computations — that

x − x

= Re



x − x

±iǫ



8.1.d Kramers-Kronig relations

Let F be a function from C into C which is meromorphic on C but holomor-

phic on the closed upper half-plane, which means that all the poles of F have

strictly negative imaginary part. Consider now the contour C described below,

with an arbitrarily large radius:

From Cauchy’s formula, we have

2πi

F (ζ )

ζ − z

dζ =

F (z) if Im(z) > 0,

0 if Im(z) < 0.

In this formula, put z = x + iǫ with x ∈ R and ǫ > 0:

F (x + iǫ) =

2πi

+∞

−∞

F (x

′

)

′

− x −iǫ

′

Assume that th e integral on the half-circle tends to 0 when the radius tends to

inﬁnity. By letting ǫ tend to 0, and by continuity of F , the preceding results

lead to

F (x) =

2πi

+∞

−∞

F (x

′

)

′

− x

′

F (x),

hence

F (x) =

iπ

+∞

−∞

F (x

′

)

′

− x

′

This equation concerning F becomes much more interesting by taking succes-

sively its real and imaginary parts; the following theorem then follows:

THEOREM 8.2 (Kramers-Kronig relations) Let F : C → C be a meromorp hic

function on C, holomor phic in the u pper half-plane and going to 0 sufﬁciently fast at

inﬁnity in this upper half-plane. Then we have



F (x)



+∞

−∞



F (x

′

)



′

− x

′

228 Distributions II

and Im



F (x)



= −

+∞

−∞



F (x

′

)



′

− x

′

These are called dispersion relations and a re very useful in optics (s ee, e.g., the

book by Born and Wolf [14, Cha pter 10]) and in statistical physics. Physicists

call these formulas the Kramers-Kronig relations,

while mathematicians say

that Re(F ) is the Hilbert transform of Im(F ).

Remark 8.3 If F is meromorphic on C and holomorphic in the lower h al f-plane (all its poles

have positi ve imaginary parts), then it satisﬁes relations which are dual to those just proved

(also called Kramers-Kronig relations, w h ich does not simplify matters):



F (x)



= −

+∞

−∞



F (x

′

)



′

− x

′

and Im



F (x)



+∞

−∞



F (x

′

)



′

− x

′

We will see, in Chapter 13, that the Fourier transform of causal functions t 7→ f (t) (those

that vanish for negative values of the variable t), when it exists, sat isﬁes the Kramers-Kronig

relations of Theorem 8.2.

Remark 8.4 What happens i f F is holomorphic on both the upper and the lower half-plane?

Since it is assumed that F has no pole on the real axis, it i s then an entire function. The

assumption that the integ ral on a circle tends to zero as the radius gets large then leads (by the

mean value property) to the vanishing of the function F , which is the only way to reconcile

the previous formulas wit h t h o se of Theorem 8.2.

Remark 8.5 In electromagnetism, the electric induction D and the electric ﬁeld E are linked,

for a monochromatic wave, by

D( x, ω) = ǫ(ω) E( x, ω),

where ǫ(ω) is the dielectric constant of the material, depending on the pulsation ω of the

waves. This relation can be rewritten (via a Fourier tranform) i n an integral relation between

D( x, t) and E( x, t):

D( x, t) = E( x, t) +

+∞

−∞

G(τ) E( x, t −τ) dτ,

where G(τ) is the Fourier transform of ǫ(ω) −1. In fact, since the ele ctric ﬁeld is the physical

ﬁeld and the ﬁeld D is derived from it, the previous relation must be c au sal, that is, G(τ) = 0

for any τ < 0. One of the main consequences is that the function ω 7→ ǫ(ω) is analytic in the

lower half-plane of the complex plane (see Chapter 12 on the Laplace transform). From the

Kramers-Kronig relations, interesting information concerning the function ω 7→ ǫ(ω) can be

deduced. Thus, if the plasma frequency is deﬁned by ω

= lim

ω→∞

[1 − ǫ(ω)], we obtain

the sum rule

+∞

ω Im



ǫ(ω)



dω.

The reader is invited to read, for instance, the book by Jackson [50 , Chapter 7.10] for a more

detailed presentation of this sum rule.

Dispersion relations ﬁrst appeared in physics in the study of the dielectric constant of

materials by R. de L. Kronig in 1926 and, independently, in the theory of scattering of light

by atoms by H. A. Kramers in 1927.

Cauchy principal value 229

 Exercise 8.1 Using the Kramers-Kronig relations, show that

+∞

sin x

dx =

(Solution page 245)

8.1.e A few equations in the sense of d istributions

Recall (Theorem 7.28 on page 191) that the solutions of the equation x·T (x) =

0 are the multiples of δ.

PROPOSITION 8.6 We have x ·pv

= 1 (where 1 is the constant function x 7→ 1).

Proof. Indeed, for any test function ϕ ∈ D(R), we have by deﬁnition of the product

of a distribution (pv

) by a C

∞

function:



x pv

, ϕ



, xϕ

= lim

ǫ→0

|x|>ǫ

x ϕ(x)

dx =

+∞

−∞

ϕ(x) dx = 〈1, ϕ〉.

THEOREM 8.7 (Solutions of x · T ( x) = 1) The solutions, in the space of distribu-

tions, of the equation

x · T (x) = 1,

are the distributions given by T (x) = pv

+ αδ, with α ∈ C.

Proof. If T satisﬁes x · T (x) = 1, t h en S = T −p v(1/x) satisﬁes x · S (x) = 0 and

thus, according to Theorem 7.28 on page 191, S is a multiple of δ.

Theorem 7.28 generalizes as follows:

PROPOSITION 8.8 Let n ∈ N. The solutions of equation x

· T (x) = 0 are the

linear combinations of δ, δ

′

, . . . , δ

n−1

Let f be a function continuous on R, with isolated zeros. For ea ch such

zero x

of f , we s ay that x

is of multiplicity m if there exists a continuous

function g, which does not vanish in a neighborhood of x

, such that f (x) =

g(x) for x ∈ R.

If f ha s only isolated zeros with ﬁnite multiplicity, then one can treat the

equation f (x) · T (x) = 0 locally around each zero in the same manner as

T (x) = 0.

THEOREM 8.9 (Solutions of f ( x) · T ( x) = 0) Let f be a continuous fu nction

on R. Denote by Z( f ) = {x

i ∈ E} the set of its zeros, and assume these zeros

are isolated and with ﬁnite multiplicities m

The distributions solutions to the equation f (x) · T (x) = 0 are given by

T (x) =

i∈I

−1

k=0

i,k

(k)

(x − x

where α

i,k

are arbitrary complex numbers.

230 Distributions II

Example 8.10 The equation sin x · T (x) = 0 has for solutions the distributions o f the form

n∈Z

δ(x −nπ).

The equation (cos x −1) · T (x) = 0 has for solutions the distributions of the form

n∈Z



δ(x −nπ) + β

′

(x −nπ)



THEOREM 8.11 (Solutions of ( x

− a

) · T ( x) = 1) Let a > 0 be given. The

distribution solutions of the equation (x

−a

) · T (x) = 1 are those given by

−a

+ α δ(x −a) + β δ(x + a),

where α and β are complex numbers, and where we denote

−a



x + a

−pv

x − a



8.2

Topology in DD

′

8.2.a Weak convergence in DD

′

DEFINITION 8.12 A sequence of distributions (T

)

k∈N

converges wea kly (or

simply “converges”) in D

′

if, for any test function ϕ ∈ D, the sequence of

complex numbers 〈T

, ϕ〉 converges in C. We then denote by 〈T , ϕ〉 this limit,

which deﬁnes a linear function T on D, called the weak limit of (T

)

k∈N

in D

′

THEOREM 8.13 If (T

)

k∈N

converges weakly in D

′

to a functional T , then T is

continuous and is therefore a distribution. The weak convergence in D

′

is denoted by

′

−−→ T .

 Exercise 8.2 For n ∈ N, let T

be the regular distribution associated to the loc al ly integrable

function x 7→ sin(nx). Show that the sequence (T

)

n∈N

converges weakly to 0 in D

′

THEOREM 8.14 (Continuity of differentiation) The operation of differentiation

in D

′

(R) is a continuous linear operation: if T

′

−−→ T , then for any m ∈ N, we

have T

(m)

′

−−→ T

(m)

Obviously this extends to partial derivatives of distr ibutions in R

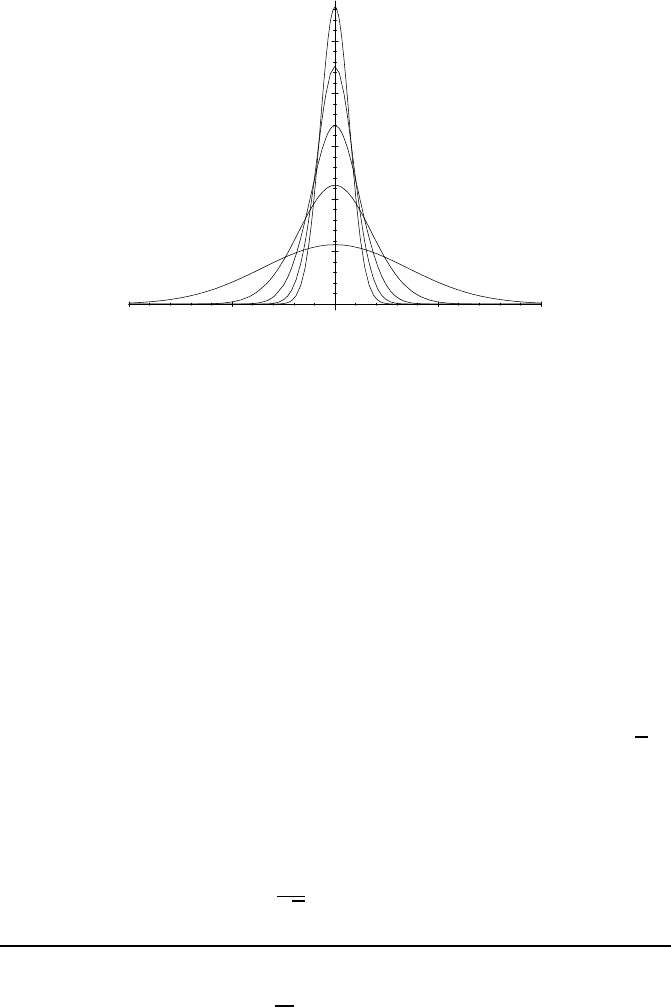
Topology in D

′

231

−2

−1 1

Fig. 8.3 — The sequence of gaussian functions (g

)

n∈N

, for n = 1, . . . ,5.

This theorem is extremely powerful and justiﬁes the interchange of limits

and derivatives, which is of course impossible in the sense of functions.

8.2.b Sequences of functions converging to δ

DEFINITION 8.15 A Dirac se quence (of functions) is any sequence of func-

tions f

: R → R locally integrable, s uch that

➀ there exists a real number A > 0 such that, for any x ∈ R and any

k ∈ N, we have



|x| ¶ A



=⇒



(x) ¾ 0



➁ for any a ∈ R, a > 0,

|x|¶a

(x) dx −−−→

k→∞

1 and f

(x)

cv.u.

−−→ 0 on a < |x| <

Example 8.16 An e xample of a Dirac sequence is the sequence ( f

)

n∈N

deﬁned by f

(x) =

nΠ(nx) for any x ∈ R and any n ∈ N. (Check this on a drawing.)

Example 8.17 The sequence of functions (g

)

n∈N

deﬁned by

(x)

def

−n

for x ∈ R

Consider, for instance, the sequence of functions ( f

)

n∈N

deﬁned by

(t) =

k=1

cos 2kt for any t ∈ R.

The functions f

are all differentiable, but their limit f is a “sawtooth” function, which is not

differentiable at the points of the form nπ with n ∈ Z.

232 Distributions II

−2

−1 1

Fig. 8.4 — The sequence of fu nct ions ψ

(x) =

sin

(πnx)

nπ

for n = 1 to 5.

is a Dirac sequence (see Figure 8.3 on the previous page).

THEOREM 8.18 Any Dirac sequence converges weakly to the Dirac distribution δ

in D

′

(R).

Proof. See Appendix D.

Example 8.19 Similarly, the sequence of functions (ψ

)

n∈N

deﬁned by

(x) =

sin

(πnx)

nπ

for x ∈ R

is a Dirac sequence (see Figure 8.4) and thus converges also to δ.

Remark 8.20 Without the positivit y assumption for x close to 0, there is nothing to prevent

contributions proportional to δ

′

to arise in the limit! Thus, consider the sequence of functions

)

n∈N

given by the graph

−n

−

It is easy to show that k

′

−−→ δ

′

. Let ( f

)

n∈N

be a Dirac sequence; then the sequence

( f

+ k

)

n∈N

, which still satisﬁes ➁, but not ➀, converges to δ + δ

′

and not to δ.

Topology in D

′

233

−2

−1

−0,5 0,5

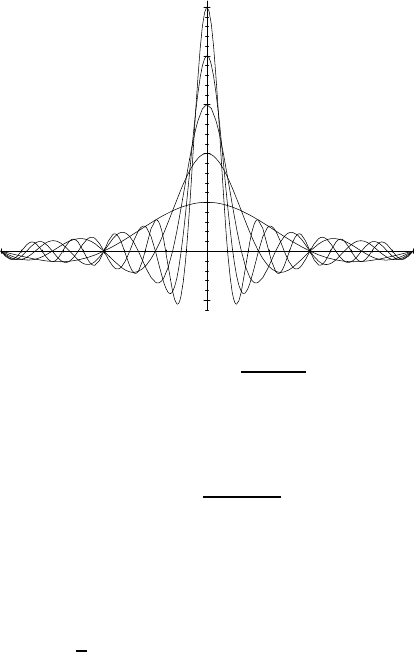
Fig. 8.5 — The functions b

: x 7−→

sin 2πnx

πx

, for n = 1, . . . ,5.

Consider now the sequence of functions

(x) =

sin 2πnx

πx

All the functions b

share a common “envelope” x 7→ 1/πx and the sequence

of real numbers (b

(x))

n∈N

does not converge if x /∈ Z (see Figure 8.5). We do

have b

(0) → ∞, but , for instance,





(−1)

/2π if n = 2k + 1,

0 if n = 2k.

In particular, this sequence (b

)

n∈N

is not a Dirac sequence. Yet, despite this

we have the following result:

PROPOSITION 8.21 The sequence (b

)

n∈N

converges to δ in the sense of distribu-

tions.

How to e xplain t his ph enomenon?

Proving the convergence of (b

)

n∈N

to δ is, b ecaus e of the lack of pos-

itivity, harder than for a Dirac sequence. In the case that concerns us, the

sequence of functions (b

)

n∈N

exhibits ever faster oscillations wh en we let n

tend to inﬁnity (see Figure 8.5), and, after getting rid of t he singularity at 0,

we can hope to be able to invoke the Riemann-Lebesgue lemma (see Theo-

rem 9.44). Moreover, it is easy to compute that

= 1 for all n ∈ N

∗

(for

example, by the method of residues).

Proof of Proposition 8.21. Let ϕ ∈ D be a test function and let [−M, M] be an

interval containing the support of ϕ.

234 Distributions II

We can always write ϕ(x) = ϕ(0) + x ψ(x), where ψ if of C

∞

class. Then we have

〈b

, ϕ〉 = ϕ(0)

−M

sin 2πnx

πx

dx +

−M

ψ(x) sin(2πnx) dx,

and the ﬁrst integral, via the substitution y = nx, tends to the improper integral

+∞

−∞

sin πx

πx

dx = 1 ,

whereas the second integral tends to 0, by the Riemann-Lebesgue lemma.

Finally, t he convergence to δ is highly useful because of the following

theorem:

THEOREM 8.22 Let ( f

)

n∈N

be a Dirac sequence of functions. If g is a bounded

continuous function on R, then f

∗g exists for all n ∈ N and the sequence ( f

∗g)

n∈N

converges uniformly to g.

If g is not continuous but the limits on the left or the right of g exist at the point x,

then f

∗ g(x) tends to



g(x

−

) + g(x

)



8.2.c Convergence in DD

′

and c onvergence

in the sense of functions

The previous examples show that a sequence ( f

)

n∈N

of regular distributions

may very well converge in D

′

to a regular distribution f without converging

pointwise almost everywhere (and even more wit hout converging uniformly)

in the sense of functions. I n particular, the Riemann-Lebesgue lemma is

equivalent to the following proposition:

PROPOSITION 8.23 The sequence of regular distributions ( f

)

n∈N

deﬁned by the

functions f

: x 7→ sin(nx) converges to 0 in D

′

, but it converges pointwise only for

x ∈ πZ.

8.2.d Regularization of a distribution

What happens w hen we take the convolution of a function f of C

class

with a function g of C

class? Assuming that this convolution exists and

denoting h = f ∗ g, the function h will be inﬁnitely differentiable in the sense

of distributions, but how many times will it be differentiable in the sense of

functions?

Compute the derivative. It is possible to write h

′

= f

′

∗ g and, since f

′

and g are f unctions, h

′

is well-deﬁned in the sense of functions. Continue. It

is not possible to differentiate f more than that, but g is still available, and

so h

′′

= f

′

∗ g

′

is still a function, as is the third derivative h

′′′

= f

′

∗ g

′′

. But

going farther is not possible.

Similarly, the convolution of a f unction of C

class with a function of C

ℓ

class will produce a function of C

k+ℓ

class.

Topology in D

′

235

These results generalize to distributions. Of course, it is not reasonable

to speak of “C

∞

class” (since distributions are always inﬁnitely differentiable

anyway). But “regularity” for a distribution consists precisely in being what

is called a “regular distribution,” namely, a distr ibution associated to a locally

integrable function.

An interesting result (wh ich is, in fact, quite intuitive) is that the con-

volution by a C

∞

function “smoothes” the object which is sub ject to the

convolution: the result is a function which is C

∞

. This is th e ca se also if

we take the convolution of a distribution with a C

∞

function: the result is

a regular distribution associated to a function, and in certain cases one ca n

show th at the result is also C

∞

THEOREM 8.24 (Regularization of a distribution) Let T ∈ D

′

be a distribution

and ψ ∈ C

∞

(R), and assume that T ∗ψ exists. Then T ∗ψ is a regular distribution

associated to a func tion f , which is given by

f (x) = T ∗ψ(x) =



T (t), ψ(x − t)



Let T ∈ D

′

and ϕ ∈ D (so ϕ is now not only of C

∞

class, but also with

bounded support). Then T ∗ ϕ exists and is a regular distribution associated to a

function g of C

∞

class, with derivatives given by the formula

(k)

(x) =



T (t), ϕ

(k)

(x − t)



8.2.e Continuity of convolution

The following theorem shows that the operation of convolution with a ﬁxed

distribution is continuous for the weak topology on D

′

THEOREM 8.25 (Continuity of convolution) Let T ∈ D

′

. If (S

)

k∈N

converges

weakly in D

′

to T , and if moreover all the S

are su pported in a ﬁxed closed subset

such that all the S

∗ T exist, then the sequence (S

∗ T ) converges in D

′

to S ∗ T .

Consider now a Dirac sequence (γ

)

n∈N

, with elements belonging to D.

It converges weakly to δ in D

′

(this is Theorem 8.18); moreover, we know

(Theorem 8.24) that if T is a distribution, we have γ

∗ T ∈ C

∞

(R). Since

∗ T −−−→

n→∞

T , we deduce:

THEOREM 8.26 (Density of DD

DD in DD

′

) Any distribution T ∈ D

′

is the limit in

′

of a sequence of f unctions in D. One says that D is dense in D

′

In ot her words, any d istribution is the weak limit of a sequence of func-

tions of C

∞

class with bounded support.

 Exercise 8.3 Find a sequence (ϕ

)

n∈N

in D which converges weakly to X in D

′

(See Exercise 8.11 on page 242)

236 Distributions II

8.3

Convolution algebras

DEFINITION 8.27 A convolution algebra is any vector space of distributions

containing the Dirac distribution δ and for which the convolution product

of an arbitrary number of factors is deﬁned.

There are two well-known and very important examples.

THEOREM 8.28 (EE

′

, DD

′

and DD

′

−

) The space E

′

of distributions with bounded sup-

port is a convolution algebra.

The space of distributions with support bounded on the left, denoted DD

′

and

the space of distributions with support bounded on the right, denoted DD

′

−

, are also

convolution algebras.

These algebras, as the name indicates, provide means of solving certain

equations by means of algebraic manipulations. In particular, many physical

problems can be put in the form of a convolution equation of the type

A ∗ X = B

where A and B are distributions (B is often called the “ source”) and the

unknown X is a d istribution.

The problem is to ﬁnd a solution and to

determine if it is unique. So we are, quite naturally, led to the search for a

distribution denoted A

∗−1

, which is called the convolution inverse of A or,

sometimes, Green function of A, which will be such th at

A ∗ A

∗−1

= A

∗−1

∗ A = δ.

Remark 8.29 This inverse, if it exists, is unique in the algebra under consideration. Indeed, suppose

there exist, in a same algebra, two distributions A

′

and A

′′

such that

A ∗ A

′

= A

′

∗ A = δ and A ∗ A

′′

= A

′′

∗ A = δ.

Then

′′

= A

′′

∗δ = A

′′

∗(A ∗ A

′

) = (A

′′

∗ A) ∗ A

′

= δ ∗ A

′

= A

′

and therefore

′′

= A

′

For instance, notice that any linear differential equation with constant coefﬁcie nts



+ a

+ ···+ a



X (t) = B(t)

can be reexpressed in the form of a convolution product



δ + a

′

+ ···+ a

(n)



∗ X (t) = B(t).