Appel W. Mathematics for Physics and Physicists

Подождите немного. Документ загружается.

Hilbert spaces 257

David Hilbert (1862—1943), born in Königsberg in Germany, ob-

tained his ﬁrst academic position in this town at the age of 22. He

left for G öttingen eleven years later and he ended his career there in

1930. A many-faceted genius, he was interested in the foundations of

mathematics (in particular the problem of axiomatization), but also

in mechanics, general relativity, and number theory, and invented

the notion of completeness, opening the way for the study of what

would later be called Hilbert spaces. His name remains attached to a

list of 23 problems he proposed in 1900 for the attention of math-

ematicians present and future. Most have been resolved, and some

have been shown to be indecidable.

DEFINITION 9.15 Let H be a pre-Hilbert space. If H is complete for its

hermitian norm, then it is called a Hilbert space.

9.3.a Hilbert basis

The spaces we are going to consider from now on will be of inﬁnite d imension

in general. It is harder to work with spaces of inﬁnite dimension since many

convenient properties of ﬁnite-dimensional vector spaces (for instance, that

∗

≃ E) are not true in general. The interest of Hilber t spaces is to keep

many of these properties; in particular, the notion of basis can be generalized

to inﬁnite-dimensional Hilbert spaces.

DEFINITION 9.16 (Total system) Let H be a Hilbert space. A family (e

)

n∈I

of vectors indexed by a set I, countable or not, is a total system if

; i ∈ I}

⊥

= {0},

or, in other words, if



(x|e

) = 0, ∀i ∈ I



⇐⇒ (x = 0).

We now wish to know if it is possible to extend completely the notion

of orthonormal basis of a ﬁnite-dimensional Hilbert space, and especially to

recover t he formulas of Theorem 9.10 — tha t is, is it possib le to write

x =

n∈N

(x|e

) e

and kxk

n∈N



(x|e

)



In what follows, we consider a countable orthonormal system (e

)

n∈N

, and

deﬁne an increasing sequence of subspaces (V

)

n∈N

given by

def

= Vect{e

, e

, . . . , e

} for any p ∈ N.

Such is the case of the continuum hypothesis, a result due to P. Cohen in 1963.

258 Hilbert spaces; Fourier series

Erhard Schmidt (1876—1959) studied in his native city of Derpt,

in Estonia, then in Berlin (with Schwarz) and in Göttingen.

There, he defended his thesis (supervised by Hilbert) in 1905.

After positions in Zurich, Erlangen, and Breslau, he was hired as

professor at the University of Berlin in 1917. He continued the

ideas o f Hilbert concerning integral equations and deﬁned the

notion of a Hil bert space (1905). He also introduced self-adjoint

operators in Hilbert space, was interested in functional analysis

and integral equations, and, in his studies of systems of inﬁnitely

many equations with inﬁnitely many unknowns, introduced “ge-

ometric” notation, w h ich le d to the geometric interpretation of

Hilbert spaces.

Also we introduce the sequence of projections of a vector x on the sub-

spaces V

DEFINITION 9.17 Let x ∈ H . The partial Fourier serie s of x wit h respect to

the orthonormal system (e

)

n∈N

is the sequence of sums S

(x) given by

(x)

def

n=0

|x) e

, for any p ∈ N.

In other words, S

(x) is the orthogonal projection of x on V

. The coefﬁcient

(x)

def

= (e

|x) is called the n-th Fourier coefﬁcient of x.

Does the partial Fourier series converge to x, which is what we would like?

The answer is no in general. However, we have the following result:

PROPOSITION 9.18 For any x ∈ H , the sequence



(x)



n∈N

is a Cauchy se-

quence for the hermitian norm, and therefore it converges in H .

Proof. From Be ssel’s inequality it follows that t h e series

|(e

|x)|

has

bounded partial sums; since it is a series with positive terms, it is th erefore convergent,

and consequently it is a Cauchy sequence. Hence the quantity



(x) − S

(x)



n= p+1



n= p+1

is the Cauchy remainder of a convergent se ries of real numbers, and it tends to 0 when

[p, q → ∞]; this shows that the sequence



(x)



n∈N

is a Cauchy sequence in H which

is, by assumption, complete. So it converges in H .

This is not sufﬁcient to s how that the sequence



(x)



n∈N

converges to x;

as far as we know, it may very well converge to an entirely different vector.

This is the reason for the introduction of the Hilbert basis.

Hilbert spaces 259

DEFINITION 9.19 (Hilbert basis) Let H be a Hilbert space. A family (e

)

i∈I

is a Hilbert basis of H if it is a free family such that Vect



; i ∈ I



dense

in H .

An orthonormal Hilbert basis is a Hilbert basis which is als o an or-

thonormal system.

DEFINITION 9.20 (Separable space) A Hilbert space H is separable if there

exists a countable (or ﬁnite) Hilbert bas is.

In wha t follows, all Hilbert spaces considered will be separable.

Take an orthonormal system (e

)

n∈N

. What does it mean for this system to

“be a Hilbert basis”? As the next theorem shows, it is a very strong propert y,

since it provides a unique decomposition of arbitrary vectors as (inﬁnite)

linear combination of the bas is vectors (the system is therefore total).

THEOREM 9.21 Let H be a Hilbert space and let (e

)

n∈N

be an orthonormal system.

The following statements are equivalent:

a) (e

)

n∈N

is a Hilbert basis, that is, Vect{e

; n ∈ N} is dense in H ;

b) for a ny x ∈ H , if we put x

def

i=0

|x) e

, then we have

lim

n→∞

kx − x

k = 0;

c) for any x, y ∈ H , we have (x|y) =

∞

i=0

(x|e

) (e

|y);

d) for any x ∈ H , w e have the Parseval identity kxk

∞

i=0



|x)



;

e) the system (e

)

n∈N

is a total system: for x ∈ H , we have



|x) = 0 for any i ∈ N



⇐⇒



x = 0



Proof. Denote T

= Vect{e

, . . . , e

} and T =

n∈N

a) ⇒ b): by assumption, T is dense in H , hence T = H . Let ǫ > 0. There exists

y ∈ T such t h at kx − yk ¶ ǫ. Moreover, since y ∈ T , there e xists N ∈ N such

that y ∈ T

, so that, since the sequence (T

)

n∈N

is increasing for inclusion, y ∈ T

for any n ¾ N . In addition the projection of x on T

achieves the shortest distance

(Pythagoras’ theorem), hence kx − x

k ¶ kx − yk ¶ ǫ for any n ¾ N .

b) ⇒ c): an easy c o mputation shows that (x

) =

i=0

(x|e

) (e

|y) and letting n

go to inﬁnity gives the result.

c) ⇒ d): immediate.

In ot h er words, for a Hil bert basis (e

)

i∈I

, any vector in H can be approached, with

arbitrarily high precision, by a ﬁnite l inear combination of the vectors e

In certain books, countability is part of the deﬁnition of a Hilbert basis.

260 H i lbert spaces; Fourier series

d) ⇒ e): immediate.

e) ⇒ a): let x ∈ H and denote y = lim

n→∞

(from Theorem 9.18 , we know that the

limit exists). Then (x − y|e

) = 0 for any i ∈ N, so that x = y. In particular, the

sequence (x

)

n∈N

of elements of T converges to x, and T is therefore dense in H .

So, an orthonormal H ilb ert ba sis is a total orthonormal system — and is

something very close to an algebraic basis, since it is a free family and it is

“almost” generating. For an arbitrary vector x ∈ H , the sequence



(x)



n∈N

of the partial Fourier series of x with respect to the Hilbert basis (e

)

n∈N

converges to x, so that it is possible to write

x =

n∈N

|x) e

n∈N

(x) e

and (x|y) =

n∈N

(x) c

( y). (9.2)

This means that the comput ational formulas seen in the ﬁ nite-dimensional

case a re equally valid here.

THEOREM 9.22 (Computations in a Hilbert basis) Let (e

, . . . , e

) be a Hilbert

basis of H . For any x, y ∈ H , we h ave

x =

∞

n=0

|x) e

, c

(x) = (e

|x) ,

(x|y) =

∞

n=0

(x) c

( y) =

∞

n=0

(x|e

) (e

|y) , kxk

∞

n=0



(x)



COROLLARY 9.23 Two elements of a Hilbert space which have the same Fourier

coefﬁcients with respect to a ﬁxed orthonormal Hilbert basis are equal.

Proof. Let x, y ∈ H have the same Fourier coefﬁcie nts with respect to an ortho nor-

mal Hilbert basis (e

)

n∈N

. We then have c

(x − y) = c

(x) − c

( y) = 0 for any n ∈ N;

hence, according to Property e), it follows that x − y = 0.

PROPOSITION 9.24 In a Hilbert spa ce, all Hilbert basis h ave the same cardinality.

In particular, in a separable H ilbert space, any Hilbert basis is cou nta ble.

COROLLARY 9.25 Every separable Hilbert space is isometric to the space ℓ

, to the

space L

(R), and to the space L

[0, a] (see the next pages for deﬁnitions).

Remark 9.26 In quantum mechanics, it is customary to choose a hermitian scalar product

which is linear on the right and semilinear on the lef t, and we have followed this convention.

However, it should be noted that many mathematics references use the opposite convention. It

is then necessary to write, for instance, c

(x) = (x|e

) instead of (e

|x).

Following Dirac, the vector e

is sometimes denoted |e

〉 and the linear form x 7→ (e

|x) is

denoted 〈e

|. The effect of th e app l ication of a “bra” 〈x| on a “ket” |y〉 is conveniently denoted

〈x| y〉 = (x|y). Then, the proper ty

This is important in quantum mechanics. Indeed, since in general the space considered is

, which is separable, it follows that the family {|x〉}

x∈R

is not a Hilbert basis.

Hilbert spaces 261

〈x| y〉 =

n∈N

(x) c

( y) =

n∈N

(x|e

) (e

|y) =

n∈N

〈x|e

〉〈e

| y〉,

which is valid for all x, y ∈ H , can be written symbolically as

Id =

n∈N

〉〈e

| and 〈x| y〉 = 〈x|Id |y〉.

Similarly, the formula (9.2) can be interp reted as

|x〉 =

n∈N

(x) e

n∈N

|x) e

n∈N

〉〈e

| {z }

x〉.

All of this is detailed in Chapter 14 on page 377.

Two Hilbert spaces will be important in the sequel, the spaces denoted

ℓ

and L

; both are extensively used in quantum mechanics as well as in

signal theory. The two following sections give the deﬁnitions and the essential

properties of those two s paces. I t will be seen that it is possible to translate

from one to the other, a nd t his is precisely the objective of the classical Fourier

series expansion.

9.3.b The ℓ

space

DEFINITION 9.27 The space of complex-valued sequences (a

)

n∈N

such that

the series

converges is called the ℓ

space.

THEOREM 9.28 The space ℓ

, with the hermitian product

(a|b)

def

∞

n=0

is a Hilbert space.

Proof. Because of its length and difﬁcult y, the proof is given in Appendix D,

page 602.

A Hilbert basis of ℓ

is given by the sequence (e

)

n∈N

, wh ere

def

= ( 0, . . ., 0

| {z }

n−1 times

, 1, 0, 0, . . . ).

(In this is recognized the “ba sis” which we were looking for at the beginning

of t his chapter!) Since this Hilbert bas is is countable, the space ℓ

is separable.

Remark 9.29 Because N and Z have same cardinality, ℓ

can also be deﬁned as t h e space of

families (a

)

n∈Z

which are square integrable.

262 Hilbert spaces; Fourier series

9.3.c The space L

[0, a]

Consider the vector space of functions f : [0, a] → C, which are square

integrable. One wishes to deﬁ ne a scalar product and norm deﬁned by

( f , g) 7−→

f (x) g(x) dx and kf k



f (x)



dx.

The problem is that if f and g are equal almost everywhere, even without

being equal, then kf − gk = 0, so this is not really a norm. The way out

of th is difﬁculty is to identify functions which coincide almost everywhere,

namely, to say

that if f and g are equal almost everywhere, then they represent

the same object.

DEFINITION 9.30 Let a ∈ R

∗+

. The L

[0, a] space is the space of measur-

able functions deﬁ ned on [0, a] with values in C w hich are square integrable,

deﬁned up to equality almost everywhere, with the hermitian scalar product

and the norm given by

( f |g)

def

f (x) g(x) dx, and kf k

def

Z



f (x)





1/2

When speaking of a “function” in L

[0, a] , th e actual meaning implied

will be the equivalence class of this function modulo almost everywhere equality.

THEOREM 9.31 (A Hilbert basis) The system (e

)

n∈Z

deﬁned by

(x) =

2πinx/a

for any n ∈ Z and any x ∈ [0, a]

is an orthonormal Hilbert basis of L

[0, a] .

Proof. The proof, which is somewhat delicate, can be found in most real analysis

books, such as [76].

This means that any function in L

[0, a] can be represented as a sum

(inﬁnite) of periodic functions, in the sense of mean-square convergence of the

partial sums toward the or iginal function. This result is the foundation of the

theory of Fourier analysis of periodic functions (see Section 9.4).

In other words, a function f ∈ L

[0, a] may be represented by a sequence

)

n∈Z

such that

n∈Z

< +∞, i.e. an element of ℓ

Mathematicians speak of “taking the quotient by the subspace of functions which are zero

almost everywhere.”

Hilbert spaces 263

9.3.d The L

(RR

RR) space

DEFINITION 9.32 The L

(RR

RR) space is the space of measurable functions de-

ﬁned on R, with complex values (up to equality almost everywhere) which are

square integ rab le, with the norm

kf k

def

+∞

−∞



f (x)



dx.

A fundamental result due to R iesz and Fischer, which is not obvious at all,

shows that L

(R) is complete. It is therefore a Hilbert basis, and it can be

shown to admit a countable Hilbert ba sis. Thus the Hilbert spaces ℓ

, L

[0, a] ,

and L

(R) are isometric.

Remark 9.33 Altho u gh a Hilbert basis has many advantages, it will also be very interesting to

consider on the space L

(R) a “basis” which is not a Hilbert basis, but rather has elements

which are distributions, such as the (noncountable) family





x∈R

, whic h quantum physicists

denote



|x〉



x∈R

, or the family



2πi p x



p∈R

, denoted (not without some risk of confusion!)



|p〉



p∈R

. Instead of representing a function f ∈ L

by a discrete sum, it is represented by a

“continuous” sum, that is, an integral. Such a point of v iew lacks rigor if a basis “in the sense

of distributions” is not deﬁned (note, for instance, that x 7→ e

2πi p x

is not even in L

(R)!).

However, t he representation of a function by Fourier transformation is simply (in another

language) the decomposition of a function “in the basis



x 7→ e

2πi p x



p∈R

.”

 Exercise 9.1 Deﬁne H

′

to be the space of functions on R, up to equality almost everywhere,

such that ( f |f )

′

< +∞, where

( f |g)

′

def

f (t) g(t) e

−t

dt.

Then H

′

with this norm i s a Hilbert space. Show that by orth ogonalizing the family

, . . . , X

, . . .) (using the method due to Erhard Schmidt), one obtains a family of poly-

nomials (H

, . . . , H

, . . .), called the Hermite polynomials.

Show (see, for instance, [20, complement B.v]) that

(t) = (−1)

−t

The family (H

, . . . ,H

, . . .) deﬁned by H

(t) = H

(t) e

−t

is then a Hilbert basis

of L

(R) (see, for instance, [47] for a proof). It is used in quantum mechanics, in partic-

ular as a Hilbert eigenbasis of the hamiltonian of the harmonic osci ll ator; it has the interesting

property of being a family of eigenvectors for the Fourier transform.

Remark 9.34 Another Hilbert basis of L

(R) is given by th e wavelets of C

∞

class of Yves

Meyer [37,54,65], which generalize the Fourier series e xpansion on L

[0, a] (see Secti on 11.5,

page 321).

264 Hilbert spaces; Fourier series

9.4

Fourier series expansion

It is also possible (and sometimes more convenient) to deﬁne the space

[0, a] as the space of functions f : R → C which are periodic with period

a and square integrable on [0, a] (since any f unction deﬁned on [0, a] may be

extended to R by periodicity

Recall that e

(x) =

2πinx/a

9.4.a Fourier coefﬁcients of a functi on

DEFINITION 9.35 Let f ∈ L

[0, a] . For n ∈ Z, the n-th Fourier coefﬁcient

of f is the complex number

def

f (t) e

−2πint/a

dt = (e

|f ) .

The sequence of Fourier partial sums is the sequence ( f

)

n∈N

deﬁned by

(x)

def

k=−n

2πikx/a

k=−n

(x).

The Fourier coefﬁcients of the conjugate, the transpose, and th e translates

of f are g iven by the following proposition:

PROPOSITION 9.36 Let f ∈ L

[0, a], and denote by

f its conjugate, by

f its

transpose and by f

, for τ ∈ R, its translate f

(x) = f (x −τ). We have

i) ∀n ∈ Z, c

(

f ) = c

−n

( f ) ;

ii) ∀n ∈ Z, c

(

f ) = c

(

f ) = c

−n

( f ) ;

iii) ∀n ∈ Z, c

( f

) = e

−2πina/τ

( f ).

THEOREM 9.37 If f ∈ L

[0, a] is continuous and is piecewise of C

class, then

′

∈ L

[0, a] and has Fourier coefﬁcients given by

( f

′

) =

2πin

( f ) ∀n ∈ Z.

Proof. This is a simple integration by par ts.

The value at points 0 and a may not coincide, but “forgetting” this value is of no impor-

tance for functions f which are deﬁned up to equality almost e verywhere.

Fourier series expansion 265

9.4.b Mean-square convergence

Let f ∈ L

[0, a] , so that it can be seen as a function f : R → C which is

a-periodic and square integrable on [0, a]. Since L

[0, a] is a Hilbert space

and the family (e

)

n∈N

is an orthonormal Hilbert basis of this space (by

Theorem 9.31), the partial Fourier sums of f converge to f in mean-square

(Theorem 9.21):

THEOREM 9.38 (Mean-square convergence) Let f ∈ L

[0, a] . Denote by c

its Fourier coefﬁcients and by ( f

)

n∈N

the sequence of Fourier partial sums of f . Then

the sequence ( f

)

n∈N

converges in mean-square to f :



f − f





f (t) − f

(t)



dt −−−→

n→∞

DEFINITION 9.39 It is customary to write (abusing notation, since the equality

holds only in the sense of the L

-norm, and not pointwise) simply that

f (t) =

+∞

n=−∞

2πint/a

+∞

n=−∞

(t) (9.3)

and to call this expression the Fourie r series expansion of f .

Remark 9.40 It is important to fully understand why the identity

f =

+∞

n=−∞

true

does not allow us to write

f (t) =

+∞

n=−∞

2πint/a

. false!

The ﬁrst equality is proved in the general case of Hil bert spaces. But t h e Hilbert space which

we use is the space L

[0, a], where fu nct ions are only deﬁned u p to almost-everywhere equality,

and where co nvergence means convergence in mean-square. The space of a-peri odic functions,

with the norm deﬁned as the supremum of the absolute values of a function, is not complete.

One cannot hope for a poi ntwise convergence result purely in the setting of Hilbert spaces.

(This type of convergence will be considered with other tools in Section 9.4.d on page 267.)

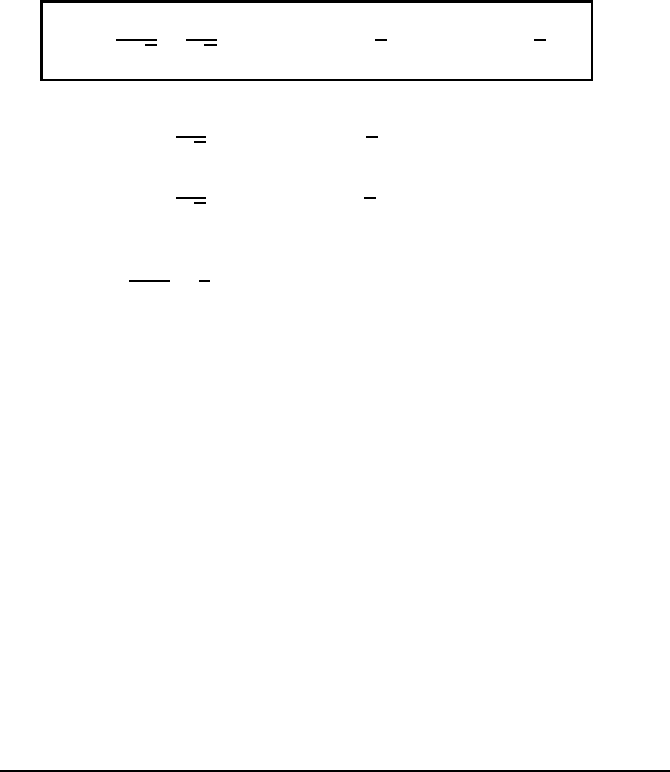
The Parseval identity is then written as follows:

THEOREM 9.41 (Parseval identity) Let f ∈ L

[0, a] and let c

denote its Fourier

coefﬁcients. Then the family (|c

)

n∈Z

is summable and we have

n∈Z



f (t)



dt =



Moreover, if f, g ∈ L

[0, a] , then ( f g) ∈ L

[0, a] and we have

n∈Z

( f ) c

(g) =

f (t) g(t) dt = ( f |g) .

266 Hilbert spaces; Fourier series

In other words, the representation as Fourier series is an isometry between the

space L

[0, a] and the space ℓ

. Sometimes the following “real” notation is

used (with the same abuse of nota tion previously mentioned):

f (t) =

+∞

n=1



cos



2πn



+ b

sin



2πn



, (9.4)

with a

def

f (t) cos



2πn



dt = c

+ c

−n

def

f (t) sin



2πn



dt = i(c

− c

−n

and

+∞

n=−∞

n6=0



+ |b





f (t)



dt.

Remark 9.42 The Fourier series expansion g ives a concrete isometry between the space L

[0, a]

and th e spac e ℓ

. To each square-integ rable function, we can associate a sequence (c

)

n∈Z

∈ ℓ

namely, the sequence of its Fourier coefﬁcie nts. Conversely, given an arbitrary sequence

)

n∈Z

∈ ℓ

, the trigonometric series

converges (in the sense of mean-square conver-

gence) to a function which is square integrable (this is the Riesz-Fischer Theorem). Frédéric

Riesz said that the Fourier se ries expansion was “a perpetual round-trip ticket between two

spaces of inﬁnite dimension” [52].

9.4.c Fourier series of a function f ∈ L

[0, a]

This bus iness of convergence in L

norm is all well and good, but it d oes not

even imply pointwise convergence (and, a fort iori, does not imply uniform

convergence

). I sn’t it possible to do better? Can we write (9.3) or (9.4)

rigorously?

We start the discussion of this question by extending the notion of Fourier

series to functions which are not necessarily in L

[0, a] , but are in the larger

space L

[0, a] of Lebesgue-integrable functions on [0, a].

Indeed, for f ∈

To take an example independent of Fourier series, the sequence (x 7→ sin

n∈N

converges

to 0 in mean-square, but it does not converge pointwise or uniformly.

Notice that, on the contrary, if f ∈ L

[0, a], then f ∈ L

[0, a] since, by the Cauchy-

Schwarz inequality we have

Z



f (t)







|f |, 1





f (t)



dt ·

1 dt =



×a.