Appel W. Mathematics for Physics and Physicists
Подождите немного. Документ загружается.


Chapter
10
Fourier transform
of functions
This chapter introduces an integral transform called the “Fourier transform,” which
generalizes the Fourier analysis of periodic functions to the case of f u nct ions defined
on the whole real axis R.
We start with the definition of the Fourier transform for functions which are
Lebesgue-integrable (elements of L
1
). One problem of the Fourier transform thus
defined is that it does not leave t h e space L
1
stable. It will then be e xtended
to functions which are square integrable (elements of L
2
), w h ich have a physical
interpretation in terms of energy. The space L
2
being stable, any square integrable
function has a Fourier transform which is also square integrable.
10.1
Fourier transform of a function in L
1
In this section, we start by defi ning the Fourier transform of a n integrable
function and deriving its main properties.
Although the Fourier transform is a generalization of the notion of a
Fourier series , it is not the L
2
or Hilbert space setting which is the simplest.
Therefore we start with functions in L
1
(R),
1
before generalizing to square
integrable functions.
1
The reason is that, in contrast with functions defined on a finite interval, L
2
(R) is not
contained in L
1
(R).

278 Fourier transform of functions
10.1.a Definiti on
DEFINITION 10.1 Let f be a f unction, real- or complex-valued, depending on
one real variable. The Fourier transform (or spectrum) of f , if it exists, is the
complex-valued function defined for the real variable ν by
e
f (ν)
def
=
Z
∞
−∞
f (x) e
−2πiν x
dx for all ν ∈ R. (10.1)
This is denoted symbolically
e
f = F [ f ] or
e
f (ν) = F
f (x)
.
Similarly, the conjugate Fourier transform of a function f is given (when it
exists) by
F [ f ] (ν)
def
=
Z
∞
−∞
f (x) e
2πiν x
dx.
Remark 10.2 The integral that ap pears does not always exist. For instance, if we consider
f : x 7→ x
2
, the integral
Z
+∞
−∞
x
2
e
−2πiν x
dx
does not exist, for any value of ν, and f does not have a Fourier transform (in the sense of
functions; i t will be seen in the next chapter how to define i ts Fourier transform in the sense
of distributions).
It is also possible to have to deal with non-integrable functions for which the i ntegral
defining
e
f (ν) converges as an improper integral. As an example, consider g : x 7→ (sin x)/x.
Then g is not integrable but, on the other hand, the limit
lim
M→+∞
Z
M
−M
sin x
x
e
−2πiν x
dx
exists for all values of ν. More details about this (not a complete description of what can
happen! this is very difficult to obtain and state) will be gi ven in Section 10.3, which discusses
the extension of the Fourier transform to the space of square integrable functions.
At least if f is integrable on R, it is clear that
e
f is defined on R. Indeed, recall that for a
function f : R → R, the following equivalence holds:
f is Lebesgue-integrable ⇐⇒ |f | is Lebesgue-integrable,
which shows that if f is integrable, then so is x 7→ f (x) e
−2πiν x
for any real value of ν.
THEOREM 10.3 (Fourier transform of an integrable function) Let f be a func-
tion which is Lebesgue-integra ble on R. Then the Fourier transform ν 7→
e
f (ν) of f is
defined for all ν ∈ R.
Remark 10.4 A number of different conventions regarding the Fourier transform are in use in
various fields of physi cs and mathematics; in the table on page 612, the most common ones
are presented with the corresponding formula for the inverse Fourier transform.

Fourier transform of a function in L
1
279
10.1.b Examples
Example 10.5 Consider the “rectangle” function (already considered in Chapter 7):
Π(x)
def
=
(
0 for |x| >
1
2
,
1 for |x| <
1
2
.
Then its Fourier transform is a sine cardinal (or cardinal sine):
e
Π(ν) =
Z
1
2
−
1
2
e
−2πiν t
dt = sinc(πν)
def
=
sin πν
πν
if ν 6= 0,
1 if ν = 0.
Similarly, the Fourier transform of the characteristic function χ
[a,b ]
of an interval is
eχ
[a,b ]
(ν) = F
χ
[a,b ]
(ν) =
sin
π(b −a)ν
πν
e
−iπ(a+b)ν
if ν 6= 0,
b −a if ν = 0.
Example 10.6 Consider the gaussian x 7→ e
−πx
2
. Then one can show (see Exercice 10.1 o n
page 295 or, usi ng the method of residues, Exercise 4.13 on page 126) that
F
e
−πx
2
= e
−πν
2
.
Example 10.7 The lorentzian function with parameter a > 0 is defined by
f (x) =
2a
a
2
+ 4π
2
x
2
.
Then, by the method of residues, one can show that
F
2a
a
2
+ 4π
2
x
2
= e
−a|ν|
.
The table on page 614 lists some of the most useful Fourier transforms.
10.1.c The L
1
space
Let L
1
(R) denote temporarily the s pace of functions on R which are Lebesgue-
integrable. Can it be normed by putting
kf k
def
=
Z
f (t)
dt ?
The answer is “no.” The reason is similar to what happened in the d efinition
of the space L
2
in the prev ious cha pter: if f and g are two functions in L
1
which are equal almost everywhere,
2
then kf − gk = 0, although f 6= g;
this means that the map f 7→ kf k is not a norm. To resolve this d ifficulty,
2
For instance, one can take f = χ
Q
, the characteristic function of the set Q, also called the
Dirichlet function, and g the zero function.
280 Fourier transform of functions
those functions which are equal almost everywhere are identified. The set of
all functions which are equal to f almost everywhere is the equivalence class
of f. The set of equivalence classes of integrable functions is denoted L
1
(R)
or more simply L
1
. Thus, when speaking of a “function” f ∈ L
1
, what is
meant is in fact any function in the same equivalence class. For instance, the
zero function and the D irichlet function belong to the same equivalence class,
denoted 0. In order to not complicate matters, there are both said to be “ equal
to the zero function.”
In the space L
1
, the map f 7→
R
|f | is indeed a norm.
DEFINITION 10.8 The space L
1
(RR
R
R
RR) or L
1
is the vector space of integrable
functions, defined “up to equality a lmost everywhere,” with th e norm
kf k
1
def
=
Z
f (t)
dt.
Consider two functions f and g which coincide a lmost everywhere. Then
R
f =
R
g. Moreover, if ϕ is any other function, it follows that f ϕ is equal to
gϕ almost everywhere. So applying this result to the case of ϕ : x 7→ e
−2πiν x
,
it follows that two functions which are equal almost everywhere ha ve the same Fourier
transform.
Hence, we can consider that the Fourier transform is defined on the
space L
1
(R) of integrable functions defi ned up to equality almost everywhere.
10.1.d Elementary properties
THEOREM 10.9 (Properties of
e
f ) Let f ∈ L
1
(R) be an integrable function and
denote by
e
f its Fourier transform. Then
e
f is a continuous function on R. Moreover,
it is bounded, and in fact k
e
f k
∞
¶ kf k
1
.
DEFINITION 10.10 The space L
∞
(RR
R
R
RR) is the vector space of bounded measur-
able functions on R, with t he sup norm defined by
kf k
∞
def
= sup
x∈R
f (x)
.
(It is possible to define functions of L
∞
up to equality almost everywhere. Th e
definition of the norm must then b e modified accordingly. This refinement
is not essential here).
THEOREM 10.11 (Continuity of the Fourier transform in L
1
) The Fourier trans-
form, defined on L
1
(R) and taking values in L
∞
(R), is a continuous linear oper-
ator, that is
i) (linearity) for any f, g ∈ L
1
(R) and any λ, µ ∈ C, we have
F [λ f + µg] = λF [ f ] + µF [g] ;
Fourier transform of a function in L
1
281
ii) (continuity) if a sequence ( f
n
)
n∈N
of integrable functions converges to 0 in the
sense of the L
1
norm, then the sequence (
e
f
n
)
n∈N
tends to 0 in the sense of the
sup norm:
Z
f
n
(x)
dx −−−→
n→∞
0
=⇒
sup
ν∈R
e
f
n
(ν)
−−−→
n→∞
0
.
Proof of the two prec eding theorems. To show the continuity of the function
e
f ,
we apply the theorem of continuity of an integral depending on a parameter (Theo-
rem 3.11 on page 77), using the domination relation
∀x ∈ R
f (x) e
−2πiν x
¶
f (x)
.
Moreover, for any ν ∈ R, we have
e
f (ν)
=
Z
f (x) e
−2πiν x
dx
¶
Z
f (x)
dx = kf k
1
,
which shows that
k
e
f k
∞
= sup
ν∈R
e
f (ν)
¶ kf k
1
.
Since t he Fourier transform is obviously linear, this inequality is sufficient to establish
its continuity (see Theorem A.51 on page 583 concerning continuity of a linear map).
The continuity of the Fourier transform is an important fact. It means
that if a sequence ( f
n
)
n∈N
of integrable functions converges to f ∈ L
1
, in the
sense of the L
1
norm, namely,
Z
f
n
(t) − f (t)
dt −−−→
n→∞
0,
then there will b e convergence in the sense of the L
∞
norm, that is, uniform
convergence of the sequence (
e
f
n
)
n∈N
:
f
n
L
1
−→ f
=⇒
e
f
n
cv.u.
−−→
e
f on R
.
Example 10.5 on page 279 shows that the Fourier transform of a function
in L
1
is not necessarily integrable it self. So the “Fourier transform” operator
does not preserve the space L
1
, which is somewhat inconvenient, as one often
prefers an honest endomorphism of a vector space to an arbitrary linear map.
(It will be seen in Section 10.3 th at it is possible to extend the Fourier trans-
form to the space L
2
and that L
2
is then stable by t he Fourier transform th us
defined.)
On the other hand, one can see that each of th e Fourier transforms previ-
ously computed has the property that it tends to 0 at infinity. This property
is confirmed in general by the following theorem, which we will not prove
(see, e.g., [76]). It is the analogue of the Riemann-Lebes gue Lemma 9.44 for
Fourier series.

282 Fourier transform of functions
THEOREM 10.12 (Riemann-Lebesgue lemma) Let f ∈ L
1
(R) be an integrable
function and denote by
e
f its Fourier transform. Then the continuous function
e
f tends
to 0 at infinity :
lim
ν→±∞
e
f (ν)
= 0.
10.1.e Inversion
The question we now ask is: can one inverse the Fourier transform? The
answer is partially “yes”; moreover, the inverse t ransform is none other than
the conjugate Fourier transform.
We just saw that, even if f ∈ L
1
(R), its Fourier transform
e
f is not itself
always integrable, and so the conjugate Fourier transform of
e
f may not be
defined. However, in the special case where
e
f ∈ L
1
, we have the following
fundamental result.
THEOREM 10.13 (Inversion in L
1
(RR
R
R
RR)) Let f ∈ L
1
(R) be an integrable function
such that the Fourier transform
e
f of f is itself integrable. Then we have
F [
e
f ] (x) = f (x) for almost all x;
and also
F [
e
f ] (x) = f (x) at any point x where f is continuous.
In particular, if f is also continuous,
3
we have F [
e
f ] = f .
Proof. We prove the inversion formula only at points of continuity. The proof starts
with the following lemma.
LEMMA 10.14 Let f and g be two integrable functions. Then
e
f · g and f · eg are integrable
and we have
Z
f (t) eg(t) dt =
Z
e
f (t) g(t) dt.
Proof of the lemma. Since
e
f is bounded and g is integrable, the product
e
f · g is also integrable and similarly for f · eg. Hence by Fubini’s theorem, we
have
Z
f (t) eg(t) dt =
Z
f (t)
Z
g(s) e
−2πi ts
ds
dt
=
Z
g(s)
Z
f (t) e
−2πi st
dt
ds =
Z
g(s)
e
f (s) ds,
which proves the lemma.
3
Then we are not looking at it simply up to “equality almost everywhere.”
Fourier transform of a function in L
1
283
Now introduce the sequence of functio ns (h
n
)
n∈N
defined by
h
n
(x) = exp(−π
2
x
2
/n
2
).
It is a sequence of gaussians which are more and more “spread out,” and converges
pointwise to the constant function 1. Since
e
f is integrable and
e
f (x) h
n
(x) e
2πi xt
¶
e
f (x)
for any reals x, t ∈ R and any n, Lebesgue’s dominated convergence theorem p roves
that
Z
e
f (x) h
n
(x) e
2πi xt
dx −−→
n→∞
Z
e
f (x) e
2πi xt
dx = F
e
f
(t) (10.2)
for any t ∈ R. The Fourier transform of x 7→ h
n
(x) e
2πi xt
is, as shown by an immediate
calculation, equal to x 7→
e
h
n
(x − t), and the previous lemma yields
Z
e
f (x) h
n
(x) e
2πi xt
dx =
Z
f (x)
e
h
n
(x − t) dx. (10.3)
But, as seen in Example 8.17 on p age 231, the sequence of Fourier transforms of h
n
is
e
h
n
: x 7−→
n
p
π
e
−n
2
x
2
,
which is a Dirac sequence. It follows that, if t is a point of continuity for f , we have
Z
f (x)
e
h
n
(x − t) dx −−→
n→∞
f (t). (10.4)
Putting equations (10.2), (10.3), and (10.4) together, we obtain, for any point where f is
continuous, that
Z
e
f (x) e
2πi xt
dx = F
e
f
(t) = f (t),
which is the second result stated.
COROLLARY 10.1 5 If f is integrable and is not equal almost everywhere to a con-
tinuous function, then its Fourier transform is not integrable.
Proof. If the Fourier transform
e
f is integrable, then f coincides al most everywhere
with the inverse Fourier transform of
e
f , which is continuous.
Remark 10.16 With the conventions we have chosen for the Fourier transform, we have, when
this makes sense, F
−1
= F . One could use indifferently F
−1
or F . The reader should be
aware th at, in other fields, the convention use d for the definition of the Fourier transform is
different from the one we use (for instance, in quantum mechanics, in optics, or when dealing
with functions of more than one variable), and the notation is not equivalent then. A table
summarizes the main conventions used and the corresponding inversion formulas (page 612).
Remark 10.17 Theorem 10.13 may be proved more simply using Lemma 10.14 and the fact
that the Fourier transform of the constant function 1 is the Dirac δ. But this property will
only be established in the next chapter. For this reason we use instead a sequence of functions
(h
n
)
n∈N
converging to 1, while the sequence of their Fourier transforms converges to δ.
To apply the inversion formula of Theorem 10.13, one needs some infor-
mation concerning not only f , but also
e
f (which must be integrable); this may
be inconvenient. In the next proposition, information relative to f suffices
to ob tain the inversion formula.
284 Fourier transform of functions
PROPOSITION 10.18 Let f be a function of C
2
class such that f , f
′
, and f
′′
are
all integrable. Th en
e
f is also integrable; the Fourier inversion formula holds, and we
have
f = F [
e
f ] .
Example 10.19 Consider again the example of the lorentzian function. We have
f (x) =
2
1 + 4π
2
x
2
.
Then
f
′
(x) =
−16 π
2
x
(1 + 4π
2
x
2
)
2
and f
′′
(x) =
192 π
4
x
2
−16 π
2
x
2
(1 + 4π
2
x
2
)
3
,
which are both integrable. We deduce from this that F
e
−|ν|
= 2/(1 + 4π
2
x
2
). Moreover,
since all t h e functions considered are real-valued, it follows by taking the complex conjugate
and exchanging the variables x and ν that also
F
e
−|x|
=
2
1 + 4π
2
ν
2
.
Hence the inversion formula is also a tool that can be used to compute
new Fourier transforms easily!
10.1.f Exten sion of the inversion formula
We can now discuss the case where f has a Fourier transform
e
f which is not
integrable, that is, such that
Z
e
f (ν) e
2πiν x
dx
is not defined in the sense of Lebesgue. In s ome situations, the limit
lim
R→+∞
Z
+R
−R
e
f (ν) e
2πiν x
dν
may still be defined. (It is not quite an improper integral, because the bounds
of integration are symmetrical.) For example, the Fourier transform of the
“rectang le” function is a cardinal sine. The latter is not Lebesgue-integrable,
but the integral
I(x, R)
def
=
Z
R
−R
sin πν
πν
e
2πiν x
dν
does have a limit as [R → ∞]. In such cas es, the following theorem may be
used.
Properties of the Fourier transform 285
THEOREM 10.20 Let f (x) be an integrable function of class C
1
except at finitely
many points of discontinuity, such that f
′
∈ L
1
. Then for all x we have
lim
R→+∞
Z
+R
−R
e
f (ν) e
2πiν x
dν =
1
2
h
f (x
+
) + f (x
−
)
i
.
By abuse of notation, this limit will still be denoted F
e
f
(x).
In other words, the inversion formula, in the sense of a limit of integrals
(called the principal value), gives the regularized function associated to f .
This should be compared to Dirichlet’s theorem on page 268.
In particular, if f is continuous and f and f
′
are integrable, the inversion
formula of Theorem 10.20 gives back the original function f .
Example 10.21 We saw that the Fourier transform of the “rectangle” function is si nc(πν) .
Since the “rectangle” funct ion has only two discontinuities and since its derivative (in the
sense of functions) is always zero and therefore integrable, it follows that
lim
R→+∞
Z
+R
−R
sinc(πν) e
2πiν x
dν = F
sinc(πν)
(x) =
0 i f |x| >
1
2
,
1 i f |x| <
1
2
,
1
2
if |x| =
1
2
.
10.2
Properties of the Fourier transform
10.2.a Transpose and translates
THEOREM 10.22 Let f be an integrable function and a ∈ R a real number. We
have
F
f (x)
=
e
f (−ν), F
f (−x)
= F
f (x)
=
e
f (−ν),
F
f (x −a)
= e
−2iπνa
e
f (ν), F
f (x) e
2πiν
0
x
=
e
f (ν −ν
0
).
These results should be compared to the formulas for similar operations on the
Fourier coefficients of the periodic f unction; see Theorem 9.36 on page 264.
COROLLARY 10.2 3 In the following table, the properties of f indicated in the first
column are transformed into the properties of
e
f in the second column, and conversely:
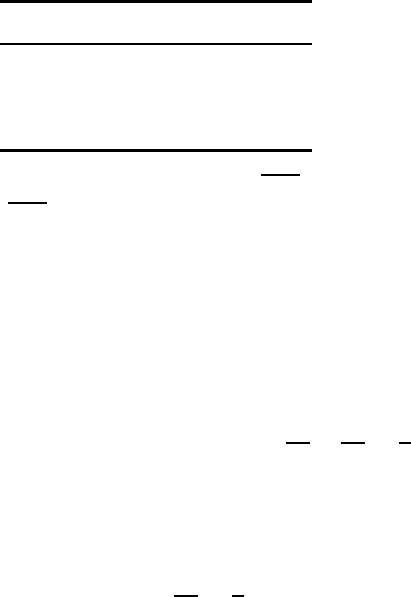
286 Fourier transform of functions
f (x)
e
f (ν)
even even
odd odd
real-valued hermitian
purely imaginary antihermitian
Here a f unction g is a hermitian func tion if g(−x) = g(x) and an antihermitian
function if g(−x) = −g(x).
10.2.b D ilation
If a dilation is performed (also called a change of scale), that is, if we put
g(x) = f (ax) ∀x ∈ R, for some a ∈ R
∗
,
the Fourier transform of g can be computed easily:
eg(ν) =
Z
f ( ax) e
−2πiν x
dx =
Z
f ( y) e
−2πiν y/a
dy
|a|
=
1
|a|
e
f
ν
a
,
which gives the following theorem.
THEOREM 10.24 Let a ∈ R
∗
and f ∈ L
1
. Then we have
F
f ( ax)
=
1
|a|
e
f
ν
a
.
In other words, a dilat ion in the real world corresponds to a compression
in the Fourier world, and conversely.
10.2.c D erivation
There is a very important relation between the Fourier transform and the
operation of differentiation:
THEOREM 10.25 (Fourier transform of the derivative) Let f ∈ L
1
be a func-
tion which decays sufficiently fast at infinity so that x 7→ x
k
f (x) belongs also to L
1
for k = 0, . . ., n. Then
e
f can be differentiated n times and w e have
F
(−2iπx)
k
f (x)
=
e
f
(k)
(ν) for k = 1, . . . , n.
Conversely, if f ∈ L
1
and if f is of C
n
class and, moreover, its successive
derivatives f
(k)
are integrable for k = 1, . . ., n, then we have
F
f
(m)
(x)
= (2iπν)
m
e
f (ν) for k = 1, . . ., n.
