Appel W. Mathematics for Physics and Physicists
Подождите немного. Документ загружается.


Physical interpretation of convolution operators 217
(if this expression makes sense), where krk denotes, with a slight abuse of
notation, the f unction r 7→ krk. I t is then easy to compute, in the sense of
distributions, the laplacian of the potential. Indeed, it suffices to compute th e
laplacian of one of the two factors in this convolution. Since we know that
△(1/r) = −4πδ, we obtain
△V =
1
4πǫ
0
△
ρ ∗
1
krk
=
1
4πǫ
0
ρ ∗△
1
krk
=
1
4πǫ
0
ρ ∗(−4πδ)
= −
ρ
ǫ
0
.
Thus we have recovered a classical result:
THEOREM 7.77 (Poisson equation) The la placian of the electrostatic potential cre-
ated by a distribution of charge ρ is
△V = −
ρ
ǫ
0
.
7.7
Physical interpretation of convolution operators
We are going to see now t hat many physical systems, in particular mea-
suring equipment can be represented by convolution operators. Suppose we
have given a physical system which, when excited by a n input signal E de-
pending on time, produces an output signal, denoted S = O (E). We make
the assumptions that the operator O that transforms E into S is
• linear;
• continuous;
• invariant under translations, that is, the following diag ram commutes
(this means that composing the arrows from the upper left corner to
the bottom right corner in the two possible ways has the same result):
E(t)
O
−−−→ S(t)
translation
y
y
translation
E(t − a)
O
−−−→ S (t −a)

218 Distributions I
THEOREM 7.78 If the three conditions stated above h old, then the operator O is a
convolution operator, that is, there exists R ∈ D
′
such that
S = O(E) = E ∗R = R ∗ E
for any E.
The converse is of course true.
Remark 7.79 The case of an excitation depe nding on time is very simple. If, on the other
hand, we consider an optical system where the source is an exterior object and where the
output is measured on a photo-detector, the variable corresponding to the measurement (the
coordinates, in centimeters, on the photographic plate) and the variable corresponding to the
source (for instance, th e angular co ordinates of a celestial object) are diffent. One must then
perform a change of variable to be able to write a convolution relation between the input and
output signals.
Knowing the distribution R makes it possib le to predict the response of
the system to an arbitary excitation. The distribution R is called the impulse
response because it corresponds to the output to an elementary δ input:
δ
O
−→ O(δ) = δ ∗ R = R.
Note that often, in physics, the operator linking S (t) and E(t) is a differ-
ential operator.
13
One can then write D ∗ S = E where D is a distribut ion
which is a combination of derivatives of the unit δ.
14
The distribution R,
being the output corresponding to a Dirac peak, therefore satisfies
R ∗ D (t) = D ∗ R (t) = δ(t)
|{z}
E(t)
.
Since δ is the neutral element for the convolution, the distribution R is
thus the inverse of D for the convolution product. This point of view is
developed in Section 8.4 on page 238. I n general, this operator D is given
by physical considerations; we will describe below the technique of Green
functions (Chapter 15), where the purely technical difficulty is to compute, if
it exists, the convolution inverse of D.
Remark 7.80 Experimentally, it is not always easy to send a Dirac function as input. It is
doable in optics (use a star), but rather delicate in electricity. It is sometimes simpler to send
a signal close to a Heaviside function. The response to such an excitation, which is called the
step response, is then S = H ∗R. If we differentiate this, we obtain S
′
= H
′
∗R = δ ∗R = R,
which shows the following result:
THEOREM 7.81 The impulse response is the derivative of the step response.
13
Consider, for instance, a particle with mass m and position x(t) on the real axis, subject
to a force depending only of time F (t). Take, for example, the excitation E(t) =
1
m
F (t) and
the response S(t) = x(t). The evolution equation of the system is S
′′
(t) = E(t).
14
In the previous example, one can t ake D = δ
′′
, since δ
′′
∗ S = S
′′
.
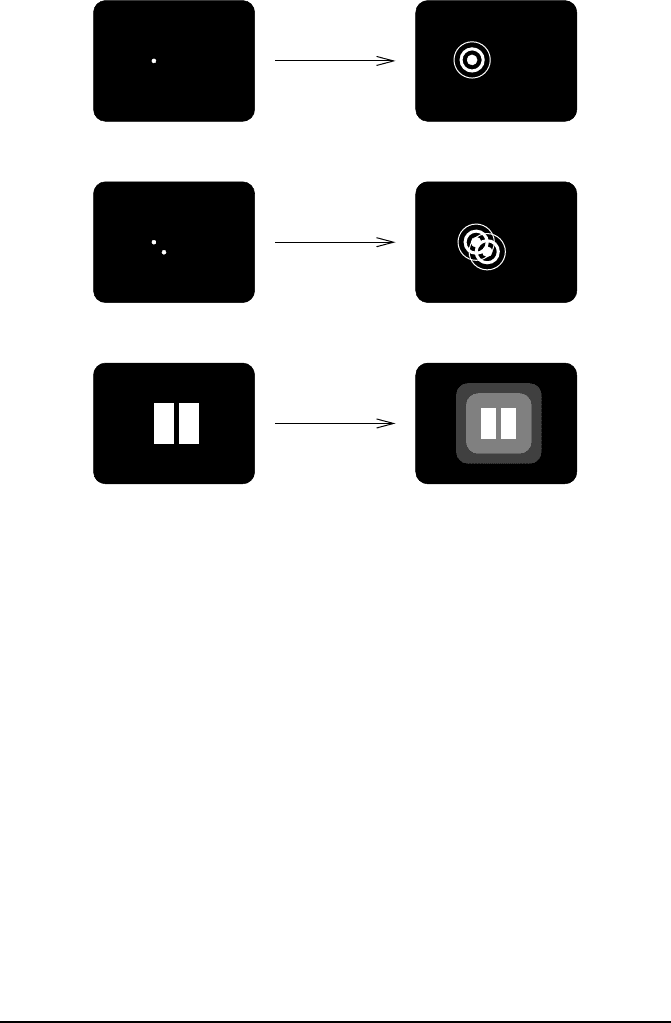
Physical interpretation of convolution operators 219
A window
Two stars
through the telescope
A star
Fig. 7.3 — Some objects to look at with a telescope.
If T is sufficiently smooth, this differentiation can be performed numerically; however, differ-
entiation is often hard to implement numerically. This is the price to pay.
DEFINITION 7.82 (Causal system) A system represented by a temporal con-
volution operator (i. e. , by a t ime variable) is called a causal system if its
impulse response R(t) is zero on R
∗−
(which means that 〈R, ϕ〉 = 0 for any
test f unction ϕ which is zero for t ¾ 0).
In a causal system, the effect of a signal cannot come before its cause
(which is usually expected in physics).
Example 7.83 (in optics) Look at the sky with a telescop e. Each star is a point-like li ght
source, modeled by a Dirac δ. Represent the light intensity of a star centered in the telescope
by a distribution aδ; then the image of this star after passing through the telescope is given
by a function of the intensity aψ(x, y), showing the diffraction spot due to the finite size of
the mirror and to the Newton rings.
15
Any incoherent luminous object (which is indispensable
to ensure linearity with respect to th e light intensity functions), with intensity function i n the
coordinates (x, y) equal to F (x, y), will give an image F (x, y) ∗ψ(x, y) (see Figure 7.3).
15
The function ψ is studied in Chapter 11, page 320.

220 Distributions I
7.8
Discrete convolution
It should be noted that it is quite possible to define a discrete convolution
to model the “fuzziness” of a d igitalized picture. Such a picture is given no
longer by a continuous function, but by a sequence of values n 7→ x
n
with n ∈
[[1, N ]] (in one dimension) or (m, n) 7→ x
m n
with (m, n ) ∈ [[1, N ]] × [[1, M]]
(in two dimensions). Each of these discrete numerical values defines a pixel.
In t he one-dimensional case, we are given values α
n
for n ∈ [[1, N ]] (and
we put α
n
= 0 outside of this interval), and the convolution is defined by
y = α ∗ x with y
n
=
+∞
X
k=−∞
α
k
x
n−k
,
the support of y being then equal to [[1 − M , N + M ]]. In two dimensions,
the corresponding formula is
y = α ∗ x with y
m n
=
+∞
X
k=−∞
+∞
X
ℓ=−∞
α
kℓ
x
m −k,n−ℓ
.
Photo-processing softwares (such as Photoshop, or the GIMP, w hich is Free —
as in Free Speech — software) provide such convolutions. An example of the
result is given in Figure 7.4 on the facing page.
The dis crete convolution may be written as a matrix operation, with the
advantages and inconveniences of such a representation. Th e inversion of this
operation is done with the inverse matrix when it exists. We refer the reader
desirous to learn more to a book about signal t heory.
—
The exercises for this chapter are found at the end of Chapter 8, page 241.

Discrete c onvolution 221
Fig. 7.4 — A square in Prague, before and after discrete convolution.
Laurent Schwartz, born in Paris in 1915, son of the first Jewish surgeon in the
Paris hospitals, grandson of a rabbi, was a student at the École Normale Supérieure.
During World War II, he went to Toulouse and then to Clermont-Ferrand, before
fleeing to Grenoble in 1943. I n 1944, he barely escaped a German raid. After the war,
he taught at th e University of Nancy, where the main part of the Bourbaki group
was located. He came back to Paris in 1953 and obtained a position at the École
Polytechnique in 1959.
He worked in partic u lar in functional analysis (under the influcence of
Dieudonné) and, in 1944, created the theory of distributions. Because of th ese
tremendously important works, he was awarded the Fields M edal in 1 950. He showed
how to use this theory in a physical context.
Finally, one cannot omit, side by side with the mathematician, the political mili-
tant, the pacifist, who raised his voice against the crimes of the French state during
the Algerian war and fought for the independence of Algeria [82].
Laurent Schwartz passed away on July 4, 2002.
Chapter
8
Distributions II
In this chapter, we will first discuss in det ail a particular distribution which is very
useful in physics: the “Cauchy principal value” distribution. Notably, we will derive
the famous formula
1
x ± iǫ
= pv
1
x
∓iπδ,
which appears in optics, statistical mechanics, and quantum mechanics, as well as
in field theory. We will also treat the topology on t h e space of distributio ns and
introduce the notion of convolution algebra, which will lead us to t h e notion of
Green function. Finally, we will show how to solve in one stroke a differential
equation wi th the consideration of initial conditions for the solution.
8.1
Cauchy principal value
8.1.a Definition
The function x 7→
1
x
does not defi ne a regular distribution, since it is not
integrable in the neighborhood of x = 0. On the other hand, one can define,
for ϕ ∈ D(R), the limit
pv
Z
+∞
−∞
ϕ(x)
x
dx
def
= lim
ǫ→0
+
Z
|x|¾ǫ
ϕ(x)
x
dx
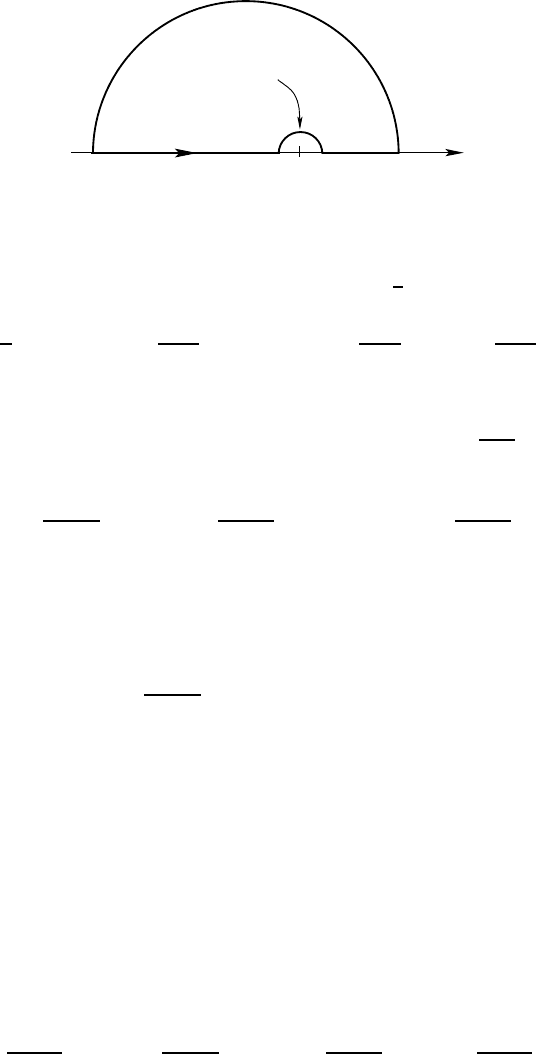
224 Distributions II
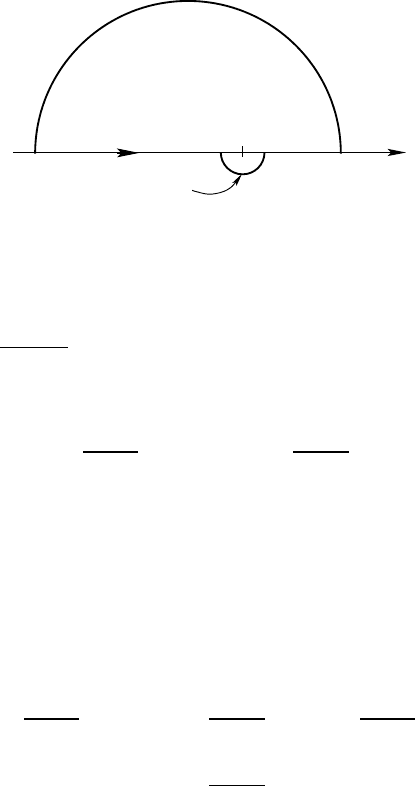
x
0
C
+
(ǫ)
Fig. 8.1 — The contour γ
+
.
which provides the definition of the distribution pv
1
x
, given by
pv
1
x
, ϕ
·
= pv
Z
+∞
−∞
ϕ(x)
x
dx
def
= lim
ǫ→0
+
Z
−ǫ
−∞
ϕ(x)
x
dx +
Z
∞
ǫ
ϕ(x)
x
dx
.
This is then generalized as follows:
DEFINITION 8.1 Let x
0
∈ R. T he Cauchy principal value pv
1
x−x
0
is defined
as the distribution on R given by
pv
1
x − x
0
, ϕ
= pv
Z
ϕ(x)
x − x
0
dx
def
= lim
ǫ→0
+
Z
|
x−x
0
|>ǫ
ϕ(x)
x − x
0
dx.
8.1.b Application to the c omputation of certain integrals
The situation that we are considering is the computation of an integral of the
type
Z
+∞
−∞
f (x)
x − x
0
dx, with f : R −→ C.
This integral is not correctly d efined without additional precision; indeed, if
f (x
0
) 6= 0, the function x 7→ f (x)/(x − x
0
) is not Lebesg ue integrable. One
possibility is to define the integral in the sense of the principal value.
We will assume that f is the restriction to the real axis of a function
meromorphic on C and holomorphic at x
0
. Moreover, we will assume that f
decays sufficiently fast at infinity to permit t he use of the method of residues.
For insta nce, we will require that this decay holds at least in the upper half-
plane.
Now define a contour γ
+
as in Figure 8.1: denote by C
+
(ǫ) the small h alf-
circle with radius ǫ centered at x
0
and above the real axis. Letting the radius
of the large circle go to infinity (and assuming the corresponding integral goes
to 0), we have then
Z
γ
+
f (z)
z − x
0
dz =
Z
−ǫ
−∞
f (x)
x − x
0
dx +
Z
+∞
ǫ
f (x)
x − x
0
dx +
Z
C
+
f (z)
z − x
0
dz ;
Cauchy principal value 225
x
0
C
−
(ǫ)
Fig. 8.2 — The contour γ
−
.
but the last integral is equal to
Z
0
π
f (ǫ e
iθ
)
ǫ e
iθ
iǫ e
iθ
dθ = −iπ f (x
0
) + O(ǫ) [ǫ → 0
+
].
Thus, taking the limit [ǫ → 0
+
], we obtain
2iπ
X
p∈H
Res
f (z)
z − x
0
; p
= pv
Z
+∞
−∞
f (x)
x − x
0
dx −iπ f (x
0
), (8.1)
where the sum
P
p∈H
is the sum over the poles of f located in the open
upper half-plane H
def
= {z ∈ C ; Im(z) > 0}.
So, to deal with the integral on a contour on which a pole is located,
it was necessary to deform this contour slightly — in this case, by making a
small detour around the pole to avoid it. One may wonder what would have
happened, had another detour been chos en. So take the contour γ
−
defined
as in Figure 8.2. We then have
Z
γ
−
f (z)
z − x
0
dz = pv
Z
+∞
−∞
f (x)
x − x
0
dx +
Z
C
−
f (z)
z − x
0
dz
= pv
Z
+∞
−∞
f (x)
x − x
0
dx + iπ f (x
0
).
Since the integral on the left-hand side is equal to 2πi times the sum of the
residues located in t he upper half- plane, plus th e residue at x
0
, wh ich is equal
to 2πi f (x
0
), the final formula is the sa me as (8.1).
8.1.c Feynman’s notation
When evaluating an integral on a contour that passes through a pole, it must
be specified on w hich side the pole the pole will be avoided (the result depends
only on the side that is chosen, not on the precise shape of the detour).
Rather than going around the pole, R ichard Feynman proposed modify-
ing the initial problem by moving the pole slightly off the real axis while
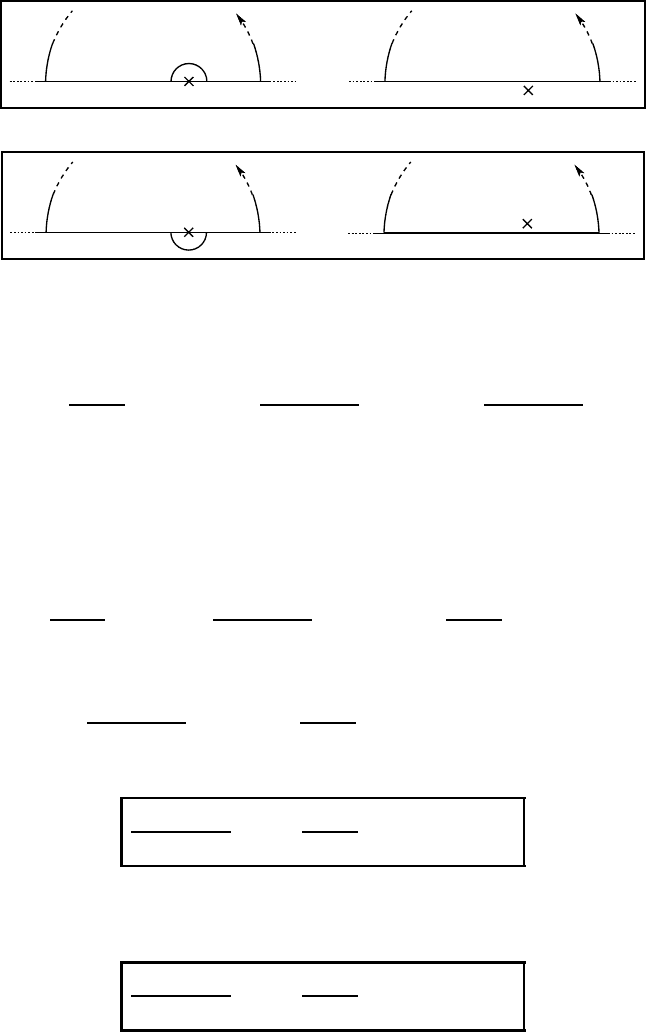
226 Distributions II
keeping the original contour intact. Suppose the function considered has a
pole on the real axis. Then one can write the symbolic equivalences
⇐⇒
and
⇐⇒
which means that the pole has been moved in the first case to x
0
−iǫ and in
the second case to x
0
+ iǫ, and then the limit [ǫ → 0
+
] is taken at the end of
the computa tions.
To see clearly what this means, consider the equalities
Z
γ
+
f (z)
z − x
0
dz = lim
ǫ→0
+
Z
γ
+
f (z)
z − x
0
+ iǫ
dz = lim
ǫ→0
+
Z
γ
f (z)
z − x
0
+ iǫ
dz,
where γ is the undeformed contour. Indeed, the first equality is a consequence
of continuity under the integral sign, and the second equality comes from
Cauchy’s theorem, which, for any given ǫ > 0, justifies deforming γ
+
into γ,
since no pole obstructs this operation. Thus we get, performing implicitly the
operation “ lim
ǫ→0
+
,”
Z
γ
+
f (z)
z − x
0
dz =
Z
+∞
−∞
f (x)
x − x
0
+ iǫ
dx = pv
Z
+∞
−∞
f (x)
x − x
0
dx −iπ f (x
0
),
or, in a rather more compact fashion
1
x − x
0
+ iǫ
, f
=
pv
1
x − x
0
, f
−iπ
δ(x − x
0
), f
.
Symbolically, we write:
1
x − x
0
+ iǫ
= pv
1
x − x
0
−iπ δ(x − x
0
),
and this formula must be applied to a meromorphic function f which is
holomorphic on the real axis.
Similarly, we have the following result:
1
x − x
0
−iǫ
= pv
1
x − x
0
+ iπ δ(x − x
0
).
