Appel W. Mathematics for Physics and Physicists

Подождите немного. Документ загружается.

Properties of holomorphic functions 107

If Z( f ) has a t least one accumu lation point in Ω, then Z( f ) = Ω, that is, the

function is identically zero on Ω.

If Z( f ) ha s no accumu lation point, then for any a ∈ Z( f ), there exist a u nique

positive integer m(a) and a function g ∈ H (Ω) which does not vanish at a, such

that one ca n write f in the factorized form

f (z) = g(z) ×(z −a)

m (a)

for all z ∈ Ω.

Moreover, Z( f ) is at most countable.

DEFINITION 4.54 The integer m(a) deﬁned in the preceding theorem is called

the order of the zero of f at the point a.

Interpretation

Suppose we have two holomorphic functions f

and f

, both deﬁned on the

same domain Ω, and that we know that f

(z) = f

(z) for any z belonging

to a certain set Z. Then if Z has at least one accumulation point in Ω, the

functions f

and f

are necessarily equal on the whole set Ω.

Thus, if we h ave a f unction holomorphic on C and if we know its val-

ues for instance on R

, then it is — theoretically — entirely determined. I n

particular, if f is zero on R

, then it is zero everywhere.

Note that Ω is not simply open, but it must also be connected. Indeed, if

Ω were the union of two disjoint open subsets, th e rigidity of the function

would not be ab le to “bridge the gap” between the two open subset s, and the

behavior of f on one of the two open subsets would be entirely uncorrelated

with its behavior on the other.

Counterexample 4.55 Assume we know a holomorphic function f at the points z

def

= 1/n, for

all n ∈ N

∗

, and that in fact f (z

) = n. In this case, f is not uniquely determined. In fact,

both functions f

(z) = 1/z and f

(z) = exp(2πi/z)/z satisfy f

) = f

) = f (z) for all

n ∈ N. What gives? Here, because f (z) → ∞ when z tends to 0, f is not holomorphic at 0;

the accumulation poi nt, which i s precisely 0, does not belong to Ω and the theorem cannot be

applied to conclude.

COROLLARY 4.55 .1 Let Ω be a c onnected open subset a nd assume that the open ball

B = B(z

; r) with r > 0 is contained in Ω. If f ∈ H (Ω) and if f is zero on B,

then f ≡ 0 on Ω.

COROLLARY 4.55 .2 Let Ω ⊂ C be a connected open subset, and let f a nd g be

holomorphic on Ω and such that f g ≡ 0 on Ω. Then either f ≡ 0 on Ω or g ≡ 0

on Ω.

Proof. Let us suppose that f 6≡ 0; let then z

be a point of Ω such that f (z

) 6= 0.

Since f is continuous, there exists a posi tive real number r > 0 such that f (z) 6= 0

for all poi nts in B

def

= B(z

; r). Hence g ≡ 0 on B and Corollary 4.55.1 allows us to

conclude that g ≡ 0 on Ω.

108 Complex Analysis I

COROLLARY 4.55 .3 Let f and g be entire functions. If f (x) = g(x) for all

x ∈ R, then f ≡ g.

These theorems also show that functional relations which hold on R re-

main valid on C. For instance, we h ave

∀x ∈ R cos

x + sin

x = 1.

The function z 7→ cos

z +sin

z −1 being holomorpic (it is deﬁned using the

squares of power series with inﬁnite radius of convergence, and is therefore

holomorphic on C) and being identically zero on R, it must be zero on C;

we therefore have

∀z ∈ C cos

z + sin

z = 1.

4.4

Singularities of a function

Certain functions are only holomorphic on an open subset minus one or

a few points, for instance the function z 7→ 1/z, w hich belongs to the set



C \ {0}



. It is in general those functions which are useful in physics.

The points at which f is not holomorphic are called singularities and have a

physical signiﬁcance in many problems.

4.4.a Classiﬁcation of singularities

There are three kinds of singularit ies: artiﬁcial (or removable) singularities,

poles, and essential singularities. The ﬁrst case is not very interesting. As the

name suggests, it is not a “true” singularity. It corresponds to the case of a

bounded f . We have the following theorem due to Riemann (see page 138).

THEOREM 4.56 Let a be a point in C. Let f be a function deﬁned on an open

neighborhood Ω of a, except at a, such that f is holomorphic and bounded on Ω\{a}.

Then lim

z→a

f (z) exists and the function f can be continued to a function f deﬁned on

Ω by putting

f ( a)

def

= lim

ζ→a

f (ζ ).

The f unction f so deﬁned is holomorphic on the whole of Ω.

Thus, in linear response theory, the response function will be, depending on conventions,

analytic (say) in the complex upper half-plane, but will have p ol es i n the lower half -p l ane.

Those poles correspond to the energies of the various modes. In particle physics, they will be

characteristic of the mass of an excitation (particle) and its half-life.

Singularities of a function 109

DEFINITION 4.57 If a is a singularity of f and f is bounded in a neighbor-

hood of a, then a is called a removable singularity, or a n artiﬁcial singular-

ity.

Consequently, if f has an art iﬁcial singularity, it must have been badly

written to begin with. Consider, for instance, z 7→ (4z

−1)/(2z+1), which has

an artiﬁcial singular ity at −1/2; we should instead have written the function

as z 7→ 2z − 1, which would then have been deﬁned on the whole of C.

All artiﬁcial singular ities are not so “ visible,” however, as shown by the next

example.

Example 4.58 The most important example of an artiﬁcial singularity is t he following. Let f

be a function holomorphic on a domain Ω ⊂ C. Let z

∈ Ω and deﬁne

g(z)

def

f (z) − f (z

)

z − z

∀z ∈ Ω \{z

Then g is holomorph ic on Ω \{z

} and has an artiﬁcial singularity at z

. Indeed, if we extend

g by continuity by putting

g(z)

def







f (z) − f (z

)

z − z

if z 6= z

′

) if z = z

then we obtain a function which is holomorphic on Ω.

Other singularities are classiﬁed in two categories.

THEOREM 4.59 (Casorati-Weierstra ss) Let a ∈ Ω be an isolated singularity of

a function f ∈ H



Ω \ {a}



. If a is not a removable singularity, then only two

possibilities can occur:

a) lim

z→a



f (z)



exists and is equal to +∞ ;

b) for any r > 0 such that B(a ; r) ⊂ Ω, the image of B(a ; r) \ {a} by f is

dense in C.

Proof. Assume there exists r > 0 such that the image of B(a ; r) is not dense i n C.

Then, for some λ ∈ C and some ǫ > 0, we have

|f (z) −λ| > ǫ for all z ∈



B(a ; r) \ {a}



Consider now the funct ion g, deﬁned on B( a ; r)\{a} by g(z)

def

= 1/



f (z)−λ



. Then g

is holomorphic on B(a ; r) \{a} and, moreover, |g(z)| < 1/ǫ for all z ∈ B(a ; r) \{a}.

According to the removable singularity t h eorem, we can continue t he function g to a

function ˆg : B(a ; r) → C. This new function ˆg is then nonzero everywhere, except

possibly at a. For all z 6= a, we have

f (z) = λ +

ˆg(z)

. (∗)

If ˆg(a) were not 0, the right -h and side of (∗) would be holomor phic on B(a ; r) and

f would have an artiﬁc ial singularity at a, which we assumed was not the case. Hence

ˆg(a) = 0 and lim

z→a



f (z)



= +∞.

110 Complex Analysis I

DEFINITION 4.60 (Poles) If f ∈ H



Ω \{a}



and if

lim

z→a



f (z)



= +∞,

then f admits a pole at a, and a is called a pole of f .

DEFINITION 4.61 (Essential singularity) If f ∈ H



Ω \ {a}



and if



f (z)



has no limit as z tends to a, then f is said to have an essential singularity

at a.

Poles and essential singularities are of a very different nature. Whereas

functions behave quite “reasonably” in the neighborhood of a pole, t hey are

very wild in the neighborhood of an essential singularity.

Example 4.62 Consider the function f : z 7→ exp(1/z). Then, in any neighborhood of 0, the

function f takes any value in C except 0 and oscillates so fast, in fact, that it reaches any

of the se complex values inﬁnitely often. This can be seen as follows. Let λ be an arbitrary

nonzero complex number. There exists a complex number w such that e

= λ. If we now put

def

= 1/(w + 2πin), then z

−−→

n→∞

0 and f (z

) = exp( 1/z

) = λ for all n ∈ N.

4.4.b Meromorphic functi ons

DEFINITION 4.63 A s ub set S of C is locally ﬁnite if, for any closed disk D,

the subset D ∩ S is ﬁnite. If Ω is an open set in C and if S ⊂ Ω, S is locally

ﬁnite in Ω if and only if for any closed disque D contained in Ω, the set

D ∩ S is ﬁ nite.

In practice, to say tha t S is locally ﬁnite in C boils down to saying that

either S is ﬁnite, or S is countable and can be wr itten S = {z

; n ∈ N} with

lim

n→∞

| = +∞.

Example 4.64 Let f be a functi o n holomorphic on an open set Ω and not identically zero.

Then the set of zeros of f is locally ﬁnite in Ω.

Example 4.65 The set {1/n ; n ∈ N

∗

} is locally ﬁnite in the open ball B(1 ; 1) (which does not

contain 0), but not in C.

DEFINITION 4.66 Let Ω be an open subset of C and S ⊂ Ω a subset of Ω. A

function f : Ω \ S → C is a meromorphic function on Ω if and only if

i) S is a closed subset of Ω and is locally ﬁnite in Ω;

ii) f is holomorphic on Ω \ S (which is then necessarily closed);

iii) for any z ∈ S, f has a pole at z.

Example 4.67 The function z 7→ 1/z is meromorphic on C. The set of its singularities is the

singleton {0}.

Laurent series 111

PROPOSITION 4.68 If Ω is an open subset of C and if f : Ω → C is holomorph ic

and not identically zero, then the f unction

F (z)

def

f (z)

for all z ∈ U \ Z( f )

is a meromorphic function.

Proof. The function F is ho l omorphic on Ω \ Z( f ) and, since Z( f ) is locally

ﬁnite, according to Theorem 4.53 on page 106, it sufﬁces to check that for any point

a ∈ Z( f ), we have lim

z→a



F (z)



= +∞, whic h is immediate by continuit y.

Example 4.69 If P and Q are coprime polynomials with complex coefﬁcients (i.e., if they have

no common zero in C), with Q 6= 0, the rational function P /Q is meromorphic on C. The

set of its poles is the set of zeros of Q, whic h is locally ﬁnite (in fact ﬁnite) because Q is

holomorphic.

Remark 4.70 Two other kinds of singularities are sometimes deﬁned:

Branch points appear when a function cannot be deﬁned on a simply co nnected open set , as,

for instance, the c o mplex logarithm or other multivalued functions (see Section 5.1.a

on page 135).

Singularities at inﬁnity are deﬁned by making the substitution w = 1/z. One obtains a new

function F : w 7→ F (w) = f ( 1 /w); then f has a singularity at inﬁnity if F has a

singularity at 0, those singularities being by deﬁnition of the same kind (see page 146).

4.5

Laurent series

4.5.a Introduction and deﬁnition

We h ave just seen that a function holomor phic on Ω \ z

could have singu-

larities of two different kinds at z

: an essential singularity or a pole. We

can shed some light on this distinction in the following manner. If f can be

written in the form of the following series (which is not a power ser ies, since

coefﬁcients with negative indices occur):

f (z) =

n∈Z

(z − z

)

then

• f h as a pole at z

if there are only ﬁnitely many nonzero coefﬁcients

with negative indices;

• f has an essential singularity at z

if there are inﬁnitely many.

112 Complex Analysis I

The next theorem stipulates that f usually admits such an expansion.

THEOREM 4.71 (Laurent series) Let f be a function holomorph ic on the annu lus

< |z − z

| < ρ

, where 0 ¶ ρ

< ρ

¶ +∞. Then f a dmits a unique

expansion, in this annulus, of the type

f (z) =

+∞

n=−∞

(z − z

)

Moreover, the coefﬁcients a

are given by

2iπ

f (ζ )

(ζ − z

)

n+1

dζ ,

where C is any contour inside the annulus turning once around z

in the positive

direction (i.e., Ind

) = 1).

Remark 4.72 The annulus considered may also be “inﬁnite,” as, for instance, C

∗

= C \{z

This motivates the following deﬁnition, due to Laurent

DEFINITION 4.73 The preceding expans ion is called the Laurent s eries ex-

pansion of f . If there are only ﬁnitely many nonzero coefﬁcients w ith

negative index in this expansion, then the absolute value of the smallest in-

dex with nonzero coefﬁcient is called the order of the pole at z

. A pole of

order 1 is also called a simple pole.

Proof of Theorem 4.71. Without loss of generality, we may assume that z

in the

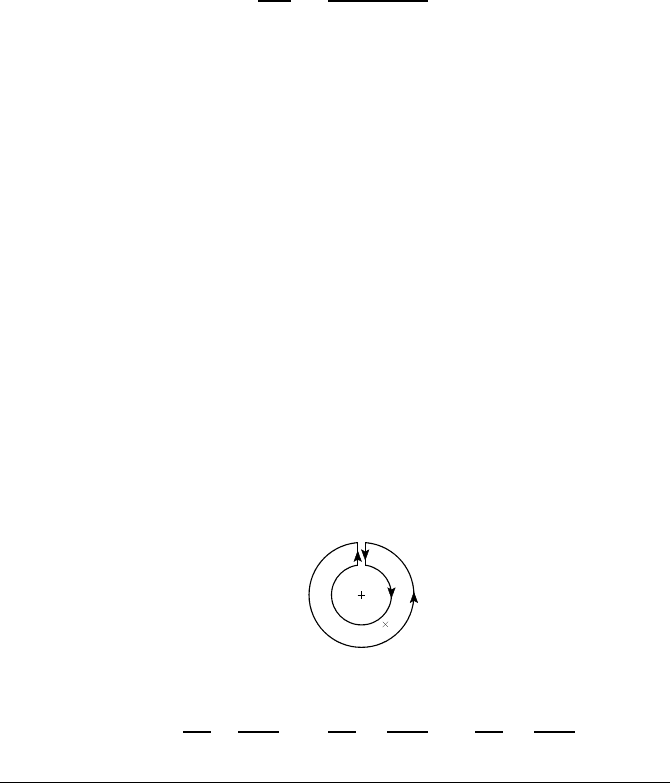
proof. We cut the annulus in the way shown in the ﬁgu re below. The interior circle,

with radius ρ

, is taken with negative orientation, and the ex terior circle with positive

orientation. The two parallel segments on the ﬁg u re are very close, and we will take

the limit where they become identical at the end of the proof. Denote by γ the

contour thus deﬁned, and by γ

and γ

the two circles with positive orientation. De note

moreover by Ω the interior of the contour γ, t h at is, the open annulus minus a small

strip. This open set is not convex, but it is still simply connected. One can therefore

apply Cauchy’s formula on Ω (or, more precisely, on a simply connected neighborhood

of Ω containing γ).

We have then, for any p o int z in t he annulus, by Cauchy’s formula applied to f

and γ:

f (z) =

2πi

f (ζ )

ζ − z

dζ =

2πi

f (ζ )

ζ − z

dζ −

2πi

f (ζ )

ζ − z

dζ

Pierre Laurent, (1813—1853), a former student at the École Polytechniqu e, was a hydraulic

engineer and mathematician.

Laurent series 113

in the limit where both segments coincide. It is enough then to write that, for any

ζ ∈ γ

, we have |ζ − z| < 1 and hence

ζ − z

1 − z/ζ

∞

n=0

n+1

whereas for ζ ∈ γ

, we have |z/ζ| < 1 and

ζ − z

1 −ζ /z

= −

−1

n=−∞

n+1

This proves the ﬁrst formula stated. Moreover, the value of a

is given by the integral

on γ

or γ

depending on whether n is posi tive or negative. The integral on any

intermediate curve gives — of course — the same result.

Remark 4.74 We know that a power series has a radius of convergence, that is, i f

converges

for a certain z

∈ C, then it converges for any z ∈ C with |z| < |z

|. We have, for Laurent

series, a similar result: if

+∞

n=−∞

converges for z

and z

with |z

| < |z

|, then it converges

for any z ∈ C such t h at |z

| < |z| < |z

|. Similarly, for a given Laurent series, th ere exi st

two real numbers ρ

and ρ

(with ρ

possibly +∞) such that the Laurent series converges

uniformly and absolutely in the interior of the open annulus ρ

< |z| < ρ

and diverges

outside. This result is element ary and does not require the theory of holomorphic funct ions.

Remark 4.75 The proof of the theorem also shows that f can be written as f = f

+ f

, where

is holomorphic in the disc |z| < ρ

and f

is holomorphic in the domain |z| > ρ

. I f one

asks, in addition, that f

(z) −−→

z→0

0, then this decomposition is unique.

4.5.b Examples of Laurent series

In the case where a function f has only one singularity, for instance,

f (z)

def

z −1

the Laurent series may be deﬁned on the (inﬁnite) “annulus”

def

= {z ∈ C ; |z| > 1}.

Indeed, one can write

f (z) =

z −1

1 −

∞

n=1

(4.6)

for |z| > 1. Note that this series has inﬁnitely many negative indices, but

beware, this does not mean that there is an essential singularity! Indeed, this

inﬁnite series comes from having performed the expansion in an a nnulus

around 0, while the pole is at 1. A Laurent series expansion around z = 1

gives of course

f (z) =

z −1

with no other term. Remark that this Laurent series e xpansion (4.6) is valid

only in the “annulus” |z| > 1, outside of which the series diverges rather

trivia lly.

114 Complex Analysis I

This same function f can be expanded on the annulus 0 < |z| < 1 by

f (z) = −

∞

n=0

When a function has more than one singularity, the annulus must remain

between the poles; for example, the function g(z) = 1/(z − 1)(z + 2) admits

a Laurent series expansion on the annulus 1 < |z| < 2. Note that it also

admits a (different) Laurent series expansion on the annulus 2 < |z|, and a

third one on 0 < |z| < 1 (this last expansion with out any nonzero coefﬁcient

with negative index, since the function g is very cosily holomorphic at 0).

4.5.c The Residue theorem

DEFINITION 4.76 An open subset Ω ⊂ C is holomorphically simply con-

nected if, for any holomorphic function f : Ω → C, there exists a holomor-

phic function F : Ω → C such that F

′

= f .

We will admit the following results:

LEMMA 4.77 An open subset Ω ⊂ C is holomorphically simply connected if and only

if, for any holomorphic f unction f : Ω → C and for any closed pa th γ inside Ω, we

have

f (z) dz = 0.

LEMMA 4.78 An open subset Ω ⊂ C is holomorphically simply connected if and only

if it is simply connected.

Consequently, we will drop the appellation “holomorphically simply con-

nected” from now on. The notion of simple connectedness acquires a r icher

meaning because of the preceding lemma however.

DEFINITION 4.79 (Residue) Let f be a function that can be expanded in

Laurent series in an annulus ρ

< |z − z

| < ρ

f (z) =

n∈Z

(z − z

)

for ρ

< |z − z

| < ρ

The coefﬁcient a

−1

(corresponding to the term with 1/(z − z

)) is called t he

residue of f at z

and is denoted

−1

= Res ( f ; z

In particular, if f ∈ H (Ω \{z

}) and f has a pole at z

, we ca n write

f (z) = g(z) +

n=1

−n

(z − z

)

Laurent series 115

with g holomorphic on Ω, and a

−1

is the residue of f at z

We will see in the next section how to compute the residue of a function

at a given point.

THEOREM 4.80 Let f be a function holomorphic on the annulus

< |z − z

| < ρ

Let γ be a closed pa th contained inside this annulus. Then we have

2πi

f (z) dz = Ind

) ×Res ( f ; z

). (4.7)

Proof. We can indeed w rite (taking z

= 0)

f (z) =

−1

+ g(z), where g(z) =

n6=−1

Since the series

n6=−1

converges, as well as the series

n6=−1

n+1

/(n + 1), it

follows that the function g has a holomorphic primitive. Hence

g(z) d z = 0 and

we get

f (z) dz = a

−1

= 2πi a

−1

Ind

(0),

which is the required formula.

Another formulation of this theorem, which will be of more use in prac-

tice, is as follows:

THEOREM 4.81 (Residue theorem) Let Ω ⊂ C be a simply c onnected open set

and let P

, . . . , P

be distinct points in Ω. Let f ∈ H



Ω \{P

, . . . , P

}



and let γ

be a closed path c ontained in Ω which does not contain any of the points P

, . . . , P

Then we have

f (z) dz = 2πi

i=1

Res ( f ; P

) Ind

Why is the Residue theorem useful?

We can make clear immediately that it s interest, for a physicist, is mostly

computational. Then it is, in many cases, irreplaceable. It relates a path

integral in the complex plane with a sum w hich depends only on the b ehavior

of the function near ﬁnitely many points. It is therefore possible to compute

the integral around a closed path (see Section 4.6.d) or, through a small trick,

an integral over R (see Sections 4.6.b and 4.6.c). Conversely, th e sum of certa in

series can be identiﬁed with an inﬁnite sum of residues, which is linked to

an integral over a closed path; by deforming this closed path, one may then

relate this integral to a ﬁnite sum of residues (see Section 4.6.e).

But before going into this, we must see how to compute in practice the

residue of a function at a given point.

116 Complex Analysis I

4.5.d Practical computations of residues

In this short s ection, we will see how to compute in practice the residue of

a function. In almost all cases we will have to consider a function f ∈



Ω \ {z

}



, where Ω is a neighborhood of a point z

∈ C. In the cas e

where f admits a simple pole at z

, namely, if one can express this function

in the form f (z) = a

−1

/(z − z

) + g(z), where g ∈ H (Ω), then we have

−1

= Res ( f ; z

) = lim

z→z

(z − z

) f (z). (4.8)

How can one know if f has a simple pole at z

and not a higher order

pole? If one has no special intuition in the matter, one can try to use the

preceding formula: if it works, this means the pole was simple; if it explodes,

the pole was of higher order!

Consider, for example, the case where f is a function that has a pole of

order 2 at z

. Then we have

f (z) =

−2

(z − z

)

−1

(z − z

)

+ g(z) with g ∈ H (Ω). (4.9)

Formula (4.8) then gives indeed an inﬁnite value in this situation. How, then,

can one obtain a

−1

without being annoyed by the “more divergent” part? If

we multiply equation (4.9) by (z − z

)

, we get

(z − z

)

f (z) = a

−2

+ a

−1

(z − z

) + (z − z

)

g(z).

Differentiate with respect to z:



(z − z

)

f (z)



= a

−1

+ 2(z − z

) g(z) + (z − z

)

′

(z).

By taking the limit [z → z

], we obtain exactly a

−1

and this without ex-

plosion. As a general rule, for a pole of order k, one can use the following

formula, the proof of which is immediate:

THEOREM 4.82 Let f ∈ H



Ω \{z

}



be such that f has a pole of order k at z

Then the residue of f at z

is given by

Res ( f ; z

) =

(k −1)!

lim

z→z

k−1

(z − z

)

f (z)

. (4.10)

Example 4.83 Let a be a nonzero complex number. Consider the function f (z) = 1 /(z

+ a

which is meromorphic on the complex plane and has two poles at the points +ia and −ia.

These poles are simple and we have

Res ( f ; ia) = lim

z→ia

(z −ia) f (z) = lim

z→ia

(z −ia)

(z −ia)(z + ia)

= li m

z→ia

(z + ia)

2ia

I agree that t h is is a dangerous method, but as long as it is restricted to mathematics and

is not applied to chemistry, things will be ﬁne.