Appel W. Mathematics for Physics and Physicists

Подождите немного. Документ загружается.

Integrals with parameters 77

3.3

Integrals with parameters

3.3.a Continuity of functions deﬁned by integrals

Let (X , T ; µ) be a measure space. Assume we have a function of two variables

f : [a, b] × X → R or C. Assuming the integ rals make s ens e, one can deﬁne

a function by integrating over X for ﬁxed values of th e parameter x ∈ [a, b]:

let

I(x)

def

f (x, t) dt for x ∈ [a, b] .

A natural question is to determine w hat properties of regularity the inte-

gral I(x) will inherit from properties of f .

The two main results concern the continuity or derivability of f . Here

again, the Lebesgue theory gives very convenient answers:

THEOREM 3.11 (Continuity of functions deﬁned by integrals) Let x

∈ [a, b].

Assume that for almost all t ∈ X , the function x 7→ f (x, t) is continuous at x

, and

that there exists a µ-integrable function g : X → R such that



f (x, t)



¶ g(t) for almost all t ∈ X ,

for all x in a neighborhood of x

. Then x 7→

f (x, t) dt is continuous at x

Proof. This is a simple consequence of the dominated convergence theorem. Indeed,

let (x

)

n∈N

be any sequence of real numbers converging to x

. For n l arge enough, the

values of x

lie in the neighborhood of x

given by the statement of the theorem. Then

for almost all t ∈ X , we have lim

n→∞

f (x

, t) = f (x

, t) by continuity, and, moreover,



f (x

, t)



¶ g(t) for all t. From the dominated convergence theorem, we deduce

lim

n→∞

I(x

) = lim

n→∞

f (x

, t) dt =

f (x

, t) dt =

lim

n→∞

f (x

, t) dt,= I(x

which, being valid for an arbitrary sequence (x

)

n∈N

, is the statement we wanted to

prove.

Counterexample 3.12 It is of course natural to expect th at the conclusi o n of Theorem 3.11

should be true in most c ase s. Here is an example where, in the absence of the “domination”

property, t he continuity of a function deﬁned by an i ntegral with parameters does not hold.

Let f be the function on R × R deﬁned by

f (x, t) =







exp



−



t −





if x 6= 0,

0 if x = 0.

For all t ∈ R, the function x 7→ f (x, t) is continuous on R, and in particular at 0.

Let I(x) =

f (x, t) dt.

78 Integr al calculus

For any ﬁxed nonzero x, the function t 7→ f (x, t) is a gaussian centered at 1 /x, so its

integral is easy to compute; it is in fact equal to 1 . But for x = 0, the function t 7→ f (0, t) is

identically zero, so its integral is also zero. Hence we have

I(0) = 0 but ∀x ∈ R

∗

, I(x) = 1.

This shows that the function I is not continuous at zero.

3.3.b Differentiating under the integral sign

THEOREM 3.13 (Differentiability of functions deﬁned by integrals) Let x

∈

[a, b]. Assume that there exists a neighborhood V of x

such that for x ∈ V , the

function x 7→ f (x, t) is differentiable at x

for almost a ll t ∈ X , and t 7→ f (x, t)

is integrable. Assume, moreover, that the derivatives are dominated by an integrable

function g, that is, there exists an integrable function g : X → R such that



∂ f

∂ x

(x, t)



¶ g(t) for all x ∈ V and almost all t ∈ X .

Then the function x 7→ I(x) =

f (x, t) dt is differentiable at x

, and moreover we

have

Z

f (x, t) dt





x=x

∂ f

∂ x

, t) dt.

Those theorems are very useful in particular when dealing with the theory

of the Fourier t ransform.

Counterexample 3.14 Here again, the assumption of domination cannot be dispensed wi th.

For instance, let

F (x) =

+∞

cos(x t)

1 + t

dt;

one can th en show (see Exercise 3.10 on page 84) that

F (x) =

−|x|

for all x ∈ R. This function is continuous on R, but is not differentiable at 0.

3.3.c Case of parameters appearing in the integration range

THEOREM 3.15 With X = R and the same assumption as in Theorem 3.13, let

moreover v : V → R be a func tion differentiable at x

. Let

I(x) =

v(x)

f (x, t) dt.

Then I is differentiable at x

and we h ave



v(x)

f (x, t) dt





x=x

v(x)

∂ f

∂ x

, t) dt + v

′

(x) · f



, v(x

)



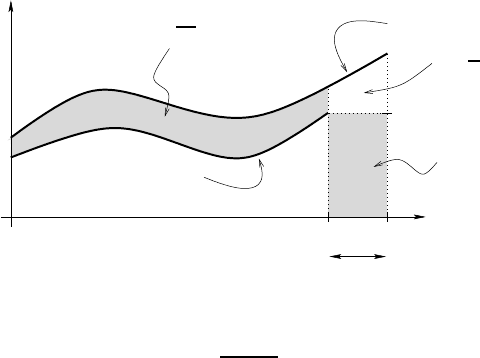
Double and multiple integrals 79

The derivative of this function deﬁned by an integral involves two terms:

one obtained by keeping th e limits of integration constant, and differentiating

under the integral sign; and the second obtained by d ifferentiating the integral

with varying boundary.

≈

v(x)

∂ f

∂ x

, t) dt

≈ h v

′

(x) · f



, v(x

)



f (x

, v(x

))

≈ C

·h

v(x

) v(x

+ h)

≈ h v

′

)

f (x

, ·)

f (x

+ h, ·)

3.4

Double and multiple integrals

For completeness and ease of reference, we recall the theorem of Fubini

mentioned at the end of the previous chapter.

THEOREM 3.16 (Fubini-Lebesgue) Let (X , T , µ) and (X

′

, T

′

, ν) be measure

spaces, each of which is the union of a sequence of sets with ﬁnite measure, for instance,

X = Y = R with the L ebesgue measure. Let f : X × Y → R be a mesurable

function on X × Y for the product σ-algebra. Then:

i) f is integrable on X ×Y with respect to the product measure µ ⊗ν if and only

if one of the following integrals is ﬁnite:

Z Z



f (x, y)





dx or

Z Z



f (x, y)





dy.

ii) If f is integrable on X × Y , then for almost all x ∈ X , the function y 7→

f (x, y) is ν-integrable on Y , and similarly when exchanging the role of x

and y.

iii) If f is integrable on X × Y , the function deﬁned for almost all x ∈ X by

x 7−→ I(x)

def

f (x, y) dν( y)

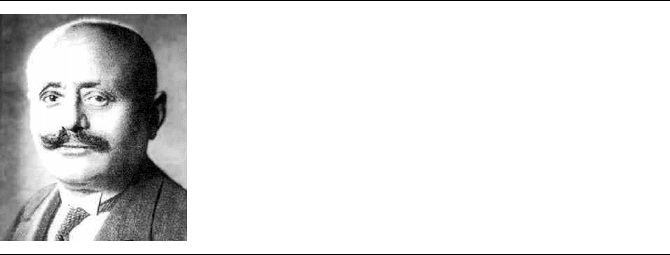
80 Integral calculus

A child prodigy, the Venetian Guido Fubini (1879—1943) found,

in nineth grade, a formula for π as a series converging faster

than anything known at the t ime. At the Scuola normale superi-

ore di Pisa, he was student of Dini (see page 22). He then held

positions at Pisa and Torino. Mussolini’s fascist regime forbade

him to teach, and he went into exile to Paris and then to Prince-

ton University in the United States, where he ﬁnished hi s career.

In addition to his work on the Lebesgue integral, he proved im-

portant results in functional analysis, differential geometry, and

projective geometry.

is µ-integrable on X and we have

I(x) dµ(x) =

X ×Y

f d(µ ⊗ν)(x, y).

Remark 3.17 Since X and Y play symmetric roles, we also have

X ×Y

f =

Z

f (x, y) dµ(x)



dν( y) =

Z

f (x, y) dν( y)



dµ(x).

For non-negat ive functions, we have a converse:

THEOREM 3.18 (Fubini-Tonelli) Let (X , T , µ) and (X

′

, T

′

, ν) be measure spaces,

each of which is the union of a sequence of sets with ﬁnite measure, for instance,

X = Y = R with the Lebesgue measure. Let f : X × Y → R be a non-negative

measurable function. Then the integral of f on X × Y with respect to the product

measure µ ⊗ ν, which is either a non-negative real number or +∞, is given b y the

Fubini formula

X ×Y

f (x, y) d(µ ⊗ν)(x, y) =

Z

f (x, y) dµ(x)



dν( y)

Z

f (x, y) dν( y)



dµ(x).

In particular, if the following two conditions hold:

i) for almost all x ∈ X , the func tion y 7→ f (x, y) is integrable on Y ;

ii) the function deﬁned for almost all x ∈ X by x 7→ I(x)

def

f (x, y) dy is

µ-integrable,

then f is integrable on X × Y with respect to the product measure µ ⊗ν.

Change of variables 81

3.5

Change of variables

The last important point of integ ral calculus is th e change of variab le for-

mula in multiple integrals. Suppose we wish to perform a ch ange of variable

in 3 dimensions

(x, y, z) 7−→ (u, v, w) = ϕ(x, y, z),

(and analogously in n dimensions); then the jacobian of this change of variable

is deﬁned as follows:

DEFINITION 3.19 Let Ω and Ω

′

be two open sets in R

. Let ϕ be a bijection

from Ω to Ω

′

, which is continuous and has continuous differential, as well

as its inverse. Denote (u, v, w) = ϕ(x, y, z). The jacobian ma trix of ϕ is the

matrix of partial derivatives

Jac

(x, y, z)

def

D(u, v, w)

D(x, y, z)

def







∂ u

∂ x

∂ u

∂ y

∂ u

∂ z

∂ v

∂ x

∂ v

∂ y

∂ v

∂ z

∂ w

∂ x

∂ w

∂ y

∂ w

∂ z







This is a continuous function on Ω. The jacobian of the change of variable

is the determinant of the jacobia n matrix:

(x, y, z) = det Jac

(x, y, z).

It is also a continuous function on Ω.

The generalization of this deﬁnition to change of variables in R

is imme-

diate.

THEOREM 3.20 (Change of variables) Let U and V be open sets in R

. Let

ϕ : U → V be a bijection which is continuous, has continuous differential, a nd is

such that the jacobian of ϕ does not vanish on U . Then for any fu nction f : V → R

we have

f is integrable on V ⇐⇒ ( f ◦ϕ) J

is integrable on U

and moreover

f (u

, . . . , u

) du

. . .du

f ◦ϕ(x

, . . . , x

)



, . . . , x

)



. . .dx

Here integration and integrability are with respect to the L ebesgue measure on R

, and

mean that each component of f is integrable.

82 Integral calculus

As an example, take the change of variable in polar coordinates in the

plane R

, denoted ϕ : (r, θ) 7→ (x, y), which we may assume to b e deﬁned on

the open set ]0, +∞[ × ] −π, π[. We can write



x = r cos θ,

y = r sin θ,







r =

+ y

θ = 2 arctan



x +

+ y



(Tricky question: why shouldn’t we write θ = arctan( y/x) as is often seen?

Why do most people write it anyway?)

The jacobian of this change of variable is



D(x, y)

D(r, θ)



∂ x

∂ r

∂ y

∂ r

∂ x

∂θ

∂ y

∂θ



cos θ sin θ

−r sin θ r cos θ



= r,

and we recover the well-known formula:

f (x, y) dx dy =

−π

+∞

−∞

f ( r, θ) r dr dθ.

Remark 3.21 We migh t be interested in the inverse transformation. The computation of

D(r,θ)

D(x, y)

directly from the formula is rather involved, but a cl assical result of differential calculus avoids

this computation: we have

D(r,θ)

D(x, y)

D(r,θ)

= 1,

and this result may be generalized to arbitrary locally bijective differentiable maps in any

dimension.

Remark 3.22 In one dimension, the change of variable formula is

f (u) du =



ϕ(x)



|ϕ

′

(x)| dx.

It is important not to forget the absolute value in the c h ange of variable, for instance,

f (x) dx =

f (−y) ×|−1| dy =

f (−y) dy.

Sometimes absolute values are omitted, and one writes, for instance, dy = −dx. In this case,

the range of integration is considered to be orient ed, and the orientation is reversed when the

change of variable is performed if it corresponds to a decreasing functio n ϕ : x 7→ y = ϕ(x).

Thus we have

+∞

−∞

f (x) dx =

−∞

+∞

f (−y) (−dy) =

+∞

−∞

f (−y) dy,

with the minus sign cancelling the effect of interchanging the bounds of integration.

Exercises 83

EXERCISES

 Exercise 3.3 Show that

|sin t|

dt ∼

x→+∞

log x.

 Exercise 3.4 Check that the jacobian for the change of variables from cartesian coordinates

to spherical coordinates (x, y, z) 7→ (r, θ, ϕ) in R

is J = r

sin θ.

 Exercise 3.5 Find (and justify!) the following limits:

lim

n→∞

1 + n

dx and lim

n→∞

∞

|sin x|

1 + x

dx.

 Exercise 3.6 Let f (ν) be the distribution function of energy emitted by a black body, as a

function of the frequency. Recall that, by deﬁnition, the total amount of energy with frequency

between ν

and ν

is given by the integral

f (ν) dν.

Max Planck showed at the end of the nineteenth century that the distribution fu nction f (ν)

is given by the formula (which now bears his name)

f (ν) =

Aν

2πβ }hν

−1

where A is a constant, β = 1/k

T is the inverse temperature, and }h is the Planck constant.

What is the distribution function g(λ) of the energy as function of the wavelength λ

def

= c/ν?

For which frequency ν

max

is f (ν) maxi mal? What i s the wavelength for which g(λ) is maximal?

Why is it that λ

max

6= c/ν

max

 Exercise 3.7 Show that the function x 7→ arctan(x) −arctan(x −1) is integrable on R (with

respect to Lebesgue measure, of course) and show that

+∞

−∞



arctan(x) −arctan(x −1)



dx = π.

Generalize this formula.

 Exercise 3.8 (Co unterexample to Fubini’s theorem) For (x, y) ∈ [0, 1]

, deﬁne

f (x, y) =

sgn( y − x)

max(x, y)

i) Compute

f (x, y) dx for all y ∈ [0, 1].

Deduce from this the value of



f (x, y) dx



dy.

ii) Similarly, compute



f (x, y) dy



dx.

iii) Any comments?

84 Integral calculus

 Exercise 3.9 (Integrability of a radial function) Let f be a (measurable) functi on on R

, such

that we have

f (x, y, z) ∼

r→0

for some real number α, where r

def

+ y

+ z

. Assuming that f is integrable on any

bounded region in R

− {0}, discuss the i ntegrability of f “at the origin,” depending on the

value of α. Generalize this to functions on R

 Exercise 3.10 (Fourier transf orm of a Lorentzian function) Let

F (x) =

+∞

−∞

cos ux

1 + u

du for all x ∈ R.

i) Where is f deﬁned? Is it continuous on its domain of deﬁnition?

ii) Let g(x) =

+∞

−∞

x cos t

+ t

dt. What is the relation between F and g?

iii) Deduce from the previous question a differential equation satisﬁed by g. (Start by

comparing

∂

∂ x



+ t



and

∂

∂ t



+ t



Solve the differential equation, and deduce the value of F .)

Solutions of exercises 85

SOLUTIONS

 Solution of exercise 3 .4. Let (r, θ, ϕ) denote spherical coordinates (where θ is latitude and

ϕ longitude). We have

x = r cos θ cos ϕ, y = r cos θ sinϕ, z = r sin θ,

and therefore the jacobian is

J =



cos θ cos ϕ cos θ sin ϕ sin θ

r sin θ sin ϕ r sin θ si n ϕ −r cos θ

−r cos θ sinϕ r cos θ cos ϕ 0



= r

sin θ.

 Solution of exercise 3 .6. The function g(λ) has the property that the amount of energy

radiated by frequencies in the interval [ν

, ν

], corresponding to wavelengths λ

and λ

, is

equal to

g(λ) dλ. The change of variable ν = c/λ leads to

g(λ) dλ =

g(c/ν)



dλ

dν



dν =

g(c/ν)

dν,

and this shows that

f (ν) = c

g(c/ν)

Note that there is no reason for the functions ν 7→ f (ν) and ν 7→ ν

f (ν) to reach a maximum

at the same point. In fact, rathe r than t he functions f and g themselves, it is the differential

forms (or measures) f (ν) dν and g(λ) dλ which have physical meaning.

 Solution of exercise 3 .7. Integrability comes from the inequalities

1 + (x −1)

¶ arctan(x) −arctan(x −1) ¶

1 + x

which are consequences of the mean value theorem. To compute the value of the integral, let

F denote a primitive of arctan. Then we have

−M



arctan(x) −arctan(x −1)



dx = F (M) − F (M −1) − F (−M) + F (−M −1)

= arctan(ξ

) −arctan(ξ

′

where ξ

∈ [M −1, M] and ξ

′

∈ [−M −1, −M ] are given by th e mean value the orem

again. The result stated follows immediately.

 Solution of exercise 3 .10.

i) The uniform uppe r bound u 7→

1+u

and the continuity theorem show that F is deﬁned

and continuous on R. It is obvious that F (x) ¶ π, with equality for x = 0.

ii) By the change of variable t = x y, we have for x > 0

g(x) =

+∞

−∞

cos(x y)

1 + y

dy = F(x).

It follows that g(x) has a limit as x tends to zero on the ri ght, namely,

g(0

) = F (0) = π.

Similarly, for x < 0 we have g(x) = −F (x) and hence g(0

−

) = −π. Since the deﬁnition

gives g(0) = 0, we see that the function g is not continuous at 0.

86 Integral calculus

iii) A simple computation shows that t h e two derivatives mentioned in the statement of the

exercise are equal. From this, we deduce that

∂

∂ x

g(x) =

+∞

−∞

cos t

∂

∂ x



+ t



dt = −

+∞

−∞

cos t

∂

∂ t



+ t



+∞

−∞

cos t



+ t



dt,

using two integrations by parts. It follows that g is a solution of the differential equation

′′

(x) − g(x) = 0.

The general solution o f this equation is of the form

g(x) = α

+ β

−x

where (α

, β

) are real constants (which give the value of g on R

∗+

), and (α

−

, β

−

)

similarly on R

∗−

Since g is bounded, the co efﬁcient α

of e

on R

is zero, and the coefﬁcient β

−

−t

on R

−

also. Since g is odd and g(0

) = π, we ﬁnally ﬁnd that

g(x) = π sgn(x) e

−|x|

Since F (x) = sgn(x) · g(x) and F (0) = π, we ﬁnally ﬁnd that

∀x ∈ R F (x) = π e

−|x|