Weinan E. Principles of Multiscale Modeling
Подождите немного. Документ загружается.


5.2. EXTENSIONS OF THE CAUCHY-BORN RULE 227
neighboring atoms via Taylor expansion:
y
j,1
y
j,2
y
j,3
=
x
j,1
x
j,2
0
+
u
1
(x
j,1
, x
j,2
)
u
2
(x
j,1
, x
j,2
)
u
3
(x
j,1
, x
j,2
)
≈
˜y
j,1
˜y
j,2
˜y
j,3
= x
i
+ u(x
i,1
, x
i,2
) +
∂u(x
i,1
, x
i,2
)
∂x
1
(x
j,1
− x
i,1
)
+
∂u(x
i,1
, x
i,2
)
∂x
2
(x
j,2
− x
i,2
) +
1
2
∂
2
u(x
i,1
, x
i,2
)
∂x
2
1
(x
j,1
− x
i,1
)
2
+
∂
2
u(x
i,1
, x
i,2
)
∂x
1
∂x
2
(x
j,1
− x
i,1
)(x
j,2
− x
i,2
)
+
1
2
∂
2
u(x
i,1
, x
i,2
)
∂x
2
2
(x
j,2
− x
i,2
)
2
(5.2.19)
where u = (u
1
, u
2
, u
3
)
T
is the displacement field, x
i
= (x
i,1
, x
i,2
) is the reference position
of the i-th atom. Substituting this into the atomistic model, we obtain the stored energy
density at x
i
:
W
LCB
(∇u, ∇
2
u) =
1
D
0
X
j,k
V (
˜
y
i
,
˜
y
j
,
˜
y
k
) (5.2.20)
where D
0
is the area of a unit cell,
˜
y
i
= (x
i,1
, x
i,2
, 0)
T
.
Note that this procedure can be used for both simple and complex lattices.
As an example, let us consider the grapheen sheet which is a two dimensional complex
lattice obtained from the union of two triangular lattices. In equilibrium, one set of basis
vectors for the triangular lattice is given by:
A
1
= a(1, 0), A
2
= a
1
2
,
√
3
2
!
(5.2.21)
where a is the lattice constant, the shift vector is:
p = a
1
2
,
√
3
6
!
. (5.2.22)
This example is treated in [1] and [46] using the Tersoff potential as the atomistic model.
Figure 5.2.3 shows the results of a twisted nanotube, m odeled using the atomistic model
as well as the continuum model obtained using the exponential Cauchy-Born rule. Figure
5.2.3 shows the resu lts of the nanotube under compression, modeled using the atomistic
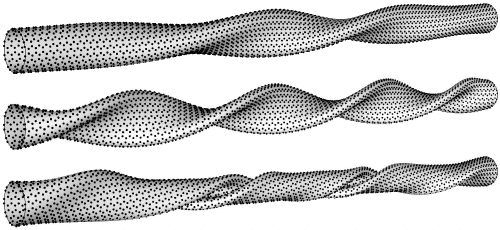
228 CHAPTER 5. EXAMPLES OF MULTI-PHYSICS MODELS
model and th e continuum model obtained using the local Cauchy-Born rule. Very good
agreement is found in both cases. This demonstrates that mechanical deformation of
nanotubes can be very well described by continuum theories in this regime. The value of
such a viewpoint has also been demonstrated using much simplified continuum models
[45].
60
220
300
o
o
o
Figure 5.4: Comparison of the results of the MD simulation and the continuum model for
twisted nano-tube using the exponential Cauchy-Born rule. Dots represent the results of
the MD simulation. The grey background is computed from the exponential Cauchy-Born
rule ([1], courtesy of Ted Belytschko).
5.3 The moving contact line problem
When water or other liquid drops are placed on a solid surface, a contact line forms
at the intersection between the liquid, the air and the solid. More generally, the same
situation arises when considering the dynamics of immiscible fluids in a channel [12, 14],
as in the case of secondary oil recovery when oil is drained out of the pores y some other
liquid. Like the problem of crack propagation, contact line dynamics has often been used
as an example to illustrate the need for formulating coupled continuum-atomistic models,
since it has been realized for some time that classical continuum models are inadequate
near the contact line and atomistic models should give a more accurate representation [20,
33]. While developing coupled atomistic-continuum models is certainly a very important
avenue of research, we will illustrate in this section that multiscale ideas can also be used
in a different way, to help formulating better continuum models.
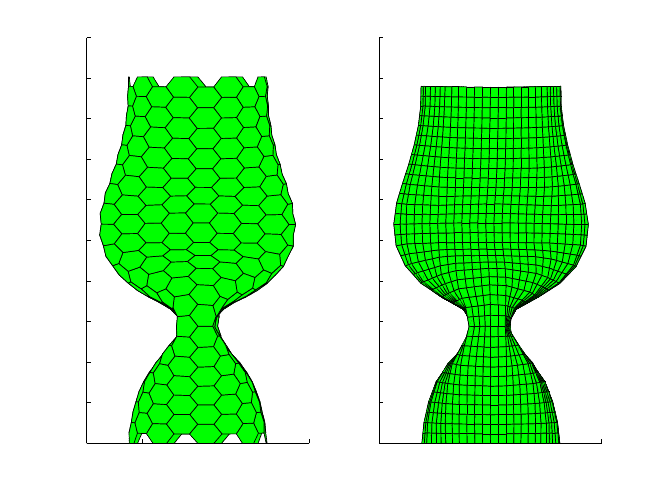
5.3. THE MOVING CONTACT LINE PROBLEM 229
−10 −5 0 5 10
0
5
10
15
20
25
30
35
40
45
50
x (
˚
A)
z (
˚
A)
−10 −5 0 5 10
0
5
10
15
20
25
30
35
40
45
50
x (
˚
A)
z (
˚
A)
(a) (b)
Figure 5.5: Comparison of the results of the MD simulation (left) and the continuum
model (r ight) for compressed nano-tube using the local Cauchy-Born rule (from Yang
and E [46]).
5.3.1 Classical continuum theory
The setup is shown in Figure 5.3. The three phases are separated by three interfaces
Γ
1
, Γ
2
and Γ
3
respectively. We denote the su rface tension coefficient of Γ
i
by γ
i
, i = 1, 2, 3.
The equilibrium problem and the static configuration of the contact line was under-
stood a long time ago in the work of Laplace, Young and Gauss [11]. At equilibrium, the
total energy of the system is the sum of the interfacial energies
E =
X
i=1,2,3
γ
i
|Γ
i
| (5.3.1)
We will only consider the case of partial wetting, i.e.
|γ
1
− γ
2
| < γ
3
(5.3.2)
In this case, the Euler-Lagrange equation of the functional (5.3.1) is given by:
γ
1
− γ
2
+ γ
3
cos θ
0
= 0. (5.3.3)
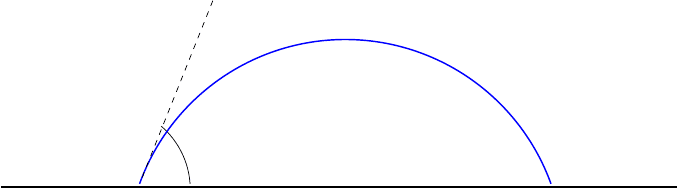
230 CHAPTER 5. EXAMPLES OF MULTI-PHYSICS MODELS
Γx
CL
Γ
Γ
Ω
Ω
1
2
3
1
2
Θ
0
Figure 5.6: A two-phase (in Ω
1
and Ω
2
respectively) fluid on the substrate. x
CL
is the
contact line (here a p oint) and Γ
i
(i = 1, 2, 3) are the interfaces. θ
0
is the contact angle
(courtesy of Weiqing Ren).
This is Young’s relation for the case of partial wetting, and it defines the static contact
angle θ
0
.
Now let us turn to the dynamic problem. To be specific, let us consider the situation
of two immiscible fluids in a channel separated by an interface. To model the dynamics
of such a system, the most obvious approach is to use the Navier-Stokes equation in the
fluid phases:
ρ
i
(∂
t
u + u · ∇u) = −∇p + µ
i
∆u, in Ω
i
∇ · u = 0, (5.3.4)
together with the no-slip boundary condition on the solid surface:
u = u
w
. (5.3.5)
Here ρ
i
and µ
i
, i = 1, 2 are the density and viscosity of the two fluid phases respectively,
u
w
is the velocity of the wall. At the fluid-fluid interface, we use the standard Laplace-
Young interfacial condition:
γ
3
κ = n · [τ ] · n, t · [τ ] · n = 0 (5.3.6)
where t and n denote the un it tangent and normal vectors to the interface respectively, τ
denotes the total stress (viscous str ess and pressure) in the fluids, κ is the mean curvature
of the fluid-fluid interface, [·] denotes the jump of the quantity inside the bracket across
the interface.

5.3. THE MOVING CONTACT LINE PROBLEM 231
It was discovered long ago that in the case of partial wetting, this model predicts a
singularity at the contact line [21], known as the Huh-Scriven singularity. This is most
clearly seen in two dimension. Let (r, φ) be the polar coordinates centered at the contact
line. Then a simple Taylor expansion at the contact line gives, for r ≪ 1,
ψ ∼ r ((Cφ + D) cos φ + (Eφ + F ) sin φ) (5.3.7)
for the streamfunction ψ, where C, D, E, F are some constants. The velocity is given in
terms of the stream function via u = (−∂
y
ψ, ∂
x
ψ). Therefore we obtain
∇u ∼
1
r
,
Z
|∇u|
2
dx = +∞ (5.3.8)
For this reason, questions have been raised on the validity of the conventional hydrody-
namics formalism, the Navier-Stokes equation with no-slip boundary condition, for this
problem. Many proposals have been made to remove this singularity (for a review, see
[33]). While they have all succeeded in doing so, it is not clear whether any of them has
a solid physical foundation.
Detailed molecular dynamics studies have revealed the fact that near the contact line,
there is a slip region in which the no-slip boundary condition is violated [24, 38]. The
existence of such a slip region is not surprising. In fact in a Couette flow setting (i.e.
channel flow driven by walls moving in the opposite direction), if the system reaches a
steady state, then the contact line has to exp erience complete slip, i.e. the relative slip
velocity between the fluids and the solid surface should be equal to the velocity of the
solid surface. What is more important is that these molecular dynamics studies have
opened up the door to a detailed analysis of what is going on near th e contact line at the
atomistic level.
Even though molecular dynamics is able to offer much needed details about the con-
tact line problem, it is n ot very attractive as a theoretical tool, compared with the
continuum models. It is much harder for us to think about the behavior of the individual
molecules instead of the continuum fields. Therefore, from a theoretical viewpoint, it is
still of interest to develop better continuum models. We will demonstrate that multiscale
ideas are indeed quite useful in this respect.
232 CHAPTER 5. EXAMPLES OF MULTI-PHYSICS MODELS
5.3.2 Improved continuum models
The strategy that we will discuss consists of two steps. The first step is to find
the simplest form of the constitutive relations including the boundary conditions, from
thermodynamic considerations. The second step is to measure the detailed functional
dependence of the constitutive relation using molecular dynamics. Such a strategy was
pursued in [35].
There are three constitutive relations that we need:
1. The constitutive relation in the bulk, which describes the response of the fluid in
the bulk. It has been argued that even for simple fluids, the linear constitutive
relation that is usually quite accurate for bulk fluids, may not be accurate enough
near the contact line, due to the presen ce of large velocity gradients there [38, 40].
The careful molecular dynamics studies carried out by Qian et al. do not support
this argument [31]. Instead, it is found that, at least for simple fluids, the linear
constitutive relation holds quite well right around the contact line. In other words,
even though the velocity gradient is quite large, it is not large enough to induce
significant nonlinear effects in the bulk response. We will assume for simplicity that
linear constitutive relations are sufficient in the bulk.
2. The constitutive relation at the fluid-solid interface. It has been observed that the
standard no-slip boundary condition no longer holds in a region near the contact
line [24, 25, 31, 32, 33, 38, 40]. This region is the slip region. Linear friction law at
the surface gives rise to the Navier boundary condition. However, nonlinear effects
might be important there.
3. The constitutive relation at the contact line, which determines the relationship
between the contact angle and the contact line velocity. One obvious possibility
is to set the dynamic contact angle to be the same as the equilibrium contact
angle. However, this effectively suppresses energy dissipation at the contact line.
Dissipative processes at the contact line can be quite important [5]. Therefore in
general, one has t o allow the deviation of the dynamic contact from its equilibrium
value.
To derive the needed constitutive relation, we follow the principles discussed in Chap-
ter 4 and ask the following question: What are the simplest forms of boundary conditions

5.3. THE MOVING CONTACT LINE PROBLEM 233
that are consistent with the second law of thermodynamics?
For simplicity, we will focus on the case of two-dimensional flows. The total (free)-
energy of the system is given by
E =
X
i=1,2
1
2
Z
Ω
i
ρ
i
|u|
2
dx +
X
i=1,2,3
γ
i
|Γ
i
|. (5.3.9)
A straightforward calculation gives
dE
dt
= −
X
i=1,2
Z
Ω
i
η
i
|∇u|
2
dx +
X
i=1,2
Z
Γ
i
u · τ · n dS + γ
3
(cos θ
d
− cos θ
0
) u
CL
, (5.3.10)
where θ
d
is the dynamic contact angle, θ
0
is the static contact angle defined in (5.3.3),
u
CL
is the velocity of the contact line, n is the unit normal vector of the solid surface.
Let u
s
and τ
s
be the slip velocity and the shear stress respectively,
u
s
= (u − u
w
) · t, τ
s
= t · τ · n. (5.3.11)
Let
τ
Y
= γ
3
(cos θ
d
− cos θ
0
) . (5.3.12)
τ
Y
is the so-called unbalanced or uncompensated Young’s stress [5, 12, 32, 33]. It represents
the force on the contact line that arises from the deviation of the fluid interface from its
equilibrium configuration. With these notations, we can rewrite (5.3.10) as
dE
dt
= −
X
i=1,2
Z
Ω
i
η
i
|∇u|
2
dx +
X
i=1,2
Z
Γ
i
u
s
τ
s
dS + u
CL
τ
Y
(5.3.13)
According to t he second law of thermodynamics, the rate of energy dissipation dE/dt
is necessarily non-positive for arbitrary realizations of u. It follows easily that each of
the three contributions has to be non-positive. We now examine the implication of this
basic requirement and the constraints it places on the form of the constitutive relations.
First, we look at the fluid-solid interfaces Γ
1
and Γ
2
. There we must have
u · n = 0 (5.3.14)
This implies that the shear stress should depend only on u
s
. Assumin g that this depen-
dence is local, then τ
s
is a function of the local values of u
s
and its derivatives. To the
lowest order, we simply have:
τ
s
= f(u
s
) (5.3.15)

234 CHAPTER 5. EXAMPLES OF MULTI-PHYSICS MODELS
Since
R
Γ
i
u
s
τ
s
dS 6 0 (i = 1, 2) for an arbitrary realization of u, the fun ction f must
satisfy wf(w) ≤ 0
Next, we turn to the contact line. From thermodynamic consideration, τ
Y
is neces-
sarily a functional of the contact line velocity u
CL
. The simplest assumption is that it is
a local function of u
CL
:
τ
Y
= f
CL
(u
CL
) (5.3.16)
The function f
CL
has to satisfy uf
CL
(u) ≤ 0.
The Navier-Stokes equation together with the boundary conditions (5.3.6), (5.3.14),
(5.3.15) and (5.3.16) constitute our continuum model for the moving contact line problem.
The constitutive relations in (5.3.15) and (5.3.16) are not yet fully specified. The exact
functional dependence of f and f
CL
has to be obtained through other means. For this
continuum model, the energy dissipation relation has a very clean form:
dE
dt
= −
X
i=1,2
Z
Ω
i
η
i
|∇u|
2
dx +
X
i=1,2
Z
Γ
i
u
s
f(u
s
) dS + u
CL
f
CL
(u
CL
) (5.3.17)
The three terms at the right hand side represent the energy dissipated in the bulk fluids,
the solid surface and the contact line, respectively.
So far, we have followed the standard procedure in generalized thermodynamics or
non-equilibrium thermodynamics [13, 30]. At this point, we have two options. One is to
continue following the standard procedure and write down linear relations between the
generalized fluxes (here the u
s
and u
CL
) and the generalized forces (here the τ
s
and τ
CL
).
The other is to use microscopic models to measure f and f
CL
directly.
If we continue to follow the standard procedure in non-equilibrium thermodynamics,
then the next step is to propose linear constitutive relations of the form (see Section 4.1):
τ
s
= −β
i
u
s
, i = 1, 2 (5.3.18)
and
γ
3
(cos θ
d
− cos θ
0
) = −β
CL
u
CL
(5.3.19)
β
i
, i = 1, 2 and β
CL
are the friction coefficient at the solid surface for the two fluid phases
and the contact line, respectively. Equation (5.3.18) is the well-known Navier boundary
condition. Putting things together, we obtain the following continu um model for the
moving contact line problem:
5.3. THE MOVING CONTACT LINE PROBLEM 235
1. In the bulk, we have
ρ
i
(∂
t
u + (u · ∇)u) = −∇p + µ
i
∇
2
u + f
∇ · u = 0
(5.3.20)
2. The fluid interface Γ is advected by the velocity field, and
n · [τ] n = γ
3
κ
t · [τ ] n = 0
(5.3.21)
where τ = −pI + µ(∇u + ∇u
T
) is the stress tensor; κ is the mean curvature of the
interface.
3. On the solid surface, we have
β
i
u
s
= t · τn, u · n = 0 (5.3.22)
4. At the contact line, we have
β
CL
u
CL
= −γ
3
(cos θ
d
− cos θ
0
) . (5.3.23)
This is the model put forward by Ren and E [33].
Some remarks about the physical significance of the parameter β
CL
are in order:
1. It is a three-phase friction coefficient.
2. It has th e dimension of viscosity. This should be contrasted to β
i
which has
the dimension of viscosity/length and this length has to be a microscopic length.
Conequently, the Navier slip boundary condition is only significant in a slip region
of microscopic size. In comparison, β
CL
should be of macroscopic significance.
3. It also arises in the sharp interface limit of the diffusive interface model proposed
in [23, 31, 32], as was demonstrated in [44].
One can write (5.3.23) as
cos θ
d
− cos θ
0
= −(β
CL
/γ
3
)u
CL
(5.3.24)

236 CHAPTER 5. EXAMPLES OF MULTI-PHYSICS MODELS
This can be viewed as a kinetic correction to the condition
θ
d
= θ
0
(5.3.25)
(5.3.25) states that the dynamic contact angle is equal to the equilibrium contact angle.
This is the analog of the Gibbs-Thomson condition in the theory of crystal growth [19]. In
this regard, (5.3.24) is the analog of the corrected Gib bs-Thomson relation with kinetic
effects taken into account [19].
It is interesting to note that if the condition (5.3.25) is used together with the Navier
boundary condition, then the Huh-Scriven singularity is removed, b ut there is still a weak
singularity [37]. Naturally one expects that if we use (5.3.24) instead of (5.3.25), then
this weak singularity is also removed. This is still an open question.
Since any function is approximately linear in a small enough interval, these linear
constitutive relations will be adequate in appropriate regimes. What is important is how
big these regimes are and what happens outside of them. To answer these questions, we
resort to microscopic models.
5.3.3 Measuring the boundary conditions using molecular dy-
namics
Thermodynamic consideration tells us what the boundary conditions should depend
on, but not the detailed functional dependence. This latter information should be ob-
tained from experiments, or, as we explain now, numerical experiments using microscopic
simulations, such as molecular dynamics.
The results of the previous section tell us that f and f
CL
depend on the slip velocity.
Therefore, we need to set up an MD system such that, at the statistical steady state, it
has a constant slip velocity. This can be done using a MD system in the Couette flow
geometry, shown in Figure 5.7, where the fluid particles are confined between two solid
walls, and the walls move in opposite directions at a constant speed U [24, 31, 33, 38, 39].
A standard way of modeling immisible fluids is to use a modified form of the Len nard-
Jones (LJ) potential:
V
LJ
(r) = 4ε
σ
r
12
− ζ
σ
r
6
(5.3.26)
and use ζ = 1 for particles of the same species and ζ = −1 for particles of different species.
Different values of ε can be used to model the fluid-fluid and fluid-solid interaction
