Voit J. The Statistical Mechanics of Financial Markets

Подождите немного. Документ загружается.

8.3 Computer Simulation of Market Models 241

being strongly non-Gaussian and nonstable, cf. Chap. 5, such as a truncated

L´evy distributions, or a power-laws with non-stable exponents

p(∆S) ∼|∆S|

−(1+µ)

exp(−a|∆S|)orp(∆S) ∼|∆S|

−4

(8.24)

is solid evidence against an independent agent approach.

The inﬂuence of communication and herding alone can best be studied

by focusing on an even simpler model: percolation, as proposed by Cont and

Bouchaud [190]. Agents again have three choices of market action: buy, sell,

or inactive, as in Iori’s model. They can form coalitions with other agents

who share the same opinion, i.e., choice of action. N agents are assumed to

be located at the vertices of a random graph, and agent i is linked to agent

j with a probability p

. A coalition is simply the ensemble of connected

agents (a cluster) with a given action ∆Φ

. This, of course, precisely deﬁnes

a percolation problem [184].

Agents in a cluster share the same opinion and do not trade among

themselves. They issue buy and sell orders to the market with probabili-

ties P (∆Φ

=+1)=P (∆Φ

= −1) = a, and remain out of the market with

P (∆Φ

=0)=1− 2a. a is the traders’ activity, and for a<1/2, a fraction

of traders is inactive. If all p

= p, the average number of agents, to which

one speciﬁc agent is connected, is (N −1)p. In order to solve the model, one

is interested in the limit N →∞. In this limit, (N −1)p should remain ﬁnite

so that the probability of a link scales as p = c/N. Finally, price changes are

assumed to be proportional to the excess demand

∆S ∝



∆Φ

. (8.25)

Random graph theory now makes statements on the sizes W of clusters

in the limit N →∞[190]. When c = 1, there is a power-law distribution of

cluster sizes in the large-size limit

p(W ) ∼ W

−5/2

for W →∞. (8.26)

For c slightly below unity (0 < 1 −c  1), the power law is truncated by an

exponential

p(W ) ∼ W

−5/2

exp



(c − 1)W



for W →∞. (8.27)

For c = 1, variance and kurtosis are inﬁnite. They become ﬁnite but large

when c<1. Notice the similarity of (8.27) to the truncated L´evy distributions

with µ =3/2, discussed earlier. When c is close to unity, an agent forms a

link with one other agent, on the average. Larger clusters can still form from

many binary links.

The law of price variations can be calculated in closed analytical form

[190]. In the limit where 2aN is small, i.e., most of the traders are inactive,

242 8. Microscopic Market Models

it reduces to (8.26) or (8.27), depending on the value of c, with the replace-

ment W → ∆S. From this model, one would therefore predict that the L´evy

exponent µ =3/2, which is close to the empirical results discussed in Chap.

5, should be universal.

In terms of percolation theory, the model formulated by Cont and Bou-

chaud [190] is in the same universality class as Flory–Stockmayer percolation

[191]. Numerical simulations, however, become easier when the random graph

underlying the Cont–Bouchaud model is replaced by a regular hypercubic

lattice. On such lattices, critical behavior with the functional forms of cluster

sizes similar to (8.26) and (8.27) is obtained, but the exponent in the power

laws generally diﬀers from 5/2. Only for lattices in more than six dimensions

is the 5/2-power law recovered [184].

Stauﬀer and Penna performed extensive Monte Carlo simulations of such

percolation problems [191]. They veriﬁed the power-law scaling of the prob-

ability distribution function of price changes, and its exponential truncation,

on hypercubic lattices from two to seven dimensions if the activity a of the

traders was chosen suﬃciently small. They were also able to show that a

crossover to more Gaussian return statistics occurred in the Cont–Bouchaud

model when the activity a was increased, at least on hypercubic lattices.

The Cont–Bouchaud model can be extended further to include interac-

tions between the coalitions of traders (percolation clusters), and the inﬂu-

ence of fundamental analysis [192]. To this end, one replaces the percolation

clusters by superspins Σ

. A superspin is a spin with variable magnitude.

This magnitude is the size of the original percolation clusters, and can be

drawn from an appropriate distribution function, such as (8.26), eventually

with a free exponent µ. In spin language, the excess demand on the market is

equivalent to the magnetization of the superspin model, and the price changes

are proportional to it. If the spin magnitudes are drawn from a power-law

distribution without truncation, one can show that the distribution of mag-

netization, and with it the distribution of returns, carries the same power

laws as the spin size distribution, if its L´evy exponent was µ<2.

Ferromagnetic interactions between the superpsins then correspond to

herding behavior of the coalitions of traders in the Cont–Bouchaud model

[192]. In practical terms, one might think of the managers of mutual funds

imitating their colleagues’ behavior. This is modeled by an “exchange inte-

gral” J

, in the same way as in the ﬁrst term of (8.18). The local energy

= −



j=i

(8.28)

is a measure of the disagreement of trader i with the prevalent opinion.

Conformism leads to energy minimization. If one assumes the same J

= J

between all spins, a ferromagnetic state results in the physics version, and a

boom or crash in the ﬁnance version of the model. This is unrealistic. One

way to avoid such a totally ordered state is through the introduction of a

8.3 Computer Simulation of Market Models 243

ﬁctitious temperature T which, when suﬃciently high, returns the system to

its paramagnetic state. In this case, the expected return is zero. This still

gives power-law price statistics, and can be mapped onto an equivalent zero-

temperature, zero-interaction model.

The agents so far behaved as noise traders, i.e., their opinions were ran-

domly chosen. In this superspin variant, one can also include opinions which

might arise from fundamental analysis of a company [192]. This can be done

by a (coalition dependent) random ﬁeld h

(t) which introduces a bias into

the spin energy. Equation (8.28) is then changed to

= −



j=i

+ Σ

. (8.29)

Such a ﬁeld must be time-dependent, too. Assume that there is a certain stock

price justiﬁed from fundamental analysis. If the actual price is much higher

than the fundamental price, there should be a bias towards selling. When

the price falls suﬃciently below the fundamental price, a buying bias must

arise. If this scheme is implemented, however, in a model with interactions,

ﬁnite temperature, and a 50% population of agents with a fundamental bias,

the price changes become quasiperiodic, and a bimodal return distribution

curve is found. As a consequence, either bubbles and crashes on real markets

are caused by much less rational behavior than included in the model [192], or

the herding eﬀect between the coalitions of traders has been overemphasized.

Adaptive Trader Populations

In the models discussed above, there was either just one population of traders

with heterogenous trading strategies (random number parameters), or there

were two or more populations of traders with diﬀerent strategies (rebalancers,

portfolio insurers, noise traders, fundamentalists, etc.). In all cases, these

populations were ﬁxed from the outset, and traders were not allow to change

camp when they saw that their competitors’ strategies were more successful.

Changing camp, however, is certainly an important feature of herding in real

ﬁnancial markets where the operators often use a variety of analysis tools to

reach their investment decisions, and the inﬂuence of the various tools on the

decisions may well change with time.

Strategy hopping is at the center of a model formulated by Lux and

Marchesi [193]. It had also been included in a simulation by Coche, of a

more realistic model with much higher complexity [177] which we do not

discuss here in detail. In the Lux–Marchesi model, traders are divided into two

groups: fundamentalists and noise traders. Fundamentalists use exogeneous

news arrival, modelled by geometric Brownian motion for a “fundamental

price” S

(the returns are normally distributed and the prices are drawn from

a log-normal distribution). An example of this process is shown in Fig. 8.10.

Noise traders, on the other hand, rely on chart analysis techniques and the

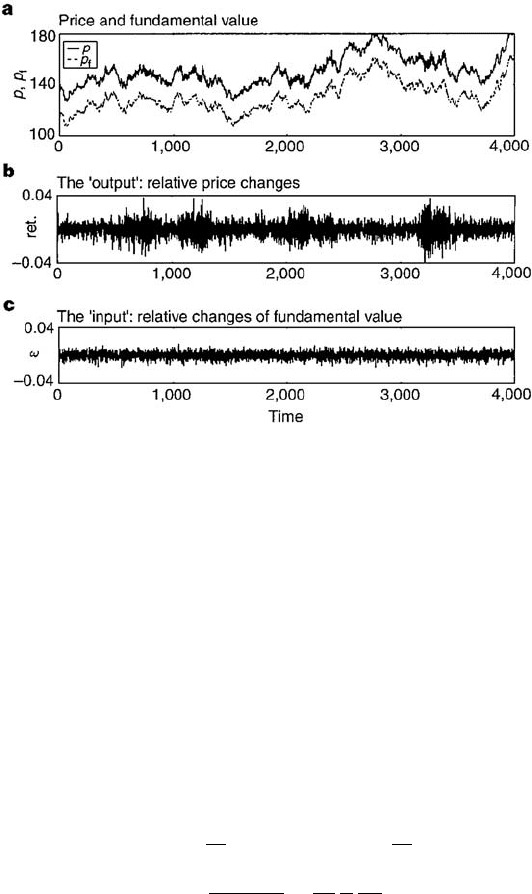
244 8. Microscopic Market Models

Fig. 8.10. Simulation of the Lux–Marchesi model. Panel (a) shows the history of

the fundamental prices (S

of our text is denoted p

here), and of the actual share

prices (S in the text is p here). Both price series have been oﬀset for clarity. There is

thus no long-term diﬀerence between both series, and the model market is eﬃcient

in the long run. Panel (b) displays the return on the share, while panel (c)isthe

return process of the fundamental price. Notice the very diﬀerent return dynamics

of both time series. By courtesy of T. Lux. Reprinted by permission from Nature

397, 498 (1999)

1999 Macmillan Magazines

behavior of other traders as information sources. Moreover, noise traders are

divided into an optimistic and a pessimistic group. When the share price

rises, optimistic noise traders will buy additional shares while pessimistic

noise traders will start selling.

The important feature of this model is the possibility for strategy change

by the traders. Noise traders change between optimistic (+) and pessimistic

(−) with rates

−→+

= ν

,π

+→−

= ν

−U

with U

= α

− n

−

. (8.30)

Here, N = n

+ n

is the total number of traders, and n

= n

+ n

−

is the

number of noise traders of optimistic (n

) or pessimistic (n

−

) opinion. n

the number of fundamentalist traders. The ﬁrst term in the utility function

measures the majority opinion among the noise traders, and the second

term measures the price trend. ν

and α

1,2

are the frequencies of reevaluation

of opinions and price movements. If both signals, majority opinion and price

8.3 Computer Simulation of Market Models 245

movement, go in the same direction, a strong population change will take

place. If they point in opposite directions, the migration between the two

noise trader subgroups will be much less pronounced.

Switching between the noise trader and fundamentalist group is driven

by the diﬀerence in proﬁts of both groups. Four rates are needed because of

the two subgroups of noise traders

f→+

= ν

2,1

,π

+→f

= ν

−U

2,1

f→−

= ν

−

2,2

,π

−→f

= ν

−U

2,2

. (8.31)

denotes the number of fundamentalists, and ν

the reevaluation frequency

for group switching. The utility functions here are more complicated because

the proﬁts of both groups are diﬀerent. The fundamentalists’ proﬁt is given

by the deviation of the stock price from its fundamental price, but the proﬁt

is realized only in the future when the stock price returns to the fundamental

value. They must be discounted therefore with a discounting factor q<1, and

are given by q|S − S

|/S. The proﬁt of the optimist chartists is given by the

excess return of dividends (D) and share price changes ν

−1

dS/dt per asset,

over the average market return R. The proﬁt of the pessimistic chartists is

just its negative, and is realized when prices fall after assets have been sold.

The utility functions U

2,1

and U

2,2

then become

2,1

= α



D +

− R − q



S − S



2,2

= α



R −

D +

− q



S − S



. (8.32)

The order size of the noise traders is assumed to be the average transac-

tion volume, and their contribution to the excess demand is then proportional

to the diﬀerence between optimistic and pessimistic noise traders. The fun-

damentalists, on the other hand, will order in proportion to the perceived

deviation of the actual stock price from its fundamental price, and the total

excess demand is just the sum of both contributions. In the Lux–Marchesi

model, the price changes are not deterministic but are given by probabilities

which depend on the excess demand [193].

An interesting result of the simulations of this model is that, on the av-

erage, the market price equals the fundamental value [193]. This is shown

in panel a of Fig. 8.10 where both price series have been oﬀset for clarity.

In the long run, the model market is eﬃcient, and there are no persistent

deviations of the share price from its fundamental value. As is apparent from

panels b and c, however, the return processes of input and output are very

diﬀerent. The input process for the news arrival is geometric Brownian mo-

tion. The output process exhibits much stronger ﬂuctuations, and volatility

246 8. Microscopic Market Models

clustering. When the statistics of the return process of the share price is an-

alyzed, one ﬁnds fat tails with power-law decay when the time scale of the

returns is one time step. The exponent of the power law has been estimated

as µ ≈ 2.64 ±0.077, but this may vary with parameters [193]. This value of µ

compares favorably with the empirical studies discussed in Sect. 5.6.1. When

the time scale is increased, the probability for large events decreases more

steeply, and shows evidence for crossover to a Gaussian, again in agreement

with what is found in real markets. Also, there are long-time correlations in

the absolute returns of the shares, and volatility clustering.

The driving force of the model performs geometric Brownian motion. The

peculiar scaling behavior found in the output stochastic process therefore

must be the result of the interactions among the agents [193]. Analysis of the

simulations shows that big changes of volatility are caused by the switching

of traders between the various groups. The volatility is usually high when

there are many noise traders. When the fraction of noise traders exceeds

a critical value, the system becomes unstable. However, the action of the

fundamentalists who can make above-average proﬁts from such situations

soon brings back the system into a stable regime. This mechanism is rather

similar to the phenomenon of intermittency in turbulence.

The preceding discussion has emphasized the variety of mechanisms con-

tributing to the price dynamics of real ﬁnancial markets: herding, fundamen-

tal analysis, portfolio insurance, technical analysis, rebalancing portfolios,

threshold trading, dynamics of trader opinions, etc. Every model discussed

contained a unique mix of these factors, and emphasized diﬀerent aspects of

real markets. In the future, it will certainly be important to quantify more

precisely the inﬂuence of the individual factors in speciﬁc markets. It is con-

ceivable, e.g., that the role of fundamental analysis is diﬀerent in stock, bond,

and currency markets. A ﬁrst step towards more quantitative investigation

of markets by computer simulation of simpliﬁed models will be a careful cal-

ibration against a minimal set of market properties, such as those discussed

in Chap. 5.

8.4 The Minority Game

Game theory deals with decision making and strategy selection under con-

straints. Game theory as applied by economists is built on one standard

assumption of economics – that agents behave in a rational manner. Loosely

speaking, agents know their aims, and what are the best actions to achieve

them. The non-trivial problem comes from constraints, and conﬂicting though

structurally similar behavior of the other agents. This assumption of ratio-

nality eliminates randomness from the games, and makes them essentially

deterministic. In this perspective, games involve an optimization problem.

The beneﬁts of a player are often described by a utility function which, of

course, depends on the strategies of all players. Under the assumption of

8.4 The Minority Game 247

complete information sharing between all players, the solution of the game

is a Nash equilibrium. A Nash equilibrium is a state which is locally optimal

simultaneously for each player, e.g., a local maximum of all utility functions.

In a physics perspective, such Nash equilibria in deterministic games

might be viewed as zero-temperature solutions, where all possible (classical)

ﬂuctuations are frozen [194]. Introducing ﬂuctuations, or randomness, then

would correspond to ﬁnite-temperature properties. Depending on the impor-

tance of ﬂuctuations, the properties of a ﬁnite-temperature system may or

may not be close to those of its zero-temperature solution.

In the presence of randomness, games, in essence, will turn into scenario

simulations. One may wonder to what extent game theory can improve our

understanding of ﬁnancial markets. Financial markets certainly provide the

basic ingredients of game theory: a common goal and the necessity of strategy

selection and decision making under constraints. However, uncertainty is an

essential feature of capital markets, and while some information is available at

high frequency and quality, the information on the strategies of other players

is very limited, and can be guessed at best. In this section, we will explore a

very simple game where agents have to make decisions using strategies chosen

from a given set. They are selected based on their perceived historical per-

formance using the available common information. Players however do not

know their fellows’ strategies. While the models grown from this seed have

evolved some way towards the market models discussed above, the emphasis

is diﬀerent. Before, each agent either operated according to a random ﬁxed

strategy, or stochastically switched strategy according to an indicator func-

tion. Here, the question is how to select winning strategies for the agents in a

market, possibly by simple deterministic rules despite the randomness present

in the game, and how the stylized facts of ﬁnancial markets may be generated

by the interplay of agents with heterogeneous strategies. This process may

be closer to real life where often strategies are selected and switched on a

trial-and-error basis.

8.4.1 The Basic Minority Game

Take a population of an odd number N

of players, each with a ﬁnite number

of strategies, N

. At every time step, every player must choose one of two

alternatives, ±1, buy or sell, attend a bar or stay at home, etc. without

knowing the choices of the other players [195, 196]. To be speciﬁc, we take

binary digits, and the decision of player i at time t is denoted by a

(t). The

rule then is to reward those players on the minority side with a point. The

winner is the player with the maximal number of points.

The time series of 0 or 1 is available to all players as common information.

A strategy of length M is a mapping of the last M bits of the time series of

results into a prediction for the next result, e.g., for M = 3 it maps the eight

3-bit signals into a set of eight predictions

248 8. Microscopic Market Models

⎧

⎨

⎩

⎛

⎝

−1

⎞

⎠

⎛

⎝

−1

⎞

⎠

⎛

⎝

−1

⎞

⎠

⎛

⎝

−1

⎞

⎠

⎛

⎝

−1

⎞

⎠

⎛

⎝

−1

⎞

⎠

⎛

⎝

−1

⎞

⎠

⎛

⎝

⎞

⎠

⎫

⎬

⎭

→{1, −1, −1, 1, 1, 1, −1, 1}

. (8.33)

The “history” h(t) is the signal broadcast to all players at a given instant of

time, i.e., the last M outcomes of the game. Agents react to information, and

they modify this information through their own actions. Diﬀerent strategies

are distinguished by the diﬀerent predictions from the same signals. There

are 2

signals of M bits, and two possible predictions for each signal. The

space of strategies of length M therefore is of size 2

(= 256 for M =3).

M is an indicator of the memory capacity of the agents. When the number

of strategies available to a player N

 2

, very few strategies will be used

by two or more players. On the other hand, when the inequality is violated,

many players will have a common reservoir of strategies, and only very few

strategies will not be available to another player.

At every turn of the game, the players evaluate the results of all their

strategies on the outcome of the game, and assign a virtual point to all

winning strategies, no matter if the strategy actually used in the game was

among them (in which case the player won a real point) or not. At every time

step, the player uses that strategy from his set which features the highest

number of virtual points, i.e., which would have been his most successful

strategy based on the historical record. The game is initialized with a random

strategy selection [196]. All agents enter the game with the same weight, i.e.,

there are no rich agents who can invest much, and no poor agents who only

can invest small sums.

To understand the outcome of the game, consider two extreme situations.

One possible result is that only one player selects one side, and all the re-

maining N

− 1 players take the other one. In this simple game, a single

point is awarded in this turn of the game, to the winning player. The other

extreme is an almost draw, when (N

− 1)/2 players take the minority side

and (N

+1)/2 players form the majority. In this case, (N

−1)/2pointsare

awarded. If one imagines the points to come from a reservoir, the second case

would be interpreted as a very eﬃcient use of resources by the player ensem-

ble (they gather the maximal number of points to be gained in a single trial)

whereas the ﬁrst result would imply a huge waste. Clearly, this is opposite to

a lottery where a ﬁxed amount of money is distributed to the winners, and a

lonely winner would gain much more than a winner in a large crowd.

The record of the game then is the time series of actions

A(t)=



i=1

(t) . (8.34)

8.4 The Minority Game 249

Points are awarded to all players with a

(t)=−sign A(t). When the game

is simulated, the time series A(t) oscillates rather randomly around 0. The

variance of the time series is high when M is small, and vice versa. In the

interpretation suggested before, players with larger memories then would

better use the available resources because, as an ensemble, they would score a

higher number of points on the average. Remarkably, this behavior is achieved

by selﬁsh players who only search to optimize their own performance.

Is there an optimal strategy for an individual player in this game? For the

ensemble of players, the optimal score is (N

− 1)/2 per turn. The maximal

average gain per trader and per game therefore is 1/2. Can this gain be real-

ized by an individual player with a simple strategy, systematically choosing

one side, say a

(t) = 1? If this were the case indeed, then other players would

be attracted to make similar choices, too, because those of their strategies

predicting an outcome 1 on a given signal would accumulate more virtual

points. Then, however, the prediction 1 would quickly become a majority

action, and not win points any longer. Notwithstanding these ﬁndings, in

every game, there are players with success rates higher than 1/2. When the

number of strategies N

of each player increases, the success rate decreases.

Players more often switch strategies and face more diﬃculties in identifying

outperforming strategies in their pool. Quite generally, the less players switch

strategies, the higher their success rates.

However, strategies are good or bad only on a given time horizon. When

the virtual points of all strategies are analyzed, the distribution at short times

is rather wide, indicating that there is a big spread between good and bad

strategies. As time increases, the distribution shrinks. This tells us that, on

the long run, all strategies become equal. Success or failure then is linked to

the good or bad timing in the use of speciﬁc strategies [196].

8.4.2 A Phase Transition in the Minority Game

The standard deviation of A(t) (volatility) displays a very interesting be-

havior as the memory size of the agents is varied [197]. For small M,the

volatility



var A(t) (8.35)

decreases steeply from high values when the memory size M of the agents in-

creases. σ

increases gently with M from rather low values, beyond a critical

memory size. Opposite behavior is found as a function of N

, i.e., the eﬀective

parameter for the transition is α =2

, the information complexity per

player. The critical memory size increases with the number of strategies N

available to each player. With reference to the extreme situations discussed

above, the highly volatile low-α (low M, large N

) regime describes a “sym-

metric” (the meaning will become clear in Sect. 8.4.4) information-eﬃcient

phase. This phase is named “crowded” because, due to the limited number

of strategies available, the “crowding” of several players on one strategy is

250 8. Microscopic Market Models

likely [197]. The more players present in the game at constant memory size,

(i.e., size of the strategy space) or the smaller the agent memory, i.e., infor-

mation, at constant number of players, the more likely this crowding eﬀect

is. Also, many of the strategies available are actually used, and information

is processed eﬃciently. On the other hand, in the “dilute” large-α (large M,

small N

) phase, the strategy space is huge, and it is extremely unlikely that

two agents will use the same strategy. This phase is termed “asymmetric”

(cf. below), and information is not used eﬃciently: many strategies remain

unexplored.

An interesting explanation can be given for these ﬁndings in terms of

crowding eﬀects [197]. Suppose that there is a speciﬁc strategy R used by

agents, who thus act as a crowd. For each strategy R, there is an anticor-

related strategy

R where all predictions are reversed. The N

agents using R

form the anticrowd. R and

R form a pair of anticorrelated strategies. Pairs of

strategies are uncorrelated. When N

≈ N

, the actions of the crowds and

of the anticrowds almost cancel, and σ

will be small. On the other hand,

when N

 or  N

, herding dominates and generates a high volatility.

It turns out that the behavior of the volatility is almost unchanged when

a reduced strategy space made up only of pairs of anticorrelated strategies

is used [197]. Diﬀerent pairs being uncorrelated, the signal A(t)canbede-

composed into the contributions of the various groups. Each of these groups

essentially performs a random walk of step size |N

− N

|. The variance of

these walks then determines the standard deviation σ

, and it turns out that

the extent of crowd–anticrowd cancellation determines the non-monotonic

variation of the volatility.

In terms of this crowd–anticrowd picture, the asymmetric large-α phase

corresponds to N

, N

∈{0, 1}. Strategies are either selected once, or not at

all. The volatility is almost that of a discrete random walk with unit step size.

When more agents play, the simultaneous use of R and

R will become more

likely, giving cancellations, i.e., zero step size in the random walk, and σ

decreases. With even more players, the crowd sizes on R and R will become

sizable but likely very diﬀerent. The step size of the random walk will grow,

as does the volatility. The behavior of σ

can thus be interpreted in terms

of repulsion, attraction, and incomplete screening of crowds and anticrowds

[197].

8.4.3 Relation to Financial Markets

In the basic form described above, the minority game shares some features of

ﬁnancial markets. Agents have to take choices under constraints, uncertainty,

and with limited information. The most fundamental decision in a ﬁnancial

market is binary: buy or sell. Speculators may ﬁnd their strategies by trial and

error, and their strategy pool may be limited. There is competition in the mi-

nority game as in markets, and agents cannot win all the time. Furthermore,

there is no a priori deﬁnition of good behavior in markets. Good behavior