Stewart J. College Algebra: Concepts and Contexts

Подождите немного. Документ загружается.

164 CHAPTER 2

■

Linear Functions and Models

(d) If Ingrid downgrades to a plan of $30 a month, how does that change the graph of C?

(e) If Ingrid chooses the $100 cell phone, how does that change the graph of C?

Month

123

Monthly cost

Month

100

200

300

123

Total cost

55. Weather Balloon Weather balloons are filled with hydrogen and released at various

sites to measure and transmit data such as air pressure and temperature. A weather

balloon is filled with hydrogen at the rate of

/s. Initially, the balloon has of

hydrogen. Find a linear function that models the volume of hydrogen in the balloon

after t seconds.

56. Filling a Pond A large koi pond is filled from a garden hose at the rate of 10 gal/min.

Initially, the pond contains 300 gallons of water. Find a linear function that models the

volume of water in the pond (in gallons) after t minutes.

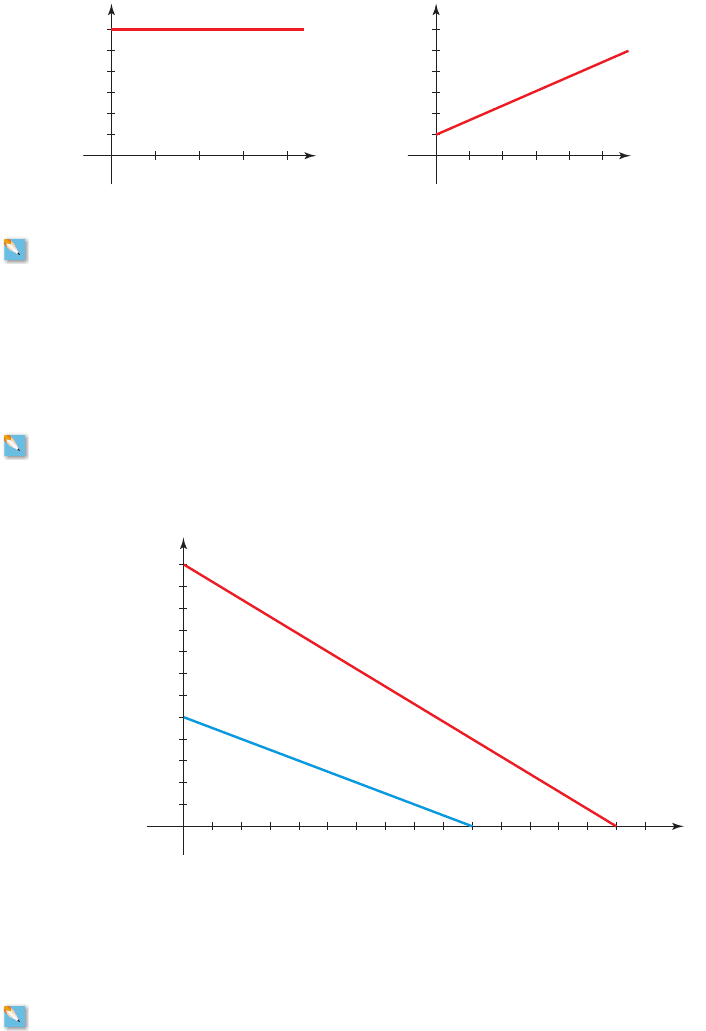
57. Mountain Biking Meilin and Brianna are avid mountain bikers. On a spring day they

cycle down straight roads with steep grades. The graphs give a representation of the

elevation of the path on which each of them cycles. Find the grade of each road.

2 ft

0.5 ft

200

400

600

800

1000

1200

2000 4000 6000 8000

Brianna

Meilin

Horizontal distance

(

)

Elevation

(ft)

10,000 12,000 14,000 16,000

58. Wheelchair Ramp A local diner must build a wheelchair ramp to provide handicap

access to their restaurant. Federal building codes require that a wheelchair ramp must

have a maximum rise of 1 inch for every horizontal distance of 12 inches. What is the

maximum allowable slope for a wheelchair ramp?

59. Commute to Work Jade and her roommate Jari live in a suburb of San Antonio,

Texas, and both work at an elementary school in the city. Each morning they commute

to work traveling west on I-10. One morning Jade left for work at 6:50

A.M., but Jari left

10 minutes later. Both drove at a constant speed. The following graphs show the

distance (in miles) each of them has traveled on I-10 at time t (in minutes), where t ⫽ 0

is 7:00

A.M.

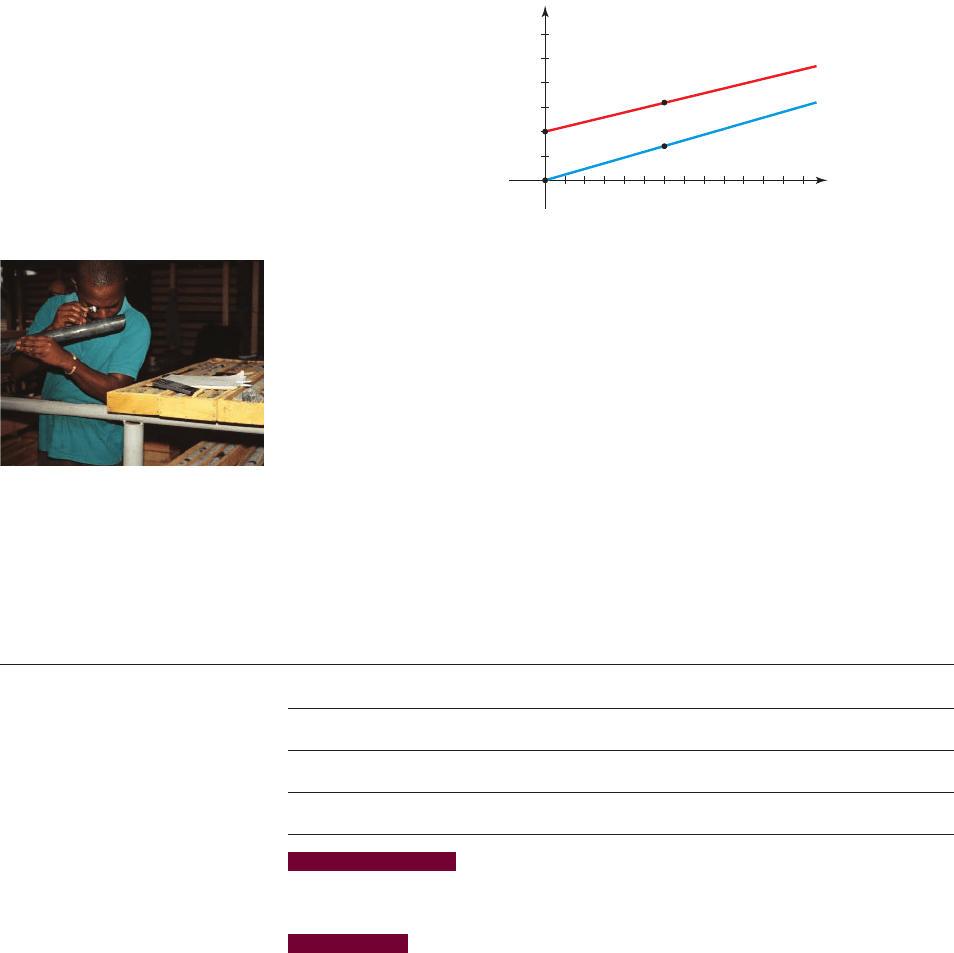
SECTION 2.3

■

Equations of Lines: Making Linear Models 165

(a) Use the graph to decide which of them is traveling faster.

(b) Find the speed at which each of them is driving.

of t.

60. Sedimentation Rate Geologists date geologic events by examining sedimentary

layers in the ocean floors. For instance, they can tell when an ancient volcano erupted

by noting where in the sedimentary layers its ash was deposited. This is possible

because the rate of sedimentation is assumed to be largely constant over geologic eras,

so the depth of the sedimentation is modeled by a linear function of time. To examine

sedimentary strata, core samples (often many meters long) are drilled from the ocean

floor, then brought to the laboratory for study. Special features in the layers, including

the chemical composition of the deposits, can indicate climate change or other geologic

events. It is estimated that the rate of sedimentation in Devil’s Lake, South Dakota, is

0.24 cm per year. The depth beneath the lake bottom at which a layer of sediment lies is

a function of the time elapsed since it was deposited.

(a) Find a linear function that models the depth of sediment as a function of time

elapsed.

(b) To what depth must we drill to reach sediment that was deposited 300 years ago?

2468

Jade

Jari

Time since 7:00

A.M.

(

min

)

Distance

traveled (mi)

10 12

(6, 7)

(6, 16)

Examining core samples

2.3 Equations of Lines: Making Linear Models

■

Slope-Intercept Form

■

Point-Slope Form

■

Horizontal and Vertical Lines

■

When Is the Graph of an Equation a Line?

IN THIS SECTION… we find different ways of representing the equation of a line. These

help us to construct linear models of real-life situations in which we know “any two points”

or “a point and the rate of change”of a linear process.

GET READY… by reviewing Section 1.3, particularly how linear models are constructed

using the “rate of change” and “initial value” of a linear process.

In Section 2.2 we learned that the graph of a function with constant rate of change is

a line. We also saw that linear functions are useful in modeling many real-world sit-

uations. To find a linear model, we needed to know the “initial value” and the “rate

of change” (or “y-intercept” and “slope”). But often this particular information is not

available: We might know the rate of change and a particular value but not the initial

value, or we might simply know two values of the linear function.

166 CHAPTER 2

■

Linear Functions and Models

For example, one way to find the speed of a moving car is to use radar to find

the car’s distance at two different times. The two observations can be used to find a

linear function that models the motion of the car (see Example 2).

Our goal in this section is to find different forms for the equation of a line that

will help us in constructing linear models in different real-world situations.

■ Slope-Intercept Form

In this section we express linear functions as equations. Recall from Section 1.5 that

a function such as can be expressed in equation form as ,

where y is the dependent variable and x is the independent variable. In general, a

linear equation in two variables is an equation that can be put into the form

. We recognize this equation from Section 2.2 as the equation of a line

(that is, an equation whose graph is a line) with slope m and y-intercept b. See

Figure 1. This form of the equation of a line is called the slope-intercept form.

y = b + mx

y = 5 + 3xf 1x 2= 5 + 3x

rise

run

(0, b)

figure 1 A line with slope m and

y-intercept b

example

Lines in Slope-Intercept Form

An equation of the line that has slope m and y-intercept b is

y = b + mx

(a) Find an equation of the line with slope 3 and y-intercept .

(b) Put the equation in slope-intercept form.

Solution

(a) Using the slope-intercept form with m ⫽ 3 and , we get

Slope-intercept form

Replace m by 3 and b by

So the slope-intercept form of the equation of the line is .

(b) To put the equation into slope-intercept form, we solve for y.

Given equation

Add 2x

Divide by 3

So the slope-intercept form of the given equation is .

■ NOW TRY EXERCISES 11 AND 17 ■

y =

x +

3 y = 2x + 1

3 y - 2x = 1

y =-2 + 3x

- 2 y =-2 + 3x

y = b + mx

b =-2

3y - 2x = 1

- 2

example

Constructing a Linear Model for Radar

A police officer uses radar to record his distance from a car traveling on a straight

stretch of road at two different times. The first measurement indicates that the car is

Slope-Intercept Form of the Equation of a Line

(The slope-intercept form can also be expressed as .)y = mx + b

350 feet from the officer; half a second later the car is 306 feet from the officer.

Assume that the car is traveling at a constant speed.

(a) Find a linear equation that models the distance y of the car from the police

officer at any time x.

(b) What is the speed of the car?

Solution

(a) Let’s choose the time of the first measurement to be time 0, that is, x ⫽ 0. So

the next measurement is at time . Then from the given data we get the

following two points:

The first of these two points tells us that the y-intercept is 350. Using the two

points together, we get the slope:

ft/s

So the linear equation that models the distance y that the car travels in x sec-

onds is

(b) The slope of the line we found in part (a) is the same as the rate of change of

distance with respect to time; that is, the slope is the speed of the car. So the

speed of the car is

ft/s

The negative sign indicates that the distance between the car and the police

officer is decreasing (because the car is heading in the direction of the officer).

For the purposes of giving the driver a ticket, the speed of the car is 88 feet per

second.

■ NOW TRY EXERCISE 57 ■

We can convert the answer to part (b) of Example 2 into the more familiar miles

per hour. There are 5280 feet in a mile and 3600 seconds in an hour. So

So the car is traveling at a speed of 60 miles per hour.

= 60 mi

= 88 *

3600

5280

miles

hour

feet

seconds

= 88

feet

seconds

* 3600

seconds

hour

5280

miles

feet

speed = slope =-88

y = 350 - 88x

m =

306 ft - 350 ft

0.5 s - 0 s

- 44

0.5

=-88

10, 3502and10.5, 3062

x = 0.5

SECTION 2.3

■

Equations of Lines: Making Linear Models 167

■ Point-Slope Form

We now find the equation of a line if we know the slope of the line and any point

on the line (not necessarily the y-intercept). So suppose a line has slope m and

Marty Bucella/www.CartoonStock.com

168 CHAPTER 2

■

Linear Functions and Models

example

Finding the Equation of a Line When We Know a Point

and the Slope

(x⁄, y⁄)

(x, y)

Run: x-x⁄

Rise:

y-y⁄

figure 2

An equation of the line that passes through the point and has

slope m is

y - y

= m 1x - x

, y

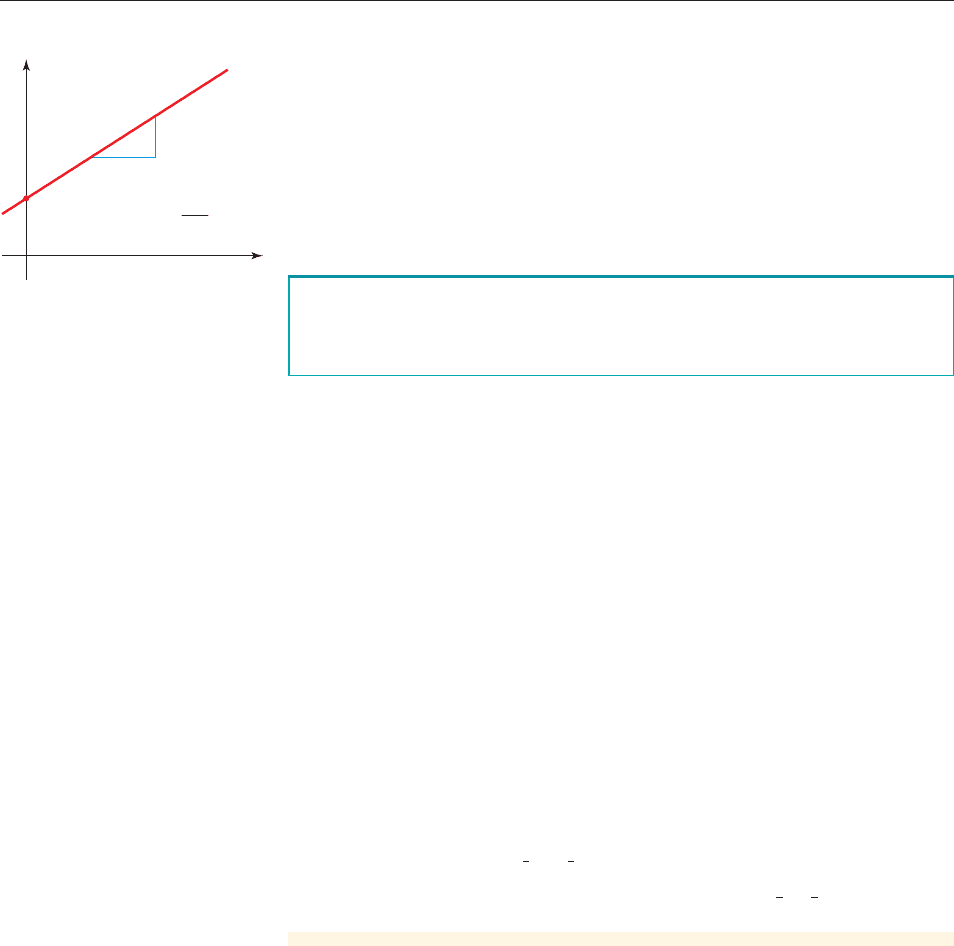
(a) Find an equation of the line through with slope .

(b) Sketch a graph of the line.

Solution

(a) We know the slope m is , and a point on the line is . So we

use the point-slope form with m replaced by , by 1, and by :

Point-slope form

Replace m by by 1, and by

Distributive Property

Subtract 3

So an equation for the line is .

(b) The fact that the slope is tells us that when the run is 2, the rise is , so

when we move 2 units to the right, the line drops by 1 unit. This enables us to

sketch the line as in Figure 3.

■ NOW TRY EXERCISE 23 ■

In the next example we construct a linear model for a real-world situation in

which we don’t know the initial value. Compare it with Example 3 in Section 2.2, in

which we were given the initial value.

- 1-

y =-

x -

y + 3 =-

x +

- 3y

, x

y - 1- 3 2=-

1x - 1 2

y - y

= m 1x - x

- 3y

11, - 321x

, y

11, - 32

Rise: _1

(1, _3)

Run: 2

figure 3

the point is on the line (see Figure 2). Any other point (x, y) lies on the

line if and only if the slope of the line through and (x, y) is equal to m.

This means that

This equation can be rewritten in the form . This is the point-

slope form for the equation of a line.

Point-Slope Form of the Equation of a Line

y - y

= m 1x - x

rise

run

y - y

x - x

= m

, y

example

Constructing a Linear Model for Volume

Water is being pumped into a swimming pool at the rate of 5 gal/min. After 20 min-

utes the pool has 300 gallons of water. Find a linear equation that models the volume

of water in the pool at any time.

SECTION 2.3

■

Equations of Lines: Making Linear Models 169

Solution

We need to find a linear equation

that models the volume y of water in the pool at time x. The rate of change of vol-

ume is 5 gallons per minute, so m ⫽ 5. Since the pool has 300 gallons after 20 min-

utes, the point (20, 300) is on the desired line. So we have the following information

Now, using the point-slope form for the equation of a line, we get

Point-slope form

Replace m by by by 300

Distributive Property

Add 300

So the linear equation that models the volume of water is .

■ NOW TRY EXERCISE 61 ■

In Examples 3 and 4 we were given one point on a line and the slope. If instead

we are given two points on the line, we can still use the point-slope form to find an

equation, because we can first use the two given points to find the slope.

y = 200 + 5x

y - 300 = 5x - 100

20, and y

5, x

y - 300 = 51x - 20 2

y - y

= m 1x - x

Slope:

m = 5

Point:1x

, y

2= 120, 3002

y = b + mx

There are 300 gallons of water in

the pool at time x = 20.

example

Finding an Equation for a Line Through Two Given Points

Find an equation of the line passing through the points and .

Solution

We first use the two given points to find the slope:

We now have the following information about the desired line:

Using the point-slope form for the equation of a line, we get

Point-slope form

Replace m by by by 2

Simplify

Distributive Property

Add 2

So the equation of the desired line is .

■ NOW TRY EXERCISE 31 ■

y =

y - 2 =-

x -

y - 2 =-

1x + 1 2

- 1, y

, x

y - 2 =-

1x - 1- 122

y - y

= m 1x - x

Slope: m =-

Point:1x

, y

2= 1- 1, 2 2

m =

- 4 - 2

3 - 1- 12

- 6

13, - 421- 1, 22

We can use either point, or

, in the point-slope equation.

We end up with the same linear

equation. Try it!

13, - 42

1- 1, 22

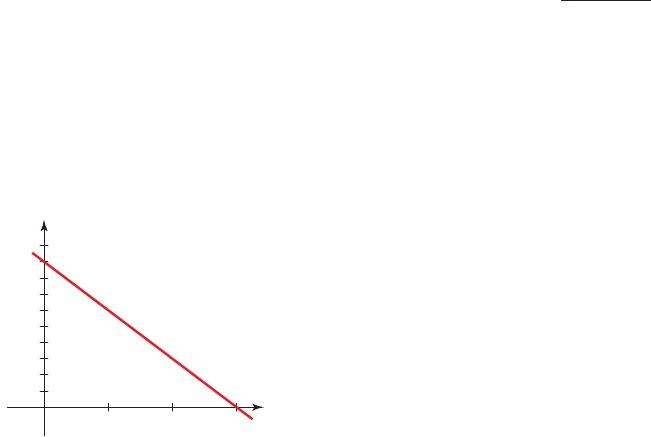
170 CHAPTER 2

■

Linear Functions and Models

example

Constructing a Linear Model for Demand

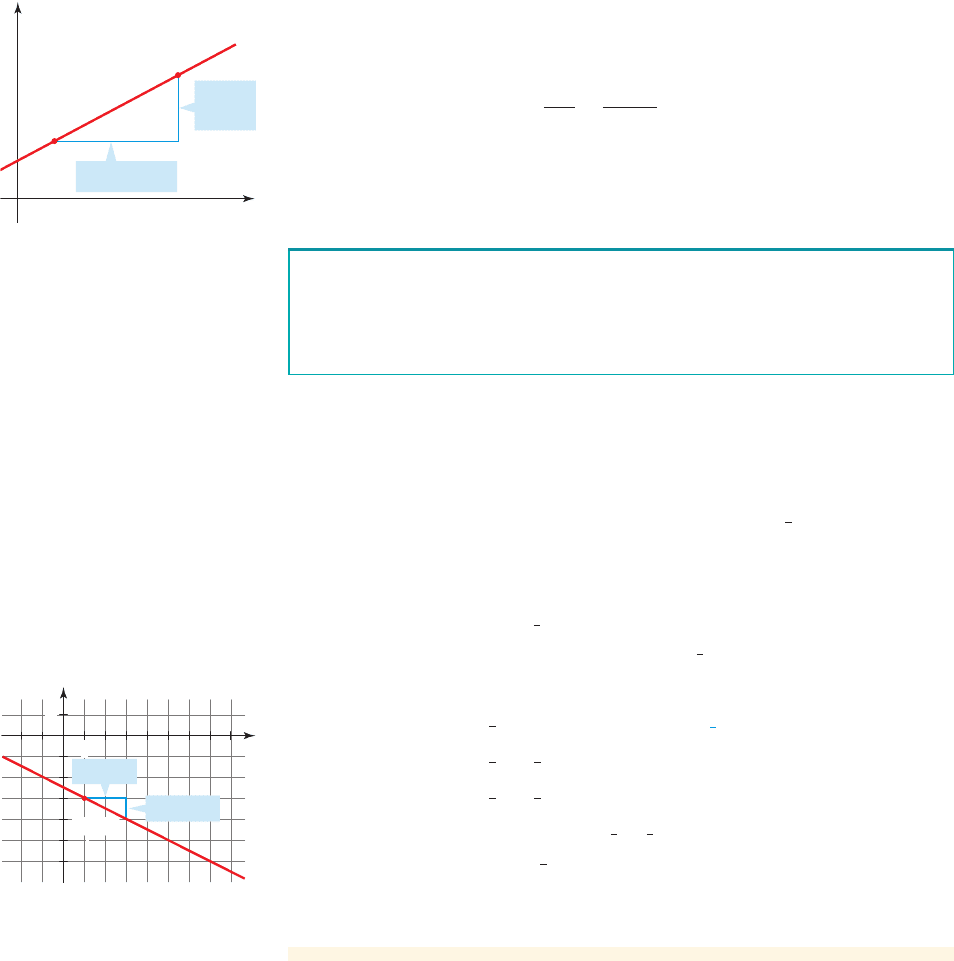
A vending machine operator services the soda pop machines in a freeway rest

area. He finds that the number of cans of soda that he sells each week depends

linearly on the price that he charges. At a price of $1.00 per can of soda he sells

600 cans, but for every 25-cent increase in the price, he sells 75 fewer cans each

week.

(a) Model the number of cans y that he sells each week at price x by a linear

equation (or line), and sketch a graph of this equation.

(b) How many cans of soda will he sell if he charges 60 cents per can? If he

charges $2.00 per can?

(d) Find the y-intercept of the line. What does it represent?

(e) Find the x-intercept of the line. What does it represent?

Solution

(a) Since the number of cans y that the vendor sells depends linearly on the price

x, the model we want is a linear equation:

We already know two points on this line:

This is because when the price is $1.00, the number of cans sold is 600, and

when the price is $1.25, the number of cans sold is 525. From these two points

we obtain the slope of the line:

So we have the following information about the desired line:

Now, using the point-slope form for the equation of a line, we get

Point-slope form

Replace m by by 1, by 600

Distributive Property

Add 600

So the desired equation is . A graph of this equation is shown

in Figure 4.

(b) At a price of 60 cents he sells . At a price of

$2.00 he sells .

increase in price.

- 300

y = 900 - 30012.002= 300 cans

y = 900 - 30010.602= 720 cans

y = 900 - 300x

y =-300x + 900

y - 600 =-300x + 300

- 300, x

y - 600 =-3001x - 12

y - y

= m 1x - x

Slope: m =-300

Point:1x

, y

2= 11, 6002

m =

525 - 600

1.25 - 1

=-300

11, 6002and11.25, 5252

y = b + mx

Notice that the rate of change of y

(the number of cans sold) is

negative. This reflects the fact that

as the price x increases, the number

of cans sold decreases.

123

200

400

600

800

1000

figure 4 Graph of the equation

y = 900 - 300x

SECTION 2.3

■

Equations of Lines: Making Linear Models 171

(d) The y-intercept is 900. This represents the theoretical number of cans he

would “sell” at a price of x ⫽ 0, that is, if he were giving the soda away for

free.

(e) To find the x-intercept, set y ⫽ 0 and solve for x.

Replace y by 0

Add 300x to each side

Divide by 300

So the x-intercept is 3. This means that if he charges $3.00 per can, he will sell

no soda at all.

■ NOW TRY EXERCISE 63 ■

Economists call equations like those in Example 4 demand equations because

they express the demand for a product (the number of units sold) in terms of the

price. With the aid of such equations economists can estimate the optimal price a

vendor should charge to get the maximum profit from sales (see Section 2.7).

x = 3

300x = 900

0 =-300x + 900

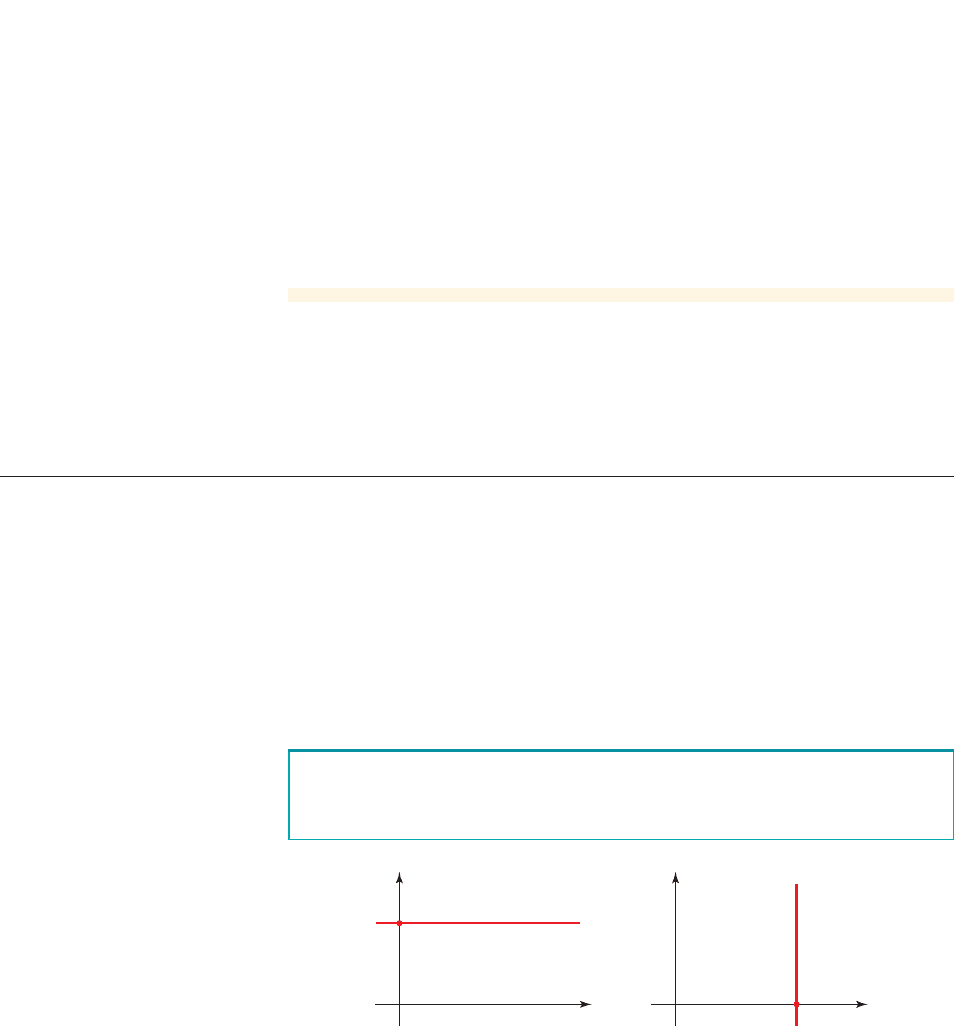
■ Horizontal and Vertical Lines

x=a

y=b

figure 5

■

An equation of the vertical line through (a, b) is x ⫽ a.

■

An equation of the horizontal line through (a, b) is y ⫽ b.

If a line is horizontal, then it neither rises nor falls, which means that between any

two points, the rise is 0 and hence the slope m is also 0. So from the slope-intercept

form of a line we see that a horizontal line has equation . A vertical line does

not have a slope, because between any two points the run is zero, and division by 0

is impossible. Nevertheless, we can write the equation of a vertical line as x ⫽ a,

where a is its x-intercept, because the x-coordinate of every point on the line is a.

(See Figure 5.)

Horizontal and Vertical Lines

y = b

example

Horizontal and Vertical Lines

(a) The graph of the equation x ⫽ 3 is a vertical line with x-intercept 3.

(b) The graph of the equation is a horizontal line with y-intercept .

The lines are graphed in Figure 6 on the next page.

- 2y =-2

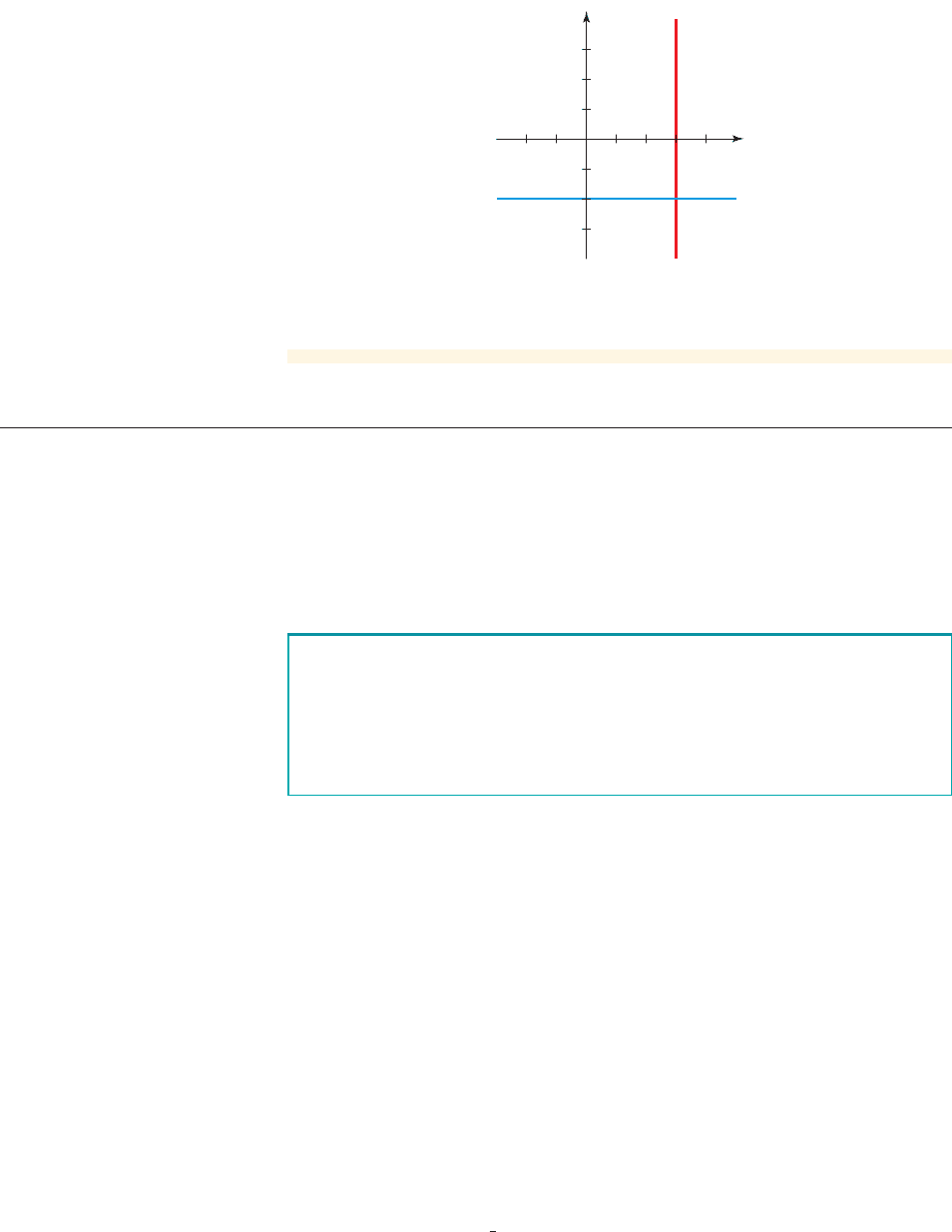
172 CHAPTER 2

■

Linear Functions and Models

x=3

4_2

y=_2

figure 6

■ When Is the Graph of an Equation a Line?

Every line in the coordinate plane is either vertical, horizontal, or slanted at a

nonzero slope m. Thus every line has an equation of the form x ⫽ a, y ⫽ b, or

. All of these equations can be put into the form ,

so this is called the general form of the equation of a line.

Ax + By + C = 0y = b + mx

The graph of every general linear equation

is a line (where not both A and B are 0). Conversely, every line has an

equation of this form.

Ax + By + C = 0

From the general form of a linear equation we can readily find the x- and y-

intercepts and then use them to graph the equation, as illustrated in the next example.

example

Graphing a General Linear Equation

For the linear equation

(a) Find the slope of the line.

(b) Find the x- and y-intercept.

Solution

(a) To find the slope, we put the equation into slope-intercept form:

General form of the equation of the line

Subtract from each side

Divide by -3 y =

x - 4

2x - 12 - 3y =-2x + 12

2 x - 3y - 12 = 0

2x - 3y - 12 = 0,

■ NOW TRY EXERCISES 41 AND 45 ■

General Form of the Equation of a Line

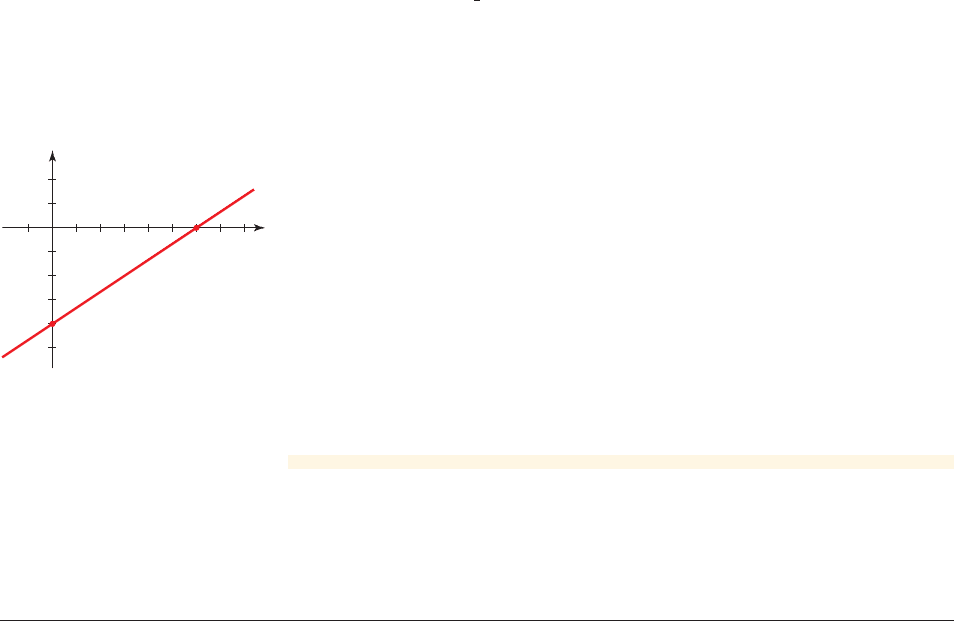
SECTION 2.3

■

Equations of Lines: Making Linear Models 173

Comparing this last equation with the slope-intercept form , we see

that the slope m is .

(b) To find the x-intercept, we replace y by 0 and solve for x.

Replace y by 0

Add 12 to each side

Divide by 2

So the x-intercept is 6.

To find the y-intercept, we replace x by 0 and solve for y.

Replace x by 0

Add 12 to each side

Divide by

So the y-intercept is .

the intercepts: and (6, 0). We plot the intercepts and sketch the line

that contains them in Figure 7.

■ NOW TRY EXERCISE 53 ■

10, - 42

- 4

- 3 y =-4

- 3y = 12

2 102- 3y - 12 = 0

x = 6

2 x = 12

2 x - 3102- 12 = 0

y = b + mx

2.3 Exercises

(0, _4)

(6, 0)

figure 7 Graph of

2x - 3y - 12 = 0

CONCEPTS

Fundamentals

1. An equation of the line with slope m and y-intercept b is . So an

equation of the line with slope 2 and y-intercept 4 is

_______.

2. An equation of the line with slope m and passing through the point is

. So an equation of the line with slope 3 passing through the

point (1, 2) is

_______.

3. (a) The slope of a horizontal line is

_______ (zero/undefined). An equation of the

horizontal line passing through (2, 3) is

_______.

(b) The slope of a vertical line is

_______ (zero/undefined). An equation of the

vertical line passing through (2, 3) is

_______.

4. To find an equation for the line passing through two points, we first find the slope

determined by these two points, then use the

_______ _______ form for the equation

of a line. The line passing through the points (2, 5) and (3, 7) has slope

_______, so

the point-slope form of its equation is

______________.

5. (a) The graph of the equation y ⫽ 5 is a

_______ (horizontal/vertical) line. The slope

of the line is

_______ (zero/undefined).

(b) The graph of the equation x ⫽ 5 is a

_______ (horizontal/vertical) line. The slope

of the line is

_______ (zero/undefined).

_______.

y -

ⵧ

1x -

ⵧ

, y

y =

ⵧ